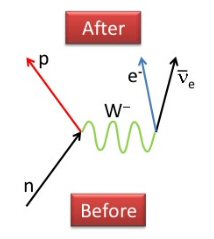
basic (n => p) beta- decay
(many radioactive isotopes decay this way)
anti-neutrino is created and comes out with the electron
(source --- http://www.slideshare.net/asober/feynman-diagrams)
A comprehensive overview of neutrino theory and detection by a retired engineer, expanded to include wimp (dark matter candidate) detection and the physics of how photons and neutrinos 'bounce off' electrons.
My related essay on 'Atoms' is here
Go to homepage
Introduction
Neutrinos and
anti-neutrinos
Wait
a minute --- How can neutrinos have anti-particles?
Chirality
is reversed
Neutrino rest
mass and speed
Neutrino oscillation
Double beta decay
Neutrino
energy - 4.4% of reactor thermal energy carried away by neutrinos
Solar neutrinos
Wide
ranging neutrino spectrum
Neutrino detection
theory
Neutrino
target overview
Beta
decay
Two
ways to detect neutrinos --- detect a new element or an emitted particle
Cowan
and Reines detect first neutrinos in 1956
Major neutrino detectors
Four
big neutrino detectors
Homestake chlorine
detector (1970 - 94)
Super
Kamiokande water cherenkov detector (1996 to present)
Sudbury heavy water neutrino
detector (1999 - 2006)
IceCube
world's biggest (ice) neutrino detector --- a neutrino telescope (2010
to present)
Kamland
mineral oil anti-neutrino detector
Halo
dectector --- another neutrino detection method
Accelerator
generated neutrinos
CERN's
Opera neutrino detector
Daya
Bay reactor anti-neutrino experiment
Dark matter particle?
Wimps
(weakly interacting massive particles)
Galactic
rotation curves are flat
Dark
matter
Unknown
particle
Possible
detection strategies
Wimp detectors
Wimps
and supersymmetry
Difficulty
of wimp detection
Wimp mass
Calculating
nucleus recoil energy from wimp 'bounce'
Liquid xenon wimp detectors
XENON1T
detector (3/21/16 update)
XENON1T
detector details
LUX
xenon wimp detector
LUX
xenon wimp detector details
ADMX
-- Axion Dark Matter Experiment (8/25/15
update)
Wimp annihilation
Photon-electron appendix
Photon/electron
interactions
Einstein
discovers light has particle-like properties
Photoelectric
effect -- particle like (low energy)
Thomson
scattering -- wave like
(medium energy)
Compton
scattering -- particle like (high energy)
Compton
scattering when incoming photon has mass energy of an electron
My
compton recoil sketches
Compton
wavelength
Electron
recoil energy for a wide range of photon energy
Compton
recoil and scintillators
Compton's
1923 x-ray scattering paper
Inverse
compton scattering
Neutrino-electron appendix
Electron
recoil from a neutrino hit
Deriving
equation for neutrino electron elastic collision
Comparison
of relativistic and classical elastic collisions
My
sketch of relatvistic elastic collision energy transfer vs mass ratio
Why
is energy transfer so efficient for electrons hit by high energy neutrinos?
Quotes
from the 1979 Noble award ceromony to Glashow, Salam and Weinberg for Electroweak
theory
Matter and anti-matter
Neutron optical
detection
Periodic
chart isotope decay modes
Neutron
decay time measurement issue
Neutrino references
What is a neutrino?
A particle physicists
will tell you a neutrino is a a very light, neutral, fermion particle (half
integer spin), sometimes called a neutral lepton, that interacts only via
the weak force with a tiny crosssection area (and gravity). It's part of
the standard model of physics. There are three basic types each paired
with a lepton of the electron family, so there is the electron neutrino,
the muon neutrino and the tau neutrino. And they have anti-particles too,
such that the electron and an electron anti-neutrino are usually generated
together. For reasons not well understood, experiments show all neutrinos
are left handed and all anti-neutrinos are right handed.
For a long time they were assumed to have zero mass and travel at the speed of light, but recent work (ongoing) shows they have a tiny mass, hence have a speed just a hair below the speed of light. Because they don't interact via the electromagnetic force they don't emit or absorb photons, hence they are invisible to light telescopes. They can however be detected by specially designed large detectors exploiting the fact that neutrinos occasionally interact with quarks (inside protons and neutrons) and electrons, both of which also respond to the weak force. The earth is continually bathed in a huge flux of neutrinos from the sun where they are emitted by the basic fusion reactions that convert hydrogen to helium.
Why an essay on neutrinos
This essay
is a comprehensive overview of neutrinos. Neutrinos are the least understood
subatomic particle, and a huge new neutrino telescope in Antarctica is
providing a new window on the universe. I always wanted to know how neutrinos
were detected, which led to understanding how photons (and neutrinos)
can 'bounce off' electrons, which led to understanding how experimenters
are planning to look for dark matter (wimps).
Neutrinos are leptons that interact with other leptons (electrons) and quarks (protons and neutrons). They can be absorbed or 'bounce off' in both cases typically bringing in high energy and momentum. A neutron or proton in a nucleus absorbing a neutrino immediately undergoes a 'beta like' change in atomic number spitting out a (high energy) electron or positron. Neutrinos that (squarely) hit and bounce off particles can transfer a lot of energy to the particles causing them to recoil strongly, in the case of electrons accelerating them to near the speed of light, in the case of deuterium splitting the nucleus.
With such a variety of interactions there's a lot of ways to detect neutrinos. In the early days neutrino detectors looked for atomic changes, detecting the small number of radioactive atoms created. These days most neutrino detection is optical. Electrons recoiling near the speed of light in a vacuum, but above the speed of light in a material (water or ice), output blue cherenkov radiation that can be picked up by phototubes lining the detector. Use of scintillating materials allows optical detection of free neutrons and gamma rays from positron-electron annihilation.
There are a bunch of different sources of neutrinos (including anti-neutrinos) that are detectable on earth. Most radioactive decay produces neutrinos, but this flux from natural sources is low and detectable only when there is a lot of mass, like the earth, or concentrated in a nuclear bomb or reactor. Most neutrinos on earth come from fusion in the sun, specifically the reactions that transform protons into neutrons for helium. 3% of the sun's fusion energy and 4% of reactor energy is radiated as neutrinos.
1) Solar
(five different reactions in proton-proton chain fusion in sun creates
electron neutrinos)
2) Atmospheric
(decay of particles created in upper atmosphere by cosmic rays)
3) Supernova
(90 - 99% of supernova energy is releases as neutrinos)
4) Deep space
(super high energy neutrinos possibly from outside our galaxy)
5) Particle acclerator (directional beams of muon neutrinos
created by smashing protons into metal target)
6) Nuclear reactor (first detection
of anti-neutrinos by Cowan and Reines from beta decay of fission products)
7) Geo-neutrinos
(decay of radioactive elements in earth crust (U238, Th232, and K40))
8) Atomic bomb
(Cowan and Reines first plan was to detect anti-neutrinos in a shallow
hole under the bomb tower!)
The largest neutrino detector in the world is IceCube in the Antarctic, a cubic km of ice with hundreds of embedded photodetectors, looking for light flashes in the ice caused by very high energy, deep space neutrinos hitting the ice's hydrogen.
Physics I learned researching neutrinos (and included
in this essay)
How feyman
diagrams for beta decay can be modified to explain neutrino detection.
How high energy
photons can cause electrons to recoil at high speed.
Deriving the
photon-electron elastic collision equation by assuming conservation of
momentum and energy (compton scattering eq).
Deriving the
energy transfer equation of neutrinos scattering (bouncing) off electrons.
How scintillators
work.
How scintillators
allow optical detection of high energy gamma photons.
How cherendov
radiation allows optical detection of high speed electrons.
How free neutrons
can be optically detected.
Which particles
neutrinos interact with and the two bacic types of interaction.
Simple view
of how neutrinos can oscillate between types.
Why neutrinos
were postulated to exist in the 1930s and how they were first detected
in 1950s.
How the major
neutrino detectors work.
How muons
travel in water/ice.
How weak force
W and Z bosons fit into the neutrino picture.
How the pp-chain
solar fusion reactions works (simple and detailed).
How the sun
generates neutrinos, and how the neutrino rate is tied to sun's energy
output and helium conversion.
Expected characteristic
of most likely dark matter particle (wimp) and plans to try and detect
it experimentally.
Calculating
recoil energy acquired by a (large) nucleus from a wimp bounce.
Mystery solved
--- How the large Japanese water cherenkov detector (Kamiokande) could
confirm missing solar neutrinos
when it detects using a scatter (bounce) reaction that some references
say works with all neutrino types.
Introduction
Only when
I came across a Scientific American article (April 2013) did I realize
that the study of neutrinos is a work in progress and much about them is
not understood. There are actually huge gaps in our understanding of neutrinos.
The Scientific American article by three researchers touched on some of
the unknowns totally new to me, and the 'neutrino' Wikipedia article confirmed
the ideas in the Scientific American article. In fact the Wikipedia article
on neutrinos is quite amazing.
A lot of engineering and money in recent years has been spent on building a new generation of huge neutrino detectors most of which are now online. They fall into two general categories: astronomical and particle physics. The astronomical detectors look for neutrinos from deep space, supernova, sun, or cosmic rays in the atmosphere. The particle physics neutrino detectors are built to detect strong fluxes of neutrinos that are generated in particle accelerators and formed into beams that travel hundreds of miles. The purpose of these detectors is to better understand the physics of neutrinos. There are three neutrino beam experiments: Fermilab, CERN and in Japan. There is also some work in using small neutrino detectors to monitor nuclear reactors.
The Scientific Article starts with an interesting 1930 quote from Pauli, who joking with his colleagues said, "I have done a terrible thing. I have postulated a particle that cannot be detected." The motivation for this was a long standing problem with beta decay where the energy and momentum of the ejected electron was found to vary with a smooth curve, so both energy and momentum conservation appeared to be violated. A couple of years later Fermi worked out a theory of beta decay and the weak force incorporating a particle like Pauli had suggested, and this defined the character of a weak force feeling particle that could carry energy and momentum, which Fermi named the neutrino. The postulated neutrino was very light and was always produced with an electron. It had no charge, so it didn't sense the electromagnetic force nor did it sense the strong force. It did however (very weakly) interact with quarks and leptons (both fermions that follow Fermi–Dirac statistics) via the (heavy) weak force carriers (W+, W- and Z). The calculated cross section was so low (around 10^-43 cm^2) that for years many physicists thought they would never be detected, but 25 years later using the high flux of neutrinos from a big nuclear reactor and a cleverly designed experiment two Los Alamos scientists were able to detect them. Detection of neutrinos is now routine.
What is really detected in all neutrino detectors is not the neutrino directly, but nuclear and/or particle reactions that it can be shown must have triggered by a neutrino 'hit'. The neutrino is either 'absorbed', causing a particle to transform, or it 'bounces off', causing a particle to recoil. The former are quark hits that cause a (single) quark conversion (up <=> dn) that results in a beta like element change as (n => p) for neutrinos, or (p => n) for anti-neutrinos. The target material is chosen so that this either results in radioactive isotopes of an adjacent element or in the case of hydrogen a (detectable) free neutron. 'Bounce' reactions are typically electron hits (to hydrogen) where the neutrino keeps going, but the hit transfers enough energy from the neutrino to the electron to accelerate it to near the speed of light, which allows it to be detected.
Hydrogen in water (and ice) is a favorite target, used in the two biggest detectors now operating (Super Kamiokande in Japan and IceCube in Antarctica). It provides a simple one proton nucleus that can be converted to a free neutron and a single electron that can be recoiled at near the speed of light. A bounce hit to the nucleus of deuterium in heavy water is able to knock free its neutron, which has the advantage that this reaction (effectively) detects not only electron neutrinos but muon and tau neutrinos too. With the use of neutron absorbing scintillators in the water both free neutrons and high speed electrons can be optically detected.
Detected how?
But what is
really measured in a neutrino detector? The answer in almost all cases
is radioactivity or light flashes. If a few atoms of an unstable isotope
are made by (n => p) or (p => n) conversions, this is measured by flushing
the tank periodically and running it by a geiger counter. The more radiation
the higher the number of atoms. One of the early large neutrino detectors,
Homestake chlorine detector, showed this method is exquisitely sensitive.
While this type of detector provides a measure of neutrino flux, it gives
no information about the direction from which they come, and very little
information about their energy. And because it requires a neutrino to be
absorbed triggering a quark conversion, it only detects one of the three
types of neutrinos, the electron neutrino.
Most of the more recent neutrino detectors use an array of phototubes to look for neutrino triggered light flashes in a transparent target material. Interestingly there are several different neutrino reactions that result in light flashes, and different reactions give a different type of light flash. In addition because neutrino triggered light flashes are monitored in real time by an array of phototubes much more information about the neutrino can be determined than with a radiation detector.
The shape and pattern of the light pulses can determine not only the energy in the neutrino, but also the type of neutrino and direction it was traveling. In some detectors a single neutrino hit can be made to create two distinct light flashes by detecting two different released particles. This provides a nearly unique signature of the neutrino reaction, allowing it to be picked out from a background of false candidates (cosmic rays, background radiation, etc), which is very useful for detecting rare events, and in fact this method was used in the very first detection of neutrinos in 1956 when an anti-neutrino absorbed by the proton in water created both a free neutron and a positron.
So what neutrino reactions create light flashes (meaning photon emissions, which can be visible light, ultraviolet, or gamma rays) that are detectable by phototubes:
a) Cherenkov radiation --- electrons traveling faster than
speed of light in a material produce a 'shock wave' light cone
emitted by the atoms of the material along the electron path. High speed
electrons can be emitted by neutrino
triggered atomic conversions, or by material electrons (mostly hydrogen)
that are sent recoiling near the speed
of light by a neutrino 'bounce' hit.
b) Scintillation --- charged particles traveling through a scintillating
material drive its electrons to higher orbits, which
then release this excess energy as a light flash as these electrons drop
down to their normal orbits.
c) Gamma rays -- gamma rays emitted can be optically detected by having
these high energy photons (impulse) hit
an electron causing it to recoil, which even if not relativistic in speed,
can still be detected by scintillators.
d) Free neutrons --- Free neutrons can be captured by the nucleus of some
atoms (cadmium) giving them excess energy,
and this can result in a flash of light as these 'neutron activated' atoms
relax, or alternately they may emit a
charged particle that triggers light flashes in a scintillator.
e) Positron annihilation --- A positron (anti-electron) comes out when
an anti-neutrino triggers a (p => n) conversion.
A positron soon hits an electron causing annihilation into a pair of 0.5
Mev (gamma) photons. Impulse hits
of gamma photons to electrons can accelerate them to moderate to high speed,
which can cause a scintillator
to flash. (see Compton scattering below)
f) Muon -- Very high energy (muon) neutrinos can create muons (heavy electrons)
that leave a long (up to a mile)
straight light path as they travel through water or ice.
A lot of materials are natural scintillators. Scintillation (emission of light) depends on the bond valence gaps of a material. If a scintillating material is also transparent, like mineral oil or (liquid) xenon, then it provides the basis for converting traveling charged particles (or even high energy photons) to light flashes. The simple picture of scintillation is this: A charged particle whizzing by atoms of the target material feels a little force from the (outer) electrons of each of these atoms causing it to lose some of its kinetic energy to them, driving the electrons of the scintillator material to higher orbits as it flys by. So a single moving charged particle can drive a lot of electrons to higher orbits causing the release of many photons (a bright flash) as they (quickly) relax. Scintillators allow the optical detection of electrons traveling too slowly to exceed the speed of light and also (in the case of Wimp detectors) the detection of the recoil of an ionized nucleus. Scintillators can be triggered by high energy photons too where (as a first step) a photon bounces off an electron knocking it free and acclerating it to a moderate to high speed via a process called compton scattering.
Scintillators have been known and used for a long time. Early atom smashers, weak alpha particle 'beams' from radium, used scintillating screens to see the individual alpha particle hits.
The electrons of scintillator materials are not (directly) excited by free neutrons because neutrons have no charge. Free neutrons can be made to cause light flashes with use of an intermediate material (a dopant), like say cadmium or lithium, added to the detector target. Cadmium has a high affinity to absorb neutrons, and after becoming 'neutron activated', it relaxes by the emission of a pair of (high energy) photons. Lithium will also absorb neutrons, and 'neutron activated' lithium is unstable emitting a charged particle (alpha particle). The charged particle emitted from the lithium, or high energy photons causing electron recoil, excite electrons in a scintillator to produce a flash.
A classic example of how neutrinos can create free neutrons is a target of water (or ice), specifically the nucleus of water's hydrogen atoms. If an anti-neutrino is absorbed by a quark in the hydrogen's nucleus causing (p => n) conversion, then obviously the chemical bond of the hydrogen (now a neutron) to the water molecule is broken, and the energized neutron is free to fly off. In this reaction a positron is created and emitted too, and it may be detected too either by its high speed travel or by the photons it releases when it annihilates with an electron.
My starting point for understanding neutrino-quark
reactions
Conservation of energy and momentum in beta decay
The best starting
point for understanding neutrino reactions is the feynman diagram of simple
beta decay (now called beta- decay). Beta decay is a form of radioactive
decay known since 1899 where a high speed electron is emitted. In beta
decay a neutron in the nucleus spontaneously 'converts' into what appears
to be a [proton + electron]. The heavy proton stays in the nucleus and
the light electron is ejected, usually at relativistic speeds (i.e. > 0.707
c). The energy for this comes from the loss of mass (via E = mc^2), since
a neutron is about 0.1% heavier than a (proton + electron), releasing 1.29
Mev. However, tests show the kinetic energy of the ejected electron on
a specific decay can vary from near zero to up to the maximum available
energy (E = neutron-proton mass difference x c^2), so the principles of
conservation of energy and momentum strongly suggest that another particle,
a neutral particle, is probably being created at the same time as the electron
and is carrying off the missing energy and momentum. This 'hard to detect'
particle is the neutrino.

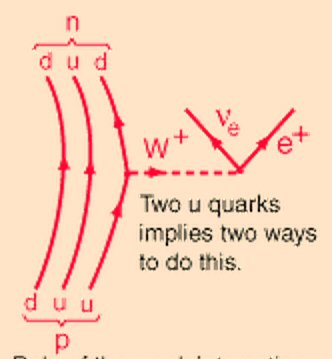
basic (n => p) beta- decay
(many radioactive isotopes decay this way)
anti-neutrino is created and comes out with the electron
(source --- http://www.slideshare.net/asober/feynman-diagrams)
Lepton# is conserved
The other key fact
to remember is that neutrinos are leptons like electrons, and experiments
show lepton# is
conserved in reactions. (At least that's the working
assumption, I have seen a paper arguing that neutrino oscillation means
lepton number may not be conserved.) Real leptons (like electron and neutrino)
are assigned a lepton# of +1 and anti-leptons (positron and anti-neutrino)
are assigned a lepton# of -1. So in beta- decay (above) to conserve lepton#
the emission of an electron (+1) also requires the emission of an anti-neutrino
(-1). Now it's easy to see how variations on beta reaction, say where the
neutrino comes in instead of out, might work and how it might lead to reactions
that could be used to detect neutrinos.
Neutrinos coming in
Take above
beta- decay (above) but replace the anti-neutrino going out with
a neutrino coming in (see below). This conserves lepton# because
a real lepton (+1) both comes in and goes out. Now instead of a neutron
spontaneously
converting to a proton, the (n => p) conversion is
triggered by
an incoming neutrino, which is 'absorbed'. This time the only particle
ejected is the electron, which carries off not only the mass difference
energy, but also the energy brought in by the captured neutrino. Note this
reaction provides two detection options: detect a free electron moving
near the speed of light (in a vacuum) or detect newly created atoms of
next higher element that is radioactive. Both techniques have been used
in neutrino detectors.
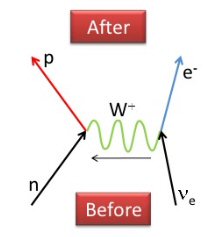
neutrino triggered (n => p) beta- like decay
increase in atomic number
neutrino comes in and is absorbed by neutron
Looking at the above figures it's easy to guess the feynman diagram for a (p => n) conversion. In this conversion the proton needs to lose it positive charge, which it can do by emitting (via W+) a positron. A positron is the electron anti-particle, so has a lepton# of -1, hence the 2nd particle emitted in the decay must be a neutrino (lepton# +1). In the same way we just did with above, the outgoing neutrino can be replaced with an incoming anti-neutrino (see below). The detection options here are the emitted high speed positron, a (radioactive) atom of the next lower element, or if the (p => n) is a hydrogen nucleus, a free neutron. Detecting both emitted particles (free neutron and positron) in the same experiment has been used to provide a clear signature of the capture of anti-neutrinos from nuclear reactors.
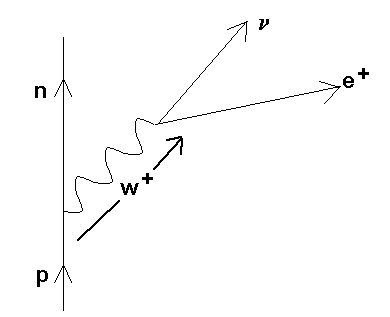 .
. 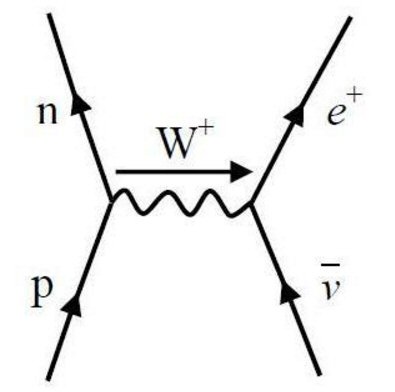
(left) beta+ decay
(right) neutrino triggered (p => n)
decrease in atomic number
anti-neutrino comes in and is absorbed by proton
(source -- https://www.studyblue.com/notes/note/n/feynman-diagrams/deck/4954056)
In fact (p => n) conversions triggered by captured anti-neutrinos in with positrons out and free neutrons out was the reaction first used to detect neutrinos in 1956. A large flux of anti-neutrinos comes from a large nuclear reactor because fission creates lots of unstable isotopes that rapidly decay via beta-.These are both 'charged current' reactions, since there is charge flow. In one case (n => p) an incoming neutrino appears to 'suck' (via W-) a negative charge from a down quark (making it an up quark) and an electron is created, or the neutrino converts to an electron if you will. In the other case (p => n) an incoming anti-neutrino appears to 'suck' (via W+) a positive charge from an up quark (making it a down quark) and a positron is created, or the anti-neutrino converts to a positron if you will.
There's another whole class of neutral current 'bounce' (or scatter) neutrino reactions where the neutrino comes in and 'hits' (via Z0) a particle transferring to it some energy and momentum, and then keeps going. The neutrino can bounce off (or scatter) an electron or the nucleus of an atom (really a quark in a neutron or proton of the nucleus). These reactions are also used in neutrino detectors. And since at energies above an Mev or so they can produce optical detectable relativistic electron recoils, they have largely replaced 'charged current' (absorption) reactions of an early generation of neutrino detectors where geiger counters were used to look for a few converted atoms.
Neutrinos
and anti-neutrinos
How
many types of neutrinos are there? Three are known, electron, muon, and
tau neutrinos, and like all other particles each of them is presumed
to
also have an 'anti' version, so three anti-neutrinos too. However, as an
early theorist showed, for there to be three distinct neutrino particles
they must be massless (and travel at the speed of light). If they have
mass, then in a sense there is only one neutrino, which is a mixture
of electron, muon, and tau types, and a neutrino should be able (presumably
if it has enough energy) to convert from one type to another. This has
now been demonstrated, and big experiments are now underway to further
explore how this mixing works.
In fact in the standard description of beta (minus) decay, like the decay of carbon 14 to nitrogen 14, out comes an electron and an electron anti-neutrino. A rule has been formulated to 'explain' this: 'lepton number' is assumed to be preserved. Neutrinos are leptons like electrons, muons, and taus, and anti-neutrinos are assigned a lepton number of -1. So a classic beta decay (now called a minus beta decay) that outputs an electron (lepton number +1) must emit an electron anti-neutrino (lepton number -1). And conversely a positive beta decay that outputs a positron (anti-electron with lepton number -1) must also emit an electron neutrino (lepton number +1). But it might be (and there are some hints) that a neutrino might be its own anti-particle, i.e. and neutrino and its anti-neutrino might be the same particle! There are experiments running now to try to figure this out. This shows how little is really known about neutrinos.
Wait
a minute --- How can neutrinos have anti-particles?
The very first
sentence in Wikedia's 'Antiparticle' is, "Corresponding to most kinds
of particles, there is an associated antiparticle with the same mass and
opposite charge (including electric charge)." But aren't neutrinos chargeless?
(Maybe the physists are going to tell me the neutrino has some some weird
kind of 'weak' charge. And from this definition, what is the anti particle
of a neutron? Ok, the answer to the neutron is simple. It is made up of
quarks, all of which have charge and an anti-neutron just has the charge
of all its quarks reversed, so they still add to zero. So what is the anti-particle
of a (chargeless) photon? Here the physicists play word games and say the
photon is its own anti-particle, whereas the skeptic might say it doesn't
have an anti-particle!
So far the big article in Wikipedia on standard model particles and force carriers has provided no info on what is reversed in an anti-neutrino.
Anti-particle word gameChirality is reversed
Ok, found this. The neutrino article says what's reversed in an anti-neutrino is chirality. Well that clears things up! And just how is this consist with the general description that anti-particles have same mass and spin, but opposite charge? Just another example of how weird neutrinos are?Digging into chirality I find this: "In geometry, a figure is chiral (and said to have chirality) if it is not identical to its mirror image", and because a neutrino has mass, its chirality is not the same as its helicity. "The helicity of a particle is right-handed if the direction of its spin is the same as the direction of its motion. ... Helicity may be thought of as 'apparent chirality'". Well that is sure clear. (Of course, all of this confusion is an attempt to put into english what is mathematically probably some sort of matrix/group transformation.)
Neutrino
rest mass and speed
Current rest mass
estimate of neutrinos (by one estimate) is around 1 ev (0.2 ev to 2 ev).
Wikipedia (neutrino) says an analysis of the cosmic background radiation
puts an upper limit on the mass of all neutrino types combined at
< 0.3 ev. Since for any particle its speed goes relativistic (> .707c)
when its total energy exceeds x1.41 its rest mass energy, for 1 kev neutrinos
this means a travel speed of around one part per million less than the
speed of light in a vacuum, for 1 Mev neutrinos the deviation is more like
one part in a trillion, and for 1 Gev a million-trillion (10^-18).
In the 1987 supernova (1987A) the neutrinos arived about three hours before the light. There are reasons why the visible light might be delayed in a supernova. This supernova was in the large Magellanic cloud 157k light years away. I calculate the travel time from there at the speed of light to be about 5 x 10^12 seconds, so the max deviation from the speed of light is (3 hr x 3,600 sec per hr/5 x 10^12 sec) = 2 x 10-7. The energy of the detected 1987 supernova neutrinos ranged from 7.5 Mev (lower limit of the Kamiokande detector) to 35 Mev, and is taken as evidence that neutrino deviation from the speed of light is less than one part per billion (< 10^-9).
Neutrinos mass estimates
Wikipedia
(neutrino) gives the mass estimate for the three types of neutrinos shown
below. Note the 'less than', these are really upper mass limits.
These should be taken with a grain of salt, especially the large muon and
tau neutrino mass limits, as recent cosmological work is pushing the mass
limit much, much lower (see below). Obviously there a conflict here, and
for the muon and tau mass a big one, since other mass estimates based on
atronomical and/or neutrino oscillation hint at a rest mass of <1 ev
for all neutrino types.
ve (electron)
< 2.2 ev
vu (muon)
< 170 kev
vtau (tau)
< 15.5 Mev
For comparison an electron has a rest mass of 0.511 Mev, so the common solar electron neutrinos are about a million times (!) lighter than an electron. The other two neutrino types could possibly be much heavier with the muon neutrino mass approaching that of an electron, and a tau neutrino could be as much as x30 heavier than an electron.
New lower mass limitsNeutrino oscillation
The same Wikipedia article reports a 2006 analysis of the cosmic background radiation puts an upper limit on the mass of all three neutrino types combined at < 0.3 ev, and two more recent experiments got almost the same limit. Oscillation experiments are generating limits on the mass difference between neutrino types (squared). Wikipedia (neutrino) says the oscillation delta (m^2) data can be interpreted that (at least) one neutrino type must have mass 0.05 ev or larger.Thus the available data tentatively puts an upper limit of 0.3 ev (0.28 and 0.23 from two other experiments) for all three types summed and a lower limit for one type of 0.05 ev.
Nobel lecture (dec 2015)
Takaaki Kajita, developer of Super Kamiokande water detector in Japan, was awarded the Nobel prize in physics in 2015 for detecting neutrino oscillation. In his Dec 2015 Nobel lecture he says the neutrino oscillations have shown the heaviest mass for a neutrino is approximately 10^7 times smaller than the electron mass. Since the electron mass is .511 mev (5 x 10^5 ev), this says the heaviest neutrino has a mass of about 0.05 ev.
The picture is a neutrino created as an electron neutrino as it travels will 'convert' into say a muon neutrino and with more travel converts back to an electron neutrino in a repeating pattern, hence the word oscillation. The distances involved for a change are not all that great, much less than the size of the earth. Muon neutrinos produced in the atmosphere are observed to change before they get to detectors. The neutrino beam experiments built to study neutrino oscillation generally have the generator and detector a few hundred miles apart, and they see flavor changes.
Theory
A simple explanation
for how neutrinos can can be found on some neutrino web sites (Sudbury).
Subatomic particles mathematically are represented by wavefunctions (complex
numbers) that represents the spread out nature of the particle. The probability
of finding a particle in its spread out area is obtained by squaring
the amplitude of the wavefunction. A key thing about wavefunctions is that
they can be added (technically 'superposition')
A Sudbury power point talk on neutrinos says this about neutrino oscillation: "What if neutrinos were made up of two different quantum waves with different wavelengths? These waves would interfere." Next to this text is a scope picture that shows two slightly different frequency (equal amplitude) sinewaves added. This produces kind of a beat, a lower (beat) frequency sinewave envelope that modulates the height of the original sinewaves. "Electron neutrinos (from sun) disappear depending on distance and mass difference of the neutrinos."
Beat frequencies are something EE engineers are familiar with. Two slightly different frequency, equal amplitude sinewave added and the sum displayed on an oscilloscope will show a low frequency envelope at the 'beat' frequency increasing and decreasing the height of the sinewave cycles. This envelope is easy to explain. When positives half cycles from one input overlaps a positive half cycle from the other input the combined signal is doubled. When positive and negative half cycles overlap, they cancel each other, so the result is zero. So the picture is a neutrino seems to be made up of two, or more likely three, wavefunctions very close in frequency. At a distance where their 'beat' summation produces a null for an electron neutrino, when squared the probability comes out near zero, no electron neutrino is found. And presumable the math shows that when one type of neutrino wavefunction nulls, the wavefunction one of the other type of neutrinos grows. Hence when this combined triple wavefunction is squared, the probability of finding a particular neutrino types comes and goes with distance.
Standard model wrong
The key thing about
neutrino oscillation is it has provided experimental proof that the standard
model of phyics was WRONG. Until about ten years ago the standard model
of physics, the bible of particle physics, had assigned a value of zero
to
the mass of neutrinos (making them speed of light particles) because from
experiments going back to the 30s the upper limit on neutrino mass was
known to be very, very low. But an Italian theorist in the 50s, Bruno Pontecorvo,
at one time an assistant of Fermi, showed that if neutrino oscillated between
types (electron, muon, tau) as they traveled, then they could not be massless,
speed of light particles. The mass of neutrinos is still not known with
any accuracy, but it looks like it might be in the range of one millionth
the mass of an electron.
History
Early radio-chemical
neutrino detectors, which captured neutrinos using a neutron to proton
conversion (n => p) in the nucleus of a molecule, were only sensitive to
electron neutrinos. All these early solar detectors, built using different
techniques, found only about 30% to 60% of the electron neutrinos that
the sun was calculated to be emitting. One possible explanation
for this was neutrino oscillation, but it was not proof of oscillation.
The Japanese Kamiokande people say it was their large water cherenkov real
time detector that provided the conclusive proof for oscillation. I don't
know the basis for this except that Kamiokande could measure the neutrino
direction of travel, so it confimed that the neutrinos being detected must
be solar neutrinos because they were coming from the direction of the sun.
The Canadian Sudbury people say their heavy water detector provided proof of neutrino oscillation in 2001. Sudbury was unique in using heavy water as a target, and this gave it an advantage: two modes of operation. If it was setup to sense electrons released by an (n => p) conversion, it was detecting only electron neutrinos, but alternately it could be setup to sense free neutrons released by a 'hit' of a neutrino to the deuterium nucleus, and in this mode it was detecting all three types of neutrinos. The first mode, electron neutrino mode, detected only about 1/3rd as many neutrinos as calculated, but the second, scatter mode, yielded a x3 times higher count making the detected and calculated numbers agree. This was strong evidence says Wikipedia (neutrino oscillation) that neutrino oscillation was real. Neutrino oscillation is now well accepted and is currently being studied in detail by the neutrino particle accelerator experiments.
Two types of oscillation
This gets
a little deep in the theoretical weeds, but it turns out there are two
types of neutrino oscillation. While neutrinos can travel through huge
volumes of materials without being absorbed, it turns out that they are
slightly affected by the material. The analogy is given that this is something
like light being affected when traveling through a transparent material,
it travels more slowly than it does in a vacuum.
The effect of materials on neutrinos is called the MSW effect (with its own Wikipedia page). It's a scattering of the electron neutrinos off electrons in the material that that raises the effective mass of the neutrinos. Since it says, "neutrino oscillations depend upon the squared mass difference of the neutrinos", neutrino oscilation is affected by travel through matter. The calculation is that the oscillation of the highest energy solar neutrinos is actually occuring within the sun itself due to the MSW effect, and this is a different mechanism from predicted neutrino oscillation that can occur during travel through a vacuum.
Wikipedia (neutrino) gives these as the (best) delta mass squared. There are three of these mass squared differences between the three neutrino flavor types.
m^2 (21) = 0.000079 ev^2
m^2 (32) = 0.0027 ev^2
m^2 (13) = ev^2
Muon neutrino beam experiments
Three large scale
experiments are currently under way to further examine neutrino oscillation.
All three use a particle accelerator to generate a stong beam of muon
neutrinos
that is aimed at a large detector hundreds of miles away. Two of the detectors
are looking to detect an oscillation of the muon neutrinos to electron
neutrinos, and one (Femilab) is looking to detect an oscillation of the
muon neutrinos to tau neutrinos. These experiments have not been running
very long, but one or two transistion events have been reported.
Oscillation mass difference limitsChirality
Neutrino oscillation depends on the mass difference between neutrino types and as data comes in the limits on mass difference are tightening. I may not understand this, but Kamland experiment reports m^2 = 8 x 10^-5, which IF m^2 is a mass difference, would imply the mass difference between electron neutrinos and some other type is only 0.009 ev, or about 3% for neutrino masses in the 0.300 ev range.
Do neutrino and antineutrino annihilate?
I have had
trouble figuring out the feynman diagram for double neutrino detection.
Most sources, like Hyperphysics show only an equation. When I draw it,
I see a potential neutrino and anti-neutrino interaction. If they cancel,
then I get the equation, But what really happens when a neutrino interacts
with an anti-neutrino (of same type). Is this a classic annihilation producing
photons? I did a google search and came up with this from a "lecturer in
physics"
What happens in neutrino antineutrino annihilation?Ok, so we first get a Z particle. This makes sense. In a typical matter anti-matter interaction the charges are opposite (by definition) and so cancel, so I suppose for neutrinos chirality might cancel too. (Wikipedia does seems to show that Z can decay into neutrino and anti-neutrino.) And an output of 'particle/antiparticle pair' is consistent with two photons, since a photon is its own anti-particle. But later the author implies the (likely) output would be electron/positron pair or more neutrinos, and I have yet to see photons shown as an output.
"The two particles meet at a single point and annihilate each other, producing a virtual Z boson, which is the neutral (i.e. no electric charge) carrier of the weak nuclear force. This Z boson then immediately decays to produce another particle/antiparticle pair, either a new pair of neutrinos, two charged leptons, or a quark/antiquark pair. What you can produce depends on how much energy there is from the colliding neutrinos."
More searching finds papers showing that neutrinos and antii-neutrinos do in principle annihilate, but in practice almost never. The cross-section is so low that the only place this really occurs is around black holes or in supernova.
Double beta decay
In a few unstable,
neutron heavy isotopes like germanium 76 the binding energies (masses)
forbid a simple beta decay (atomic number going up one), but allow atomic
number to go up by two. The reason germanium 76 cannot beta decay
to the next higher element (arsenic) is that arsenic 76's atomic weight
is higher, so without the input of external energy this decay is forbidden.
I checked the atomic weights on Wikipedia, and this is right, the atomic
weight of arsenic 76 is just a hair higher than germanium 76:
#32 germanium 76
75.9214026
#33 arsenic 76
75.922394 heavier (decay disallowed)
# 34 selenium 76
75.9192136 lighter (decay allowed)
This is a relatively unusual occurrance in the periodic table, so of the hundreds of isotopes only about a dozen (possible) double beta decays are known. Germanium 76 isotope is only about 7% of naturally occuring germanium, and I am sure this is very important to the double beta decay experiment. A paper says the probability of a double beta decay is much less than a normal (single) beta decay, and Wikipedia gives the (double beta decay) half life of germanium 76 as 2 x 10^21 years, a very, very low decay rate. In fact I read that the decay rate of germanium 76 is so low that many references call it stable, but double decays of it have been detected since the 1990s.
Hence when germanium 76 decays it is always a double beta decay. The test experimentally is whether it ever decays without neutrinos being emitted. The figure below (top) shows a typical (single) beta decay (carbon 14) and (bot) one possible way germanium 76 could decay. This decay mode would be the least interesting, two (normal) single beta decays happening at exactly the same time, i.e. two neutrons in germanium 76 would convert to protons (n => p) simultaneously each throwing off an anti-neutrino.
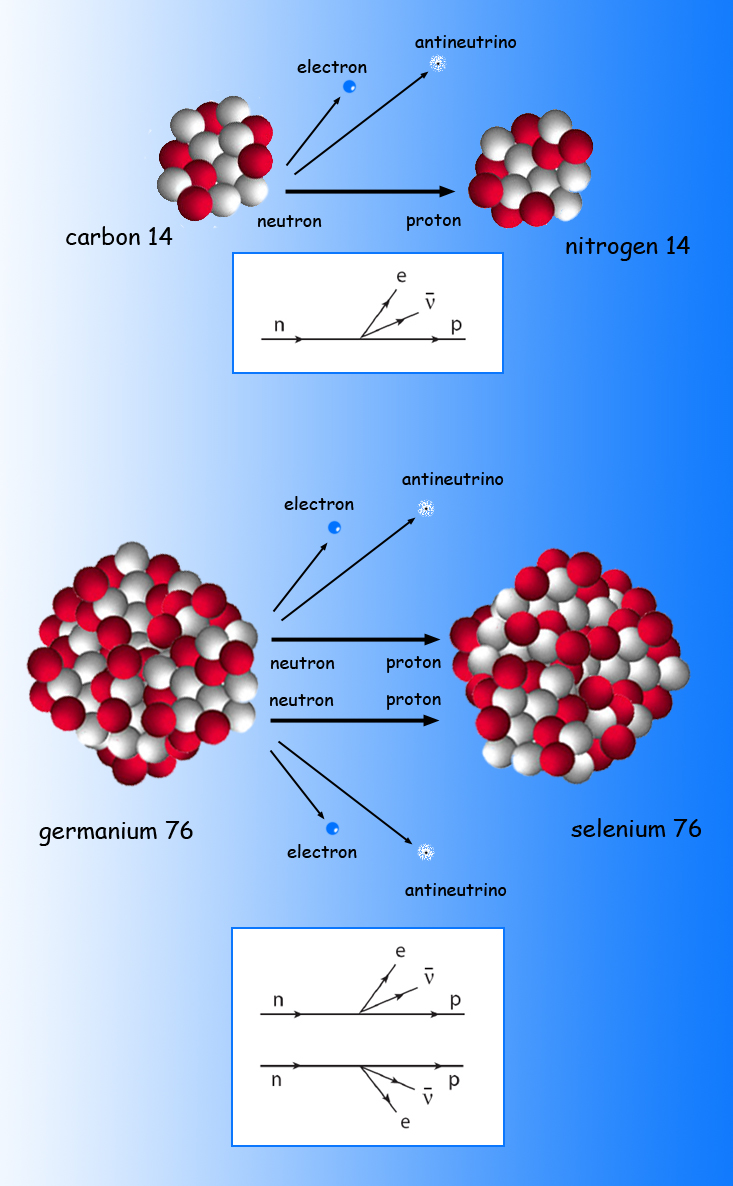
(top) normal beta- decay, atomic # +1
(bottom) double beta- decay, atomic # +2
(source --- http://newscenter.lbl.gov/wp-content/uploads/single-and-double-beta-decays.jpg)
The other way germanium 76 could potentially double beta decay, without emission of neutrinos, is shown in a figure a little further down. If this decay mode could be confirmed experimentally, it would be quite interesting, because it would appear to show that two seemingly identical neutrinos have somehow canceled each other.
MAJORANA double beta decay experiment
Because double
beta decay of germanium 76 is so rare, the Majorana experiment proposes
to use a metric ton of germanium enriched to 86% germanium 76, and it appears
to be in the form of germanium diodes. (Wow) As I do the math, this is
about 10,000 moles of germanium (76 grams/mole), which at 6 x 10^23 atoms/mole
means the experiment has 6 x 10^27 atoms of germanium 76. Dividing this
by 1.3 x 10^21 (their number for half life) means that about 5 x 10^6 decays
per year, and since there are about 31 million seconds in a year the expected
decay rate is about one atom every 6 (or is that 12) seconds. However,
since I read it is germanium diodes doing the detecting, it might
be that only a small fraction of the germanium (active region of the diode
where the E field is) that might be doing the actual detecting.
Berkley labs running Majorana says they have been working with germanium detector diodes for years. They are going to have a reactor in russia do the enrichment and work with a commercial vendor to make the diodes. Finally the detection strategy is explained:The first stage of this experiment is the 'Majorana Demonstrator' which will only use 40 kg of germanium. It's purpose is to show that they have the background 'noise' low enough to justify building a large detector. Wikipedia adds that a secondary goal of the experiment is wimp detection. My guess is the sensitive low noise current detectors are also be used to look for a (larger) 'ionization spike' from a wimp hit to a germanium nucleus knocking electrons free."Such an event (neutrinoless double beta decay) would be easy to identify, because the energy of its two electrons would add to precisely 2.039 million electron volts (2.039 MeV), and none would be shared with antineutrinos." Later they go on to say, "With a low enough background, MAJORANA will be able to easily separate the sharp spike of a 2.039 MeV two-electron event from a broad smear of energies shared among four different particles."So here it is, the key to the experiment. Unlike a summary I read elsewhere the guys running the experiment say what is being detected, to determine that there are no neutrinos emitted, is that the (known) energy released in double beta decay all goes into the electrons. But wait, isn't this a current pulse consisting of two electrons? Yikes!!
http://newscenter.lbl.gov/feature-stories/2012/05/16/majorana-demonstrator/Easy, really? I'm think there's a lot of PR puffery in the above....Ok, the later text indicates that a key to doing this is that it is a two electron 'spike', and they say this will be "easy" to separate from the background. Seems to me the key to getting this detector to be able to detect a two electron pulse must involve very low temperature and quiet materials so that the background 'leakage' current breaks up into a series of single electrons, with the probability of two electrons coming together is negligibly small. So I guess what they are they going to see for a signal (assuming background is rejected) is small/variable height pulses from a double beta decay with neutrinos, and what they are looking for is a few 'full' height pulses? Wiki page on the experiment adds that they are using a "point contact" diode and that its small contact area will help separate out a two electron spike.
'Point contact' germanium for wimps too
These same, or related, 'point contract' gemanium detectors are also being used to search for wimps. My wimp reference explains the value of the 'point contact' design is that its recoil detection threshold is very low, sub 1 kev, which is important for a wimp search where as wide a range of recoils as possible needs to be probed.
Detecting the energy of two electrons!
A double beta decay
creates two electrons. The Majorana site says germanium is perfect not
only as a source of the decay but also for detection in that it conducts
current easily so the electrons can be detected. Really? Two electrons?
The germanium must be awfully cold (-100C, actually not that cold) because
germanium has nasty leakage at room temperature and was abandoned for electronics
as soon as good silicon transistors became available. Reading along further
it says it is the 'pulse shape' that "will clearly distinguish background
events from a neutrinoless double-beta decay".
For some perspective on detecting the current output by a single atom undergoing a double beta decay consider this. Very low leakage across an insulator (germanium is a semi-conductor) in electronics at room temperature is in the range of 1 femtoamp (10^-15A), but an amp in round numbers is 10^19 electrons per second. So the extremely tiny current of 1 femtoamp in terms of electrons is the flow of 10,000 electrons per sec! This experiment is trying to reliably detect a current 'pulse' of two electrons.
But the detection problem is much worse than this. All double beta decays output two electrons as two neutrons change to protons. Detecting a two electron 'current pulse' only tells you that one atom has (likely) undergone a double beta decay, but it does not tell you if neutrinos were emitted or not.
The success of the experiment depends on being able to reliably detect the energy (or power pulse) carried by two electron current pulse. The energy, of course, is the kinetic energy carried by the ejected electrons, so you can think of it as determining the speed at which they are ejected. Only by confirming that all the energy released by mass loss of two neutrons converting to protons is being carried away totally by the two emitted electrons can it be confirmed that there is no energy left over for neutrinos. In spite of the happy talk in the newsletter that this should be easy and clear, I suspect it is going to be very difficult. And I think this is confirmed by the fact that as a first stage in the experiment they are only going to build and install a few diodes to see if they can get the noise level low enough.
Thinking about this I suspect one of their key discrimination tools will be a very short time window. The two electrons they are looking for will be emitted at the same spot in the crystal with exactly the same speed (though is what directions?). Use of a point contact diode probably means the time to drift the short distance to where they are collected is small giving them little time to separate, so they should arrive near simultaneously. If this time window is tight enough, they can probably drastically reduce the sensitivity to the low background leakage current, which should thin out into a stream of (mostly) individual electrons (with random spacings).
I saw one mention that this experiment is looking for a time difference. Maybe this is a hint that if neutrinos are coming out, meanng the energy is (randomly) shared with the electrons, the two electrons in any specific decay will come of the atom with different speeds. Maybe with the right choice of drift voltage they can thus be separated a little so they arrive at slightly different times. In this scenario the experimentors would be looking for tight double height pulse, rather than a lower more spread out pulse. Their press release did says they were looking for a 'sharp' pulse.
Do the neutrino and anti-neutrinos cancel?
The Scientific
American article says in double beta decay the neutrino and anti-neutrino
'cancel out'. Wikipedia ('Double beta decay') puts it this way: "In
essence the two neutrinos annihilate each other, or equivalently, one nucleon
absorbs the neutrino emitted by another nucleon of the nucleus". Wikipedia
('Majorana fermion') says, "Neutrinoless double beta decay, which can be
viewed as two beta decay events with the produced antineutrinos immediately
annihilating with one another, is only possible if neutrinos are their
own antiparticles."
Two viewpoints
Below left
is a figure showing one possibility for double beta decay with no neutrinos
coming out. On top (n = > p) decay emits an anti-neutrino along with an
electron as is normal conserving lepton number, but shazam (!) before the
anti-neutrino can fly away, it immediately and somewhat mysteriously
'flips' to a neutrino that is absorbed by a different neutron in the same
nucleus (bottom) triggering it to also (n = > p) convert. The lower reaction
need only emit an electron to conserve lepton number since this conversion
was (in effect) triggered by a neutrino coming in and being absorbed. Summarizing:
In this picture an anti-neutrino comes out during (beta) decay of neutron
#1, immediately 'flips' to a neutrino, is absorbed by neutron #2 triggering
its conversion to a proton.
Below right from Wikipedia ('Double beta decay') is a slightly different (and simpler) view point. Here there is no neutrino 'flipping'. The neutrino emitted and absorbed are treated as same particle. In other words there is no neutrino and anti-neutrino there is just a neutrino (ve), which is how the figure right is labelled.
The Wikipedia ('Double beta decay') text under the figure below (right) is, "Feynman diagram of neutrinoless double beta decay, with two neutrons decaying to two protons. The only emitted products in this process are two electrons, which can occur if the neutrino and antineutrino are the same particle (i.e. Majorana neutrinos) so the same neutrino can be emitted and absorbed within the nucleus (virtual particles). In conventional double beta decay, two antineutrinos — one arising from each W vertex — are emitted from the nucleus, in addition to the two electrons. The detection of neutrinoless double beta decay is thus a sensitive test of whether neutrinos are majorana particles."
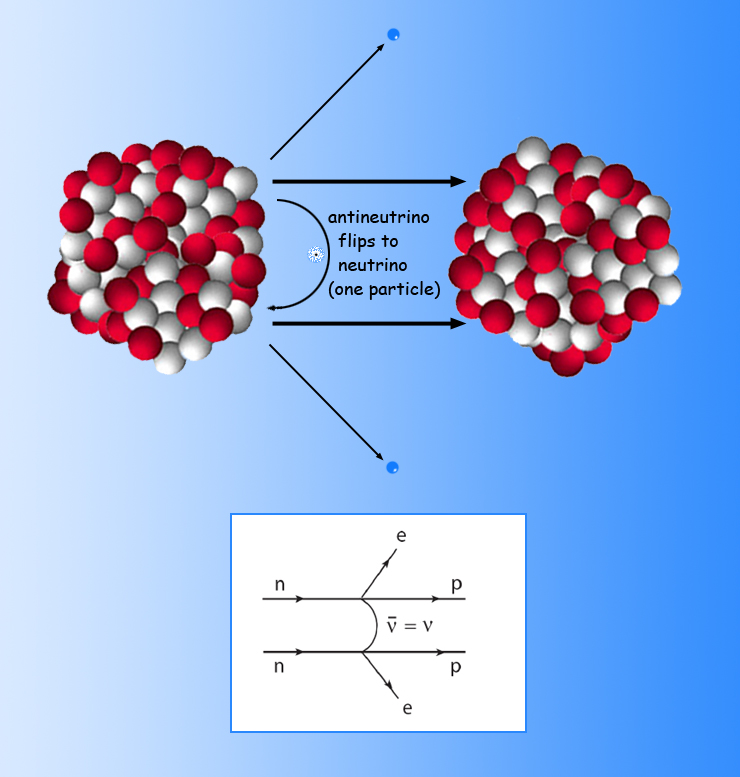 .
. 
neutrinoless double beta decay
(source (left) --- http://newscenter.lbl.gov/wp-content/uploads/single-and-double-beta-decays.jpg)
(source (right) --- https://en.wikipedia.org/wiki/Double_beta_decay#Neutrinoless_double_beta_decay)
The neutrinoless double beta decay (in figure above left), whose existence would violate lepton number conservation, is described in the MAJORANA newsletter this way:
"A diagram of neutrinoless double-beta decay shows a right-handed antineutrino emitted when a neutron decays (also emitting an electron). The antineutrino flips its handedness and is absorbed by a second neutron, which also decays (and emits a second electron). Only a single antiparticle/particle is involved. How fast it can flip its handedness depends on its mass: the more massive, the easier the flip, and the more often this kind of decay will occur."The last sentence indicates that the experiment has the potential to give information about the mass of neutrinos, because the mass affects the probability of the flip. This should be indicated by how many of the detected double beta decay current spikes are full height.
Also note the above description is describing two normal reactions, one after the other, separated by a new phenomena in between, a flip of the neutrino chirility. The first reaction is a normal beta- decay (an electron and anti-neutrino emitted), and after the flip, a second reaction that is a standard neutrino sensing reaction (an incoming neutrino absorbed by a neutron triggering conversion to a proton with an electron emitted).
Cancelling?
To me it doesn't
make sense in this experiment to view the two neutrinos as cancelling.
Normally when a particle and its anti-particle meet and annihilate, the
sum of their energies is carried off by photons, or in the case of neutrinos
by other leptons. If this experiment does demonstrate that neutrinos are
majorana particles (a particle is its own anti-particle), what they expect
to find is that all the energy released by the mass loss goes into
the electrons and atomic recoils with zero energy going into the
neutrinos. Since the neutrinos in double beta decay are identified by Wikipedia
('Double beta decay') as 'virtual particles', maybe this is by definition.
It does seem reasonable that only real particles can carry off energy.
--------------------------------------
Neutrino
energy - 4.4% of reactor thermal energy carried away by neutrinos!
Here's a fascinating
engineering perspective on neutrino energy. Fission power reactors produce
neutron rich fragments that quickly decay via beta (minus), so reactors
are a major source of anti-neutrinos. Wikipedia gives these numbers for
a large (1.3 Gw) power reactor: thermal power or core heat 4.0 Gw, yields
electrical power 1.3 Gw, but the "total power production from fissioning
atoms" is actually 4.185 Gw (4.4% higher). 185 Mw from fission goes missing,
it does
not heat the core. The reason is the power flys out
of the reactor carried by the anti-neutrinos. A typical large power reactor
is pumping (and losing) 185 million watts into neutrinos all the
time! Amazing, never before have I seen this feature of a reactor mentioned.
185 million watts is a shitload of power!
It falls directly out of a typical fission reaction which yields 200 Mev of energy (for perspective the rest mass of an electron is 0.5 Mev and a proton 1 Gev) of which with 4.5% or 9 Mev is carried away by a single (?) anti-neutrino. (Later the same Wiki article says only 3% of reactor anti-neutrinos have energy above a detection threshold of 1.8 Mev, so I don't understand the apparent contradiction. Maybe it is this simple, there are several daughter beta decays for each uranium split, so the 9 Mev is divided among several neutrinos.)
In a paper I found numbers about neutrinos from the Savannah reactor that Cowan and Reines used to first detect anti-neutrinos: reactor is outputting 2.3 x 10^20 anti-neutrinos per sec and the reactor neutrinos have energy less than 10 Mev. Cowans and Reines had a flux of about 10^13 per cm^2 per sec.In the sun 3% of the fusion energy of the sun is carried away by solar neutrinos. In the sun for every helium created by fusion two protons (hydrogen) must convert to neutrons. A (p => n) conversion in the core of the sun is essentially a thermally driven beta+ reaction that also outputs a positron and (electron) neutrino.Check: If I use 5 Mev (obviously carefully picked!) for energy of each anti-neutrino, everything checks nicely. [2.3 x 10^20 anti-neutrinos/sec x 0.5 x 10^7 ev/neutrino x 1.6 x 10^-19 joule/ev) = 1.85 x 10^8 watts (185 million watts)]
How many neutrinos do we get from the sun? A lot.... Each sq cm facing the sun on earth has 65 billion neutrinos flying through it every second!
And the reason the sun makes so many neutrinos is simple. Sun 'burns' hydrogen to helium, helium requires neutrons, neutrons are made by protons 'throwing off' their positive charge (as anti-electrons), and to preserve lepton balance this requires electron neutrinos be thrown off too, one neutrino for every (p => n) conversion, two neutrinos for every atom of helium created.
There is more than one fusion process that powers stars, but in the sun the bulk of the energy output comes from the proton-proton chain (below). Step 1 in the pp chain is (what looks like a standard) proton to neutron conversion (p => n) that outputs a positron and an electron neutrino combined with (or followed by?) a pairing of the neutron with a proton (pn, held together by strong force). Step 2 another proton joins the nucleus to make an He3 nucleus (with energy released as a gamma photon). In step 3 two He3 join together spitting out two of the protons. The net result is four protons have combined into one helium nucleus plus some energy and two electron neutrinos emitted.
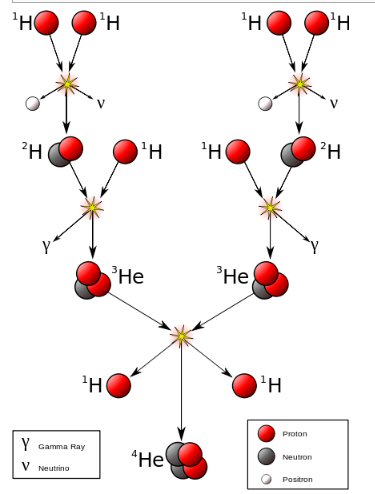 .
. 
Solar proton-proton fusion chain
sources: Wikipedia (left) Hyperphysics (right)
Since neutrons weight more than protons, where does the energy come from? From the fact that there is a binding energy in a multiple particle nucleus too and as the numbers below show a helium nucleus weighs just a tiny bit less (0.69%) than the four protons that go into it, leaving energy left over for the neutrinos and for high kinetic energy to drive protons close enough to continue the reaction.
proton mass .938272 Gev/c^2
4 x proton mass
3.753088 Gev/c^2
helium nucleus mass
3.727379 Gev/c^2
-------------------------------------------------
mass loss 0.025709
Gev/c^2
(0.69%)
The above diagram shows the neutrino output from the sun is directly related to its energy output, which can be accurately measured. In the pp chain a neutrino is emitted for each proton that converts to a neutron, or put another way two electron neutrinos emitted for every helium nucleus created. The reaction emitting the neutrinos is a simple one, either the same (or a variant) of the well characterized beta+ reaction.
Numbers add up!PP chain details
The numbers above show each helium created is associated with 25.7 Mev of mass conversion to energy, and the reaction chain creating each helium outputs two electron neutrinos, one for each proton converted to a neutron. I read in a neutrino book that about 3% of the sun's fusion energy is released in the form of neutrinos. Do these numbers add up? Yes they do. 3% of 25.7 Mev energy released per helium is roughly 800 kev available to be shared by the two neutrinos that are emitted per helium, or 400 kev/neutrino. How does this compare with the actual solar neutrino energy spectrum? Very nicely! (see below)While most solar neutrinos that get detected are of higher energy that is the result of the detection thresholds of nearly all neutrino detectors being in the Mev range. As the solar neutrino spectrum below shows most (92%?) solar neutrinos have a broad energy spectrum (consistent with a beta+ reaction, where energy is shared by the neutrino and the positron) with an upper of limit of (wait for it) about 400 kev. Checks very nicely. (I didn't get this from any reference, I pieced it together myself.) This dominant pp chain neutrino spectrum is in the upper left corner of the spectrum below. This is most of the solar neutrinos, and note the spectrum is smooth and falls off a cliff just above 400 kev.
All this detail about the pp chain is very important when it comes to understanding how the various solar neutrino detectors work, because most neutrino detectors that have been built do not detect the neutrinos from the primary path. The reason is that the energy of these neutrinos is low, 400 kev max, so they are more difficult to detect. The big water/ice cherenkov detectors (Super Kamiokande, IceCube) have a minimum detection threshold of several Mev, so they can only detect neutrinos from some of the alternate pathways of the pp chain. They primarily detect the continuous 8B neutrinos, which result from a sort of beta decay (p => n, boron #5 => beryllium #4).
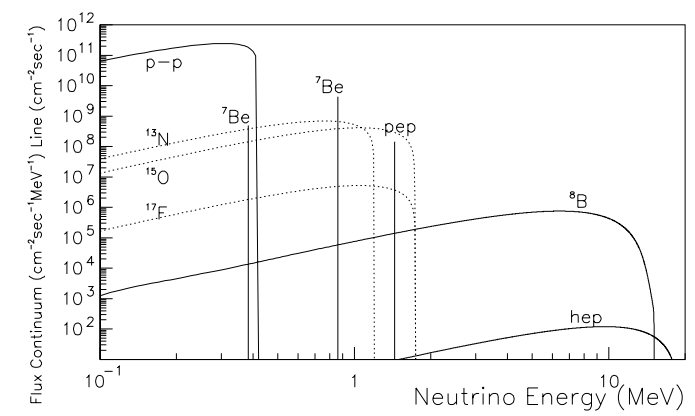 .
. 
Solar neutrino fluxes --- pp chain (solid lines)
(dotted lines are CNO neutrinos, which is less than
2% of total)
right -- superimposes the neutrino detection threshold
of the major neutrino detectors
(source left -- http://www.slac.stanford.edu/econf/C990809/docs/suzuki.pdf)
(source right -- screen capture from 2002 Davis Nobel
lecture)
The Slac neutrino report identifies five different neutrino reactions in the pp chain. They are plotted with solid lines in the above solar neutrino flux chart from the same report. Notice three (p-p, 8B, hep) have a continuous spectrum and two (7 Be and pep) an impulse spectrum. The five pp chain equation from the Slac report are below, but I rearranged them to bring out the underlying pattern.
Continuous
p-p
p + p => d + e+ + ve
(Ev < 412 kev)
(p => n) (beta+ type reaction)
8B
B8 => Be8 + e+ + ve
(Ev < 15 Mev)
(p => n) (boron #5 => beryllium #4)
hep
He3 + p => He4 + e+ + ve
(Ev < 19 Mev)
(p => n)
Impulse
7Be
e- + Be7 => Li7 + ve
(Ev = 380 kev, 860 kev) (p => n) (beryllium
#4 => lithium #3)
pep
e- + p + p => d + ve
(Ev = 1.44 Mev)
(p => n)

complete solar pp chain with all five neutrino generating
reactions (red)
The pattern is clear. Each reaction is a (proton => neutron) conversion with the output of an electron neutrino. In all cases the lepton # is preserved. Each reaction is at root the same quark conversion (p => n). For charge balance this requires either a (single) positron coming out or an electron coming in, and lepton # preservation requires the reaction include a neutrino too, which in all these cases is the creation of an electron neutrino.
The continuous spectra are sort of a beta decay with emission of a (positron + electron neutrino) and like beta decay the energy split between them varies, which is the source of the continuous neutrino energy spectrum. In the two impulses cases the output is a nucleus and an electron neutrino. In these cases the lepton # is preserved by an electron coming in. (I can see there is only a single lepton output in these two cases, but why exactly this generates an impulse spectrum I don't know. After thinking about it, I suspect it's because the sun's core temperature is quite constant, so the thermal energies of the inputs are relatively constant.)
Impulse is due to (p => n) electron capture? (update 12/6/13)Solar efficiency
I later realized that the two solar impulse reactions (above) look just like, and so probably are (yup, Be7 decays by electron capture), an isotope decay reaction known as 'electron capture', a variant on beta+ decay. In electron capture an electron comes in and triggers a (p => n) with only a neutrino emitted, just as in the equations above. And the interesting thing is that Wikipedia says the energy of the neutrino in this reaction is always 'mono-energetic', that is it is the same every time, it's an impulse. (Of course, the solar reaction is not really 'electron capture'. An electron may be acquired to trigger the reaction, but it's a plasma electron not an electron orbiting the nucleus as on earth. There ain't no atoms in the core of the sun!)
Stars and supernovas
An estimated
3% of the fusion energy of the sun is carried away by solar neutrinos.
The dominant path of the pp-chain shows that a neutrino is generated when
a solar proton converts to a neutron in what is essentially a thermally
driven beta+ reaction. Since there are two neutrons in helium, the sun
outputs two neutrinos for every helium nucleus created by fusion. And because
the neutrinos come from a beta+ like reaction where a positron-neutrino
pair are created, it means all solar neutrinos, as created in the core
of the sun, are electron neutrinos, which later turns out to be
important when early neutrino detectors measure only a fraction of the
calculated solar neutrino flux.
Much higher
energies of a supernova allows the creation of all three types of neutrinos:
electron, muon, and tau. Theory predicts that something like 90+% of the
energy of a supernova is released as neutrinos!
----------------------------------------
Wide
ranging neutrino spectrum
Here's a wide ranging
plot of natural neutrino fluxes and energy levels. (The original source
of this plot is unclear. I found it with an image seach, which references
an odd
blog, but almost for sure it comes from a technical paper or report.)
This figure spans a huge range of energy from 10^-2 ev to 10^17 ev. Only
neutrinos with energy above a few hundred kev to a few Mev are detectable
by detectors.

IceCube is so large and has so few high energy events
because it is designed to detect
the very low flux of astrophyysical neutrinos (lower
right corner)
The upper left corner, high flux, super low energy neutrinos, marked 'Cosmological' are probably from the big bang. While I haven't researched them, they are the neutrino equivalent of the cosmic background radiation. I have seen no one even suggest an idea for trying to detect these neutrinos. Turns out they have their own Wikipedia page (Cosmic neutrino background) and are indeed from the big bang. They are older than the photon microwave background, because it formed (decoupled) 380,000 years after the big bang whereas the relic neutrinos decoupled 2 seconds after the big bang (1 second says another Wikipedia page). Wikipedia says this:
"Before neutrinos decoupled from the rest of matter, the universe primarily consisted of neutrinos, electrons, positrons, and photons, all in thermal equilibrium with each other." I find this interesting. No mention of protons, neutrons or quarks. At 10 sec most of the leptons and anti-leptons annihilate (leaving some leptons) transferring their energy to photons. At 3 minutes protons and neutrons must be around because they begin combining into nuclei (helium).The major source of neutrinos on earth is from nuclear reactions within the sun. The solar neutrino spectrum is shown in detail far above. It plots the neutrino flux and energy from various nuclear reactions within the sun at one astronomical unit (at earth's radius). It shows that some reactions, beta like reactions, give a continuous spectrum whereas other reactions emit neutrinos with a relatively fixed energy that plots as a line (or impulse) on the spectrum.
Most solar neutrinos have energy in the 100 kev to 2 Mev range with 99.9% less than 400 kev (elsewhere in this essay I show where this 400 kev comes from). About ten in a million have energy in the 2-20 Mev range, and these high energy solar neutrinos were the only solar neutrinos detectable by the big solar neutrino detectors. Super Kamiokande water cherenkov detector has about a 5 Mev threshold. Sudbury's deuterium fission mode required neutrinos with at least 2.22 Mev of energy to overcome the proton to neutron binding energy in the deuterium nucleus. The detected 1987 supernova neutrinos had energies of 7.5 to 35 Mev.
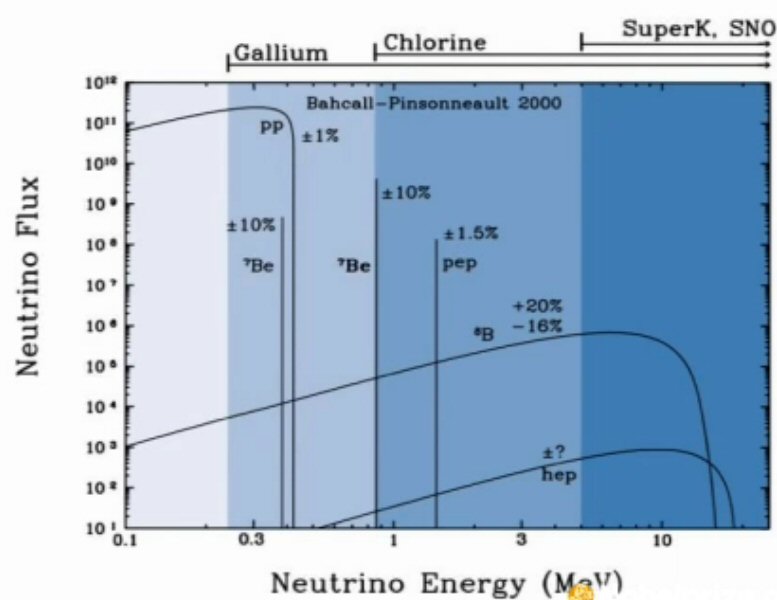
Gallium neutrino detectors
The exception
to high detection thresholds was two 1990s radio-chemical neutrino detectors
[Sage, Gallex] whose target was the nucleus of gallium. These detectors
had a threshold of 233 kev, the first detectors with a low enough threshold
to detect the main solar flux from the first step in the pp chain (energy
< 400 kev). About 40% of (stable) gallium is Ga71, and a neutrino triggered
(n => p) reaction changes it to an isotope of germanium that is radioactive
with a half life of 11 days.
ve + Ga71 => Ge71 + e- (233 kev threshold)The detection rate of Gallex (30 tons of gallium) was only 3/4th of a neutrino/day. Like all the charged current radio-chemical neutrino detectors it could only detect electron neutrinos, and it found the same discrepency (solar neutrino problem) in the main solar flux that earlier neutrino radio-chemical detectors had found.
Solar neutrino unit
Turns out
there is a standard unit for comparing the capture sensitivity of neutrino
detectors per atom called the 'solar neutrino unit' (SNU) defined as: 10^-36
captures/atom/sec. From its units it looks like it combines the neutrino
capture cross section of the target atom with the strength of the solar
neutrino flux over the usable capture energy. The Slac paper gives these
numbers:
-- Chlorine
detector Homestake with an 814 kev threshold reported SNU = 2.56 (about
1/3rd of what had been calculated)
-- Gallium
detectors Sage and Gallex with a much lower threshold of 233 kev reported
much higher SNU = 67 and 77
Cosmic ray
neutrinos are presumed to exist with energies of 100 Mev to 1 Gev. In 2012
Icecube found two neutrinos with energy of about 1,000 Tev (1 Pev = 10^15).
----------------------------------------------------------------------------------------------------------------------------------
How can neutrinos be detected?
Can a neutrino
detector be built based on a neutrino emitting reaction run backwards?
Well, sort of. It turns out you don't need to reverse the reaction, just
reverse the direction and type of neutrino! The classic beta- (radioactive
decay) reaction is a neutron converting to a proton (n => p) spitting out
an electron and anti-neutrino. This reaction still works if the outgoing
anti-neutrino is replaced by a neutrino (non anti-neutrino) that comes
in.
The only real difference is that the energy of the emitted electron now
increases (substantially) because instead of just getting a share of the
energy difference between n and p, it gets all the mass difference energy
plus most of the energy the neutrino brings in.
There is also a timing difference. When a particular atom will spontaneously decay via (n => p), an electron emission beta- decay reaction, is unpredictable, the timing depends on quantum uncertainty. A neutrino detecting (p => n), also an electron emission reaction, is (in effect) triggered by the absorption of a neutrino into the atom. However, it's very likely that quantum uncertainty plays a major role as to whether or not a particular atom will absorb a passing neutrino.
I had first studied up on Feynman diagrams of neutrino-quark interactions, and I knew there was a diagram on a variant on beta decay which showed an incoming neutrino interacting with a quark (in a neutron) converting the neutron to a proton (n => p) while throwing off a (relativistic) electron. This seemed to fit perfectly with how ice/water neutrino detectors like IceCube work. They detect neutrinos (not anti-neutrinos) and their phototubes are designed to look for light from relativistic electrons (not the light flash from positrons annihilating with electrons). And a review of their web sites did not abuse me of this, because they say little about the underlying physics they use. There is no detection equation! However, for the (n => p) reaction to work the neutrino target had to be a neutron in the oxygen of the water (cause there ain't no neutrons in hydrogen!), which would be converted to an atom of fluorine, and nobody ever seemed to mention this. Curious.
Then when this essay was well along, I came across a 1995 technical neutrino report online which described the operation of various neutrino detectors and importantly gave their detection equations. Kamiokande, which detects neutrinos with water, was described as based on the principle of neutrino induced electron scattering. I had forgotten what I first wrote about neutrinos, that they interact (via weak force) with both quarks and leptons, the electron of course being the classic lepton. I needed to look for a feynmann diagram showing a neutrino-electron interaction, and sure enough I found one. The diagram (below far right) shows that a neutrino can hit and effectively 'bounce off' an electron with the reaction transferring both energy and momentum from the neutrino to the electron. Clearly the water/ice detectors could very well be detecting neutrinos this way.
Neutrino target
overview
There are
two
basic
ways a neutrino can interact with matter (electrons and quarks). When a
neutrino hits an electron, it 'bounces off' causing the electron to recoil.
In transparent media like water and ice this generates a light flash if
the energy transfer is high enough to cause the electron to recoil at a
speed that is higher than the speed of light in that media. This is the
detection method used in modern large water and ice detectors (Super Kamiokande
and Ice Cube). Neutrinos can also bounce off quarks in neutrons (and protons?)
knocking them free of an atom. This was one of the detection modes in the
heavy water Sudbury detector.
A neutrino (or anti-neutrino) can also be absorbed by a quark in a neutron or proton. A neutrino absorption can trigger (n => p) and an anti-neutrino absorption can trigger (p => n). Both have been used in neutrino detectors. The former was used in the Homestake mine solar neutrino detector to convert chlorine 37 to argon 37, which is radioactive. The later was used in the first ever detection of neutrinos by Cowan and Reines from a nuclear reactor converting a hydrogen atom in water to a free neutron and a positron both of which they detected.
Absorption (via W) is the more complex than a bounce in that it causes a quark flavor change, resulting in an atomic # change, and new particles are created and emitted. Because the charge of the quark changes, particle physicists call this a 'charged current' reaction. (The +/- change in quark charge (in effect) flowing (via W- or W+) to the emitted electron or positron.)
A bounce is simpler. It's the quantum (relativistic) equivalent of classical (elastic) collision. An incoming neutrino 'hits' and bounces off (via Z) an electron or quark in a nucleus causing the particle to recoil. Since there is no charge transfer, particle physicists call this a neutral current reaction. No new particles are created, and the neutrino (in effect) keeps on going after transferring some of its momentum and energy to the particle it hits. (That's one view, the simpler view, but I suppose it's also possible the reaction could be viewed as the incoming neutrino being absorbed with the recoiling particle creating and emitting a new (lower energy) neutrino. Quantum diagrams lend themselves to multiple interpretations.)

left --- neutrino absorbed (n => p), only electron
neutrinos
middle --- scatter off neutron (knocking it free),
all neutrinos
right --- scatter off electron, all neutrinos
(caveat --- electron scatter (right) is mostly due
to ve, see Kamiokande mystery)
(source --- excellent Hyperphysics site: http://hyperphysics.phy-astr.gsu.edu/hbase/particles/neucur.html)
Both types of interaction can (in principle) result in an electron moving near the speed of light. In the case of a neutrino triggered (n => p) conversion the electron is newly created to 'carry away' the negative charge and comes out with much of the energy brought in by the neutrino and released by the mass loss. In the case of a bounce hit the electron preexisted (in an atom) and is accelerated to high speed by the energy and momentum tranfer from the neutrino bouncing off.
For a long time I found no info on the fraction of neutrino energy that could be transferred, but I found in Wikipedia that massless photons with the energy of electrons (0.5 Mev) can transfer 2/3rd of their energy to electrons in a direct hit. I later worked this problem, solving the equations for relativistic elastic collisions of photons and neutrinos, and details of the energy transfer from neutrinos and photons to electrons can be found in the appendix. Suffice it to say that 5 Mev solar neutrinos, which have x10 times the (rest mass) energy of an electron, can (in a square hit) transfer most (> 90%) of their energy to the electron in an elastic collision, a result very different from a classical elastic collision.The designer of a neutrino detector has to choose which type of interaction to optimize for. Only near the end of this writing did some aspects of how detector builder chose their neutrino targets become clear.
Absorption -- charged current
A complex nucleus,
meaning not hydrogen, is used as a target only when the goal is
the modification of the atomic number to make an adjacent radioactive isotope.
This is a charged current reaction where the neutrino (or anti-neutrino)
is absorbed by a quark triggering a (n => p) or (p => n) conversion. Detection
is by sensing radiation from the newly created radioactive element. Feyman
diagrams show these reactions also emit high energy electrons or positrons,
but (as far as I can figure out) no detector ever appears to try and detect
them. This may be either because they can't escape a big nucleus, or they
come out with too little energy, or (my favorite) the reaction probabilities
within a big, complex nucleus just cannot be accurately calculated (or
simulated) and without accurate calculations the experiment is meaningless.
The simplest nuclei, hydrogen and deuterium atoms, make good targets for charged current reactions and here different detection strategies are employed. The absorption of an anti-neutrino by the hydrogen proton triggers a (p => n) conversion. This is a beta+ type decay, which lowers atomic number by one, here taking element #1 (hydrogen) down to 'element #0', a bare neutron. This, of course, breaks chemical bond of the hydrogen so the newly created neutron is free, and the reaction also creates a positron that is emitted. One or both of the newly created particles can be detected. (In 1956 for the first detection of neutrinos the detector was small and noisy since it was not deep below ground, so to get adequate S/N both the free neutron and positron were detected from each anti-neutrino hit.)
In the case of deuterium a neutrino triggered (n => p) conversion of its one neutron emits a detectable high speed electron and also breaks breaks apart the nucleus, because a 'helium' nucleus of two protons is not stable.
Bounce -- neutral current
A different
approach to neutrino detection is a target where a neutrino can 'bounce
off ' knocking free particles that already exist and are not too tightly
held. These are scatter hits, where (in effect) the neutrino bounces off
of an (existing) electron or quark transferring substantial energy and
momentum to it and then keeps on going. No new particles are created and
no charges move, so this is a neutral current reaction. The target of the
big cherenkov water (and ice) neutrino detectors is a scatter hit to an
electron of the water's hydrogen. (It's unclear if any of the (non valance)
oxygen electrons are knocked free, but I don't think so.) The high speed
electrons are detectable by the cherenkov radiation as they cause the atoms
along their path to radiate.
The only nucleus target for a scatter hit (that I know of) is the relatively simple (two nucleons) nucleus of deuterium in heavy water. Here a high energy neutrino bounces off a quark in the neutron (maybe the proton too?) and is able to knock the deuterium nucleus apart producing a free neutron. This free neutron can be detected by neutron absorbing atoms (cadmium) that visibly flash to release excess energy as they settle into a new isotope state.
A variation on bounce detection can be found in plans for Wimp detectors. Here the idea is that a bounce hit from a (heavy) wimp to a complex nucleus of an atom will cause the (whole) nucleus to recoil. The recoiling nucleus is expected to (in effect) pull away from some of its cloud of electrons making free electrons and a fast moving positive ion both of which can be detected.
Neutrino detector with multiple modes
Sudbury was
unique among large neutrino detectors in using (expensive) heavy water
(on loan from the Candian nuclear power people). This provided several
targets including one no other neutrino detector has ever had before or
since, a two nucleon nucleus of deuterium (np), which can be knocked appart
(freeing the neutron) by a scatter hit of any type of neutrino of
sufficient energy. Below are the three general classes of neutrino interaction
with quarks and leptons of D2O (heavy water) available at Sudbury:
1) Nucleus (n => p) conversion due to (dn => up) quark change. Charged
current reaction, electron
neutrino only, neutrino (in effect) converts to its associated lepton (electron)
and electron carries
away a fraction of the neutrino energy causing it to move at relativistic
speed. The conversion of
(np => pp) breaks up the deuteron, because a (helium) pp nucleus is not
stable.
ve + d => p + p + e-
(9 events/day) (1.44 Mev)
2) Scattering of a neutrino off a quark, here shown as energy and momentum transfer to the neutron. Scattering is aThe Sudbury heavy water neutrino detector was able to detect both (n => p) conversions and (two types of) bounce hits, principally a 'bounce' off deuterium nuclii causing them to fission, and a secondary mode where neutrinos 'bounce' off electrons accelerating them to relativistic speeds.
neutral current reaction. Any type of neutrino: electron, muon, or tau type. Neutrino loses energy, but keeps
going. Enough energy can be transferred from the neutrino to one of the nucleus particles to break the
deuterium nucleus apart releasing a free neutron that can be (optically) detected.
vx + d => p + n + vx (3 events/day) (2.25 Mev)3) Scattering of a neutrino off an electron. Scattering is a neutral current reaction (discovered in 1973 at CERN).
Any type of neutrino: electron, muon, or tau type, but electron neutrinos favored. Neutrino loses energy,
but keeps going (with no flavor change). The recoil direction of the relativistic moving electron provides
information about the direction (momentum) of the incoming neutrino that 'bounced' off.
ve + e- => e- + ve (1 event/day)
Still questions remain that no reference seems to address. If the reaction is electron scattering, is the target any electron of the H2O molecule or is it just the hydrogen bonding electrons? Are maybe both neutrino reactions in play: (n => p) quark and electron scattering? I went back and checked both the IceCube homepage and the related Wikipedia article. This is the world's largest neutrino telescope, and there is no detection equation on its site explaining how it really works! I posted below on the Wikipedia IceCube Talk page and a variant on this in a direct email to the IceCube people via their web site. (no reply)
"Some expert should include the neutrino detection equation with a short description written for the scientifically literate non-specialist. Does the telescope detect via neutrino induced electron scattering? Is the neutrino electron target any electron of water or just the hydrogen bonding electrons? Does (n => p) conversion in the oxygen nucleus with ejection of a relativistic electron play any role? I don't understand why this basic information is missing in so many Wikipedia articles on neutrino detectors."More on neutrino detection
Cherenkov radiationAn anti-neutrino can convert a proton to a neutron (p => n), typically in water, and in this case a positron (positive electron) is emitted. When a positron meets an electron, they anniliate in gamma photon flash. With the use of scintillators, which absorb gamma rays and re-flash, positrons can be optially detected too.
Cherenkov radiation results when a charged particle, usually an electron, travels through a dielectric (non-conductive electrically polarizable) medium with a speed greater than the speed of light in that medium, which for water is 0.75c. In analogy with the shock wave that forms in front of a plane going faster than the speed of sound, the electro-magnetic field of the particles creates a leading cone of disturbance in the electrons of the medium, driving them to higher orbits. As the electrons relax a broad spectrum of high frequency light is emitted. This is the blue glow of water covering a reactor absorbing beta electrons from decay products (see below). In the detectors rings of light from single high speed electrons are detected.
W-, W+ decay
When W- forms,
it carries off a negative charge, delivering it when it 'decays'. Thus
W- links to (up/down) quark changes and electron/neutrino (leptron)
emissions. It is the virtual weak boson link in beta decay, linking a quark
change [-1/3 down quark in a neutron => +2/3 up quark in a proton] to the
emitted output of [electron + anti-neutrino]. W+ works the same way except
it transfers a positive charge, so it's involved in the opposite beta reaction.
W bosons have a very large mass/energy (80 Gev), which means they have very short lifetimes (10^-25 sec) second and operate over tiny distances (10^-18 m, or 1/10,000 of a nucleus dia). The explanation given for this is that as virtual particles they have to 'borrow' energy from the vacuum and to stay within the Heisenberg uncertainty limit [Energy x time < 1/2 x hbar], so the time has to be very short.
The distance is so short that in beta decay it would follow that the electron is created right where the quarks are, which must be inside the nucleus. How does this work? This would apply to incoming neutrinos being detected too. If the W boson can only travel 1/10,000 the dia of the nucleus, then in a simple picture the incoming neutrino must burrow (deep) into a neutron or proton to hit a quark, and this presumably is where the whole reaction takes place, the neutrino is absorbed, the quark changes charge and the electron or positron is created all inside the neutron or proton. No one every mentions this! Since a heavy Z is the means for a (neutral current) bounce hit, the neutrino here too must penetrate the neutron or proton to transfer momentum.
Feynman style diagrams
Feynman drew
his diagrams with time vertical, and usually with W boson horizontal (often
with no polarity or direction, which can be inferred). The diagrams below
are in this style.
Beta decay
The first
diagram (below) is beta- decay. This is one of the first three radioactive
decay modes discovered, described by Rutherford (sans neutrino) over 100
years ago. A neutron 'decays' to a proton (n => p) with and electron and
anti-neutrino emitted. This is the most connon feyman diagram. In an atom
the electron emitted is not an orbital electron. It is generated (apparently)
in the nucleus at the neutron whose down quark (-1/3) is converting to
an up quark (+2/3) to make a proton. The second diagram is beta+ decay
(p => n). It is similar to diagram 1 except a proton converts to a neutron
(energy input required) and the output is a positron (anti-electron) and
an (electron) neutrino.
Since the rest mass energy of a neutron is higher than a proton, the first reaction (beta- decay) can occur spontaneously, but to get the second reaction (beta+ decay) to go either energy must be supplied externally, or the binding energies of the nucleus must be such that the daughter isotope has less mass than the mother isotope. The energy is needed for the extra mass of the neutron and to provide the (rest mass + KE) energy of the emitted positron and electron neutrino.
 .
. 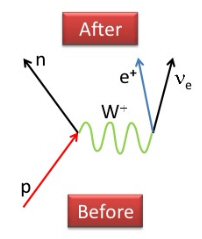
Beta - decay
n => p + e- + ve bar
Beta+ decay p => n +
e+ + ve
(Feynman diagrams by Alessio Bernardelli, Network
Coordinator at Institute of Physics on Nov 24, 2011
http://www.slideshare.net/asober/feynman-diagrams)
Above has neutrinos going out, but the same reactions can occur (below) with neutrinos of opposite type coming in and increasing the energy of the outgoing electron/positron, which is a good thing if you want to detect it. In both of these reactions the leptron # is conserved since by definition (e- and ve) have same lepton # (+1) and 'anti' particles (e+ and ve bar) are (-1).
 .
. 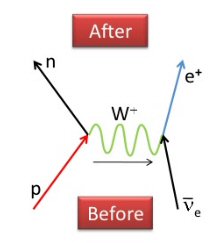
(left) neutrino collides with neutron
ve + n => p + e- (w/high energy)
(or neutron captures neutrino)
(right) anti-neutrino collides with proton
ve bar + p => n + e+ (w/high energy)
(or proton captures anti-neutrino)
Note these feynman
diagrams hint at possible neutrino detection strategies. The first diagram
says an incoming neutrino can bump an atom of any element up
the periodic chart by one step by converting one its neutrons to a proton.
And an incoming anti-neutrino does the opposite, it can bump an
atom of any element down the periodic chart by one step by converting
one its protons to a neutron. So one neutrino detection strategy would
be look for a few atoms of a radioactive isotope of an adjacent element
in the periodic chart that slowly show up over time. And in fact this was
the detection stategy used in the first bing solar neutrino detector, Homestake,
where a few atoms of stable chlorine were converted to radioactive argon.
-------------------------------------
Interesting variation on beta+ decay --- incoming
electron captured by proton
There's is
another interesting variation on the beta+ decay. The beta+ feyman diagram
(upper rt) shows a (p => n) conversion emits a positron and a neutrino.
We saw earlier that the neutrino direction could be 'flipped' to come up
with a reaction that could be (and is) used to detect anti-neutrinos. In
the figure below we see that the lepton can also be be flipped. In this
case instead of a positron going out, we have an electron coming
in.
Clearly lepton # is conserved since a lepton (electron) comes in and a
lepton (neutrino) goes out.
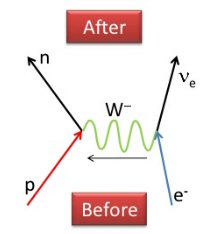
proton
captures electron
p + e- (orbital) => n + ve
(or electron collides with proton)
(Source --- http://www.slideshare.net/asober/feynman-diagrams)
Does this reaction really happen?
Yes, it occurs
in the sun as part of the pp fusion chain, and it occurs in some atoms
that decay too. In the sun it makes neutrons for helium, and in atoms it
is one way that some neutron shy, unstable isotopes decay to a lower atom
number by grabbing off one of their orbiting electrons. (see Appendix --
'Periodic chart isotope decay modes')
An interesting thing is that the neutrino energy is now fixed, mono-energetic says Wikipedia, on a solar neutrino flux vs energy plot it looks like an impulse. This reaction is part of the pp chain in the sun and explains the source of the 'fixed energy' neutrinos on the neutrino spectrum that plot as a line (or impulse). In fact it is these higher energy (5 to 15 Mev) fixed energy neutrinos that the water cherenkov detectors detect.
In the atom Feyman's describes this reaction as a proton 'capturing' an electron to form a neutron (with a neutrino thrown off). Wikipedia (radioactive decay) says the electron 'captured' in this reaction is usually an inner orbital electron. This reaction is common in radioactive decays of isotopes that are deficient in neutrons. Wikipedia says it can proceed with less energy than the classic beta+ decay, because no energy is carried off by the positron and the electron can bring in energy.Mono-energetic?
I was originally puzzled by this. If the particle input KE varies, doesn't that mean the output neutrino energy must vary, so how can it be mono-energetic? Took me a few minutes to figure this out. The answer to the question is yes if the energy input varies, then the energy of the neutrino will vary. But this ignores the fact that the reaction in the sun's core is occurring at a fixed temperature, so the KE of the incoming particles will likely be pretty close together, hence the total output energy (to be shared by the neutron and neutrino) is fixed. I suppose it can be argued the neutron at a fixed temperature also has a known energy, or maybe the argument is like in many elastic collisions the light particle gets most of the KE, but the result is that with input energy (relatively) fixed, so is the neutrino energy.The real contrast is with the (n => p) beta reaction where a zero momentum nucleus separates into three particles: proton, electron and anti-neutrino. Apply conservation of momentum and most of the energy goes to the two light weight particles (electron and neutrino), and here (apparently) quantum variation causes the split of the energy between the electron and anti-neutrino to be wildly variable. In the above reaction that can't happen as there is only one light weight output.
Capture cooling-------------------------------------
However, the neutrino does carry off some energy, and obviously it's capable of carrying off energy from deep within experimental apparatus. And in fact this trick, called 'capture cooling', is used in the lab. (There's also a class of reactions called 'neutrino heating' that probably involve incoming neutrinos bringing in energy.) Footnote --- Luis Alvarez in 1937 first experimentally demonstrated orbital electron capture (using vanadium 49 and gallium 67).
All four of these diagrams are just different ways of sketching (and interpreting) exactly the same physical reaction. W- and W+ are each others anti particles and it is a convention on diagrams to show the arrow of anti-particles as going backward in time, which is what two of the diagrams below do. I marked them 'not causal', which is only in the conventional sense, since, of course, in the quantum world this is perfectly Ok.
Four (close up) views of a neutrino triggered
(n => p) conversion,
which was used by early radio-chemical neutrino detectors.
A virtue of sketches like these is it allows us to think about (make a picture of) how neutrinos works. For example:
-- neutrino can 'explode' into a charged pair (W+, e-) (lower left)
with virtual W+ being absorbed by a down quark converting it to an up quark
-- neutrino can convert to an electron by absorbing a W- (which brings
in negative charge)
incoming W- can be going either forward in time (upper left) or backward
in time (lower right)
-- neutrino can convert to an electron by emitting W+ (retaining a negative
charge for charge neutrality)
outgoing W+ can go either forward in time (lower left) or backward in time
(upper right)
---------------------------------------------------------------------------------------------------------------------------------
Two
ways to detect neutrinos --- detect a new element or an emitted particle
In summary
the big neutrino detectors have detected incoming neutrinos in basically
two ways: by detecting an atom of a new element created by a neutrino hit
to a quark in the nucleus or by detecting a particle emitted, usually a
high speed electron or (slow) neutron.
A neutrino absorbed by a quark in a neutron can convert it to a proton (n => p), and conversely an anti-neutrino absorbed by a quark in a proton can convert it to a neutron (p => n), both cause the atomic # to change. Neutrinos can also bounce (or scatter) off either electrons or nucleii causing recoil or fission. Electrons as light particles are easily accelerated by a neutrino hit to near the speed of light. An anti-neutrino bounce off the nucleus of deuterium can split (or fission) it knocking a neutron free. A hit by super high energy neutrinos from deep in space can create a cascade of charged particles.
Element change
A neutrino hit to
a down quark in a neutron can result in it being absorbed changing the
down quark to an up quark. This changes the neutron to a proton (n => p),
bumping up the atomic number by one, plus an electron is created and emitted
at high speed. Since the neutrino 'transfers' charge to the quark, it is
called charged
current neutrino reaction. Early radio-chemical neutrino
detectors worked this way. Target materials were chosen where an increase
in atomic # by one (with no change in atomic weight) would result in radioactive
isotopes with a half life of a few weeks. The amount of radioactivity in
the detector was periodically measured (decays counted) and taken to be
a measure of the number of neutrino hits in past days or weeks.
Beta+ (p => n) conversions, triggered by absorbed anti-neutrinos, lower the atomic number by one, just the opposite of atomic number increasing beta- conversions. But what happens if the atom having it atomic number reduced by one is hydrogen (typically hydrogen in water)? How do you reduce the atomic number, when it starts off at one? Think of it as a special case. When the proton nucleus of hydrogen absorbs an anti-neutrino and a (p => n) conversion is triggered, we get (in effect) an 'atom' of zero atomic number, an 'atom' of atomic weight one with no protons. Of course, it's usually called a (bare) neutron, and because it no longer has an attraction to electrons it means the new 'atom' has no chemical attraction to the atoms of its old molecule. In the case of water it will not be captured by the oxygen nucleus, so it is free to sail off where by use of scintillators (and in various other ways) it can be detected. This method was used in the very first experimental detection of neutrinos (specifically anti-neutrinos from a nuclear reactor).
Two pictures of (n => p) conversionKnocked free
In a neutrino triggered (n => p) conversion two things happen virtually simultaneously (10^-25 sec), but for picture purposes each can be viewed as happening first which leads to two pictures can 'explain' the conversion. One, an approaching neutrino first 'sucks' (via W-) the negative charge from a down (-1/3) quark making it an up (+2/3) quark. The neutrino (a lepton) with the negative charge is then transformed into an electron (a related lepton) that flys off. Or, two, an approaching neutrino first 'explodes' into a positive charge particle (W+) and an electron that flys off. The W+ (weak force boson) merges with, and adds its charge to, a down (-1/3) quark making it an up (+2/3) quark).
Neutrinos can
also interact without being absorbed, without a charge transfer, these
are so-called neutral current interactions. The neutrino (in effect)
bounces off a particle (electron or quark) transferring energy and momentum
and keeps going. This type of neutrino induced 'recoil' (or scatter) can
knock electrons free and accelerate them to high speeds allowing them to
be optically detected. A special case is a neutrino bounce off the nucleus
of deuterium (really one of its quarks), which is able to split (fission)
the proton and neutron apart allowing the neutron to escape to be detected
as a free neutron.
------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
Cowan
and Reines detect first neutrinos in 1956
Anit-neutrino flux from nuclear reactor measured
The neutrino flux
next to a nuclear reactor was calculated to be 1 to 10 trillion per cm^2
per sec, far higher than any other source (solar flux is 0.064 trillion/cm^2
per sec). In 1956 Cowan and Reines using this high flux were the first
to experimentally detect neutrinos (Nobel prize in 1995). In the classic
Cowan and Reines experiment instead of an outgoing neutrino, you have an
incoming anti-neutrino from a nuclear reactor.
ve bar + p => n + e+ (p => n) (E > 1.8 Mev)Above we have an (anti)neutrino hitting a proton (bringing in the energy necessary) to create a neutron (by converting an up quark to a down quark) with emission of a positron. Note this is a beta+ like (p => n) conversion triggered by an incoming anti-neutrino. The reason Cowan and Reines are using protons as targets is that the reactor neutrino flux is really an anti-neutrino flux. Reactors create copious anti-neutrinos because inside there are freshly split, neutron heavy, uranium atoms fragments that are decaying via beta- (n => p) and outputting electrons and anti-neutrinos, and anti-neutrinos absorbed by protons trigger (p => n) conversions.
e+ + e- => pair of 0.5 Mev min photons(gamma) (anti-electron annihilation)
n + cd108 => cd109* => cd109 + gamma
(Wiki tald poster says this is the wrong isotope, it should be cd113 => cd114 + gamma)
Detecting gamma ray emissions
Cowan and
Reines came up with a way to detect both the positron (e+) and the
neutron, thus providing a unique signature for the reaction. Turns out
that certain scintillating materials will flash when hit with a gamma photon
allowing gamma photons to be detected. A positron-electron annihilation
generates a pair of gamma photons (each 0.51 Mev min, the electron
rest mass) that fly off in opposite directions (conserving momentum), so
scintillation material will respond to a positron annihilation with a flash
(or double flash). Cadmium is a great absorber of neutrons (used in control
rods in nuclear reactors). When it absorbs a neutron, it allows the neutron
to be 'seen' because it will emit a high energy gamma photon. In this case
the neutron induced gamma flash occurs about 5 usec after the positron/electron
flash pair, so a unique signature of an anti-neutrino absorption is created.
All the gamma induced scintillation flashes were detected with phototubes.
Anti-neutrino targetCowan and Reines in the 1950s used cadmium chloride dissolved in water as the neutron absorber and (liquid) scintillation detectors on the sides to detect the gamma flashes. The emitted positron hits an electron and annihilates producing a pair of photons each with 0.5 Mev energy. A few usec later the created and ejected neutron (moderated by the water) is absorbed by a cadmium ion causing an 8 Mev gamma ray emission. The IceCube neutrino telescope web page calls the 0.5 Mev photons produced here 'gamma rays', which I guess they must be because visible light photons have only a few ev of energy. They say, "scintillating material gives off flashes of light in response to the gamma rays" and these light flashes can be detected with photomultiplier tubes, so this is how the gamma rays from the positron/electron annihilation were detected.
The Wikipedia article on this experiment clears up a point not clear in other references: the proton target. The neutrino target material here is a (small) tank of water (just 1 cubic meter), but which proton of water? The experiment is designed to detect a single free neutron (created from a proton), and this is most likely to happen when the nucleus of one of the hydrogen in water absorb an anti-neutrino and converts, even though most of the protons in water are in the oxygen.Why hydrogen in a molecule is a good target
In other words the experimenters want the anti-neutrino created (p => n) neutron to get free so it can be detected, and this is much more likely to happen, and certainly easier to analyze says Wikipedia (neutrino), if the proton target is a hydrogen nucleus. Clearly a converted hydrogen nucleus, now a neutron, has no electromagnetic force holding it to the water molecule, so even if it has not acquired much KE from the neutrino hit (which it probably has), it can just drift off. By comparison a (p => n) neutron created in the oxygen nucleus has converted the oxygen to an isotope of nitrogen (N16), and the new neutron will likely be held in place (at least initially) by the strong nuclear force. A check of N16 shows it is unstable (half life of 7 sec), but 99.99% of it just throws off an electron (via beta-) taking back to oxygen 16 with the remaining tiny fraction also throwing off an alpha particle taking it down to carbon. No free neutron is produced.
Their experimental setup was located about 40 feet under the big Savannah River reactor to reduce the noise from cosmic ray neutrinos. They used 200 liters of water and 110 photomultiplier tubes. They detected about three anti-neutrinos per hour says Wikipedia, but Cowan in his lecture (see below) says it was three per day. As a confirming test that neutrinos from the reactor were really being detected, the reactor was shut down to give a baseline. The calculated neutrino capture cross-section for the reaction was 6 x 10^-44 cm^2 , and they measured 6.3 x 10^-44 cm^2 (within 5%, quite amazing results).In 1995 Cowan gave a one hour lecture at US Naval Acedemy on his classic experiment done decades earlier. It is available on YouTube (I have listened to all of it).Relevant comments I found on a physics blog
-- Cross-sections can be thought of (roughly speaking) as the particle target size the neutrino has to hit for the reaction to occur. Or another way to look at it is cross-sections indicate the probability of a reaction occurring.-- W and Z have very high mass (80-90 Gev) typically much larger than the neutrino mass-energy. This means they must be virtual particles, i.e. they must 'decay' almost immediately after they form, because they only way they can briefly (10^-25 sec) pop into 'existence' (probably a loaded term) is by borrowing energy from the vacuum consistent with the uncertainty principle.
http://www.youtube.com/watch?v=AYqEtm0X2Sc&feature=youtu.be
He describes in great detail the history of the experiment. At the time he and Reines were working at Los Alamos. At first they planned to use the huge flux of neutrinos from an atomic bomb, gathering data for just a fraction of the second with a crazy experiment in a hole drilled near the tower directly below the fireball. But a lab director suggested they try and see if they could detect neutrinos from a reactor even the though the flux would be 10,000 times lower. He says the breakthrough was the realization that if counted only paired positron/electron gammas and neutron gammas it enhanced the S/N by a factor of a million.
In the talk (at min 54) he gives the count rate they achieved as three per day, but this disagrees with the Wikipedia article (and with a history on the IceCube page) on the experiment which says it was three per hour. I left a note on Wikipedia pointing out the conflict.
Detecting neutrinos in nature
After Cowan
and Reines in 1956 demonstrated that neutrinos existed and that a modest
size detector was enough to detect a few events per day using the high
neutrino flux (10^14/cm^2) near the surface of a large reactor, planning
for detection of neutrinos in nature began. In the 1960s the 1st generation
of neutrino detectors were built and succeeded in detecting neutrinos of
local cosmic ray origin. In following decades neutrino detectors got bigger
to detect solar neutrinos and nearly all were built deep underground to
minimize noise from cosmic rays. A shift from radio-chemical to real time
dectectors (with phototubes) provided information about energy levels from
which direction the neutrinos were coming, confirming that neutrinos were
coming from the sun. And a single event (1987 supernova) confirmed
that detection of neutrinos from energetic events outside the solar system
is possible. A really huge neutrino detector has just been built to look
for super high energy neutrinos from deep space. Called Icecube, it has
strings of photodectors mounted in 1 kg^3 of clear antartic ice looking
for neutrino cherendov light flashes. In its first two years it detected
28 neutrinos with energies in the 30 to 1,000 Tev range, which are thought
to come from outside our galaxy.
-------------------------------------------------------------------------------------------------------------------------------------------------------------------------
Four big neutrino
detectors
While a lot
of specialized neutrino detectors have been built over the years, a good
overview of neutrino detection can be understood by just looking at the
four biggest neutrino detectors ever built, two of which are still operating,
all of which detect neutrinos differently.
1) Homestake
2) Super Kamiokande
3) Sudbury
4) IceCube
1) Homestake (1970 - 1994)
Homestake
was a big tank of cleaning fluid (C2Cl4) almost a mile down in the Homestake
Gold Mine in South Dakota. Neutrino detection was by means of a neutrino
triggered beta-like reaction in the nucleus of the chlorine that converts
one of its neutrons to a proton creating a radioactive isotope of the next
higher element (argon). Periodically the tank was flushed of argon which
was run by a geiger counter that (effectively) provided a count of the
number of neutrino created argon atoms. Homestake detected neutrinos (presumed
to be) from the sun, but only measured about 1/3rd of the expected number.
The Homestake detector provided no information about the direction or energy
of the neutrinos.
2) Super Kamiokande (1996 to present)
Super Kamiokande
is a huge tank of (regular, but ulta pure) water deep in a zinc mine in
Japan surrounded by an array of specially designed large phototubes. It
detects neutrinos by a variation of the bounce reaction Sudbury used, but
here the neutrinos bounce off an electron of the water molecule (probably
a hydrogen valence electron). A hit from a solar neutrino transfers most
(> 95%) of the neutrino energy to the electron, which because it is light
particle recoils at > 99% speed of light in a vacuum, substantially above
the speed of light in water, and as it loses energy it caused the atoms
along it path to emit a cone of cherenkov radiation that is picked up by
the phototubes. The high quality of this detector data confirmed from the
recoil direction of the electrons that the neutrinos were coming from the
direction of the sun. Even though a neutrino bounce off an electron works
(in principle) for all types of neutrinos, it is about x6 times more likely
to happen with an electron neutrino, so Kamiokande confirmed Homestake's
'missing solar neutrinos' measurement. In a lucky break an earlier 'proof
of concept' version of Kamiokande picked up a dozen or so neutrinos in
1987 from the closest supernova to earth in 400 years, the first detection
of neutrinos from outside the solar system.
3) Sudbury (1999 - 2006)
Sudbury was
a big tank of heavy water (D2O) located in a deep nickel mine in Ontario
Canada about 1.25 miles below ground. This rare (and expensive) material
gave Sudbury unique capabilites for detecting neutrinos.The heavy water
was surrounded by a huge number of phototubes. Sudbury had two ways in
could detect neutrinos, but not at the same time. One method of neutrino
detection that Sudbury could be set up for was a neutrino triggered beta-like
conversion of the single neutron in the deuterium nucleus to a proton.
This was the same type of neutrino reaction used in Homestake and can be
viewed as converting hydrogen to an unstable isotope of helium. However,
unlike Homestake Sudbury detects not the newly created element, but a high
speed electron that beta-like (n => p) reaction emits. The speed of the
electron is above the speed of light in the heavy water so it emits a cone
of cherenkov radiation as it slows, which is picked up by the photodetectors.
In its other mode of operation Sudbury can be set up to detect neutrino triggered fissions of the deuterium nucleus which is barely stable. Mev level solar neutrinos hitting (and bouncing off) a deuterium nucleus can impart enough energy to overcome the nucleus binding energy to separate it into a proton and free neutron. The free neutron is detected by doping the heavy water with a scintillator that absorbs the neutron and then flashes, the flash picked up by the phototubes. All three types of neutrinos impacting the nucleus can trigger the fissioning reaction, but in the other Sudbury mode only an electron neutrino can be absorbed by a neutron and trigger an (n => p) conversion with emission of a detectable electron. Because Sudbury had these two different modes, one sensitive only to electron neutrinos and the other sensitive to all neutrinos, it was able to show that half to 2/3rd of the neutrinos generated in the sun as solar neutrinos were being received on earth as muon and tau neutrinos, strong evidence for neutrino 'oscillation'.
4) IceCube (2010 to present)
IceCube is
a new ice cherenkov detector, far larger than any other neutrino detector
ever built, a cubic km of Antarctic ice located about a mile deep instrumented
with optical light detectors. Because of its huge size and relatively open
array of optical sensors this detector is designed principally to look
for very high energy deep space neutrinos (energy levels orders of magnitude
higher than solar neutrino). At these high energy levels neutrino bounce
reactions can produce muons (heavy electrons) which can travel long (up
to one mile) and straight in the ice at speeds above the speed of light
in ice. The cherenkov radiation from these high energy muons is mostly
what this detector is looking for, and the long muon paths indicate where
in the sky the neutrinos come from (to an accuracy of about +/- 1 degree).
A couple of dozen deep space neutrinos have been captured so far.
----------------------------
Homestake
chlorine detector (1970 - 94)
Solar neutrino flux is measured
The much lower
flux of solar neutrinos were first detected in 1970 with a different type
of detector. This was a huge (olympic swimming pool size) vat of cleaning
fluid (tetrachloroethene, C2Cl4) deep in the Homestake mine. The motivation
for this experiment was a check on the understanding of nuclear fusion
reactions inside the sun. Proton to proton fusion process in the sun was
understood to merge two protons to produce a deuterium nucleus throwing
off a positron and neutrino (p + p => np + e+ + ve),
hence the high flux of neutrinos from the sun. The 1995 neutrino report
says 3% of the sun's fusion energy is carried away by neutrinos, and it's
99% for a supernova.
The sun is on the main sequence 'burning' hydrogen to helium. To make a helium atom two neutrons are required, and since there are no free neutrons floating around in the sun, it would appear likely that the solar fusion chain would contain a (p => n) conversion step, and it does, and this is the source of the neurtinos. Elsewhere in this essay I shows the details of the pp chain in the sun, and you can see a neutrino comes out where a proton converts to a neutron.The Homestake experiment did the seemingly impossible. It detected a change in only 10 atoms (over a month) of the 10^30 atoms in the tank (that's ten in a million, trillion, trillion atoms)! It did this by a neutrino induced beta conversion (n => p) in the chlorine that made a few atoms of argon that were radioactive. The radioactive argon was then flushed out of the tank, and its quantity measured by means of its radioactivity.Each neutron is created by a beta+ like (p => n) reaction that also outputs a positron and neutrino. The energy input needed for the higher neutron mass is probably obtained thermally, which in turn comes from the energy released by the lower mass of the helium compared to the four protons from which it is created.
Details of the Homestake detector
The reaction used
by Homestake was a neutrino triggered beta- like (n => p) conversion that
changed a neutron in chlorine to a proton (ve + n => p + e-) making
a radioactive isotope of argon with the right lifetime, fast enough so
that its decay could be captured and counted, yet not so fast that it decayed
away inside the tank. The combination that works is Cl 37 => Ar 37. Ar
37 has a half life of 35 days and about 1/4th of chlorine atoms are Cl
37. (The other 3/4th of chlorine atoms are Cl 35, but they converts to
Ar 35 which has a half life of 1.7 sec and by throwing off a positron and
neutrino just decays back to Cl 35.)
The operative feynman diagram of this neutrino detector shows a neutrino-triggered (n => p) conversion that throws off an electron. Since an electron comes out, it follows that the only type of incoming neutrino that can be absorbed to trigger the (Cl 37 => Ar 37) conversion is an electron neutrino. Homestake is counting only electron neutrinos.
Where the experiment was run, there was a surprise. Radioactive Ar 37 was detected as predicted, but the quantity was only about 1/3rd what had been expected based on calculations of solar fusion reactions. Initially people were skeptical suspecting that either the calculations or experiment were in error, but later neutrino detectors found a shortfall too, and it became known as the 'missing solar neutrino' problem.
The reason for the 'missing' solar Homestake neutrinos was found in 1998 when other neutrino detectors that were able to show that neutrinos were likely oscillating between the three flavors. A simplistic oscillation model from Homestake data might be that 1/3rd of the time neutrinos appear to be electron neutrinos, 1/3rd of the time muon neutrinos, and 1/3rd of the time tau neutrinos, but the I don't think it's that simple. For one thing while other neutrino detectors found shortfalls it was a different ratio. This is still a problem being worked and was a major motivation to build neutrino detectors to detect accelerator generated neutrino beams. I think the hope is that by knowing more about the oscillation, they can work backwards to determine the mass of the three neutrino types, since theory says the oscillation depends (in some way) on the neutrino mass differences squared.
A classic example of the radiochemical type of detector was a big tank (615 tons) of cleaning fluid (C2Cl4) in the Homestake gold mine in South Dakota that began operation in 1970 and ran for 24 years. This was a 'chlorine detector', the first neutrino detector big enough and sensitive enough to detect a few solar neutrinos. One event every 16 hours had been calculated. Neutrinos hitting Cl37 converted it (n => p reaction) to Ar37, which is radioactive with a half life of 35 days. After a couple of months the radioactivity of the tank would come to steady state with the neutrino induced creation rate balanced by the decay rate. Periodically helium was bubbled through the tank to extract the radioactive argon for measurement.
ve + Cl37 => Ar37 + e- (814 kev threshold)
The event rate (argon creation rate) in this experiment turned out to be very low, about one atom of argon 37 every two days in the whole tank, and yet the experiment was so good it was still measurable! Calculations based on solar models and neutrino intereactions with chlorine predicted that about 48 atoms of Ar37 should accumulate in the tank, but the measurments found only 17. This was the basis for the classic missing solar neutrino problem, the number of electron neutrinos being detected was only about 1/3rd of the predicted rate.
Homestake's trick to quiet the background
I watched
the nobel lecture of the designer of Homestake, Raymond Davis, which explained
a lot of the details of the experiment. The neutrino hit rate at Homestake
was quite low (10 neutrinos/month), so the key to it being a successful
experiment was getting the background count rate very low. The background
rate during most of the run was very low, but it didn't start off that
way. The tank had been built deep underground to shield it from cosmic
rays, but still in its first (monthly) runs the data was barely visible
above the background. (Partly this was because the data counts were x3
less than expected.)
Background and signal prior to rise time discriminator
being added
(screen capture from Davis Homestake 2002 Nobel lecture)
(source --- http://www.nobelprize.org/mediaplayer/index.php?id=510)
Within a few months Homestake made some changes that resulted in the background count rate being reduced by an order of magnitude! Mostly this was done by the simple addition of adding an electronic discriminator between the geiger counter tube (analog pulse) and the digital counter. This trick worked because most of the background counts were were coming not from radiation getting into the tank, but from background noise picked up by the geiger tube itself. This is something that would have been obvious, they would be getting counts when no argon was in the tube. An oscilloscope look at current pulses from the geiger tube would have revealed that when radioactive argon was added to the tube, 'decay' current pulses appeared that had a different character (shape and amplitude) than the background pulses the tube put out with no argon. If they could figure out how to only count pulses with the 'decay' shape, then most of the the background radiation the tube was picking up would not matter, because it would not get counted.
Here's how the trick worked: The argon trapped from the tank was put and held in a small geiger tube with an electric field across it and an anode wire running down the center (see figure). They then waited (an unspecified time), but probably a month or so for much of the argon 37 to decay. Each Ar 37 decay emitted a low energy (2 kev) ionizing electron into the gas that traveled only a short distance (0.1 mm). The electrons it knocked free drifted to the collecting wire and coming from the same local region the electrons arrive at the wire at nearly the same time. The result was a current pulse from the tube with a fast rising edge, this pulse shape being the signature of a real Ar 37 decay.
In contrast most of the background pulses coming out of the geiger tube were the result of gamma rays coming into the tube and hitting electrons of the gas in the tube causing them to recoil strongly. These high speed, high energy 'compton scatter' electrons traveled a long way in the tube. Since free electrons were generated along a long path with varying distance to the wire, they arrived spread out in time, so the tube background current pules had a larger amplitude, but a slower rise time than Ar 37 decay pulses. Hence the electronic discriminator was designed to only pass pulses with fast rise times to the digital counter. How well the rise time discriminator worked can be seen in the figure below were only the counts in the pink zone were counted. All the green dots in the pink zone were Ar 37 decays, the other (red) dots were background noise pulses now no longer counted.
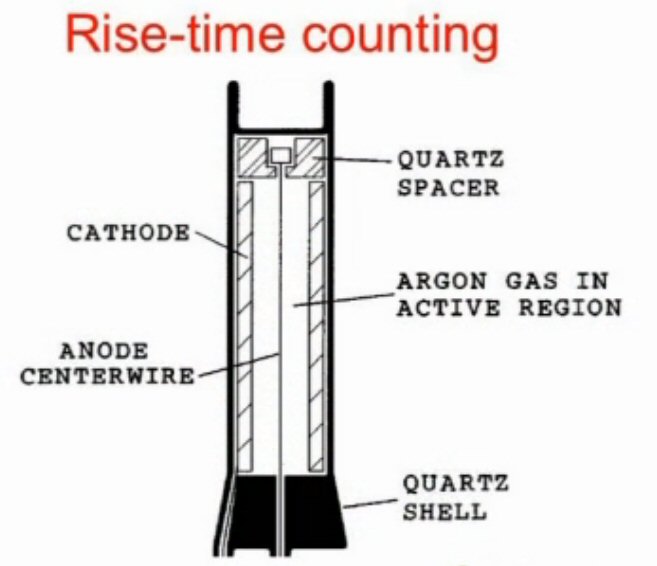 .
. 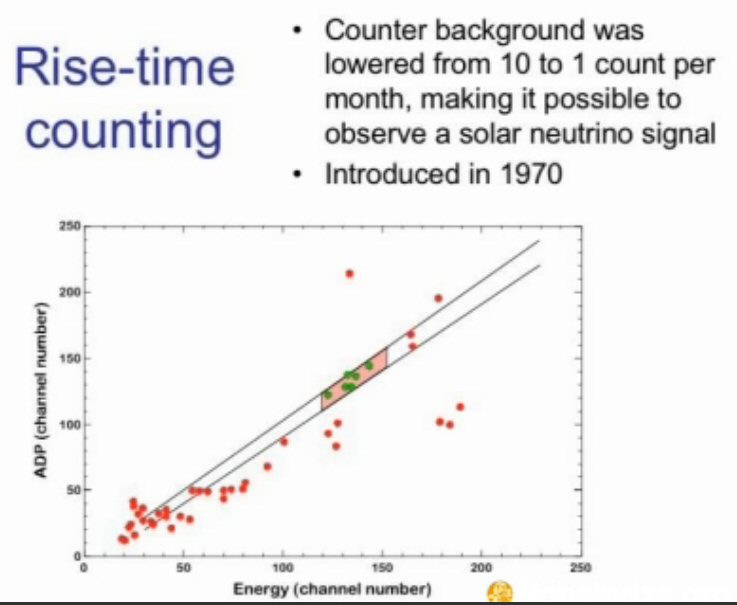
Rise time discriminator passed only pulses in pink
region,
Passed: green are Ar 37 decays
Rejected: red are background (gamma) pulses
(screen capture from Davis Homestake 2002 Nobel lecture)
This trick worked spectacularly well. The background count rate went from being about the same as Ar37 count rate to about x10 smaller, allowing Ar37 decay to be clearly seen and accurately measured. Later the experiment background rate was further quieted by surrounding the tank with water to reduce neutrons coming from the mine walls. Also before each tank flush a little Ar36 and Ar38 was added to the tank, so the efficiency of the argon flush could be checked (about 95%). Davis, the designer of the Homestake experiment, concluded his 2002 nobel talk by saying:
"The collision between standard solar model and experimental results ended in a spectacular way. Nothing was wrong with the experiment, nothing was wrong with the theory, something was wrong with the neutrinos!"Here's a look at the variability of Homestake's neutrino hits over 108 runs and nearly 25 years of operation. The vertical axis is marked off in terms of counts/day, but in terms of a typical 30 day run it can be rescaled to 0 to 45 counts/month. The data is pretty noisy and with only one data point a month it took a lot of years of operation to get a good value. Below shows the monthly eq Ar37 count ranged from about 0 to 38 with an average (red) a little below 15.
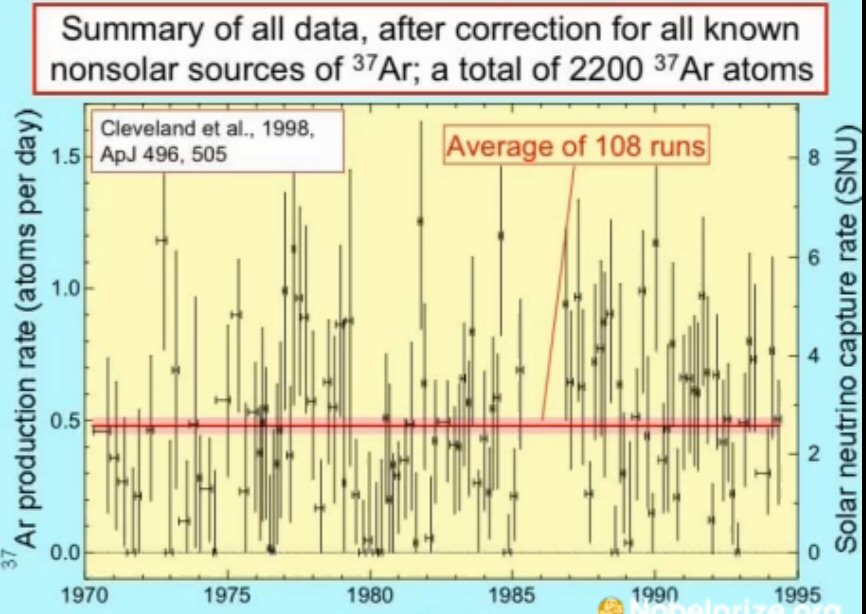
(screen capture from Davis Homestake 2002 Nobel lecture)
Super
Kamiokande water cherenkov detector (1996 to present)
Neutrinos are confirmed to be coming from sun and
a few neutrinos from a nearby supernova are detected
The original
Kamiokande detector in Japan was built in 1983 with 3,000 tons of water
and 1,000 phototubes not to search for neutrinos, but to look for proton
decay. This was a hot topic in the 1980's when proposed grand unified theories
predicted the proton might not be stable, so a lot of experiments were
launched to try and capture a proton decay. (Thirty years later proton
decay has never been observed in any of the decay experiments!)
Prior to Kamiokande the big neutrino detector(s) were 'radio-chemical' in their operation. Meaning they were designed to look for a few radioactive atoms that would accumulate over time due to a neutrino (n => p), or anti-neutrino (p => n), reactions in the target material. Kamiokande operated totally differently. It was an active detector that detected neutrinos in real time by sensing light flashes from electrons accelerated to near the speed of light by neutrino hits, and because the light comes out as cone, the direction of the neutrino can be determined.
In 1985 the detector was upgraded (how? my guess is they begin to look for different light patterns) to detect solar neutrinos. Kamiokande was the first of what the neutrino book calls active neutrino detectors. The energy threshold for neutrino detection in water based cherenkov detectors like Kamiokande is a few Mev, consequently it detects primarily a reaction in the sun's pp chain labeled on the solar flux chart as B8, which extends to 15 Mev. This is the beta+ decay of atomic element five boron (B8) to element four berrylium (Be8) as a proton converts to a neutron (p => n) emitting a positon and a (high energy) electron neutrino. Kamiokande detected about 54% (Super Kamiokande 48%) of the electron neutrinos that it was calculated it should detect (absent neutrino oscillation).
Supernova neutrinos detected
In 1987 the original
Kamiokande detected (sort of by accident) a few neutrinos from the supernova
in the largest satellite galaxy of the milky way, the large magellanic
cloud. Since the neutrinos arrived just 3 hours before the first light,
this provided important data both about supernova and speed at which neutrinos
travel. The Kamiokande detector was featured in a Nova show about the 1987
supernova. In 1988 Kamiokande began regular operation to detect neutrinos
from space and from the direction of the light cones strong evidence that
the neutrinos being detected were in fact coming from the sun.
Supernova triggered a different reactionSuper Kamiokande
The Nobel lecture describes how the 1987 supernova neutrinos were detected via a different reaction, described as: "anti-neutrino ve on p producing e+ plus neutron". Translation: Electron anti-neutrinos from the supernova triggered a charged current (p => n) conversion, probably the proton of a hydrogen, with release of a relativistic positron (anti-electron) and a neutron. I think the speaker said the positron was detected from its cherenkov radiation (prior to its annihilation).
Until recently the most famous big neutrino detector has been the Kamiokande detector(s) in Japan. They have been operating for 30 years, Inside it is a huge tank of water surrounded with photomultiplier tubes. It's been expanded and upgraded several times, and now with 11,000 multiplier tubes is known as the Super-Kamiokande detector. It's huge size is clear from this picture. The small orange thing in the center is two men paddling around in a rubber raft!
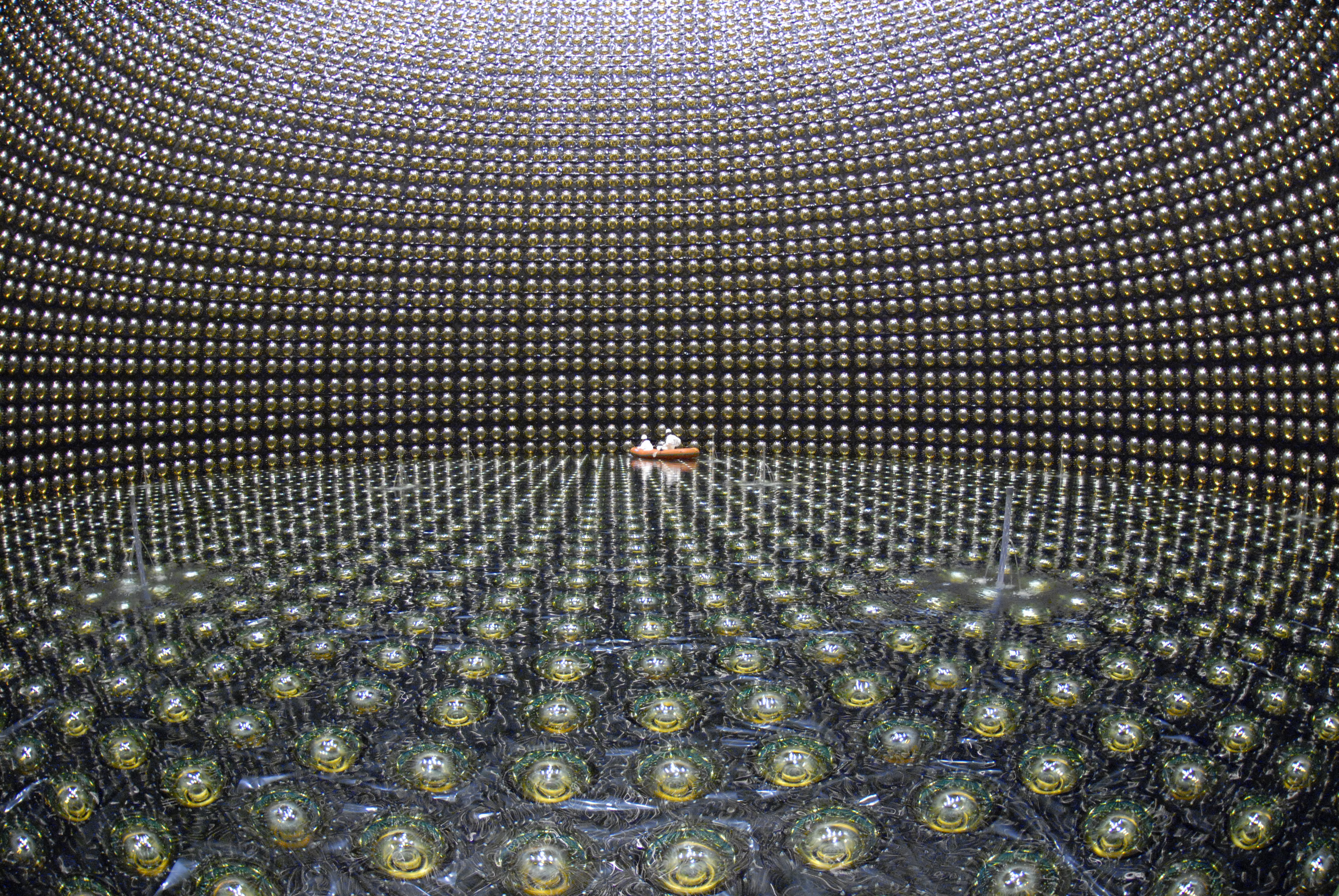
The 64 thousand dollar question is how they got this
huge tank down in a mine?
Did they weld it together from a jillion little pieces?
Notice the huge dia of these custom designed phototubes!
Kamiokande neutrino detection mystery
I ran into
a real mystery with this detector. Consider the following facts:
These three statements are not consistent. If Kamiokande works by detecting recoiling electrons (scatter), and this type of neutrino-electron interaction is not restricted to electron neutrinos, but works for all types of neutrinos, then how (the hell) could Kamiokande confirm the long standing problem of 'missing solar neutrinos' that Homestake had found? If Kamiokande's detection reaction works for all neutrino types, why didn't it find the calculated number of solar neutrinos?1) References give the operating principle of the detector as electron scatter, in other words what is causing the high speed electrons that are being detected is a hard, elastic 'hit' (or bounce) by a neutrino.2) Hyperphysics site (see figure above) shows electron scatter (recoil) from neutrino hits (via Z0) works for all types of neutrinos: electron, muon, and tau.
3) References say Kamiokande recorded only about 50% of the neutrinos it was calculated to detect (sans oscillation), thus confirming the 'missing solar neutrino' problem that Homestake found.
I was stumped, so I started researching. I listened to the video of the Nobel lecture by the designer of Kamiokande (2002 Nobel prize for physics), and he confirmed the detection principle was electron scatter of water electrons, that confirmed one. I've never known the excellent Hyperphysics site to make an error, and since this is just an energy-momentum transfer (via Z), probably a lot like a photon-electron collision, it made sense to me it would work with all neutrino types, that confirmed two. Multiple technical references stated that Kamiokande had a neutrino shortfall of about about 50%, confirming three.
Solution to the Kamiokande neutrino detection mystery
So I reread all
my technical neutrino references that I had dug up in three months, and
in one of them finally found the answer. The problem is with two, it is
oversimplified. It is true in principle that it works with all neutrino
types, but it turns out the probabilities (cross-sections) of a hit vary
a lot depending on the neutrino type, at least at the relatively
low energy levels of solar neutrinos. This technical neutrino reference
said that (at the energy of solar neutrinos) a scatter hit to an electron
was x6 more likely to come from an electron neutrino than a muon or tau
neutrino. That's a game changer.
Kamiokande, a water cherenkov detector, is therefore primarily an electron neutrino detector with something like 75% (6 out of 8) of its hits coming from electron neutrinos even though its detection mode is scatter. This is confirmed in a viewgraph of the 2002 Nobel lecture by Kamiokande's designer where he decribes the solar detection mode as "ve -e scattering". (I had initially missed the 've' (electron neutrino) reference. Also the Slac report gives the Kamiokande detection equation as [ve + e- => ve + e-], which is a scatter reaction with electron neutrinos.
So this is how Kamiokande could confirm the Homestake missing solar neutrino finding, and even probably explains how its shortfall was less than Homestake's (50% detection vs 33% detection), since it was likely getting some muon neutrino and tau neutrino hits.
Email to founder of Hyperphysics Dr. Rod Nave, Georgia State Univ (2/17/14)---------------------------------Prof Nave
As a retired engineer I want to thank you for Hyperphysics, it is a wonderful piece of work, a great reference. Here is a little feedback on your neutrino pages. Neutrino detection is moving target, your neutrino detection pages could use some updating. However, the main purpose of this email is give you some feedback on a figure on this page: http://hyperphysics.phy-astr.gsu.edu/hbase/particles/sno.html#c1 showing neutrinos interacting with electrons via Z (on right), where it is marked that the reaction works for all neutrino flavors (vx).It's not wrong, but it is misleading for solar neutrino detection. It shows a neutral current (bounce hit) of a neutrino off an electron (via Z) which causes electron recoil. This is the detection reaction used by large water cherenkov detectors like Super Kamiokande in Japan. It's widely reported that Super Kamiokande found only about half the neutrinos from the sun as calculated thus confirming the 'missing neutrino problem' first found by Homestake. I looked at your figure and was baffled. If the water cherenkov detection reaction works for all three flavors of neutrinos, how did Super Kamiokande have a shortfall?
It took my quite a bit of digging to figure this out. Turns out that the cross-sections of this reaction are very different for different neutrino flavors. An electron neutrino is about x6 times likely than a muon neutrino or tau neutrino to hit an electron. Hence Super Kamiokande is really (mostly) an electron neutrino detector because it is far more sensitive to electron neutrinos than the other two types. If you look at the video of the 2002 physics nobel speech by the designer of Super Kamiokande ( Masatoshi Koshiba), you will see he identifies the neutrino in the detection equation as ve (electron neutrino) not vx as in your figure.
I know you need to simplify to make Hyperphysics readable and useful, but I wanted to provide some feedback on this (potentially) confusing point.
Don Fulton
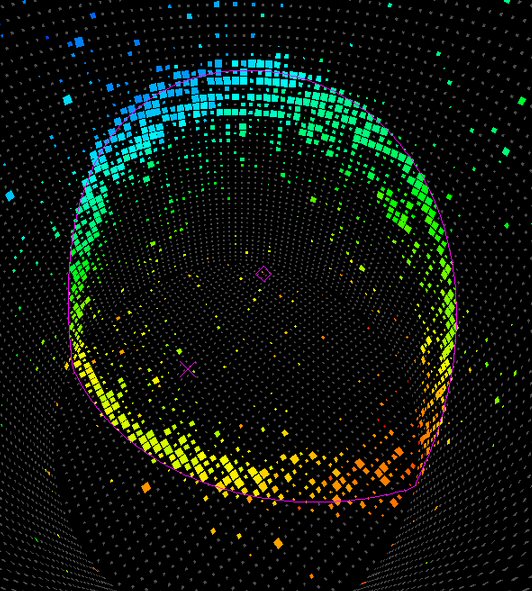 .
. 
Real Super Kamiokande data from a high energy neutrino
beam hits (blue is earlier time)
(left) muon light cone
(time scale = 162 nec, 603 Mev)
(right) electron light cone
(time scale = 130 nsec, 492 Mev)
(reference: speed of light (vacuum) is
about 1 nsec/ft)
(source --- http://www.ps.uci.edu/~tomba/sk/tscan/compare_mu_e/)
What is happening above? (update 2/7/14)The original Kamiokande tank held only about 6% as much water as above surrounded by 1,000 photomultiplier tubes. It was the original, smaller tank that in 1987 detected a few neutrinos from supernovae in the Large Magellanic cloud (real coup). The current, much bigger tank is able to measure the direction of neutrinos, and it has confirmed most neutrinos come from the direction of the sun. While I read it has some sensitivity to solar muon neutrinos, which can also bounce off electrons, to what extent it can separate an electron neutrino hit from a muon neutrino hit at solar energy levels I don't know. As the data above shows, it can separate high speed muons from high speed electrons in the water by the shape of their light cones, but I doubt this is relevant to the solar neutrino study. Still I read that in 1998 it collected the data confirming solar neutrino oscillation, which in turn confirmed that neutrinos, long assumed to be massless, have a little mass. The amount of mass is (probably) just a fraction of an eV, in comparison an electron has 511,000 eV of mass.
Rereading this material I realized I didn't understand what above data represents, especially the 'muon' data. Was this a) light cone of an electron hit by a muon neutrino, or b) light cone generated by an actual (high speed) muon? I wasn't sure. When I went back to reread the original reference, I found it unclear. It's titled was 'neutrino events', but it read as though the muon cone was from an actual muon. A muon is essentially a heavy, unstable electron, with x207 times the mass and a 2 usec lifetime. If it was a muon, where did the muon come from?Digging deeper I think I have the answer. It's a real muon. These are neutrino triggered events, but these incoming neutrinos have very high energy, hundreds of Mev, and at this energy more complicated reactions can occur than at the few Mev level of solar neutrinos. In particular such high energy neutrino can turn into other particles which in turn may (rapidly) decay into high speed muons or high speed electrons. Ok, so this explains how muons get into the tank of water, and it probably means the muon light ring above comes from an actual muon traveling in the tank. The reference identifies the particle the neutrino creates as a 'pi-zero', which with a little digging I find to be a pion, a combination of a quark and anti-quark.
This reminded me of a picture I found in Wikipedia (neutrino) (below) showing the first neutrino ever seen in a cloud chamber. Notice the markings on the photo. A neutrino comes in, a proton recoils and and mesons (pions) come out. The three lightest mesons are called pions with rest mass energy about 135 Mev and decay in 20 nsec or less. Wikipedia says the uncharged pi-zero pion decays to (gamma) photons, but the other two charged pions do decay to muons (plus anti-muon neutrinos). So not sure what the exact reaction is at these high energies in the Kamiodande detector, but it might be that a neutrino hits an electron (or proton) picking up charge 'transforms' into a charged pion, which then very quickly decays to a relatively long lived (2 usec) muon, which the phtototubes detect as it slows down. (Or maybe the pi-zero decays to gamma photons, which start a cascade ending in muons.)

Cloud chamber filled with liquid hydrogen
(source -- Wikipdia Neutrino)In the photo a neutrino, obviously a high energy neutrino, is shown hitting a proton causing the proton to recoil with a u-meson and pi-meson also coming out. Mesons (pions) can be generated from neutrinos because they consist of a quark and anti-quark, and my guess is the outgoing pion(s) may be charged or uncharged depending on what the neutrino hit. (This reaction may be what I read is called 'deep neutrino scattering'.)
Super Kamiokande atmospheric neutrinos
Super Kamiokande
also studied atmospheric neutrinos, and this work provided some evidence
of neutrino oscillation. (Davis in his 2002 nobel lecture made specific
reference to this.) The decay trail of pions (etc) created by cosmic rays
(usually high speed single protons) hitting gases of the upper atmosphere
continually produces neutrinos that hit earth, and this neutrino flux contains
both muon and electron neutrinos. What's interesting is that dominant decay
path is known to produce two muon neutrinos for every electron neutrino.
Thus neutrinos coming from the atmosphere, meaning not from the direction
of the sun, should show a 2:1 ratio between muon and electron neutrinos.
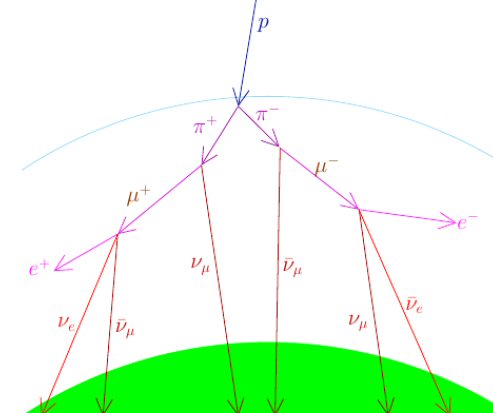
Atmospheric neutrino creation by cosmic rays.
Charged pions decay into muons with release of a muon
neutrino.
Muons decay to an electron (or positron) with release
of both a muon neutrino and an electron neutrino.
Ratio of (muon neutrinos to electron neutrinos) =
2
(source --- MIT Phd thesis http://dspace.mit.edu/bitstream/handle/1721.1/52783/528746983.pdf?sequence=1)
Super Kamiokande found this not to be the case, they got a different ratio. They got the expected # of electron neutrinos, but there was a deficit of muon neutrinos that varied with distance and energy. This was evidence that neutrinos might have changed flavor traveling from the upper atmosphere to the detector. Atmospheric neutrinos provide a nice natural setup for examining the flavor vs distance character of neutrino oscillation, because the distance to the atmospheric neutrino sources varies with angle at the detector. The screen (below) shows the Super Kamiokande atmospheric data, and it concludes that the measured atmospheric muon neutrino deficit is evidence that muon neutrino from the atmosphere are oscillating to tau neutrinos during their travel from the atmosphere to the detector.
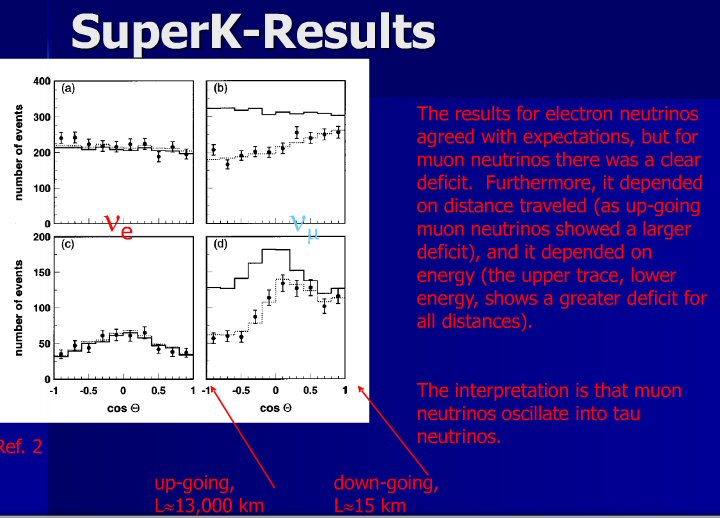
screen capture from a 2004 talk on atmpospheric neutrinos
left: electron neutrinos match expectation
right:muon neutrinos show a deficit that increases
with distance
source -- http://www.docstoc.com/docs/122541453/Atmospheric-Neutrinos)
How do detectors really detect (atmospheric) neutrinos?
For a long
time I didn't really understand how the big neutrino detectors in their
study of atmospheric neutrinos were able to distinguish muon neutrino hits
from electron neutrinos hits. Most references say little to nothing about
this. However, I found some info in an MIT Phd thesis on atmospheric neutrinos
(link below image above). The short answer is they don't need to. What
the detectors are actually seeing (getting light from), he says, is muons
that result from the decay of muon neutrinos. Ok, muons are easy to detect
optically because they travel long and straight, probably all the way through
the detector, and produce a well defined cone (image from Super Kamiokande
of a muon cone is included in this essay).
Wait a minuteSo picture the thesis (and other sources) paint for muons is this. Muons created from decay of pions in the atmosphere can penetrate several km below the surface and some will penetrate into underground detectors. The neutrino detectors are not really interested in these muons, effectively they are noise, because they have not come from neutrinos. These muons are downward traveling. What the detectors do is look for upward traveling muons.
Muon neutrinos decay to muons? I see problems here. For one thing all neutrinos have no charge, so a muon neutrino could only decay into a muon and anti-muon pair. But there's another issue, energy. Muons are x207 times heavier than electrons meaning they have rest mass energy of about 100 mev, so this type of muon neutrino decay only works for neutrinos with > 200 Mev of energy. Do atmospheric neutrinos have this amount of energy? Surprisingly they do. A neutrino flux chart in this essay shows that atmospheric neutrinos start at about 100 Mev and go to 1 Gev and still higher, so it is perfectly possible from an energy and charge viewpoint for muon neutrinos to decay into muons (and anti-muons).
Version #1 -- The explanation given for the source of these upward traveling muons would be this: They come from (high energy) muon neutrinos created in the atmosphere on the opposite side of the earth that have traveled through the earth, and (presumably) when they get within a few km of the detector have 'decayed' into muons, which then pass through the detector and are detected. (Seems a little unlikely, I need to see if I can confirm this picture.)
Version #2 --- In a technical paper on atmospheric neutrinos (detected in Super Kamiokande) I find a little different story. The paper is quite technical, but it appears to be saying that the target of the muon neutrino is the nucleus of the oxygen of the water. So my guess is that this is charged current reaction (n => p) with a muon neutrino coming in and being absorbed and a muon coming out carrying away the negative charge. This means the muon is created right in the tank. A big advantage here is that the energy threshold is cut in half to 100 Mev (maybe) since only one muon is created. (This version sounds more plausible to me.)
However, reading deeper into the paper the reaction inside the oxygen nucleus appears to be quite complicated,. They describe it as quasi-eleastic scattering (whatever that is). The energy level of atmospheric muon neutrinos they say Super Kamiokande is detecting is about 1 Gev, so there is plenty of energy to make muons. At these high energy levels (and higher, like at IceCube) reactions are so complicated that no one but a specialist can really understand it. But as I scan the equations it looks a lot like what happens in the atmosphere. The muon neutrino interaction with the oxygen neutron looks like it creates pions (plus n and p), and as shown in the first branch in the atmospheric figure above pions decay into muons and (another) muon neutrino, which would just escape. The (high energy) muons created by the pion decays (in or near the oxygen nucleus) are what is being optically detected. This makes sense.
Kamiokande later confirmed its atmospheric work hinting at neutrino flavor changing with the K2K experiment. This was a muon generated in a particle accelerator 250 km from Kamiokande and aimed at it. A scaled down version of Kamiokande (1 ton vs 50 ton of water) was built near the source to provide a comparison. Wikipedia (K2K) says the experiment looked for vu to vtau changes during the travel. (This statement I think conflicts with Cern's recent claim that they are the first to try and detect vu to vtau changes in a muon neutrino beam.)
Don't know all the details, but to do this Kamiokande must have been able to both detect and distinguish muon neutrinos from electron neutrinos. I know it's neutrino electron 'bounce' detection reaction does respond to muon neutrinos (with about 1/6th sensitivity of electron neutrinos). Do muon neutrino hits create muons, or was Kamiokande able to separate out from light cone patterns electrons recoiling from muon neutrino hits? I don't' know the answer to this one.Super Kamiokande phototube accident
How do water/ice neutrino detectors really work?
What is the
neutrino target of the water (or ice) molecule in Kamiokande and IceCube?
Since these detectors look for flashes of cherenkov radiation and their
descriptions talk of relativistic electrons (muons too for Icecube), I
had early assumed the source of the high speed electrons was an
(n => p) reaction which is known to output a high speed electron. If this
is right, it must mean the neutron converted is in the nucleus of the oxygen,
since H of regular water has no neutrons. But the neutrino book (and Wikipedia
vaguely) refer to neutrino induced 'electron scattering'!
(Update 2/7/14)Is the neutrino interacting directly with an electron of the water, either in the hydrogen or the oxygen? Yup, it's apparently electron 'scattering', because the neutrino book specficially says "electrons struck my solar neutrinos recoil in the direction of the sun to the earth", and it and another detailed neutrino report (SLAC Stanford neutrino report) gives the detection equation for the water cherevkov Kamiokande detectors as:
My early views of how neutrinos reacted in water/ice neutrino detectors were very simplistic, based as they were on the only neutrino detection method I then knew, which I had learned about from looking at feyman diagrams, a beta like (n => p) conversion with release of an electron.For one thing neutrino energy needs to be taken into account. IceCube doesn't work like Kamiokande because the IceCube is designed to detect neutrinos with much, much higher energy (from deep space) than solar neutrinos, which Kamiokande normally detects. Once neutrino energy exceeds 135 Mev pions can be generated and their decay can lead to muons and gamma photons. IceCube data shows long trails caused by high energy muons traveling through the ice. How exactly neutrinos generate these muons in IceCube I never see explained. Muons can be seen in Kamiokande too, but this is only when it is receiving a beam of high energy neutrinos generated by a particle accelerator.
It took me a while to understand that neutrinos at the few Mev solar neutrino energy level can react a second way, they can (effectively) just 'bounce' off an electron or quark in a proton or neutron in the nucleus causing them to recoil. Electrons being only 0.511 Mev when 'hit' by a 5 Mev solar neutrino easily obtain enough momentum and energy from the collision to recoil at near the speed of light (in a vacuum), which can be above the speed of light in water, thus generating a cone of cherenkov (blue) light. The detector people never seem to detail which electrons of the water molecule are knocked free and detected, but my guess is that they are probably the hydrogen valence electrons, though there's probably enough energy to knock free the outer electrons of oxygen too. And this is really how water based cherenkov detectors like Kamiokande work.
ve + e- => ve + e-
Looks pretty boring, the output is the same as the input. What this means is that some fraction of the incoming neutrino's energy and momentum is transferred to the electron it hits, making its speed relativistic, while the balance of the energy and momentum is carried off by an outgoing neutrino, hence this can be characterized as a 'bounce' (or scatter) reaction. The detection threshold of Kamiokande was pretty high at 7.5 Mev (x15 the rest mass energy of an electron (0.51 Mev)) and is about 5 Mev for super Kamiokande. Neither report says if the electron target is in the hydrogen or oxygen (or both) of the water.
Tidbit from the Slac Stanford reportSudbury heavy water neutrino detector (1999 - 2006)
-- Super Kamiodande records a solar neutrino event about every 30 min (11,000 events in its first 27 months of operation), which is about x100 times higher hit rate than the earlier Homestake detector (30 min vs two days)!
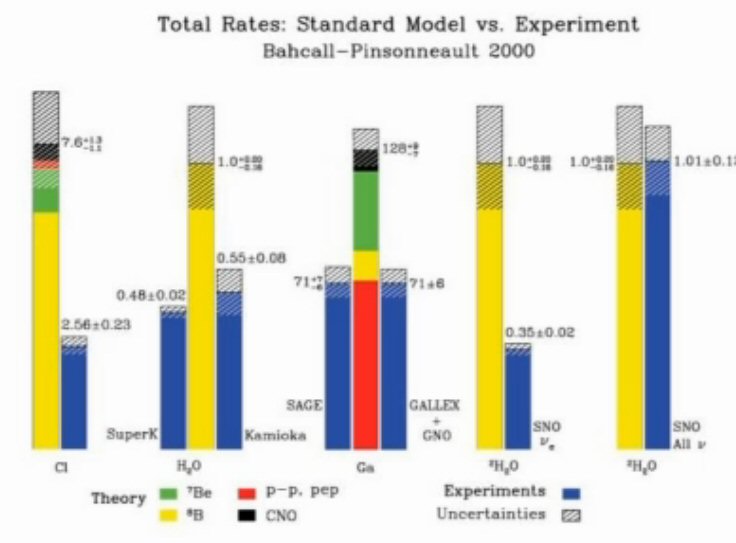
Measured (blue) vs calculated (yel) neutrino rates
for all major neutrino detectors up to year 2000.
Only Sudbury (SNO) in its deuterium fission mode (right)
shows the measured rate matching the calculated rate.
(screen capture from Davis Homestake 2002 Nobel lecture)
The Sudbury neutrino detector, another big tank of water (1,000 tons, 10,000 phototubes) in a deep mine, operated from 1999 to 2006, but it was different from the Kamiokande in that it was not regular water but heavy water (D2O). Heavy water was available in Canda in large quantities as they had a heavy water plant to make it for use as moderator in Candian power reactors. Sudbury was designed to capture solar neutrinos with a capture rate of about ten hits per day.
The key to Sudbury as a neutrino detector is that the proton and neutron in deuterium are 'barely' bound together (2.22 Mev binding energy) and presenting a relatively large cross-section it can be disassociated, i.e. fissioned, by a bounce hit from solar neutrinos. As this is merely an impact hit with no nuclear transformation it works for all three flavor of neutrinos.
Its operation is described by the equations below showing it could sense neutrinos in two basic ways: charged current (atomic # change) reaction, and a neutral current (scatter/bounce) reaction. For the former the neutron target was not in the oxygen of water, but the (neutron + proton) nucleus of the deuterium. Separating these two modes might have been a little tricky since both equation 1 and 3, via two different mechanisms output a high speed electron. Equation 2 outputs a free neutron. Below are the Sudbury heavy water neutrino detection equations from the Slac Stanford report.
"charged current" (n => p) ve + d => p + p + e- (9 events/day) (1.44 Mev)Translation
"neutral current" vx + d => p + n + vx (3 events/day) (2.25 Mev)
"neutrino elastic electron scattering" vx + e- => e- + vx (1 event/day (75% ve)#1 Target deuterium neutron undergoes a charged current (n => p) conversion emitting a high speed electron
Detect relativistic electron light cone#2 Target deuterium neutron (or nucleus) split (fissioned) by a neutral current hit causing recoil
Detect free neutron with scintillator (salt)#3 Target electron of hydrogen (?) in water accelerated to high speed by a neutral current hit causing recoil
Detect relativistic electron light cone-- Hit statistics. The event counts above provides the first statistics I have seen on the relative probabilities of different types of neutrino hits. What is interesting is that in a simple atom like deuterium (one proton, one neutron, one electron) a neutrino appear to be about ten times more likely to 'hit' one of the six quarks in the nucleus than the one electron. (This is in spite of the fact that this reaction is only triggered by electron neutrinos, which make up 1/3rd to 1/2 the total neutrino flux.) 9 of 13 hits are to the neutron with atomic conversion, 3 of 13 scatter off the nucleus, and 1 in 13 scatter off the electron.
Most technical report on neutrinos when discussing Sudbury focues on the first two equations, and from the event/day numbers above the 3rd equation is clearly a secondary contributor. It is a scattering of an electron neutrino off an electron in the heavy water (D2O), the same electron-scattering process that is detected by Kamiokande. While the equation shows it responds to all neutrino types (vx), this is misleading, it is mostly an electron neutrino detector. The reason is that at solar neutrino energy levels the capture scatter cross-section for ve is x6 larger than for vu or vtau.
In other words with heavy water a neutrino can interact in three detectable ways: (n => p) quark conversion in the deuterium nucleus, bounce off the deuterium nucleus that splits the nucleus apart freeing the neutron, and bounce off an electron (probably a hydrogen electron) causing it to recoil at relativistic speeds.
The key result of Sudbury, according to the book by Lincoln Wolfenstein and Joao Silva, is that the reactions sensitive to all neutrino types (#2 & #3) detected for the first time the number of neutrinos calculated to be hitting the earth. These reactions works for all types of neutrinos because they are bounce-recoil reactions, and the key reaction (#2) knocked a neutron free from deuterium, which with help of a scintillator could be optically detected. In contrast reaction #1 only responds to electron neutrinos. This is because the neutrino is absorbed by a neutron (n => p), and since an electron comes out, it means an electron neutrino had to have been absorbed. Reaction #1 like earlier detectors found only about 1/3rd of the calculated number of neutrino hits. It was the x3 higher ratio of hits (from the same experiment) of reaction #2, which could detect all neutrino flavors, over reaction #1, which could detect only electron neutrinos that was taken as strong evidence for neutrino oscillation as the explanation for missing solar neutrinos.
Missing calculationSudbury's two operating modes
All references simply note that equation #2, the breakup of deuterium, is (apparently) equally sensitive to all types of neutrinos. This must fall out of cross-section calculations, but no reference I have seen details this. This is kind of an important, because the Sudbury's importance in detecting the missing solar neutrinos depends on this. It's probably something to do with the fact that the rest mass energy of the deuteron is much higher than the energy of the incoming neutrino, and/or maybe it's also related to the fissioning energy of deuterium not being very high. In the case of neutrino bounces off much less massive electrons, the reaction works in principle for all types of neutrinos, but the cross-sections strongly favor a hit by an electron neutrino.
Sudbury has a 3rd detection mode
Sudbury might have a 3rd detection mode because surrounding (acrylic plastic) heavy water tank is a tank of regular water, and from the diameters it looks like the there is x3 more ordinary water than heavy water. Wikipedia says neutrino reactions in the ordinary water of the outer tank can be picked up by photodetectors too, but there is little information about how cherenkov detection in the ordinary water are used. I always figured it was a screen of some sort or a way to subtract out background, and maybe that's what they mean.

What is of interest here is that the detector was able to respond not just to electron neutrinos, like previous generation of detectors, but to muon and tau neutrinos too. It was the ratio of these two reactions (properly scaled for different cross-sections) that was expected to provide information about neutrino oscillation. If some solar neutrinos were coming in as muon or tau neutrinos, then reaction #2 would have more hits than #1, which the equation shows only responds to electron neutrinos. (The reason I think is that an incoming electron neutrino can only generate an electron. If a muon neutrino comes in and triggers a (n => p), it will output (very likely) a muon, but the detector is designed to detect only relativistic electrons not muons, which are 200 times heavier.)
ve + H2 => p + p + e-
(n => p), detect electron
electron neutrino + d (deuterium) => p + p + e-
vx + H2 => p + n + vx
deuterium fission, detect neutron
any neutrino + d (deuterium) => p + n + any neutrino
The first equation shows a charged current electron neutrino induced (n => p) conversion, and it is clear the neutrino target for this reaction is the neutron of the deuteriums. What is a little less clear is what exactly 'p + p' means. My guess is that a relativistic electron coming out and two bare protons, which makes sense since there is no stable helium isotope without neutrons. The protons are unlikely to be relativistic because there rest mass is nearly 1 Gev, far higher than the Mev energy the neutrino brings in. (Wikipedia gives the half life of He2 as 0 seconds!) This reaction is only triggered by an electron neutrino.
The second equation is drawn like it might be an (elastic) scattering equation involving the nucleus, i.e. a neutrino hitting a quark in either the neutron (or maybe the proton) (see diagram below) transfers energy and momentum to the particle containing the quark and then keeps going.
Why are hits to the neutrons in oxygen not detected?I just noticed that the primary Sudbury heavy water reaction is the reverse of the solar pp chain neutrino emitting reaction! The report gives the primary pp chain reaction as the first equation below (d is pn, deuterium):
One minor mystery (to me) that I never see anything written about is why charged current neutrino hits to neutrons in the oxygen of heavy (or regular) water don't seem to be detected. I would think the same physics would apply, that a relativistic electron would be emitted (and could be detectd). If the picture is the electron is 'created' in the nucleus, does the much higher positive charge of the oxygen nucleus compared to hydrogen nucleus perhaps greatly slow down, or even prevent, the electron from escaping?Confirmation?
I researched this point and found my guess is right (or close to right). A (popular science) book by a major neutrino researcher says this: "The neutron in oxygen (of water) is too tightly bound to be excited by an electron neutrino." ('Exploring Fundamental Particles' by Lincoln Wolfenstein and Joao Silva, 2010, p181). I can understand how a neutron bound in a big nucleus might not recoil much from a neutrino scatter, but I don't see how (or am surprised that) the probability of a capture of a neutrino by one of the neutron quarks (and consequent emission of a relativistic electron) would be affected by how tightly the neutron is bound in the nucleus.sun pp chain p + p => d + e+ + ve (p => n)
add e- to both sides p + p + e- => d + energy + ve
reverse and it's Sudbury energy + ve + d => p + p + e- (n =>p)
Deep inelastic scattering?Deuterium binding energy
But is it this simple, or is this 'deep inelastic scattering' (see below), which was used at CERN years ago to provide evidence that quarks actually exist? ('Deep' here implies high energy neutrinos) The energy and momentum a neutrino transfers to a (single) quark excites the whole bag of quarks and gluons that is a neutron or proton. At CERN it was observed that (at high enough energies) this could split up the particle into a shower of short lived particles (pions, kaons, etc).
atomic mass unit
1.0000
931.49432
proton
1.00727646681
938.27231
why is this not 1?
neutron
1.00866491600
939.56563
------------------------- ----------------
2.01594138281
1,877.83794
deuterium
-2.01355321272
-------------------------
0. 002388 x 931.5 Mev (atomic mass unit) = 2.22 Mev
check
Wikipedia (deuterium) says that deuterium is "barely bound" and that it can indeed be "disassociated" by neutral current (hits) of neutrinos as demonstrated in the Sudbury neutrino detector.
About deuterium and heavy waterSudbury with all its phototubes and transparent media is detecting cherenkov radiation. A Subury site describes it as "a heavy-water Cherenkov detector". Since reaction #2 does not output an electron, the source of the cherenkov radiation I first assumed must be a relativistic proton, but with a little thought I realized there is a BIG problem. To make a proton relativistic would mean the neutrino energy would need to exceed the proton rest mass and this is x2,000 higher than for an electron, too high for a solar neutrino.
Harold Urey finds evidence for a heavier isotope of hydrogen from spectroscopy in 1931. This is hard to explain at the time, since it is not until a year later than the neutron is discovered as a sub-atomic particle. It is Urey who in 1934 coined the words 'deuterium' and 'tritium'.The general rule is that chemistry is little affected by isotopes of elements dominated as it is by electron bonding, but heavy water is slighly toxic to eukaryotic creatures, and more surprising substituting heavy water for regular water at 25% causes problems and at 50% death can result a few biological functions begin to fail.
How reaction #2 is detected
A power point
presentation on neutrinos (neutrinostory.ppt)
says Sudbury detects a light flash from the electron (reaction #1) and
a light flash from the neutron (reaction #2), so my guess is the
neutron was detected with a flash from a scintillator material excited
by a moving neutron. The
1995
neutrino report says this: "In addition to the unique reactions of
neutrinos on deuterium, Sudbury will observe the same neutrino electron
scattering process that is detected by Kamiokande".
A technical Sudbury report says the neutron is captured by Cl 35 (salt is dissolved into the D2 and that (in turgid english) results in gamma rays, an electromagnetic shower, and the detector captures the cerenkov radiation from the shower. But this doesn't check out right. Most chlorine in salt is Cl 35, and with neutron capture would become Cl 36, which is unstable, but it has a 100,000 year lifetime and only 2% of its decay is (I think) via gamma ray emission. A few sentenced down it implies that the gamma rays might be coming from the Cl 35 capture, but I don't understand what triggers the cascade. Maybe this is wrong, because it might have been an early method that was rejected. Also (at least originally) reaction #1 and #2 were to be done months apart. For #1 boron was put into the water to absorb the neutron and for #2 salt was put in to capture the neutron and (somehow) trigger a cascade. Scintillators were one neutron detection option looked at.
IceCube
world's biggest neutrino detector --- a neutrino telescope (2010
to present)
Designed to detect super high enegy neutrinos from
deep space
The world's
biggest neutrino detector is now IceCube. 1 cubic km (!) of deep ice in
Antarticia outfitted with long strings of photodetectors, built with funds
from the National Science Foundation (270 million). It works pretty
much like Kamiokande, looking for light flashes from high speed electrons
(and its cousins) traveling above the speed of light in ice. The
structure of this telescope was built by mother nature. There have been
light flashes occuring in the ice of Antartica for eons. To make it a neutrino
telescope all that had to be done was melt 86 holes, about a mile deep
arranged in a grid pattern, and insert in each (in the lower 1 km) a long
string of photdetectors (5,000 total) to look at the light flashes.

IceCube Neutrino Observatory at the Amundsen-Scott
South Pole Station in Antarctica
(guts of the telescope are in ice below)
This neutrino detector has been designed to detect very high energy (> 1 Tev, or 10^12 Ev) neutrinos from astronomical objects deep in outer space. It can determine the type of neutrino (electron, muon, tau, and presumably their aniti-neutrino versions), measure its energy, and to within about 1 degree determine the direction from which it comes. "IceCube is more sensitive to muons than other charged leptons, because they are the most penetrating and thus have the longest tracks in the detector. Thus, of the neutrino flavors, IceCube is most sensitive to muon neutrinos."
From a talk by a designer of an underwater neutrino detector I learned the following tidbits: 'Observation of high energy neutrinos is mainly observing the muon that results from charged current interactions.' 'Muons travel straight until they run out of energy, and a 1 Tev muon will travel 2.5 km in water. High energy electrons scatter multiple times off electrons, they produce sort of a local shower, so no good direction information. Taus decay so fast they make showers too.In its first two years it has recorded 28 neutrinos with energy above 30 Tev include two with 1,000 Tev (1 Pev) of energy. This is far higher than any particle accelerator, the CERN Large Hadron Collider max energy will be 14 Tev. (As I write, there is no details yet as to the neutrino source as data analysis is not yet complete.) A small section of the detector has more closely spaced light sensors to detect lower energy neutrinos. I read IceCube registers 100,000 atmospheric neutrinos per year, which is a flash every 5 minutes. (It's not clear if the atmospheric neutrinos are making muons in the ice, or if muons created in the atmosphere are making it deep into the ice into the detector, probably the latter.) To help filter out the atmospheric muons the telescope is set up to look for muons traveling upward triggered by neutrinos that have come through the earth.Just as an incoming electron neutrino absorbed by a quark outputs an electron, I suspect the same sort of thing can happen with a muon, i.e. a muon neutrino absorbed by a quark outputs a muon. However, it may be more complicated than this. I read that real high energy neutrinos hitting a proton or neutron can affect not just a single quark but all the gluons inside, the result being (two quark) pions are created and (charged) pions decay to muons. This is how muons are made in the atmosphere from cosmic rays.
The 'noise' level in IceCube is quite astounding. Wikipedia (icecube) says there are million muons in the detector for every neutrino induced muon. Most of these atompheric muons are downward traveling having been created in the atmosphere above the Antarctic. Even most of the upward traveling muons are created by upward traveling neutrinos that originate in the atmosphere on the other side of the earth. So IceCube filters by direction and energy to try and separate out deep space neutrinos.
IceCube reports on first two years of data
IceCube has
reported on their first two years of data: 28 neutrinos all from outside
the solar system. Some come from the direction of the center of our galaxy
(Milky Way), but a lot of them don't making it likely they come from outside
our galaxy. The NYT article on the IceCube paper points out this telescope
is opening a new window onto the universe, that with the exception of two
dozen neutrino detected in 1987 by three neutrino observatories from the
1987 supernova in the large Magellanic cloud, all neutrino detected prior
to 2010 have been local, most from our sun or neutrinos created by cosmic
ray induced reactions in the upper atmosphere (decay of two quark pions
and kaons emit neutrinos). IceCube does not detect these neutrinos, which
are thought to be rare above 60 Tev. The reported energies of the 28 neutrinos
ranged from 30 Tev to 1,200 Tev.
Energy perspectiveIceCube was specifically designed for much higher energy neutrinos (that it was hoped) would be coming from distant sources. No one knew if really knew if they existed until this telescope was built to look for them, and they do exist. One current puzzle is that theory would suggest neutrinos of still higher energy than have been found should exist and a few by now should have been detected.
To put the IceCube neutrino energy in perspective the energy threshold of cheerenkov water detector Super Kamiokande is 5 Mev and the solar neutrinos it primarily detects top out at 18 Mev. So IceCube operating in the Tev range is detecting neutrino 'hits' that are vastly more energetic, with something like 100 million times more energy!
No high energy neutrinos found?The capture capability of IceCube over previous neutrino observatories is revealed by this observation from one of the IceCube team. Even though IceCube is "not tuned to find the low-energy neutrinos from supernovas in the galactic neighborhood", if a supernova like 1987 went off again at the same distance (165k light years), the number of neutrino detected would be not two dozen, but 100,000.
Wired magazine has an interesting background story. In its first year of operation while a neutrino was being detected every six minutes not a single high energy neutrino that the telescope was designed for had been discovered. The IceCube scientists were getting frustrated. It turned out to be an issue with data analysis. The IceCube team hadn't yet figured out what they should be looking for. When sort of by accident they found two high energy neutrinos, they combed back through their first year data and combined with data from a second year came up with a total of 28 neutrinos which they describe in the Nov 2013 paper.
High energy dection physics
"The method
of observing high energy neutrinos consists mainly of observing the muon
that is produced from charged current interactions" (From Nestor a planned
underwater observatory, but it seems to sum up what IceCube is doing.)
Icecube says high energy deep space neutrinos whether they produce an electron, muon, or tau, all produce a cascade, i.e. the first high energy lepton soon makes others. The key to detecting a muon is that it leaves a long path in the ice because at x208 times the mass of an electron it is very penetrating. Also at 2.2 usec (stretched by time dilation) it lives long enough to go thousands of feet. On the other hand the tau, while very heavy, has a lifetime of 10^-13 sec, so it decays almost immediately and only its cascade can be seen.
Momentum is transferred from the neutrino to the muon, so the path of the muon points back (sloppily, +/- 1 degree) to the region in deep space from which the neutrino came.
IceCube detection procedures
The relevant
feynman diagram(s) for neutrino detection is a neutrino capture (presumably
by a neutron in the nucleus of the ice's oxygen) triggering a neutron-to-proton
conversion with ejection of high energy leptron, presumably the leptron
carring off mostof the energy of the neutrino. The IceCube specs say an
electron neutrino ejects an electron, a muon neutrino ejects a muon, and
a tau neutrino ejects a tau muon, which are probably the most likely events.
IceCube video says something different!IceCube neutrino energy is vastly higher than solar neutrino energy
IceCube telescope has a YouTube video. In it an IceCube guy (unidentified, but speaks like a phyicist) says the target of the neutrino is a proton, and adds (sort off hand) that the proton is the hydrogen (of the ice). He says a proton hit makes a cascade, and it is the cascade particles (traveling relativistically) that get detected by the long light paths they create.Amanda detector (Antarctic Muon and Neutrino Detector Array)
Amanda was an early, smaller, prototype of IceCube built into the ice to optically detect 50+ Gev neutrinos. Amanda's Wikipedia page decribes its neutrino detection this way: "The neutrino collides with nuclei of oxygen or hydrogen atoms contained in the surrounding water ice, producing a muon and a hadronic shower. The optical modules detect the Cherenkov radiation from these latter particles..."
At the diagram level the three anti-neutrino can be captured too by a proton-to-neutron conversion (in the oxygen or hydrogen of the ice) with anti-leptons ejected. The IceCube spec doesn't mention anti-neutrino detection. I suspect there would be light flashes, because the anti-leptrons would be moving near the speed of light in a vacuum, but how big, where, or how long the flashes would be probably depends on how long the positron and its high energy cousins live before annihiting.
Icecube strings respond to a neutrino ejected high
energy muon
I got this
image off Bing, but it looks like Icecube strings lighting up (very likely
a simulation) from a cherenkov radiation cone from a high speed muon traveling
upward entering lower right (red is earliest). Icecube looks mostly for
neutrinos coming upward after traveling through earth. Notice some strings
have yellow in center and go greenish in the tails. The bright center bulge
is where the tip of the cone passes and is detected first, and then detectors
up and down the string light up from the outer and dimmer parts of the
cone passing.
Icecube strings light up -- (probably) a muon traveling
upward from lower right
(rainbow colors show time, red is earliest)
Details of IceCube's strings
A grad student
working on IceCube details how its light detecting strings are arrayed
over its 1 km^2 surface area:
"The detector itself is as awesome as the objects it is meant to observe. The baseline design consists of 80 strings with 60 digital optical modules (DOM) each, each DOM contains an extremely sensitive light detector, called photomultiplier tube (PMT), and all the associated electronics to do the data readout each time the PMT “sees” something. The strings are placed in a triangular grid with a 125 m spacing between neighboring strings. The 60 DOMs in each string are 17 m apart and are deployed between 1.5 and 2.5 km below the surface, because of the extreme transparency of the ice at those depths."Found this sketch on the web page of the Nestor neutrino high energy detector of the cherkov light pattern from a high speed muon and a cascade. The cherkov light pattern from a single charged particle has a well defined angle to the travel of the particle (in the range of 40 degress about as shown), and can be calculated from equation angle = arccos (1/n) where n is the refractive index.
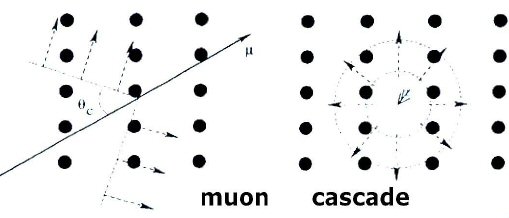
cherenkov light from a muon and a cascade
(source-- http://www.inp.demokritos.gr/nestor/seminar/lkr_presentation.pdf)
Cherenkov radiation in reactor
Here is a beautiful image of blue cherenkov radiation. This is a look at the core of a large 1,000 Mw nuclear reactor in Switzerland built 40 years ago. I saw this blue radiation myself when I was in HS and toured the small nuclear reactor on the MIT campus. On a walkway over the reactor we could look down into the open reactor bathed in water and see that the water was blue.
IceCube goals and specifications
http://aether.lbl.gov/www/projects/neutrino/
Kamland
mineral oil anti-neutrino detector
In 2002 a
large anti-neutrino detector began operation in Japan (1,000 tons of mineral
oil + scintillators) monitored by 1,900 phototubes) able to detect electron
anti-neutrinos from beta decay of fission products in dozens of nuclear
reactors located around the country. (This was before most Japanese reactors
were shut down by the earthquake and tidal wave.) The primary purpose was
theoretical work on anti-neutrinos including their expected oscillation.
Kamland detects anti-neutrinos pretty much the same way that Cowan and Reines did forty years earlier. The target is a hydrogen proton in the hydrogen rich minweal oils. When an electron anti-neutrino hits a quark in the hydrogen nucleus and is absorbed, it converts the proton to a neutron (p => n) emitting a positron. The newly created neutron is no longer chemically bound to the oil molecule, so it drifts off as free neutron.
vebar + p => n + e+
The positron soon meets an electron, and they annihilate emitting two gamma rays. The free neutron soon runs into a hydrogen nucleus and is captured making deuterium, and this capture emits a gamma flash. The scintillators in the mineral oil allow these gamma flashes to be detected by the phototubes. And as Cowan and Reines found, they use the time difference (about 200 usec) between the positron and later deuterium flashes to provide a clear signature of an anti-neutrino detection. Because the incoming anti-neutrino has to provide the energy for both extra neutron mass and for the positron the threshold of the detector is 1.8 Mev. They only get an anti-neutrino hit about once every three days.
Kamland mineral oil targetHalo dectector --- another neutrino detection method
I had no idea what mineral oil was. Turns out it a colorless light oil derived (mostly) from petroleum that chemically is an alkane. An alkane is series of molecules of various lengths made up only of carbon and hydrogen. The carbons are single bonded to each other in an open chain each carbon having two hydrogen. The length of the chains in mineral oil is C15 to C40. Kamland mineral oil had benzene added to it (ratio not given). Benzene is another colorless hydrocarbon (C6H6) where the carbons form a six sided ring. So the Kamland target is basically 1,000 tons of a colorless liquid made up of carbon and hydrogen atoms with a small amount of an unspecified scintillator material added.

Halo neutrino detector under construction,
a grid of lead blocks and He3 filled tubes
(source -- http://www.snolab.ca/halo/albums/construction/index.html)
The neutron target for the neutrino in Halo is in the nucleus of the lead. The transmuted lead atom ejects a neutron. Helium 3 is one of several known 'neutron detectors', because upon absorbing a neutron it transmutes to (charged) tritium and a bare proton as the electrons are stripped away. These two moving charged ions will ionize gas molecules forming current conducting paths, hence they can be detected and counted in a geiger counter.
Lead has a large nucleus with a lot of neutrons, so a big pile of lead provides a large capture area. When a neutron in a lead nucleus absorbs a neutrino and converts to a proton, an atom of the next higher element bismuth is created. The bismuth nucleus is said to be 'excited', likely because it has absorbed the energy of the incoming neutron and the (n => p) conversion releases 1.29 Mev of energy. The bismuth nucleus relaxes by emitting a high energy neutron. The neutrons travels out of the lead into surrounding tubes of He3 that capture them forming (moving) charged tritium and hydrogen ions. The tubes of He3 gas probably also act as 'geiger' counters with an electric field across the gas and electrodes to detect and count current pulses that flow in the ionized paths of the moving charged particles.
Neutrino detector web sitesWhat is the neutrino target?
You would think that going to web sites of a neutrino detectors would be a good way to learn about how they detect neutrinos. Wrong! Most of them do a poor job of describing the physics they are using. The Halo site was particularly bad with a single sentence (plus equation) describing how He3 detects neutrons. (I figured out what they are doing only by going to Wikipedia to read about He3 neutron detectors. I sent an email to Halo suggesting they expand the He3 neutron detection description. No reply.)
"An electron neutrino interacts with a neutron in a nucleus of a water molecule (in the hydrogen??) to produce an electron and a proton." In other words the detection equation is a charged current (n => p) reaction [ve + n => p + e-]. Yikes! (http://t2k-experiment.org/supporting-material-for-t2k-press-release-19-7-2013/)To further confuse how Kamiokande works I found a detailed neutrino site which linked to actual pictures of the 1987a supernova the original Kamiokande detected, and the detection of those supernova neutrinos was described this way:And Wikiedia (neutrino) seems to be on the same page, saying this about Super Kamiokande, "As neutrinos can interact with atomic nuclei to produce charged leptons which emit Cherenkov radiation, this pattern can be used to infer direction, energy, and (sometimes) flavor information about incident neutrinos."
"(It was) probably an anti-neutrino interaction on a proton giving a positron and a neutron (p => n). The neutron goes undetected, but a positron produces a cone of cherenkov light in the water which is detected by some of the 2048 photomultiplier tubes arrayed on the six walls of the detector." So it's an anti-neutrino charged current reaction with a positron (apparently surviving long enough) to give out a detectable cherenkov light cone. (I am beginning to think there are several mechanism in a water detector like this that can produce high energy charged particles, so various types of light cones exist and all to some extent can be detected.)I sent an email to the T2K site asking about the neutrino target.
There's another class of neutrino detectors which are built for an altogether different purpose than telescope neutrino detectors which monitor the sun and deep space. These are part of the particle physics world and are specially built neutrino detectors used to measure the properties of neutrinos created by particle accelerators. There are three of these experiments:
Opera
CERN => new detector in Italy
vu => vtau detection
Nova
Fermilab => new detector
vu => ve detection
T2K
proton accelerator in Japan => Super Kamiokande
vu => ve detection
There's one in the USA associated with the Fermilab accelerator (we make the strongest neutrino 'beams' in the world says its web site) call Nova. And one in Europe associated with the CERN acclerator called Opera. Both accelerators generate muon neutrino beams. The Femilab Nova experiment is looking for a neutrino change to an electron neutrino while CERN's Opera is looking for a neutrino change to a tau neutrino. (My guess is that the former is probably much more likely than the latter since the electron neutrino mass (see below) is roughly x100,000 lower than the muon neutrino mass, while the tau neutrino mass is roughly x100 larger.) Opera's tau detector is expecting a very low event count. I later discovered a 3rd experiment in Japan. A proton accelerator is generating an strong neutrino beam directed toward the super kamiokande detector 200 miles away. T2K is also looking for a change from muon neutrinos to electron neutrinos. In 2013 they reported conclusive evidence (7.5 sigma) of a (vu => ve) transition with 28 recorded events.
Smaller neutrino experimentsWith accelerators there is a pair of neutrino detectors, one near the accelerator to measure the outgoing beam and another one a few hundred miles away. The purpose of the Nova experiment is to examine the so-called 'oscillation' of neutrinos as they travel, which is a quantum mechanical property of neutrinos and depends on the mass difference between neutrino flavors. Neutrino oscillation is considered the expanation of the missing solar neutrinos. Most early solar dectectors were set up to detect electron neutrinos, so if a neutrino having been created as an electron neutrino in the sun was in one of the other (so-called) flavors (muon or tau) as it passed through the detector it would not be counted.
Fermilab has a bunch of other neutrino experiments: Minos (earlier experiment where a muon neutrino detector 500 miles away records a deficits), Long Baseline neutrino experiment (considering putting a neutron beam detector down in the Homestake mine where noise is lower), Microboone (70 ton liquid argon for a special purpose)Minos determines from its muon deficit oscillation data a value of square of mass difference (m^2) = 0.0031 (ev)^2, which is a delta m of {sqrt 0.0031} = .055 ev. (Minos ppt results)
Neutrino oscillationThe Nova pictures show the neutrino detectors are quite large. All I know about them at this point is that they are built in a modular form and each contains photodetectors. I think they use minerial oil (carbon chains of moderate length decorated with hydrogen). Wikipedia says about minerial oil: "Mineral oil is a natural scintillator, so charged particles without sufficient energy to produce Cherenkov light still produce scintillation light. Low energy muons and protons, invisible in water, can be detected."
The simplified description of neutrino 'oscillation' is that is that a neutrino, being a true quantum mechanical entity, can be thought of as a superposition of electron neutrino, muon neutrino, and tau neutrinos. In a Nova Fermilab video a physicist makes an analogy with two tuning forks that start off the same. He then sticks a little mass onto one tyne and when both are struck, a beat note appears. Obviously the beat note is a function of the extra mass, and in sort of the same way the flavor oscillation of neutrinos is a function of the mass difference between the three types of neutrinos (squared I think). A major purpose of these experiments is to determine (or more closely bound) the mass of neutrinos, all of which are only crudely bounded, which is tricky because what they are measuring is a function of neutrino mass differences.What I don't understand (discussions are impenetrable) is how the oscillations of solar neutrinos can experimentally reduce the occurrence of electron neutrinos to 1/3rd to 1/2 when the mass difference between them is so huge. In short how can an electron neutrino turn into a tau neutrino with any appreciable probability when a tau neutrino may be x10 million heavier! I could understand this if the neutrino energy were far in excess of its rest mass, but this does not appear to be the case with solar neutrino none of which has over 18 Mev of energy, with more than 90% < 400 kev.
Wait a minute
Wait a minute, I think jumped to conclusions above: 400 kev is a lot of energy in terms of rest mass. An electron's rest mass energy is 511 kev, and most solar neutrino detectors work in the Mev range. If neutrino rest masses under consideration are a lot less than an electron, which I think they are, then solar neutrinos probably have plenty of excess energy to make a little mass. My guess is that the fraction of the neutrino energy that would be in its rest mass is so small that is has little effect on the the probability of oscillation conversions. (It might even be that the conversion probabilities are dependent on the neutrino total energy and invariant to its rest mass.)Probably what caused me to write the original statement is the high mass limits shown on the Wikipedia neutrino page. The Wikipedia article starts off my saying that neutrino mass is tiny even by the standards of subatomic particles, which probably means much lighter than an electron, the lightest of subatomic particles, but later there is a table with known mass limits and it goes way up: ev limits for electron neutrinos, kev for muon neutrinos and Mev for tau neutrinos.

Fermilab Nova near neutrino detector (222 ton) under
construction
(Far Nova neutrino detector is 28 modules totalling
15,000 tons (!)
making it x67 larger than the near detector.)
(Near and far detectors are built from the same modules)
Neutrino beam
The trick
Fermilab uses to make a (muon) neutrino beam is to first create pions,
which are charged particles. They get steered with magnets to travel in
the direction they want the beam to go. The pions soon decay into muons
and muon neutrinos which continue to travel along the same path. (Why the
neutrinos travel in the direction of their pion source is not explained,
but it probably follows from conservation of momentum.) 400kw of power
goes into the neutrino beam. A new facility was built in northern Minn
about 500 miles from Fermilab to detect the neutrino beam. The energy of
the neutrinos is about 2 Gev and the received flux is "large". The beam,
which starts out at six feet wide at Fermilab, expands to several miles
wide at the receiver distance of 500 miles.
I found details about how particle acclerators make pions and a feynman diagram for how negative pions decay (via W-) into muons and anti-muon neutrinos. (A good bet is that positive pions decay (via W+) into positive muons and muon neutrinos! Yup) Pions are unstable particles made up of two quarks: a quark and an anti-quark. Protons are accelerated to high energy levels (Gev) and hit metal targets generating pions. The charged pions are directed down a tunnel 100 feet long and with half lives of 26 nsec or so they mostly decay to muon and muon neutrinos. At the end of the tunnel a shield absorbs the residual pions and muons, and the muon neutrino continue to the remote detector hundreds of miles away, which since the earth is curved means the beam leaving the accelerator is aimed slightly downward into the earth.
A negatively charged pion is made from a dn quark (-1/3) and an anti-up quark (- 2/3). Here is its decay feynman diagram (time is left to right, ignore the arrow directions which follow strange conventions) generating a muon and (in this case) an anti-muon neutrino. Wikipedia (pion) says 99.988% of pions decay to the muon leptons as shown here, but the residual will be electron leptons, so there will be a small number of electron neutrinos in the beam which is background noise for the experiments looking for oscillation of muon neutrinos to electron neutrinos.
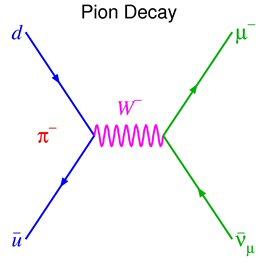
negatively charge pion decays (26 nec or so) to a
muon and muon neutrino
(99.988% of pions decay as shown just to leptons)
time is left to right, dn quark (-1/3), anti-up quark
(-2/3)
Atomospheric muon neutrinos and muons
A muon researcher
in a video says we are all hit by an (atmospheric) muon about once a day.
And in a nobel lectures it was stated we are 'hit' by a neutrino about
once in a lifetime. Muons are created in the upper atmosphere by the decay
of pions (etc) that cosmic rays (mostly high energy protons) create when
they hit nitrogen and/or oxygen. Via time dilation (muons have a half life
in lab of 2.2 usec) many muons are able to make it to the surface of the
earth and even penetrate several km into the ground.
Here's a figure from Wikiedia (cosmic rays) showing a shower created by high energy cosmic rays, which are 99% the nuclei of hydrogen (90%) and helium (9%). Notable are that via short lived pions (two quark particles with lifetime of about 26 nsec) muon neutrinos and muons (of both polarities) are generated. Muon neutrinos are a source of noise to many neutrino experiments, and Wikipedia says some muons not only make to the surface of the earth but a few can penetrate into mines. It is this 'noise' that drives most neutrino experiments deep below the surface into mines or under lots of water or ice.
high energy cosmic ray (proton) hitting upper atmosphere
gas molecule
creates (via short lived two quark pions) muon neutrinos
and muons (both polarity)
(source --- http://en.wikipedia.org/wiki/Cosmic_ray#Primary_cosmic_rays)
Wikipedia (cosmic rays) also says that at sea level 13% of background radiation that people are exposed to comes from cosmic rays, but it doesn't break out the components of this radiation.
How do the Nova detectors work?
Here's the
description of how the Nova detectors work from the Nova web site. This
is typical of how poorly the physics is described on neutrino detector
web sites.
"The detectors are made up of 344,000 cells of extruded, highly reflective plastic PVC filled with liquid scintillator. Each cell in the far detector measures 3.9 cm wide, 6.0 cm deep and 15.5 meters long. When a neutrino strikes an atom in the liquid scintillator, it releases a burst of charged particles. As these particles come to rest in the detector, their energy is collected using wavelength-shifting fibers connected to photo-detectors. Using the pattern of light seen by the photo-detectors, scientists can determine what kind of neutrino caused the interaction and what its energy was." (Well that's sure clear!)This is new project (construction expected to end in Jan 2013) with plans to run six years. For three years the generated beams will be composed of muon neutrino, then they will shift to making muon anti-neutrino beasms. A Fermilab video says they are detecting electron neutrinos.
CERN's
Opera neutrino detector
The best data
I found on any neutrino experiment is a video made by CERN. They have a
six min video (below) with researchers and lab spokesmen describing the
experiment in considerable detail. Like at Fermilab the purpose of the
Opera neutrino experiment is to better understand neutrino oscillation.
The video implies that all other work on neutrino oscillation has been
based on observations that neutrinos are missing. That is certainly
the case with the early solar neutrino detectors that found only 1/3rd
of the neutrinos that solar models predicted.
Youtube video --- CERN First Appearance of Tau Neutrino
The Opera experiment wants to document the appearance of new flavor of neutrino as the neutrino travels the 500 miles or so from the source. To do this they generate a neutrino beam at CERN that is composed entirely of muon neutrinos. The detector in Italy is looking for a tau lepton, which (the video implies) can only be created by a captured tau neutrino. And (get this) the detection of a tau neutrino in the huge detector will be such a rare event that they only expect to see 15 events over the life of the experiment! They have captured to date what they considered one clean tau event, and this is what triggered the release of the video.
The Opera tau neutrino detector is built most ly of lead. There are 200,00 'bricks' each of which is 50 lead sheets with some sort of file emulsion between them. The implication is that the lead nucleus probably spits out a high energy (charged) tau lepton, which probably exits the lead into the film emulsion where it leaves a trail. To identify which brick to examine (apparently manually) between bricks are scintillation detectors (must be optical monitoring). The implication is that the tau lepton has enough energy to get out of the brick and trigger the scintillation detector.
CERN Opera tau neutrino detector built from 200,000
lead layered bricks
(image captured from CERN video)
A figure in a CERN report shows that in atmospheric cascades a muon can split (decay) into an electron and two different types of neutrinos: (muon => muon neutrino + e- + electron neutrino). (I think one of the outgoing neutrinos must be an anti-neutrino to maintain lepton #.)
Daya
Bay reactor anti-neutrino experiment
I added this
2009 Chinese reactor anti-neutrino experiment for two reason. One, they
have the best pictures. And two I read in the newsletter from the LUX wimp
detector people in Berkley that Daya Bay is important because it has demonstrated
the effectiveness of multiple layers of shielding to quiet the background,
which is what the LUX people really need. The are apparently planning to
build on the success of the Daya Bay detector with their planned seven
ton xenon wimp detector. The Daya Bay experiment is measuring parameters
of neutrino oscillation using anti-neutrinos from a large reactor.
"The technology of nested vessels of different kinds of scintillator, surrounded by photomultiplier tubes, was recently put to the test at Daya Bay, which achieved spectacular success in measuring neutrino oscillations even before the experiment’s full array of antineutrino detectors had been deployed." (http://newscenter.lbl.gov/feature-stories/2012/05/23/lux-lz/)

Daya Bay reactor anti-neutrino detector
A new class of detectors have been built to look for a possible new particle, the wimp, a particle that may or may not exist, a particle not in the standard model of physics. The motivation for this search is that this particle may be contributing more mass to the universe than all baryonic matter combined, it might be the mysterious 'dark matter', in other words it might be the stuff most of the universe is made off! And perhaps just as important, finding a new particle not in the standard model would lead to a whole new physics beyond the standard model!
These new wimp detectors share a lot of properties with neutrino detectors. They look for flashes of light (or sounds) and/or electrons knocked free when a wimp occasionally collides with the nucleus of an atom transferring energy and causing it (via the weak force) to recoil, and like neutrino detectors wimp detectors are all located deep underground.
Galactic
rotation curves are flat
Newton's laws
show the speed of an orbiting body is proportional to sqrt{M/r}, where
r is the radius and M is the mass inside the orbit radius 'r'. In the solar
system nearly all the mass is in the sun, so planet speeds fall off as
sqrt{1/r}. Star rotation speeds in spiral galaxies were expected to also
fall off approximately the same way, because it looked from the bright
luminosity of the central bulge that this was where most of the mass of
spiral galaxies was located.
However, in the 1970s when the speed of rotation of many spiral galaxies were measured by Vera Rubin, she found in the typical galaxy it did not roll off as expected, it was flat. Instead of outer stars moving more slowly than inner stars, as planets do in the solar system, they moved as fast as the inner stars as far out as it could be measured. A serious problem, either gravity was wonky or (more likely) mass within galaxies was not aligned with its light output.
Most astronomers, believing gravity is well understood (even on a large scale), take this as evidence that substantial mass must exist inside galaxies that is non-luminous (dark). And a flat rotation speed means this unknown mass density must vary approx as (1/r^2), which mean it pervades the galaxy, all the orbiting stars must be passing through it, and its density grows as the bulge is approached. [Reason the mass density in a simple model varies as r^-2 is that volume of a sphere goes as r^3, so then the mass (M) inside a sphere increase as r holding the orbital speed term (sqrt{M/r) constant.] This unknown mass has come to be known as 'dark matter'. Rubin once said in a video interview I saw that she expected the mystery of dark matter would be solved within 20 years after her flat rotation paper. Nope, we still have only crude guesses, there is no hard evidence as to what dark matter really is.
Dark matter
About 85%
of matter in the universe, i.e. not counting mass-energy from 'dark energy',
is an unknown substance referred to as 'dark matter'. Astronomers have
run a lot of tests over many years to see if dark astronomical objects
like failed stars, dim stars, planets, mini-black holes, etc, might make
up the missing mass, but find that they can only contribute a small fraction
of the missing mass. Neutrinos too have been ruled out as the source of
the missing mass not only because their mass-energy is too low, but also
because they travel too fast (nearly at speed of light). Computer simulations
show that for dark matter to clump to provide the basis for galaxies to
form, it must not be moving too fast, i.e. it must be cold dark
matter.
From astonomical gravity considerations, however, the mean density of dark matter in our region of the galaxy can be been calculated, and the value given in my major wimp reference (with a 10% error band) is below:
dark matter density (mean) = 0.39 Gev/cm^3.
With what is thought to be a pretty tight constraint on the mean density, but loose constraints on the mass, what it means for detector design is this. At the lower end of the mass range as the mass goes down, the flux goes up, but detection gets harder as recoil energies fall off. At the upper end of the mass range as the mass goes up, recoils get stronger, but detection gets harder because the rate of collisions rolls off (as 1/mass).
Unknown particle
So with all known
particles and astonomical objects ruled out, the guessing was (and is)
that dark matter is most likely a totally unknown new particle. If this
new particle is only sensitive to gravity,which it might be, then detection
of the particle directly in a detector is hopeless as gravity effects
on individual particles are unbelievably tiny. Hence particle physicists
are
hoping, though I don't believe there is any hard evidence for
this, that the particle is sensitive to the weak force, and hence can interact
with normal matter something like the way a neutrino does. With this assumption
and some guesses about the mass range (1 GEv to 1,000 Gev, another ref
says 10 Gev to 10,000 Gev) and its speed, they can do some calculations
about frequency and what will happen, and with this information the design
of detectors is possible
Possible
detection strategies
Calculations
for weak force sensitive dark matter candidate indicate that the most likely
interaction of a weak matter sensitive dark matter particle with normal
matter is a bounce (scatter) off the nucleus (of a heavy atom). Heavy atoms
are preferred because the cross-section goes up with mass. Also the target
nucleus should ideally have high mass, a mass in the range of dark matter
particle, because then an elastic 'bounce' off the nucleus will transfer
more energy to it. The reason for this is unlike with neutrino collisions
the speeds here are relatively low (non-relativistic) so the equations
for a classical elastic collision apply. Whereas the first generation of
dark matter detectors used relatively light silicon and germanium, because
detection was easier with a crystal, the latest generation of dark
matter detectors are using (liquid) xenon, the heaviest noble element that
is not radioactive.
A dark matter particle bounce off the nucleus of a heavy atom would transfer to it a relatively small amount of energy (tens of kev, see below), but this is enough to cause a recoil motion of the nucleus that under the right conditions is potentially measurable. For example, the recoil is expected to (partially) ionize the atom, so free electrons are generated, which in an insulating material might be sensed. These electrons are not moving relativistically, because the available energy is far below their 0.511 Mev rest mass, so they do not generate a cherenkov flash and cannot be detected optically. [Not really. I read that a hit to an xenon nucleus causes a scintillation flash that can be picked up optically.] A nucleus recoil of a few mm in a crystal will disrupt the crystal and make a ping sound that can be picked up as well as some local heating. In (liquid) xenon a strong nucleus recoil causes a scintillating flash (as the recoiling nucleus dumps its energy) that can be picked up by phototubes.
Wimp detectors
It took me
a long time to realize that there is another whole new class of related
detectors just coming online: WIMP detectors. These have been built to
look for the most likely theoretical candidate particle of dark matter:
Weakly Interacting Massive Particle. Unlike neutrino detection, which after
fifty years of experimental work is now routine, wimp detectors are cutting
edge experimental physics. They are about where neutrino detection was
sixty years ago. They are at the prototype stage, the largest about 1/10th
the size of the original Kamiokande detector. They are looking for a particle
that may nor may exist, a particle that may or may not interact via the
weak force, a particle that may or may not have the properties that researchers
guess.
To date (Dec 2013) not a single confirmed detection of wimp has occurred, and according to Wikipedia (weakly interacting massive particles) 17 different Wimp detectors have either been built or planned. The Jan 2014 issue of Scientific American has a one page update on first results from the biggest and most sensitive of these new wimp detectors: LUX wimp detector, a tank of 370 kg of liquid xenon a mile underground in South Dakota. They report null results in three months of operation, and the article quotes researchers as saying that wimp experiments have now ruled out about half of the possible wimps that had been predicted.
What 'ruled out' may mean is this. At one point, before experimentors got into the game, there were two supersymmmetic wimp candidates: sneutrino and neutralino. What these are I have no idea (except that they are 'partners' of the neutrino and neutron), but from the reference below null results from early wimp detectors have excluded the sneutrino (at least for dark matter in our galaxy) leaving, in the supersymmmetic world, the neutralino as the prime wimp candidate.Wimps and supersymmetry
In fact no super symmetric particle has ever discovered, and whether they exist or not no one really knows. If wimps are detected, they might very well turn out to be a supersymmetric particle, most likely the lightest stable supersymmetric particle, a neutralino, but this raises a problem. The theory of supersymmetry, once thought to be extremely promising since it solves some difficult theoretical problems in particle physics, has over time been getting more and more hemmed in by experiments, which have failed to detect any possible super symmetric partner particles, so this theory now carries a lot of baggage.
Baggott in his recent book (and other references) say wimps could still exist without supersymmetry. There are other extensions to the standard model which include a wimp like particle. And if a wimp detector can pick up any wimp like candidate, new extensions would undoubtedly soon be crafted.
Difficulty
of wimp detection
The theorists
suggest that what is essentially a very heavy, slow neutrino may exist,
dubbed the Wimp (for Weakly Interacting Massive Particle). This is a particle
that like the neutrino that interacts via the weak force (and gravity),
but not the strong or electromagnetic force. There seems to be two possible
approaches to detection: wimps may hit each other and annihilate outputting
high energy neutrinos. There is also some probability, though apparently
a lot lower than with neutrinos, that wimps may interact with ordinary
matter detectable by light flashes, free electrons, and various other ways
like a sound ping or heat pulse as energy is transferred and a recoil occurs.
Since wimps have not shown up in neutrino detectors (they are never even mentioned), my guess is that one of the reasons is that their (weak) cross section must be smaller than the neutrino crosssection, which is on the order of 10^-43 cm^2. [Perhaps more importantly wimp interactions are not expected to produce relativistic electrons or quark-element changes that many neutrino detectors are designed to detect, nor is it expected to create a cascade of high energy particles, because the transfer of energy from a wimp to ordinary matter is expected to be relatively small.] And sure enough a 2013 technical paper from the LUX wimp detector team reports they find that at a wimp mass of 33 Gev/c^2e the cross-section can be no more than 7.6 x 10^-46 cm^2 (90% confidence). This is about x100 less than neutrino cross-sections, so this probably means wimp interactions with ordinary matter would be much less likely than neutrino interactions.
Wimp mass
A Caltech
set of slides on wimp-nucleus scattering suggests that the wimp is expected
to have a mass in Gev to Tev range (10^9 to 10^12 ev). The Lux wimp detector
team seems to agree as their plot (below) shows pretty much the same mass
range, and I note that its maximum sensitivity (33 Gev) is right in the
geometric center of 1 Gev to 1 Tev.
Protons and neutrons have mass-energy a little below 1 Gev and the largest naturally occuring element (U238) is x238 times heavier, so weight of atoms of the periodic table nicely overlap much of the (guessed at) mass range (1 Gev to 1 Tev) for wimps. This opens the door for a possible detection strategy. A wimp 'bounce' off the appropriate nucleus, one whose weight is fairly near that of the wimp, might be expected to transfer substantial energy to the nuclues causing it to recoil. (The working assumption here being this is a classical (non-relativistic) elastic collision where the maxiumum energy transfer occurs when the two masses are equal.)
Calculating
nucleus recoil energy from wimp 'bounce'
The atomic weight
of a xenon nucleus is 131. A freshman physics calculation of an elastic
collision between two equal masses with one initially still is very simple.
It's like the swinging balls on desktop, the incoming mass stops dead transferring
all
its kinetic energy (and momentum) to the mass it hits. I have no idea if
a classical calulation like this applies to quantum 'scattering' (bounce)
interaction (probably does), but I am going to assume it does and calculate
the recoil energy.
One key assumption the wimp detecting people are making is that wimps are probably moving randomly (and probably not too fast?) relative to the galaxy, but our solar system is rotating at 220 km/sec (2.2 x 10^5 m/sec about 0.1% speed of light) around the galactic center, so 220 km/sec is used in a baseline calculation for the velocity of a wimp-xenon collision. The speed of the earth around the sun is 30 km/sec, so depending on the orientation of the earth's orbit relative to the galaxy plane, there is likely to be an annual variation in any measured winp-matter collision energy. Another assumption for the calculation is that the mass of the xenon nucleus = the mass of the wimp, meaning a (square) hit would cause all (or most) of the wimp energy to be transferred to the nucleus. Most references say a wimp would cause a recoil with ten of kev energy transferred to the nucleus.
Moving slowly relative to the galaxy?Let's do a (classical) calculation for how much kinetic energy a xenon nucleus would acquire with these assumptions. That is it acquires a recoil speed of 220 km/sec, the average earth galactic rotation speed, from a (square) hit of an equal mass wimp [122 Gev = 131 atomic mass x 0.931 Gev] that transfers all its (apparent!) KE to the xenon nucleus:
The reference don't explain what this means, but I suspect it means the assumption is that wimps are moving like stars in a globular cluster. In photographs globular clusters looks like huge spherical balls of stars with no hint of structure, but, of course, those stars cannot just be sitting there motionless, because if that were the case, over time the gravity of the cluster would have long since pulled them all into the center. The explanation the astronomers give is that each individual star has its own individual, elliptical orbit around the cluster's center of mass, i.e. they move separately with orbits like comets in our solar system, going in, looping around the center of mass, and coming back out again.If the wimp orbits (aka 'dark matter' orbits) are elliptical about the center of our galaxy, those we could intercept could either be passing through our radius coming or going from further out, or they could be near their maximum radius slowly turning around. I suspect the statistics favor the latter, and this is what is meant by 'moving slowly relative to the galaxy'. If they are in the outer part of their elliptical orbits, they would not be moving very fast relative to the galaxy, so the closing velocity would then be pretty much our rotational velocity. This appears to be the working assumption of the wimp detection people.
The other way wimps could potentially be moving (relative to the galaxy) is rotating about the center of the galaxy like we are. But if this is the case and the wimps are rotating in the galaxy disk, the intersection velocity will be low if they are rotating in the same direction we are. Wimps moving like this would probably not be detected because the energy transfer, the recoil energy, would be very low. If they are rotating in the opposite direction (probably unlikely), the closing speed would be double, the recoil kinetic energy higher by x4, so if this is the case I would think such wimps would be detected.
E recoil = 1/2 x m v^2
= 1/2 x (131 xenon atomic weight x 1.67 x 10^-27 kg mass of proton)
x (2.2 x 10^5 m/sec)^2
= 109 x 10^-27 x 4.8 x 10^10
= 523 x 10^-17 joules
= 5.23 x 10-15 joules x (1 ev/1.6 x 10^-19 joule)
= 3.3 x 10^4 ev
= 33,000 ev (33 kev)
yes!
Checks nicely, a xenon nucleus I find acquires a recoil energy of tens of kev in an elastic collision where the wimp stops and all its (assumed) speed as seen on earth (i.e. the average speed of the solar system rotation around galactic center) appears to be transferred from the wimp to the nucleus. This agrees nicely with what I see the references saying (without of course explaining it!), and this is the ballpark nucleus recoil energy the wimp detectors are designed to detect.
Really it is the other way aroundMore complicated
Of course, it is really the other way around! What is really happens (from a galaxic viewpoint) is not that the wimp transfers its KE to the nucleus, but the nucleus transfers its KE to the wimp! Looking from outside the galaxy we see a xenon nucleus moving along fast (due to solar system rotation around the galactic center) at 220 km/sec and hitting a stationary (or near stationary) heavy wimp particle elliptically orbiting the mass center of the galaxy, a wimp with mass assumed to be about the same as the xenon nucleus. And this wimp is just sort of just 'sitting there', because it is in the outer reversal part of its elliptical orbit, as the xenon nucleus on the fast moving earth slams into it. In an elastic collision of equal masses the fast moving xenon nucleus transfers its energy and momentum to the wimp which recoils off, so the xenon nucleus just comes to a stop relative to the galaxy, meaning it suddently stops orbiting, while the solar system sails on. But of course in the lab we see the xenon nucleus which has suddenly 'stopped' its orbiting of the galaxy center as suddenly starting to 'recoil' backwards at 220 km/sec!
Liquid xenon wimp detectors
XENON1T
detector (3/21/16 update)
According
to a short article in Feb 2016 Scientific American a very large scale liquid
xenon wimp detector is scheduled to begin a two year run starting in March
2016. XENON1T is located deep underground at the Gran Sasso National Laboratory
in Italy. This detector will be by far the most sensitive xenon wimp detector.
It has a tank of 3,500 kg of liquid xenon compared to the 370 kg of xenon
of Lux in South Dakota, which is still operating, so combined with improved
shielding it will be at least an order of magnitude more sensitive than
Lux. As the pictures show, this is big science, 15 million dollar experiment
with scientists from many countries.
 .
. 
XENON1T wimp detector deep underground in Gran Sasso
mountains Italy
The overview of the Scientific American story is this is may be make or break for current wimp models. Many potential wimp particles have already been ruled out experimentally. If this detector fails to detect anything, then the whole wimp picture of supersymmetric particles with a small crosssection to collisions with normal matter may be fatally flawed.
XENON1T detector
details
XENON1T uses
reliable dual detection similar to LUX. A collision produces an instantaneous
scintillation flash (S1) picked up by phototubes, and a collision with
a xenon nucleus is expected to knock free several electrons. A strong electric
field across the xenon causes the electrons to drift upward at a known
rate (2 mm/usec) to the surface. At the liquid gas interface "a strong
electric field extracts the electrons and generates proportional scintillation
(S2) which is recorded by the same photomultiplier arrays as a delayed
signal." This configuration allows them to localize where in the tank (to
within a few mm) the collision occurs, which they say allows them to dramatically
reduce background interference.
S2 flash'The ratio S2/S1 (light flashes) allows us to discriminate nuclear recoils, which are the expected dark matter interaction, from electronic recoils, which are the dominant background noise.' "And of course, the more energy a particle deposits in the detector, the brighter both S1 and S2 signals are, hence allowing us to reconstruct the particle’s deposited energy as well." 'Together, these two signals provide the energy and position of the interaction as well as the type of the interacting particle.' The predicted XENON1T sensitivity at 50 GeV/C2 is 2.0 x 10^-47 cm2, a 100x lower than the current limit published for XENON100.
"The ionization (free electrons) is then extracted into the gas phase by the stronger electric field in the gaseous phase. The electric field accelerates the electrons to the point that it creates a proportional scintillation signal that is also collected by the photomultiplier tubes, and is referred to as the S2 signal."
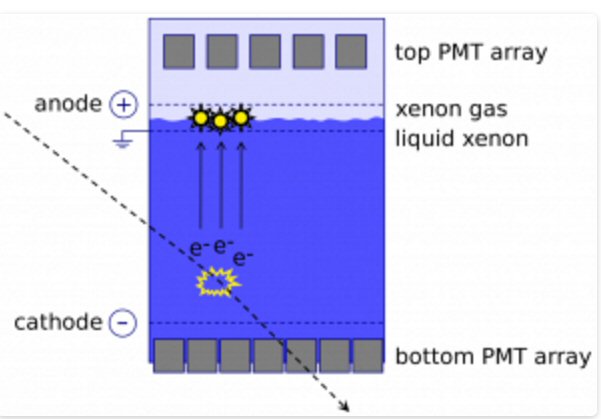 .
. 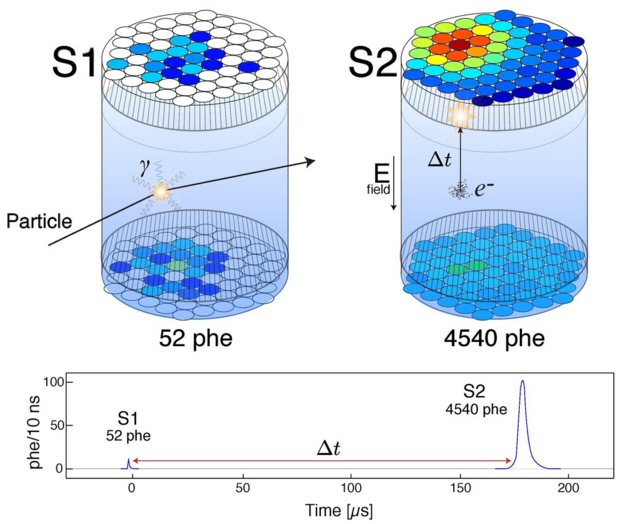
(left) XENON1T cylindrical chamber with phototubes
(PMT) top and bottom
(right) LUX S1/S2 dual flash detection (same as Xenon1T)
LUX xenon wimp
detector
The LUX (Large
Underground Xenon experiment) 370 kg liquid xenon detector has just begun
operation in South Dakota and is the world's most sensitive wimp detector.
It is x3 more sensitive than previous detectors and x20 times more sensitive
than previous detectors for detection of low mass wimps (< 33 Gev).
The latter is important because an earlier generation of silicon cryogenic
wimp detectors had reported three possible wimp hits. The LUX project newsletter
said if those silicon hits had been real, then this larger, more sensitive
detector would be expected to have a hit nearly once an hour. In fact in
its first three months of operation in 2013 it found zero wimps
(of any mass), thus making it appear very likely the earlier low mass wimp
hits were false alarms.
LUX looks for both (ultraviolet) light flashes (with phototubes) and free electrons. Xenon is element 54, the heaviest of the stable noble gases, on the periodic chart between krypton (36) and radon (86). Xenon being a noble element should not absorb free electrons, thus allowing an electric field across the tank to drive them to the top surface and into gas above where they can be detected.
A technical paper on their first three months run in summer 2013 gives their detection strategy: "spin independent Wimp-nucleon elastic scattering". So the wimp-matter interaction is a 'bouncing' of the wimp off the nucleus, and unlike some other wimp detection approaches, this weak interaction is apparently not spin sensitive. The paper goes on to say calculations show a wimp bounce off a nucleus could transfer to it several tens kev of recoil energy. The detection rate is estimated to be less than 1 event/kg/year, so with 370 kg presumable Lux could find up to 1 event per day, yet in their first 85 days of operation they found zero.
While the outside shielding tank is large, the real Lux wimp detector inside is tiny. It is a liquid/gas xenon tank only about 1.5 ft (on a side) sitting inside a 20 ft water tank that provides more shielding, since they are looking for rare events. Being deep underground shields from cosmic ray, the water shields from radiation coming from the rock of the mine. I verified that 370 kg of xenon would fit in this space. The xenon tank is about 1/8 cubic meter, but xenon is heavy and even 1 cubic meter of water weighs an amazing 1,000 kg.
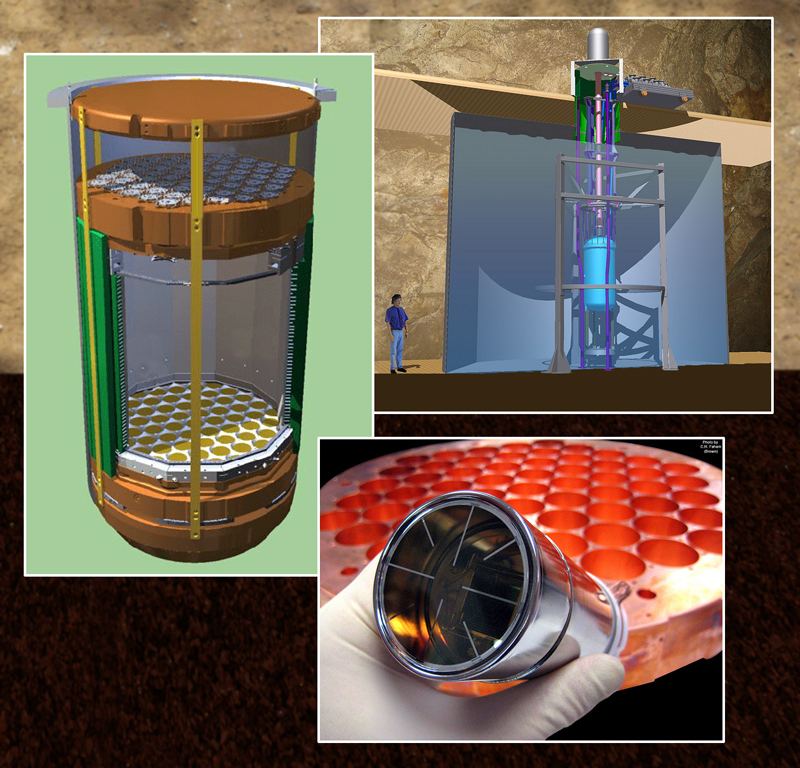
"The LUX detector (above left) is filled with liquid xenon cooled to minus 108 degrees Celsius. Arrays of photomultiplier tubes (lower right) are at top and bottom and catch the faint light when a WIMP interacts with a xenon nucleus. Electrons knocked loose in the collision are pulled by a strong electric field into the xenon gas near the top of the tank and emit a brighter flash; by comparing the flashes and the time between them, the energy, position, and nature of the collision are determined. The xenon container is immersed in a tank of water to provide extra shielding (upper right)."
LUX xenon
wimp detector details
LUX looks for
two
outputs from each event, a pair of light flashes, the 2nd (and
brighter) flash occurring within a time window centered a few hundred usec
after the first flash. This same two output 'trick' was used in the first
neutrino detector of the 1950's as it is very effective at rejecting background
events and privides (hopefully) a unique signature. This is just what you
want when looking for rare events.
The nucleus recoil produces an ultraviolet scintillation in the liquid xenon, which they detect with a few dozen phototubes under the liquid, and the freed (ionization) electrons when driven by electric field up into xenon gas above the liquid produce an electroluminescence (scintillation) that is detected by a few dozen phototubes on the top. I read both liquid xenon and xenon gas are natural scintillators, the recoiling nucleus sheds at least some of its energy with a light flash. The recoil apparently strongly ionizes the xenon as the event triggeres look for at least 8 electrons. A high voltage (6 kv/cm) is applied across the xenon gas, so the strongly accelerated electrons hitting the (neutral) gas molecules must be what causes the electroluminesence flash. The second flash with energy added by the electric field is much stronger than the first. In fact I read the energy of the wimp collision is estimated from how bright the second flash is. This must mean that the number of electrons released (ionized) depends on how hard the recoil hit is. A xenon atom has 51 electrons in three shells and Wikipedia shows it has three levels of ionization. Xenon is widely used in lamps because it ionizes readily.
Here's the result of the first 85 day LUX run in the form of a curve of upper limit of wimp cross-section vs wimp mass. I read that the one or two (possible) events detected by the small silicon and germanium crystal first generation wimp detectors if real, would have produced something like a thousand events in the much larger LUX detector in 85 days. The fact that it recorded no hits in 85 days is thus very significant, and (probably) means the events from the 1st generation wimp detectors were not real, just background events.
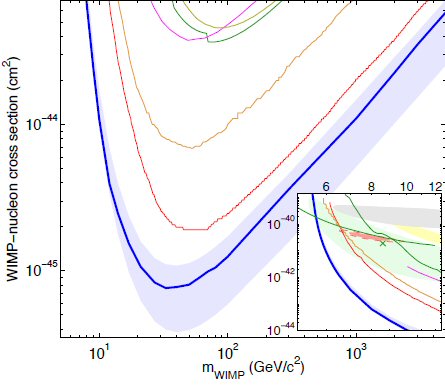
Lux experiment upper limit of wimp cross-section vs
wimp mass (blue, 90% confidence)
upper limit cross-section 7.6 x 10^-46 cm^2 at 33 Gev/c^2
(source --- http://luxdarkmatter.org/papers/LUX_First_Results_2013.pdf)
Jim Baggott in his book 'Farewell to Reality' says the meaning of the lower and lower cross-section limits found in these wimp experiments is that the range of a possible wimp particle is smaller and smaller so it mass is being pushed higher and higher.
LUX ZEPLIN (coming)
The team running
LUX has plans (and funding) to scale up the experiment by a factor of 20.
In other words the present detector is really just a prototype. The existing
1/3rd of a metric ton LUX xenon tank would be replaced by a seven ton xenon
tank, and the external water filter will be replaced by an organic scintilator
(moniotored by new phototubes) to help reject (veto) hits for penetrating
cosmic ray or neutron hits.
Phototubes as a source of radiationXENON100 (older)
Ironically as the filtering gets better and better much of the remaining background radiation (neutrons and electrons) affecting the experiment will come from the phototubes themselves, which (almost by defintion) need to be inside most shielding. The team is working with the phototube manuf to try and reduce their output radiation.
Early wimp detectors
The first
wimp detectors (CDMS or Cryogenic Dark Matter Search) were built starting
20 years ago. These were crystals of silicon or germanium that looked for
a sound ping (phonon) and charge generated by wimp recoil hits to the nucleus.
Improved versions of these detectors have produced a cross-section limit
of 4.6×10^-44 cm^2 (for 60 Gev wimp mass).
------------------------------------------------
ADMX
-- Axion Dark Matter Experiment (update 8/25/15)
The Aug 2015
Scientific American (Einstein edition) has an article on a (modest scale)
Univ of Washington dark matter detection experiment. Their detector is
looking for a hypothetical elementary particle called the axion,
which I never heard of. Wikipedia says it would be expected to have no
charge, no spin, very low mass (10^-6 to 1 ev) and to interact electromagnetically
and gravitaionally.
The experiment is a barrel size, high Q, tunable microwave cavity cooled to 0.1C absolute zero with a strong magnetic field (8 tesla) across it. Theory predicts axions in a magnetic field have a small probability of changing ('decaying') to photons, which in this experiment means a tiny burst of microwaves. This experiment has been operating for a while, but is now being upgraded with a much better, super sensitive, low noise microwave detector composed of an antenna coupled to a Superconducting QUantum Interference Device (SQUID) amplifier. Since the expected frequency of the microwaves depends on the axion mass, which is not known, they cycle the resonance frequency of microwave cavity by moving two tuning rods. Wikipedia says this is the first experiment with a realistic chance of detecting dark matter candidate axions. The principle ADMX investigator says by 2018 they should know if axions are passing through earth or not.
What's interesting (re:winps) is that the axion principal investigator says wimps have for quite a while been the favored candidate for cold dark matter, but one wimp detector after another has come up empty. And he says with the failure (so far) of the large and very sensitive LUX xenon wimp detector to find anything, it's time to to check out if axions exist.
Wimp annihilation
The
wimp annihilation theory goes like this. Wimp mass is thought to have helped
galaxies to form, so presumably the sun and earth as they travel around
the galactic center have been traveling through a mass of wimps for billions
of years. So for billions of years wimps have been bouncing off the protons
and neutrons of matter and losing energy with the result that some probably
should have been captured by the sun's or earth's gravity, then over time
with more energy loss they would sink to the center of the sun or earth.
What?Now in the center of the sun, and maybe the earth too, there could be enough of them that is some reasonable chance of them colliding, and this annihilation would (supposedly) create neutrinos which carry away most of their mass energy. My wimp technical reference says wimp anniliation can include: "neutrinos, gamma rays, positrons, antiprotons, and antinuclei", in other words a zoo of particles with a wide range of mass. (How firm or shaky an analysis like this can be with an unknown particle, I never see comments on, but the wide ranging zoo implies it is not very constrined.) Hence these will be high energy neutrinos in the Gev to Tev range coming from the center of the earth or the sun (above the usual 15 Mev limit due to fusion), so this could be a signature of wimps.
Isn't above pretty hard to swallow? I now think yes, even though it is what you read in Wimp press announcements. As an explanation for wimps in the center of the earth and sun, it is so oversimplified that it doesn't make any sense. Consider our earlier wimp collision analysis. What is 'really' happening here I think is that occasionally slow moving wimp particles get hit by earth atoms and are accelerated to around 220 km/sec in the direction of the earth's rotation about the galactic center, so (for a while) they begin to travel with the earth in its rotation around the galaxy center. This then provides time for earth's and sun's gravity to work on them, and if there is an energy loss mechanism like maybe more collisions over billions of years, they might be expected to sink to the center of the earth and sun (and of course all the planets too).
Smoking gun detection
My wimp technical
reference gives these two possible (indirect) detections as a "smoking
gun" wimp signal:
* GeV neutrinos coming from the center of the sun or earth
* Monoenergetic photons from WIMP annihilation in space (see
below)
Job for Icecube?
I suppose there
are some efforts to look for these neutrinos, but in all my reading about
neutrinos I never saw any mention of this, so this must be either a small
or new effort. A likely detector for this job I would think would be Icecube
which is optimized for detection of high energy neutrinos and can determine
the direction (within 1 degree) from which they come.
Sure enough, when I do a google search I find that in Wikipedia (Icecube neutrino detector) there is mention that Icecube might be able to detect neutrinos coming from wimp annihilation. However, it says "(they) could be observed by IceCube as an excess of neutrinos from the direction of the Sun." Really? From my reading I would think the signature would be an excess of 'high energy' neutrinos (> 30 Mev). (I left a note to this effect in the Talk section of the Wikipedia Icecube page.)
Reference
Good discussion
of wimp detection criteria. It points out that the sensitivity of wimp
detector looking for nucleus recoil is highest when the mass of the wimp
is equal to the mass of the nucleus. This gives the highest energy transfer
when the 'bounce' occurs.
http://pdg.lbl.gov/2011/reviews/rpp2011-rev-dark-matter.pdf
--------------------------------
Dark matter annihilation in galaxy center? (11/27/15)
It's a couple
of years since I wrote above and (suspected) dark matter annihilation is
again in the news. It's the lead story in the science section of the 11/14/15
issue of the Economist. This time the focus is on 'excess' emissions from
our galaxy center, and the emissions in question are not neutrinos, but
high energy gamma rays detected by Fermi, a US gamma ray telescope launched
in 2008.
The proposed theory is that dark matter has concentrated in the galaxy center and (via the 'weak' effect?) dark matter particles collide and annihilate producing as one of the final outputs a high energy gamma ray flash (or flash pair). Six years ago Hooper and Goodenough subtracted known sources of gamma rays and found an excess in the galaxy center and put forward this case for others to knock down. It has survived for six years, but my online searches showed in 2014 everyone was waiting for the Fermi team analysis. What triggered the Economist article was that the Fermi team has just released its analysis, and it agrees the signal is real, there is an excess, and it is not an artifact of the satellite operation.

Galactic center with 'excess' gamma rays shown in
false color
(This is the figure that was in the Economist 11/14/15)
This makes the particles emitting these gamma rays, now informally called 'hooperons', the strongest candidate yet for the detection of dark matter. The Economist talks in terms of the dark matter particle being the Wimp (weakly interacting massive particle), but an online search shows others are proposing the signal could come from the neutralino (see below). Wikipedia (neutralino) says, "As a heavy, stable particle, the lightest neutralino is an excellent candidate to form the universe's cold dark matter." The range of possible neutralino mass is extremely wide: 10 - 10,000 Gev. The only other proposed source for these gamma rays is a collection of millisecond pulsars at the galaxy center, but this is a minority view.
Whoops --- neutralino is a wimpThe Fermi telescope provides three types of information relevant to the dark matter hunt. The energy of the gamma rays (30 to 100 Gev), which is thought to be in the right range for a weak interaction. The intensity of the signal at the galactic center, and its fall off with radius, which also looks reasonable.
I (above) assumed the neutralino was a different particle from the wimp! Wrong. Wimp refers is a general class of particles. The neutralino, a theoretical supersymmetric particle, is a wimp! If a wimp is dark matter, then the working assumption of those in the field appears to be that wimp (class) particle will likely turn out to be the neutralino.
Three potential ways to detect dark matter
There are
three totally different approaches being taken to directly or indirectly
detect dark matter. One is astronomical, a detection of 'excess' gamma
rays or neutrinos from possible dark matter particle annihilations in areas
where dark matter is expected to concentrate. The current focus is on gamma
ray data from the Fermi telescope, but high energy neutrinos detected by
IceCube are still a possibility. I read that IceCube to date has only detected
37 high energy neutrinos so data here is very thin.
Second is an indirect detection of dark matter particles on earth by looking for a particle rebound due to a dark matter particle 'hit', meaning an energy transfer from a dark matter particle to a heavy atom nucleus via the weak effect as the dark matter particle bounces off. There must be dozen or more highly specialized 'rebound' detection experiments running, many quite large scale, most of them cryogenic. The detection methods are all highly specialized, some looking for a 'ping' sound, others for a released charge, still others for a tiny temperature increase. All of these experiments have come up empty so far, but are putting tighter and tighter limits on the mass and energy of the particle, and the experiments continue to be scaled up to increase sensitivity.
The third detection possibility is in the realm of particle physics. It is thought that the large hadron collider, which just began operation again summer 2015 at a higher energy level, a level close to its target level, may be able to directly create dark matter particles. In fact this is to be one of its primary goals. If successful, it would be a game changer, because there is no such particle in the standard model! It might be the very first detected particle of a whole family of supersymmetric particles that have long been speculated to exist.
Relic dark matter particles
Wikipedia
(wimp) briefly outlines the thinking on relic dark matter particles. The
starting point is that dark matter and anti-dark matter particles are assumed
to have been created by the big bang and to be in equilibrium with their
lighter collision products when the universe was very hot. As the universe
cools, the lighter products no longer have the energy to create new dark
matter/anti-dark matter pairs, so now the density of dark matter and anti-dark
matter particles begins to rapidly decline as they continue colliding and
annihilating. This 'stops' when the density gets low enough that they rarely
collide, but a key point of the argument is that it stops first for dark
matter particles with a lower cross section. Any dark matter particles
with a higher cross sections will collide for a longer time and thus end
up at very low densities.
Dark matter annihilation cross-section
With this
model and the current estimated abundance of dark matter in the universe
an upper limit to the cross section for dark matter particles can be derived.
It comes out to be [says Wikipedia (wimp)] no larger than the cross section
for weak interactions (about (10^-46 cm^2). This is (probably) why the
hypothetical Wimp, a weakly interacting, slow moving heavy particle,
is a prime candidate to be the dark matter particle. Having a cross-section
target is essential for design of direct detection experiments. Typically
what they do is design smaller prototype experiments sensitve to 10^-43
cm^2 or better, then after shaking out the bugs (and getting funding!),
plan to scale up the volume of the detector to approach and hopefully to
exceed the very low 10^-46 cm^2 level.
Several dark matter power point presentations have been authored by Jonathan Feng over the last few years. Below are two graphs from a 2013 Feng talk showing [cross-section sensitivity vs Wimp mass] of many direct wimp detecting experiments. Note the detectable mass range is about 10 to 10,000 Gev. 10 Gev is the dividing line between 'light' and 'heavy' wimps. As of 2012 the most sensitive of the experiments had a (best case) cross-section threshold of 10^-45 cm^2 around 33 Gev. Experiments in the future could potentially be 1,000 times more sensitive with a best case threshold of 10^-48 cm^2. To date (nov 2015) none of these experiments has (reliably) detected dark matter.
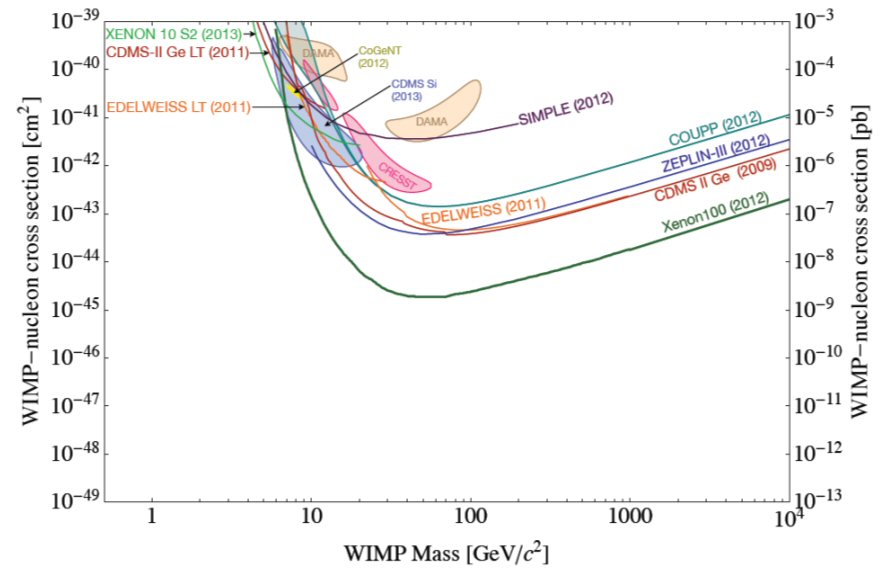 .
. 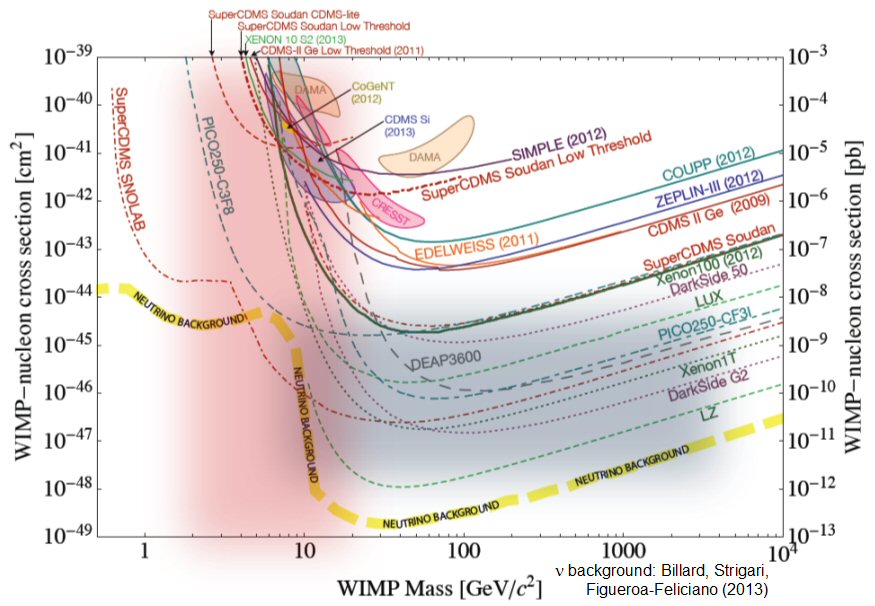
left: cross-section sensitivity vs Wimp mass for dark
matter experiments (2013)
right: cross-sections of planned (larger) experiments
Jonathan Feng 2013 dark matter talk (Cosmic Frontier
Group)
Fermi gamma ray telescope
A check of
the Fermi gamma ray telescope home page shows that this space borne telescope
has impressive specs. It can detect gamma rays with energy ranging from
30 Mev to 300 Gev. It was designed to have high sensitivity above 10 Gev,
because prior to Fermi almost nothing was known about gamma rays in this
energy range. For bright sources the telescope can determine direction
to 1 arc-minute (about 1/30 of the diameter of the full Moon). (This is
what allows it to look at the galaxy center.)
A serious problem in detecting gamma rays (photos) is that the telescope not be affected by a much higher flux of cosmic rays (charged particles: protons, electrons, and their anti-particles). The Fermi home page says the telescope has to reject 100,000 to 1 million cosmic rays for every gamma ray it detects. This is a lot trickier than you might suppose because Fermi is actually measuring the energy of charged particles!
Fermi gamma ray 'telescope' showing how it detects
gamma rays and rejects cosmic rays.
(What is labelled 'conversion foil' is elsewhere called
tungsten foil.)
As a gamma ray photon enters the 'telescope' it passes through the top (anti-coincidence) layer and then hits layers of (high mass) tungsten where it interacts creating a (high energy) electron/positron pair. The direction of the photon is determined by measuring the track of the electron and positron (something similar is done in the IceCube neutrino detector). The energy of the photon is determined by a calorimeter that absorbs and measures the energy of both the electron and positron. The anti-coincidence detector layer of the telescope responds if a charged particle passes through, but not for a photon. This is how cosmic rays are rejected.
Working assumptions about dark matter
According
to Lisa Randall, a Harvard physicist (writing in the New Republic of all
places, Oct 15) "physicists generally take all dark matter to be composed
of a single type of particle".
A major point she makes is that current thinking is that the large amount of dark matter we model must be present is responsible for universe we see today. It greatly sped up the collapse of the tiny density deviations from the big bang, allowing the galaxies we see today to form. In part this is because it doesn't interact with radiation. Normal matter collapsing gets hot and radiates, and radiation she says tends to wash out density differences. (I don't follow this argument. Also if dark matter can't radiate away energy doesn't that mean when it collapses it must get hot and stay hot? Maybe this is why it is thought to form a relatively large, spherical so-called 'halo'.)
She also notes (in passing) that 'dark matter' is a poor name. Dark normally denotes a material that absorbs photons and dark matter doesn't interact with electromagnetic energy at all. A much better name she says would be 'invisible' matter.
-- In a slide talk about dark matter insights an Australian physicists says 'It is not known if dark matter has an non-gravitational interaction with standard model particles or even with itself'! (Obviously the dark matter search is betting that it does both interact with inself (annialiation) and with standard model particles allowing it to be potentially detected. It is further betting that the interaction is via the weak force.)
-- An MIT Haystack observatory talk says, "If the particles that make up dark matter are small, then dark matter is said to be hot. If the particles are large, then it is called cold." (What? Doesn't hot/cold would refer to the velocity of particles not their mass?)
-- Feng 2013 talk, "Dark matter has already been discovered through: galaxy clusters, galaxy rotation curves, weak lensing, strong lensing, hot gas in clusters, bullet cluster, supernovae, CMB (cosmological background radiation).
-- Speculated
(Feng) standard model particles that could result from dark matter annihilation:
positrons and electrons, protons and anti-protons, neutrinos and photons
(gamma rays). Ting's big satellite cosmic ray detector can detect the first
four and is finding a huge excess of positrons over electrons. Nobody knows
what this means. Charged particles are affected by magnetic fields in space,
so Ting's cosmic ray detector provides no directional information.
=============================================================================
The Wikipedia page ('scintillators') says not only charged particles excite scintillators, but (high energy) photons can too. At first I was a little surprised that photons could drive the electrons of a scintillator material to higher orbits, but that just show my ignorance of eletron/photon interactions.
Einstein
discovers light has particle-like properties
By a curious
coincidence while writing this essay I came across in a new book I am reading
the history of how the (light) photon came to be recognized as having particle-like
properties. [see 'Einstein and the Quantum' by a Yale physics professor
Douglas Stone]. It's all due to Einstein, who in 1905 published a paper,
'On a Heuristic Point of View about the Creation and Conversion of Light',
a paper that was the real beginning of the quantum revolution. Stone points
out that no one in nearly a century had thought about light as being a
particle. It was clearly a wave, an electromagnetic wave as Maxwell had
conclusively demonstrated in 1865. But Einstein in his paper showed that
by thinking of light as a train of little quanta of energy separately moving
along in space, certain otherwise mysterious properties of light, like
Stokes rule and recently discovered photoelectric effect, were readily
explainable.
The key feature of quanta, now called photons, that Einstein discovered was that the energy of each quanta depended only on the frequency of the oscillating electron (charge) that emitted the light. Yes, higher intensity (brighter) light carried more energy, but not because the energy of each quantum was higher, but simply because there more quanta (in a given region of space). In Maxwell's (classical) wave theory of light how energy is carried is exactly the other way around. The energy of light is completely independent of its frequency and dependent only on its intensity (square of its electric field). In the 1905 Einstein found an equation, a very simple equation, for quanta energy of light [Equant = h x freq].
I remember being astounded when I first found this equation in my college physics textbooks. Is this true? It looked so odd. Light energy is proportional to frequency? My memory is that my textbook never explained where this equation had come from.Remarkably the constant 'h' in the quantum energy equation, now known as planck's constant, was the very same value that planck had conjured up five years earlier. Planck had found this value of the constant by fitting his radiation formula to the measured curve. Planck found he needed, ad hoc, to restrict the energy level of his resonators (oscillating electrons) in the walls of a blackbody cavity to multiples of this (tiny) value [6.6 x 10^-34 joule-sec] to get the correct blackbody radiation equation.Confusion over a small point
A (possible) hidden constraint of the formula, usually fudged by most references, even in Wikipedia it is not clear, is whether or not 'freq' has to be an integer. Planck's original constraint on the energy of his molecular resonators was that their energy is "not as a continuous, infinitely divisible quantity, but as a discrete quantity composed of an integral number of finite equal parts". If is oscillator energy varies in discrete steps, then in Einstein's formula does 'freq' vary in discrete steps too, presumably in steps of one hz?Not having a quantum physics textbook at hand I will admit I am somewhat unsure about 'freq' quantization. A little work with Wikipedia did not clarify. One of the confusions is that the units of plank's constant ('h') are not energy, but 'joule - sec'. So is multiplying 'h' by 1 hz (one cycle/sec) the smallest possible photon energy value? Who knows, but with real light this quantization issue I suspect is meaningless, tip-toeing in the tulips. Consider green light's frequency is about 6 x 10^14 hz! A search for the smallest quantum of energy found someone asked my 'h x 1 hz' question on a physics forum. One respondent said while properties in quantum mechanics are quantized, the units setting the size of the quantum, like 'freq', are continuous, and a few others answered in a similar vein, so maybe freq is not quantized.
Stone explains how Einstein came upon this idea. Einstein using the new theory of statistical mechanics worked out the entropy equation for molecule of a gas radiating light. Then working backward from what he knew was an accurate approx for short wavelength radiation equation, he derived a similar entropy equation for blackbody radiation. The two equations should match up and were similar, except where the number 'N' of gas molecules should be the radiation version had the expression [E/h x freq], where 'E' is total energy of the the radiation. Einstein immediately drew the conclusion, says Stone, that if light looks like a train of particles ('quanta'), each of energy [h x freq], then [E/h x freq] is the number of those quanta. In other words the short wavelength limit of the blackbody radiation law suggests that light has particulate properties!
Because energy quanta are so tiny and traveling separated in space the odds of two of them coming together at the same time to hit an electron is very improbable (but not zero). The interaction of (normal intensity) light with materials comes down to individual photons interacting with individual electrons. The best an electron can do, Stone notes, is absorb all the energy in one quantum of light, and hence the most energetic quantum it can reemit will have at most the same energy. Einstein in his paper stated his view that energy quanta "can be absorbed or generated only as a whole". So at the molecular level energy is transferred only in quanutm size packets, packets whose energy Einstein found depends only on the frequency (scaled by plank's constant). With this new picture of energy transfer it could be explained how in the photoelectric effect weak blue light could knock electrons free of the surface of a metal while (much) brighter red light could not.
And later experimentors, like Compton, found that many photon-electron interactions could be analyzed simply by applying the laws of conservation of energy and momentum to an elastic collision at relativistic speeds. The difference between a relativistic collision and the classical mechanics elastic collion (solved in freshman physics) is that relativistic definitions of mass and speed are substituted.
Introduction
There sort of a
hierarchy to photon/electron interactions: photoelectric effect: thomson
scattering, compton scattering. In the first case photons are 'absorbed'
and actually knock electrons free of atoms. The second two are called 'scattering'
which is physics code for the fact that the light appear in some sense
to 'bounces off' the electrons. Thomson scattering is a lower energy
interaction and the bounce is 'elastic', so no energy is lost in the collision.
Compton scattering it a higher energy interaction and the bounce is 'inelastic',
so here some energy is lost from the light (transferred to the electron).
At still higher energy (> 2 x 0.511 mev) a photon can break up into an
electron and positron pair.
a)Photoelectric effect --- The photoelectric effect is photons knocking electrons free from the surface of a material, one photon per electron, and it takes only a few ev of photon energy to do this with some materials. The electrons released (into a vacuum) are barely moving and are typically swept up by an externally applied electric field. Wikipedia describes the 'all or nothing' nature of this photon energy absorption:
"Electrons can absorb energy from photons when irradiated, but they usually follow an "all or nothing" principle. All of the energy from one photon must be absorbed and used to liberate one electron from atomic binding, or else the energy is re-emitted. If the photon energy is absorbed, some of the energy liberates the electron from the atom, and the rest contributes to the electron's kinetic energy as a free particle."b) Thomson scattering --- Thomson scattering is an elastic collision and includes how light (few ev for visible light) reflects off material surfaces. In classical terms the E field of the light causes an electron to oscillate at the same frequency of the light (alone the plane of the E field). An important caveat here is that this scattering mode applies to free electrons where the electrons motions are not restricted by quantum wave resonances. Oscillating free electrons emit light, so the energy absorbed by the electron is immediately sent out with the outgoing light. The designation elastic implies that no energy is lost in the collision. When the constraint is applied that the outgoing light is at the same frequency as the incoming light and the energy of photons depends only on frequency, this must mean that at low energy levels there is no energy transfer from the photon to the electron. Wikipedia ('thomson scattering') puts it this way:
The particle kinetic energy and photon frequency are the same before and after the scattering. The energy threshold for this type of reaction is (much) less than [0.511 Mev], the rest mass energy of the electron.How visible light is reflected off materials
A table I have below shows how a particle-like compton analysis shows that for light frequencies down near visible light the transfer of photon energy to the electron is neglible (micro ev). This is why material surfaces reflect light so well with the outgoing light at the same (or virtually the same) frequency as the incoming light. The picture being the E field of the light gives the electron a little shake, but virtually all of this energy is returned in the outgoing photon. (And I think physicists model this light reflection as being instantaneous, no delay, which must either fall out of the quantum physics, or else is an approximation.)
c) Compton scattering --- This is a 'bounce' at higher energy levels and here the collision is often said to be inelastic, however, it is elastic if the KE of the recoiling electron is taken into account. It's also sometimes said that this scattering mode applies to electrons in atoms, but really it makes little difference if the electrons are free or in atoms, because the energy transfer is usually at levels far above the ionization level of atoms so electrons in atoms are knocked free often at relativistic speeds. Scattering' implies light comes in and goes out, which is correct, the recoiling electron (immediately) emitting an outgoing photon. An inelastic collision classically implies some energy is lost, which is true here in the sense that the recoiling electron if traveling in material will over time dissipates its fraction of the incoming photon energy as heat and light.
Compton scattering
Elastic collision between a photon 'particle' and
a stationary electron
What is characteristic
of this collision is that an energy and momentum transfer occurs between
an incoming photon and an electron with the recoiling electron always
emmitting an outgoing photon to balance the energy and momentum equations.
In the classic compton analysis the electron is assumed to be at
rest, which is obviously not true for an electron in an atom, but at the
higher end of the compton range this is a very good approximation, because
the incoming photon has far more energy than (outer) electrons in atomic
orbits. The orbit speed of the electron in hydrogen is a little less than
1% the speed of light, while the electron recoil speed can be 80% the speed
of light and higher.
HistoryCompton scattering when incoming photon has mass energy of an electron
In the 1920's when x-rays were bounced off materials, it was found the outgoing x-rays had lower frequency (hence lower energy) than the incoming x-rays. Compton was able to explain this energy transfer between the photon and electron by using the same sort of 'photon as particle' model that Einstein had used to explain the photoelectric effect.Hyperphysics compton diagram
The picture (below) from the wonderful Hyperphysics site of compton scattering shows an incoming photon 'hitting' an electron. The electron absorbs some of the energy and recoils while simultaneously emitting a lower frequency photon that carries off the balance of the energy. Momentum is conserved too. The sum of the recoiling electron and and emitted photon momentum vectors equaling the incoming photon momentum vector.

Compton formula is at the bottom of the figure.
[Added wavelength = (h/m0c) (1 - cos(theta))]
It shows the output photon wavelength is the input photon
wavelength that has been stretched by an added term.
'E' in electron momemtum equation is TOTAL energy of electron
(source -- http://hyperphysics.phy-astr.gsu.edu/hbase/quantum/imgqua/compton2.gif)KE (relativistic kinetic energy)Note Hyperphysics in the figure above writes the relativistic expression for the momentum of the recoiling electron in what I initially found to be a rather confusing way, but I now see is the best way to write it. This is way to obtain the electron's momentum from its energy. 'E' above turns out to be the total energy of the recoiling electron, which is the root sum square of 'pc = mvc' and the rest mass energy. Turns out that the classical formula for momentum (p = mv) applies relativistically too if 'm' is the relativistic mass. When this is combined with the Einstein rest mass equation (E = mc^2), we have the two simple relativistic equations below for energy and momentum.
While writing this essay for a while (not having a textbook) I got off the beam on the KE. For particles there is a relativistic momentum energy term 'mvc' and somewhere I saw it called KE, and I began to use the equation [KE = mvc]. It's an easy mistake to make. The total energy of an electron equals the root sum square of 'mvc' and the rest mass energy, i.e. [Etot = sqrt{(mvc)^2 + (m0c^2)^2}], but Etot also equals the simple sum of KE + rest mass energy, i.e. [Etot = KE + m0c^2]. My equations with 'mvc' were right, and 'mvc' is an energy term, but it is not KE. There is (apparently) no simple experession for KE other than [KE = mc^2 - m0c^2].Key idea of KEIt is certainly true that total energy (mc^2) is equal to the 'root sum square' of 'mvc' and rest mass energy (m0c^2), and the final equation (below) shows how relativistic momentum is related total energy and rest mass energy.
The key idea is that the (conceptual) definition of KE is the same at lower speeds and at relativistic speeds. It is the energy input (work) required to accelerate a mass from zero to a particular speed, even a relativistic speed, and which if not disturbed it retains this as 'energy of motion'. Hence the total energy of a moving particle is its rest mass energy, which it has at zero speed, plus the work-energy done on it to accelerated it to speed.E = sqrt{(mvc)^2 + (rest mass energy)^2}
= sqrt{(mvc)^2 + (m0c^2)^2}
E^2 = (mvc)^2 + (m0c^2)^2
(mvc)^2 = E^2 - (m0c^2)^2
mvc = sqrt{E^2 - (m0c^2)^2}
p = (mvc)/c = (1/c) x sqrt{E^2 - (m0c^2)^2} (agrees with 'p' formula in figure above)Applied to compton collision
This means for compton scattering the energy split between the output photon and electron is intuitive. If the incoming photon brings in 511 kev and the outgoing photon takes away (say) 1/3rd of it (170 kev), the electron must have acquired a KE of the difference (341 kev). The recoiling electron's total energy is [341 kev (KE) + 511 kev (electron rest mass energy) = 852 kev]. It is the 852 kev total energy (mc^2) that must be used to find the speed of the recoil, which means finding the speed for a mass increase of (852 kev/511 kev) = 1.667, and the answer is v = 0.80c. Check: [1/sqrt{1 - (0.80c/c)^2} = 1/sqrt{0.36} = 1/0.6 = 1.667]E = mc^2 (E is total energy = KE + m0c^2 (rest mass energy))
p = mv (momentum 'as energy' trick: pc = mvc, pvc is not KE)
where
m = m0/sqrt{1 - (v/c)^2}
m0 = electron rest massFor reference --- In the case of a moving electron its total energy is scaled up by 1.41 when it is traveling at .707c. Check using the usual 'special relativity' factor [1/sqrt{1 - (v/c)^2}], which for v = 0.707c is [1/sqrt{1 - 0.5} = 1.41].
If I understand the compton equation correctly, for a given energy photon there is a range of solutions, because the angle of the outgoing photon (relative to the path of the incoming photon) can vary. The compton formula gives the added wavelength vs this angle. (I suspect there is a way to calculate the probability of the various solutions, but I have seen nothing on this.) Three angles are easy to visualize: 0, 90, 180 degrees. However, 0 is not very interesting, because the outgoing photon has the same energy as the incoming photon and is on the same path, so for all practical purposes no collision occurred, no energy is transferred to the electron. At 90 degrees the multiplier is one, so the constant in the equation (wavelength of 511 kev photon) is added to the outgoing photon wavelength, and at 180 degrees the multiplier is two.
This means for an incoming 511 kev photon, if the outgoing photon comes out at 90 degrees, it carries half the energy (x2 wavelength) of the incoming photon, and if it comes out at 180 degrees, meaning it retraces the path of the incoming photon, it carries one third of the energy (x3 wavelength) of the incoming photon.
Since there is no energy lost (or at least that is the assumption) in these high energy (compton) photon-electron collision, it is tempting to say that the kinetic energy of the recoiling electron must be the difference in energy between the incoming and outgoing photons. Common sense, right? At one point I thought the answer was no, but it is yes, but there's a trap here. This is not a classical collision in mechanics, it's a relativistic collision, and it involves a photon. The trap is that unlike classical mechanics, you can't directly calculate the KE. The conservation of energy and momentum apply, but the energy balance involves total energy, so at high speeds the (electron) rest mass is included, and I find (never having done these calculations before) that it makes the recoil of the electron non-intuitive.
Relativistic energy and momentum equationsPhotons have no rest mass energy, all their energy is (in essence) KE and of course their v = c.
Conservation of energy here means the conservation of total energy. And for particles like the electron this means its rest mass energy must be considered. The electron's total energy is the (simple) sum of its KE (kinetic energy) and its rest mass energy (m0). [E total = KE + (m0c^2)] KE is intuitive in that its the energy brought in by the photon minus what the outgoing photon carries away. Inclusion of the rest mass in the energy calculation leads to results that are different from a classical calculation and hence non-intuitive. It is the total energy of the electron that must be used to find it recoil velocity (via scaling up of its mass). The conservation of momentum is a vector calculation, pretty much the same as in a classical calculation, except the the momentum vector lengths are set by the relativistic equations, in the case of the electron controlled by its equation for 'mvc' and in the case of the photon proportional to it energy (as shown below). (The simpliest way to set up the momemtum balance equation is in terms of energy using the 'trick' that (momentum x c) = energy.)
For the photon
E = h x freq = hc/wavelength
(debroglie wavelength = h/p = h/mc = hc/mc^2 = hc/E)
p = mc
(photon effective mass can be found from Einstein's mass-energy, E = mc^2)
= (E/c^2)c = E/c
For the electron
E = mc^2
(E = sqrt{(mvc)^2 + (m0c^2)^2})
p = mv
(pc = mvc)
where
m = mo/sqrt{1 - (v/c)^2}
m0 = electron rest mass
The energy
equation can be reworked to allow KE to be found from the electron's (total)
energy
E = KE + m0c^2
KE = E (total) - m0c^2
The calculation proceeds as follows: Equate the total energy before and after the collision, then subtracting off the energy of the outgoing photon gives the total energy of the recoiling electron. The length of momentum vector of the recoiling electron can be found by appropriately subtracting off its rest mass energy from its total energy to get the pvc. In general [momentum x c = energy], so the momentum vectors are just proportional to energy, total energy for the photon, but for the recoiling electron the momentum energy term is not KE, but [pc = mvc = sqrt{E^2 - (moc^2)^2}]. Vector addition of the two output momentum vectors will equal the momentum vector of the incoming photon, so this gives the angle of the recoiling electron. The total energy of the recoiling electron allows its speed to be found. Relativistically [E total = mc^2], so electron's total energy relative to its rest mass is equal to the ratio of the relativistic mass to rest mass, and this is only a function of speed.Neutrino equations
Most of the above equations apply to neutrinos too. The neutrino is a particle with a tiny mass and a speed just a hair less than 'c'. (Supernova 1987 neutrinos arrived 3 hr before the light after traveling 163 thousand light years.) This means the relativistic equations for the electron (above) apply to the neutrino too including the equation right connecting energy and momentum. Neutrinos at first glance don't (appear to have) a simple relationship between energy and frequency like light photons, but there is an equivalent. The debroglie equation (above) assigns a particle a wavelength that varys inversely with it energy.Because the speed of neutrinos is so close to light, the electron equation can be simplified. As a baseline assume a one Mev neutrino, a rest mass of 1 ev, which from E = mc^ makes the relativistic mass increase one million. Assign [v = c - dv] where dv is delta speed, one millionth of 'c'
p = mv (p = mc within one part in a million)
E = sqrt{(mvc)^2 + (m0c^2)^2} => E = sqrt{(mvc)^2 + (m0c^2)^2}
= sqrt{(mvc)^2}
= mvc = pc (E = mc^2 within one part in a million)m = mo/sqrt{1 - (v/c)^2} = mo/sqrt{1 - (1 - dv/c)^2} = mo/sqrt{1 - (1 - 2dv/c)}
= mo/sqrt{2dv/c)}so if (m/m0) is a million, then (dv/c) must be on the order of one trillionth.
My compton
recoil sketches
90 degree case
Here is the
energy balance equation, where [E0 is the energy of the incoming photon
= 511 kev = electron rest mass]. For the case of 90 degrees the added wavelength
is equal to the incoming wavelength, so the outgoing wavelength is doubled.
This results in the output photon having energy of E0/2 with the recoiling
electron taking the balance 3/2 E0, which is [E0 (rest mass energy)
+ (1/2) x E0 (KE)]
E0 (photon in) + E0 (electron rest mass) = E0/2 (photon out with x2 wavelength) + 3/2 E0 (recoil electron total energy)
The 'pc' energy term of the recoiling electron can be found from it total energy. This is root sum square relationship, so [pc squared = E total squared - rest mass energy squared].
pc = sqrt{E^2 - (m0c^2)^2} = sqrt{(3/2 E0)^2 - (E0)^2} = E0 x sqrt{(3/2)^2 - 1}= E0 x sqrt{1.25} = 1.118 E0
my pencil sketch of compton electron recoil for Ein
= electron rest mass (511 kev),
outgoing photon at 90 degrees and electron recoil
v = 0.745c
Energy: 511 kev + 511 kev = 255.5 kev + 767 kev
Assume photon comes in from left, then with output photon angle at 90 degree (down) the electron recoil is up and right. Summing the vectors the angle of the electron recoil = arcsin (0.5/1.118) = 26.56 degrees. As a check 1.118 x cos(26.56 degrees) = 1, so the sum of the output momentum vectors = photon input momentum vector.
Finding the recoil speed means finding the speed where the relativistic mass is 150% the normal mass. Notice the electron having a total energy of 150% m0 (767 kev) means the collision increased its total energy 50% or E0/2 = 255.5 ev. This makes intuitive sense because the energy brought in by the (absorbed) photon went half to the output photon and half to the (recoiling) electron as KE. To find the recoil speed we need to solve [1/sqrt{1- (v/c)^2} = 1.5] and the solution is v = .745c [check: 1/sqrt{1- (.745)^2} = 1/sqrt{0.445} = 1/0.666 = 1.5].
180 degree case
For a 180
degree recoil of a photon it returns along the same path as the incoming
photon. The compton equation for 180 degrees says to add twice the
wavelength to the outgoing photon, so for the same E0 (511 kev) input photon
of the angle 90 case here the output photon wavelength is x3 longer so
its energy is 0.333 E0, and this makes the electron total energy 1.667
E0. A mass increase of 1.667 means the recoil velocity has increased to
.80c.
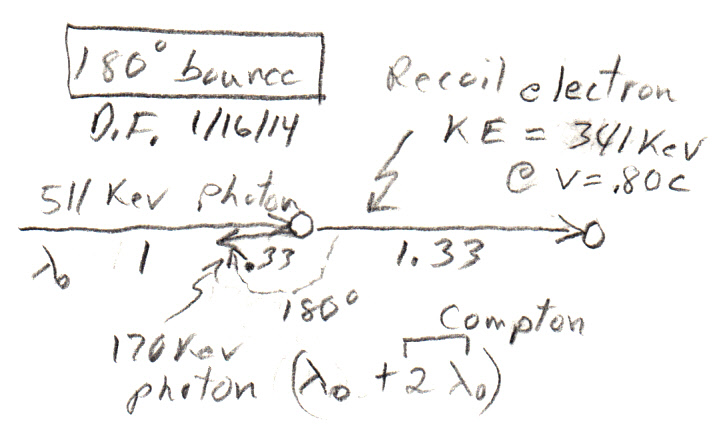
my pencil sketch of compton electron recoil for Ein
= electron rest mass (511 kev),
outgoing photon at 180 degrees and and electron recoil
v = 0.80c
Energy: 511 kev + 511 kev = 170 kev + 852 kev
The momentum vector diagram in the 180 degree case is simple, all the vectors are aligned along the path of the incoming photon. With the recoil photon momentum pointing 0.33 left, then the electron recoil momentum has to be 1.33 right to sum to the 1.000 right, the momentum of input photon. To find the electron's 'pc' energy term the rest mass energy needs to be subtracted off in a 'root sum square' sense from its total energy, sqrt{(1.667)^2 - 1} = sqrt{2.779 - 1} = sqrt{1.779} = 1.33. (pc = mvc = 1.667m0 x .80c = 1.33 m0c.) Yes, it checks.
Compon sets up the equations
Wikipedia points
out that the success of Compton's (particle) scattering analysis of 1923
in explaining his x-ray data was an important confirmation of earlier work,
such as Einstein's photoelectric analysis, that light sometimes shows a
particle like nature. Compton assumed a photon 'particle' hit a non-moving
electron and that the energy split with some going to a recoil motion of
the electron and the balance to a new photon which the recoiling electron
emits. To find the final values he just applied the laws of conservation
of momentum and energy. The analysis is not much different from how a freshman
calculates the final values of a collision in mechanics, except that here
the relativistic expressions for energy and momentum must be used, since
the electron's recoil speed gets up near the speed of light when energy
of the incoming photon approaches its rest mass energy (511 kev):
photon energy E = hf where h is planck's constantThe result of the analysis for a photon with incoming energy nominally the same as the rest mass of an electron (511 kev) is the plot below vs angle (between incoming and outgoing photons). In a classical collision of equal masses, one not moving, 100% of the momentum and energy would be passed from the incoming to the outgoing particle. The result here is not too different, at the optimum angle 67% of the energy is passed from the photon to the electron. Since compton scatter always has an outgoing photon, all of the incoming photon energy cannot be delivered to the electron.
equate to Einstein's mass-energy hf = mc^2
solve for 'equivalent' photon mass m = hf/c^2
photon momentum (p) must be p = mc = hf/c
How photon Ein splits between photon out and electron
recoil vs angle of reflection of the photon out.
At 90 degrees the energy divides equally, half to
the electron and half to the outgoing photon.
In the 180 degree case, where the photon out retraces
the photon in path,
the photon out wavelength is tripled, so it has 1/3rd
of the photon in energy
with 2/3rd going to the electron's recoil KE.
(source --- http://en.wikipedia.org/wiki/Compton_scattering
)
Compton wavelength
The compton
formula makes a rather curious prediction (confirmed by experiment). It
predicts the maximum increase in the wavelength of the outgoing light is
independent
of the wavelength of the incoming light (energy) and depends only
on angle. The angle being the deflection angle of the output photon, meaning
the angle of the outgoing photon relative to the path of the incoming photon.
The formula has a constant scaled by [1 - cos (theta)], whose value is
0 at 0 degrees, 1 at 90 degrees and 2 (max) at 180 degrees. The constant
in the equation, now known as the compton wavelength, has the value [h/m0c],
where m0 is the rest mass of the electron. And interestingly the [compton
wavelength/2 pi = hbar/m0c] is one of the possible 'radii' of an electron.
(see my essay
Electron)
Hyperphysics works out the value of the compton wavelength as 0.00243 nm
or (2.43 x 10^-12 m). The compton wavelength of an electron = wavelength
of a 511 kev photon, a photon with the same mass-energy as a stationary
electron.
Is a complete transfer of energy from a photon to an
electron even possible?
Here's a little
proof I ginned up showing that a photon must come out in any photon-electron
collision, i.e. there is no set of conditions where the photon can pass
100% of its momentum
and energy to the electron. Let's write the
momentum balance equation setting the electron relativistic momentum equal
to the photon momentum. (Def: Ei = energy of incoming photon, Etotal
= total energy of recoiling electron, m0 = rest mass of electron)
p electron = p photon
sqrt{(Etotal)^2 - (moc^2)^2}/c = Ei/c = (Eic/c^2) = (Ei/c^2)c = mc
(Etotal)^2 - (moc^2)^2 = (Ei)^2
[Etotal = m0c^2 + KE]
[(m0c^2)^2 + 2 m0c^2 x KE] + (KE)^2 - (moc^2)^2 = (Ei)^2
2 m0c^2 x KE + (KE)^2 = (Ei)^2
(KE not equal to Ei)
We find that if the momentums balance, KE of the recoiling electron cannot be equal to Ei of the photon. Thus unlike the classical collision it is impossible to transfer all the photon's momentum and energy to an electron, but when an emitted photon is allowed Compton showed there is range of solution where both energy and momentum are conserved, and not surprisingly experiments confirm that this is the way mother nature works.
The reason why a 100% momentum and energy transfer is possible in a classical collision of equal masses is that the same equation applies to both particles. If the output particle and input particle have the same speed and same mass, they also have the same momentum [mv] and kinetic energy [0.5 mv^2].
How is the compton wavelength(s) related to the electron
debroglie wavelength?
In the iconic
case where the energy of the input photon equals the rest mass energy of
the electron I expected that I would find some relationship between the
compton wavelength and the debroglie wavelength of the recoiling electron,
and/or maybe some insight into the size of the electron vs its velocity.
Also the compton wavelength, the constant in the compton equation, divided
by 2pi defines a possible radius for an electron. This allows a time to
be defined: time for an electron to move its diameter at the calculated
recoil velocity. Isn't there some relationship among these terms?
Debroglie wavelength
The classic
debroglie wavelength of a particle = h/p. Momentum (p) of the electron
is 'mv', where 'm' is the relativistic mass of the electron, and 'v' its
recoil velocity. So right away we see the debroglie wavelength is not fixed,
it depends on the speed of a matter particle.
An interesting question is how is the debroglie wavelength of the recoiling electron related to the wavelength of the incoming photon when the energy of the photon equals the rest mass energy of the electron (511 kev)? And this wavelength (see below) = h/m0c = (2.43 pm) is the constant in the compton formula, also known as the compton wavelength.
For the photon
Ei (511 kev) = hc/wavelength = m0c^2 (electron rest mass)
h/wavelength = m0c
wavelength = h/m0c = hc/m0c^2
(compton wavelength)
= hc/(Ei = 511 kev)
For the electron
debroglie wavelength = h/p = h/mv = hc/mvc
(mvc = momentum energy term)
Clearly the
debroglie wavelength will equal the photon input wavelength at the speed
where [mvc = m0c^2], and this speed is 0.707c.
Check
m @ .707c = m0/sqrt{1 - (.707c/c)^2}
= m0/sqrt{0.5}
= 1.41 m0
mvc @ .707c = 1.41m0 x .707c x c
= m0c^2
check
One interesting relationship found
** Found one interesting
relationship --- At a recoil speed of .707c the electron (debroglie)
wavelength = wavelength of an (incoming) 511 kev photon, and this wavelength
is (surprise!) the compton wavelength.
KE is the excess of the electron energy over its rest mass energy, which in this case is [1.41 x 511 kev - 511 kev = 212 kev]. 212 kev is a little less energy and a slightly slower recoil than the 90 degree angle case. From the Wikipedia curve above we can see that at about 80 degrees the KE of the electron is 212 kev, so there is a compton angle of photon emission which makes the [electron recoil wavelength = incoming photon wavelength], but it's kind of an odd angle.Restating the compton formula
The compton formula says that the outgoing photon wavelength is the incoming photon wavelength plus an additive wavelength that varies from zero to twice a wavelength related to the mass of an electron (lamda0 = hc/m0c^2 = hc/511 kev). Landa0 is the wavelength of a photon whose energy is equal to the rest mass energy of an electron (511 kev).The reason the scaling factor tops out at two can also be explained. Conservation of energy and momentum shows that the maximum fraction of a photon's energy that can be absorbed by the KE of an electron is 2/3rd. This requires that the output photon wavelength be x3 times the input wavelength to carry off the 1/3rd of the input energy, and this in turn requires that the compton formula show that the wavelength to be added to the input wavelength be x2 the wavelength of the 511 kev input photon.
Overview of photon-electron collision
The results
of a photon-electron collision look a little odd because what is conserved
is total energy and relativistically this includes the rest mass
of the electron on both sides of the equation. However, it is true that
once the compton formula gives the extra wavelength (for the outgoing photon)
the energy of the outgoing photon is easily figured and subtracting its
energy off from the photon input energy gives the electron recoil KE. Thinking
of the electron's energy increase in terms of its rest mass energy gives
the relativistic multiplier for its mass, and from this the recoil speed
can be found. Working the momentum vectors gives the angle of the recoil.
Comparison to classic elastic collision
When the incoming
photon has the same energy as the electron, a (compton) photon 'bounce'
with electron recoil comes pretty close to the classic case. In the classic
case the incoming mass comes to a stop transferring all the (kinetic) energy
and momentum to the outgoing mass. In the case where the photon bounce
angle is 180 degrees, meaning the outgoing photon returns on the same path
as the incoming photon, here's what my calculations show:
What happens to the energy brought in by an incoming 511 kev photon when it hits (and is absorbed by) an stationary electron?Electron recoil energy for a wide range of photon energyAns:
The amount of the photon energy transferred to the electron depends on the angle (relative to the incoming photon path) of the photon that the recoiling electron throws off. (To date I have seen nothing on what determines this angle. Does it depend (to use a simple picture) on how 'squarely' the photon hits the electron, or is it random?). Anyway the compton formula shows that the fraction of the energy transferred to the electron varies from 0 to a maximum when the angle is 180 degrees. This fits with common sense in that the best 'hit' to the electron is when the emitted photon comes directly back (on the same path) and the electron recoils out on the same path.When the energy of the incoming photon is equal to the mass energy of the electron, 1/3rd of the incoming energy is carried away by the outgoing photon bouncing back (at 180 degrees), and the remaining 2/3rd goes into accelerating the electron to 80% speed of light.
For higher energy photons where the stretching of the rebound wavelength is much more extreme the maximum is still at 180 degrees but the transfer of energy is greater. For example, for a photon with x5 higher energy (2.5 Mev), its output photon wavelength at 180 degrees would be stretched about x10 times the incoming photon wavelength, so about 90% of the photon energy would transfer to the electron.
Check:
An electron traveling at .80c has an increased relativistic mass (E = mc^2) increased by a ratio: [1/sqrt{1- (v/c)^2} = 1/sqrt{1- (.8)^2}= 1/sqrt{1- .64} = 1.666. Since the unmoving electron's total energy was initially just its rest mass energy (1) and post collision, when moving at .8c, it has total energy of 1.666, it must be that the collision imparted an additional energy of (1.666 - 1) = .666. In other words 1/3rd of the energy of the (absorbed) incoming photon departs with a newly created out going photon, and the remaining 2/3rd is transferred to the electron sufficient to accelerate it to 80% the speed of light (hard recoil).
wavelength ratio
photon (incoming)
compton to photon
electron recoil energy
-------------------------------------------------------
-----------------------
-------------------------
5 ev
near ultra violet
240 nm
10^-5
5 x 10-5 ev
50 ev
24 nm
10^-4
5 x 10-3 ev
500 ev
x-ray
2.4 nm
10^-3
0.5 ev
5 kev
x-ray
0.24 nm
10^-2
50 ev
50 kev
x-ray
0.024 nm
10^-1
5 kev
500 kev E = electron rest mass
2.4 x 10^-3 nm
1
250 kev
5 Mev
gamma ray
2.4 x 10^-4 nm
10
4.5 Mev
In the case where the ratio is one (500 kev) the wavelength of the outgoing photon is double the incoming, so the light has lost half of its energy. In the case where the frequency is x10 higher (5 Mev in gamma range) the wavelength of the outgoing light is approx ten times longer wavelength than the incoming photon, so about 90 % of the photon energy has been transferred to the electron.What's apparent from the above numbers is that the light energy loss, which is the energy available to cause an electron recoil, goes up at lower energies as thesquare of the frequency. At visible light frequencies a photon bounce transfers negligible energy to the electron, something like one part in a million of the incoming photon energy, which is only a few ev, so the electron gets just a micro-tickle in the range of 10^-6 ev transfer. This negligible transfer of energy to the electron at lower frequencies shows how compton scattering shades into thomson scattering. Wikipedia puts it this way:
'Thompson scattering is just the low-energy limit of compton scattering: the particle kinetic energy and photon frequency are the same before and after the scattering.' (Wikipedia ('thomson scattering')It's only when the light energy level gets in the range of a few kev does the energy transfer to the electron get in the range of ionization energy of atoms. For a strong beta- type kick to the electron the light energy level has to approach (or exceed) the rest mass energy of the electron (0.511 Mev). Only at these energy levels does a bounce transfer a substantial fraction of the incoming photon energy to the electron. At still higher energy levels (few Mev) most of the input photon energy is now transferred to the electron causing a hard recoil at speeds close to the speed of light.
Compton
recoil and scintillators
So my guess is that
some of the scintillators (visibly) flash when hit with gamma photons due
to the compton effect. In other words a single gamma photon can hit an
electron in a target (or dopant) so hard that it transfers a substantial
fraction of its energy to the electron causing it to recoil out of the
atom with relativistic (or near relativistic) speed. This ejected electron
would look a lot like a beta- ejected electron and have substantial ability
to travel (several mm in metal). So this high speed charged particle would
then, as is usual in scintillators, transfer tiny fractions of its energy
to a lot of electrons in the scintillator material resulting in
a bright flash.
Compton's
1923 x-ray scattering paper
Compton's
original paper on scattering of x-rays by electrons is online (here).
He points out that experimentally a key observation that thomson scatter
theory could not explain was that the frequency of the reflected x-rays
were lower than the incident x-rays and the frequency varies with the reflection
angle. Here is the sketch in Compton's 1923 paper. (I did find one reference
that said clearly that Compton assumed a stationary electron, which is
what the equation imply.)
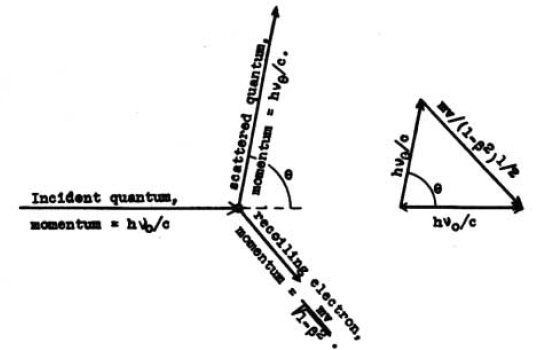
Compton's original sketch
(capture from facsimile of Compton's 1923 paper)
In his paper says "it is perhaps surprising that the (wavelength) increase should be the same for all (incident) wavelengths". He responds that it agrees with experiment, that he has experimental measured the wavelength difference (at 90 degrees) for x-rays whose wavelength varied over a factor of 28 and found it constant in "very satisfactory agreement" with the theory. His incident x-rays (K rays from molybdenum) have a wavelength of .708A and his theory predicts (at 90 degrees) the scattered wavelength (scattered by carbon) should be .024A longer (.732A). He measures the scattered x-rays at .730A, a lengthening of .022A, which is about 90% of the predicted value.
He mentions that the electron recoil speed for x-rays reflected back should be about 80% of the speed of light (correct). In his x-ray experiments he does not see the recoiling electrons arguing they are swamped by another effect that liberates electrons. However, he says that what he calls 'secondary beta-rays' seen in gamma ray (?) experiments are very likely the recoiling electrons. About direction of the reflected x-rays he says only that it is well known that moving objects radiate more strongly in the direction of their motion, and he finds in general agreement with that that the radiation in the forward direction, i.e. in the direction of the electron recoil, is the strongest.
It's interesting that by 1923 the quantum/relativistic formulas for momentum and energy of photons and particles were well developed, identical to their present form. Compton's sketch from 90 years ago of a photon-electron collision is the same one used today. According to Wikipedia the idea that light had momentum had been proposed by Einstein not that many year earlier, and Compton's experiment was confirmation that light photons can act like particles with the predicted momentum. The only (very minor) difference I noticed in his paper is that Compton had written his formula in a less intuitive way, involving sin^2 and a doubled angle, but in fact it is identical to today's compton scattering equation if a simple trigonometric identity is applied to convert the sin^2 term to [1 - cos (theta)].
Inverse compton scattering
In the compton
effect the electron is assumed to be stationary. When hit by a photon,
it absorbs some of the photon's energy and recoils. The balance of the
energy goes into an outgoing photon, which therefore has a lower frequency
(longer wavelength) than the incoming photon. For a given angle of scattering
the compton formula shows wavelength difference between incoming and outgoing
photons is fixed. [As Wikipedia 'Compton Scattering' points out
this wavelength difference for a 180 degree recoil is twice the 'compton
wavelength' of the electron = (2.43 x 10^-12m).] Clearly as the frequency
of the radiation goes down and the wavelength lengthens, the incoming and
outgoing photons have almost exactly the same energy. The interaction is
now characterized as 'thompson scattering', which Wikipedia ('thompson
scattering') points out is the "low-energy limit of Compton scattering."
High speed electron collides with photon
There is a variation
of compton scattering called the 'inverse compton' scattering, which is
important in astronomy. This is also a collision of an electron and a photon,
but in this case the electron is moving fast, really fast, very
close to the speed of light, such that the electron's KE far exceeds the
photon's energy and the electron's 0.511 Mev rest mass energy. In astronomy
electrons participating in this reaction can have energies of 5 Gev, which
means the electron has a relativistic mass increase of 10^4 [i.e. Lorentz
factor = 1/sqrt{1 - (v/c)^2} = 10,000]. What happens in this collision
is that the energy transfer goes the other way from the compton effect.
Here the electron transfers energy to the (outgoing) photon as it slows
down a bit.
I thought maybe I could easily write the equation for a 180 degree recoil, but in the few minutes I spent I didn't get far. The derivations I find online don't do this relativistic collision directly. What they typically do is shift the reference frame twice. A shift to the (relativistic) moving electron's frame allows the compton result to be written down, then the frame is shifted back to the laboratory frame, each frame shift bringing in a scaling term.
A 15 minute video showing the calculation is here: https://www.youtube.com/watch?v=2KFVxPENcEw&feature=youtu.be]. This video calculation shows that in compton/thompson scattering when the incoming photon is a 'light', meaning it has much less energy than the electron's rest mass energy (0.5 Mev), then very little energy is lost to the (heavy) electron, so the energy of the outgoing photon is about the same as the incoming photon, Note denominator of the equation below is approx = 1 when [E photon-in < (me x c^2)]:
E photon-out = E photon-in/[1 + (E photon-in/me x c^2)(1 - cos (phi))]
where
me x c^2 = electron rest mass energy (0.511 Mev)
The inverse compton calculation includes the result above, but the double frame shifts introduce an energy scaling factor = [lorentz factor)^2], so now the energy of the outgoing photon can be much higher than the incoming photon. An example in one reference shows 1 Ghz (4 x 10^-6 ev) radio photons being scaled up in energy by about (10^8) by the inverse compton collisions by 5 Gev electrons (lorentz factor = 10^4) to x-ray photons (about 10^17 hz or 0.4 kev). In an accreting black hole inverse compton scattering scales up the energy of the thermal photons of the accretion disk to produce (some of) the black hole's x-ray spectra. As this example shows near a black hole the relativistic inverse compton collision energy transfer from the (high energy) electron to a (low energy) photon is really, really tiny, a 5 Gev electron passing only 0.4 kev of its energy to a photon. This is a loss in KE of the electron of only about 10^-7 of its energy.
In classical mechanics something (roughly) similar occurs. When a heavy moving object hits a much lighter stationary object, the moving object loses only a tiny fraction of its energy to the light object. The reason for this is that the ability of the light object to absorb energy is strictly limited, because the speed it can acquire from the collision is strictly limited. Classically the outgoing speed of object hit is limited to be no more than twice the speed of the incoming object, thus the energy transfer goes as [m(2V)^2/MV^2]. If say the mass of the incoming object (M) is a million times the mass of the object hit (m), the incoming object has only passed to the object hit 4 x 10^-6 of its energy. One millionth due to the mass ratio, and the factor of 4 from the double speed that the small object has acquired.==========================================================================================
Introduction
Surprisingly in
none of my neutrino references, including Wikipedia, did I find any details
about how a neutrino scatters an electron. Since electron scatter by neutrinos
is the reaction used by big water cherenkov detectors (like Super Kamiokande
in Japan) to detect solar neutrino, I wanted to understand it, and include
it in this essay. I wondered how much of a neutrino's energy and momentum
could be transferred to an electron in an elastic collision (a 'square'
hit). It had to be at least a reasonable fraction, since 5 - 15 Mev level
solar neutrinos cause 0.5 Mev rest mass electrons to recoil at relativistic
speeds.
I early came across the formula for electron recoil when it is hit (elastically) by a high energy photon. It seemed like this might shed some light on neutrino scatter of electrons, so I worked out a bunch of cases and put them in the appendix. One reason analysis of a photon-electron impact is easy to find it that it has a specific name, 'Compton scattering', and Compton was awarded the Nobel prize for physics in 1927 for experimentally confirming it. However, his analysis is in terms of photon wavelengths, so it looked pretty photon specific.
Transfer formula
My first break came
when I found a technical
paper by a Dutch prof of Nuclear Engineering that did the neutrino-electron
collision calculation in detail starting with the wavefunction. I am not
a physicist and this was deeper mathematically than I wanted to go, but
the paper included a simple result (below) that shows the maximum
fraction of the neutrino's energy that can be transferred to the
electron, and it was pretty simple.
E electron recoil = [2(Ev1/m0c^2)/(1 + 2(Ev1/m0c^2)] x Ev1
(p140)
where
Ev1 = energy of incoming neutrino
m0c^2 = rest mass energy of electron
I worked out the numbers for a few cases, and it sure looked like the energy transfer vs (energy ratio of the incoming 'particle' to the electron's rest mass energy = (Ev/m0c^2)) was the same for neutrinos bouncing off electrons as for photons bouncing off electrons! Who knew, this was something of a surprise. The table and calculations (above) for electron recoil vs incoming photon energy applies to incoming neutrinos too. However, this was hinted at by another paper I found on relativistic collisions. This latter paper set up conservation of momentum and energy equations written in relativistic energy terms. Their result was pretty complicated, but they were able to extract the compton equation as a special case, and they noted their general result applied both to particles with and without mass.
The Dutch paper's detailed result was full of reflection angles for the neutrino and electron, but found the maximum energy transfer from the neutrino to the electron occurred when the electron recoils along the path of the incoming neutrino and the outgoing neutrinos shoot backward along the same path. A result very like the photon bounce case (at 180 degree). For detection, of course, an electron recoil along the path of the neutrino is a very nice results, measure the electron recoil path and you have found the path of the incoming neutrino. In practice this provides the neutrino path in a detector like Super Kamiokande to an accuracy of about 1 degree.
Let's look at this equation, starting with neutrino energy of 511 kev equal to electron rest mass energy [(Ev/m0) =1], then transfer ratio is [2(1)/(1 + 2(1)] = 2/3. The result is up to 2/3rd of the neutrino energy can be transferred to the electron, the same ratio for a 511 kev photon hitting an electron (see plot above from Wikipedia). For a 5 Mev neutrino about 95% of its energy (or 4.76 Mev) is transferred to the electron. This is the same result as the photon too. The table (above) shows 4.5 Mev, but this is for a 90 degree deflection, when the maximum transfer is figured (180 degrees), the wavelength of the outgoing photon is doubled, so its energy is halved (500 kev => 250 kev), making the energy transferred to the electron 4.75 Mev. (Table numbers are rounded, so match is not exact)
Ratio of 10: 5 Mev neutrino [2(10)/(1 + 2(10)] = 20/21 = 95.2% of 5 Mev = 4.76 Mev electron recoil
In the case of solar neutrinos (5 to 15 Mev) detected in a water cherenkov detector via scattering the equation shows a square hit transfers nearly all the neutrino energy (95+%) to the electron recoil, so the outgoing neutrino is quite feeble.
Scatter cross-sectionsDeriving equation for neutrino electron elastic collision
This same Dutch paper confirmed that the electron neutrinos are x6 more likely than muon neutrinos or tau neutrinos to scatter off electrons, the confirmation being in terms of the detailed scatter cross-sections for various neutrino types at solar energy levels. Curiously the scatter cross-section for anti-neutrinos is only about 40% that of neutrinos.
Once the equations for energy and momentum conservation are set up correctly, which is in terms of energy, the results falls out in a few lines, as several terms, including square terms, cancel.
One of my false starts was I set up the momentum equations as one normally does classically expecting the 'bounce back' neutrino momentum term would come out negative. I got a result that was mathematically correct, but not interesting, 100% of the energy and momentum had transferred from the incoming neutrino to the outgoing neutrino, which proceeded forward along the same path. In other words no collision.Here's the derivation:I realized the source of my problem. The momentum vectors are all written in terms of energy and the energies can't be negative. To get the bounce back neutrino case I needed to insert a negative sign in the momentum balance equation. When I did that, I ended up with the correct transfer equation, consistent with the Dutch paper.
Conservation of momentum (along path
of incoming neutrino) (p = E/c for neutrino) (see Hyperphysics compton
scatter figure (above) for equation for 'p electron recoil' in terms of
electron total energy)
p incoming neutrino = - p outgoing neutrino + p electron recoil
Ev1/c = -Ev2/c + (1/c) x sqrt{(E tot electron)^2 -
(m0c^2)^2}
where
KE = (Ev1 - Ev2)
E tot electron = KE + moc^2
(recoil kinetic energy + rest mass energy)
= (Ev1 - Ev2) + m0c^2
= (Ev1 + m0c^2) - Ev2
Solve for Ev2 as a function of knowns Ev1 and moc^2
Ev1/c = -Ev2/c + (1/c) x sqrt{(E tot electron)^2 -
(m0c^2)^2}
(Ev1 + Ev2) = sqrt{(E tot electron)^2 - (m0c^2)^2}
(Ev1 + Ev2)^2 = (E tot electron)^2 - (m0c^2)^2
Ev1^2 + 2 Ev1Ev2 + Ev2^2 = ((Ev1 + m0c^2) - Ev2)^2
- (m0c^2)^2
= (Ev1 + m0c^2)^2 - 2(Ev1 + m0c^2)Ev2 + Ev2^2 - (m0c^2)^2
Ev1^2 + 2 Ev1Ev2 = (Ev1 + m0c^2)^2 - 2(Ev1 + m0c^2)Ev2
- (m0c^2)^2
= Ev1^2 + 2Ev1m0c^2 + (m0c^2)^2 - 2Ev1Ev2 - 2m0c^2Ev2 - (m0c^2)^2
= Ev1^2 + 2Ev1m0c^2
- 2Ev1Ev2 - 2m0c^2Ev2
2 Ev1Ev2 = 2Ev1m0c^2 - 2Ev1Ev2 - 2m0c^2Ev2
Ev1Ev2 = Ev1m0c^2 - Ev1Ev2 - m0c^2Ev2
2Ev1Ev2 = Ev1m0c^2 - m0c^2Ev2
2Ev1Ev2 + m0c^2Ev2 = Ev1m0c^2
Ev2 (2Ev1 + m0c^2) = Ev1m0c^2
Ev2 = [m0c^2/(2Ev1 + m0c^2)] x Ev1
fraction of incoming neutrino energy
carried off by outgoing neutrino
Electron recoil (KE) energy is the remaining fraction of the incoming energy. The term in the brackets is the fraction of the energy of the incoming neutrino that has been transferred to the electron.
E electron recoil = [1 - m0c^2/(2Ev1 + m0c^2)] x Ev1
= [2Ev1/(2Ev1 + m0c^2)] x Ev1
= [2(Ev1/ m0c^2)/(1 + 2(Ev1/m0c^2)] x Ev1 same
as Dutch paper
Putting in some numbers to get the overall shape of the energy transfer. Assume m0c^2 (electron rest mass energy) = 0.5 Mev (round numbers). For the case of a 5 Mev (solar) neutrino we find 95% of the neutrino energy is transferred to the recoiling electron! The remain 5% is carried off by the outgoing neutrino that retraces the path of the incoming neutrino. Nowhere have I even seen it mentioned how effective the energy transfer is when a solar neutrino bounces (squarely) off an electron in a scatter reaction (>95%). For the electron to acquire 4.76 Mev of KE, which makes its total energy 5.26 Mev. The ratio of total energy to rest mass energy is reflected in the increase in relativistic mass, and from this the recoil speed can be calculated (standard relativity multiplier). For a .5 Mev electron to have a total energy of 5.26 Mev its relativistic mass must be increased by x10.52, which calculates to a recoil speed of .9955 c
Ev1 (Ev1/
m0c^2) [2(Ev1/ m0c^2)/(1
+ 2(Ev1/m0c^2)]
Etot electron
Recoil speed
-------
----------------- -----------------------------------------
-----------------------
----------------
500 ev
.001
.002 = .002/(1 + .002)
5 kev
.01
.020 = .02/(1 + .02)
.020 x 5 kev + 500 kev
.020 c
50 kev
.1
.166 = .2/(1 + .2)
.166 x 50 kev + 500 kev
.180 c
equal
0.5 Mev
1
.666 = 2/(1 + 2)
.666 x 500 kev + 500 kev
.800 c
solar
5 Mev
10
.952 = 20/(1 + 20)
.952 x 5 Mev + .5 Mev
.9955 c
50 Mev
100
.995 = 200/(1 + 200)
.995 x 50 Mev + .5 Mev
.99995 c
A plot of the fraction of the input particle energy transferred during a relativistic collision has an interesting shape (see below). For light, low energy incoming particles (Ev1 << .5 Mev) the fraction of incoming energy transferred rises linearly with energy (slope of +45 degrees on a log/log plot). For a heavy, high energy particles (Ev1 >> 0.5 Mev) nearly all (> 99%) the incoming energy is transferred, the recoil speed becoming extremely close to speed of light in a vacuum to produce the required large pump up in relativistic mass. At the energy mid point [Ev1 = 0.5 Mev] the fraction of input energy transferred is .666. For input neutrinos or photons with energy above 0.5 Mev rest mass energy of the electron a square hit transfers most of the energy to the electron. Obviously very important for neutrino and photon 'bounce' interactions.
Comparison
of relativistic and classical elastic collisions
I find it
very interesting to compare the table above for a high energy, speed of
light input elastic collision to the table below for classical elastic
collision. I searched everywhere for a plot of this comparision. It must
be in some text books (or maybe a homework problem), but couldn't find
it. I have plotted it up. (I could have looked up the velocity equations,
but wanted to confirm I could still do a (simple) freshman mechanics problem
with two equations, two unknowns!) Here's the result of the classical case
(details below):
(m1/m2) (v1/v0) = [(m1/m2) - 1)]/[(1 + (m1/m2)] 1 - (v1/v0)^2My sketch of relativistic elastic collision energy transfer vs mass ratio
------------ ---------------------------------------------- --------------------
.001 - .998 = (0.001 - 1)/(1 + .001) .004
.01 - .980 = (0.01 - 1)/(1 + .01) .04
.1 - .818 = (.1 - 1)/(1 + .1) .33
equal mass 1 0 = (1 - 1)/(1 + 1) 1.00 100%
10 .818 = (10 - 1)/(1 + 10) .33
100 .980 = (100 - 1)/(1 + 100) .04
The unusual shape of the blue curve, with energy transfers from neutrinos approaching 100% when their energy is above the rest mass energy of a (static) electron (0.511 Mev), is what makes 'bounce' neutrino detection possible!
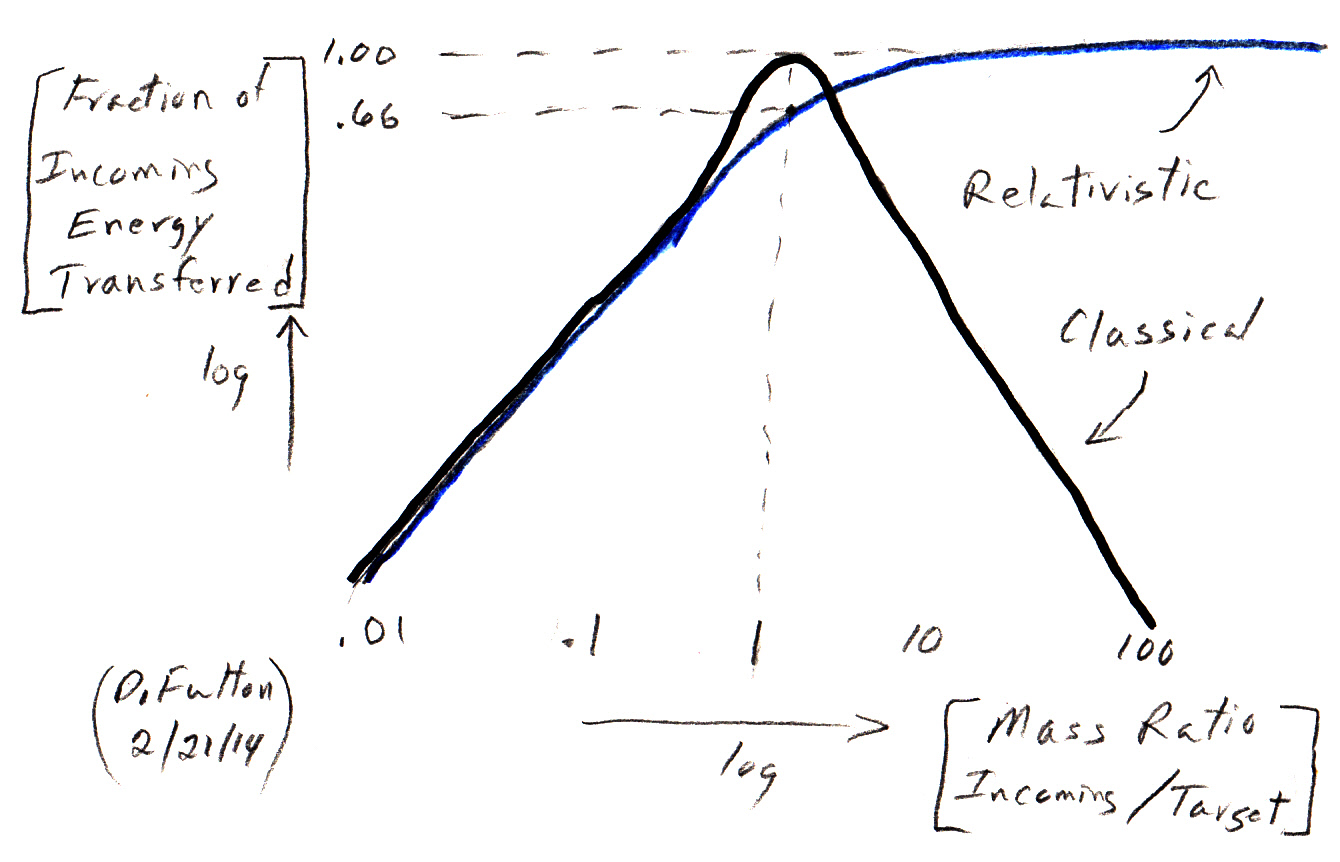
My sketch of relativistic and classical elastic collision
[Input Energy Transfer Ratio] vs [Mass Ratio (Incoming/Target)]
Relativistic curve (blue) applies to neutrino 'bounce'
(scatter) reactions
and photons (compton scattering).
(On a log/log plot the assymptotic slopes here are
+/- 45 degrees)
Notes on the sketch above
I have never
see a sketch like above anywhere, not in any of the neutrino references,
not even from searches of relativistic dynamics. Why? Certainly this physics
is well known to specialists like reactor designer, particle physicists.
(Hell, the photon version, Compton scattering, was derived 90 years ago
by Compton to explain how his x-rays bounced off electrons in matter.)
When I do find a relativistic elastic collision result, it always seems
to be from someone solving a specific problem. No one ever gives the big
picture, or compares the result with the classical case.
Also there is more than one way to plot the data. For example, above I am sketching the fraction of the incoming energy that gets transferred to the target during the collision, which I think is most interesting. This shows (left side) the fraction transferred varies linearly with mass ratio, i.e. when the input neutrino (or photon) has say energy of 1% of the target rest mass energy, it transfers only about 1% of its energy to the target in a collision. However, this same data could also be sketched to show absolute energy the target receives vs mass ratio. Here for low mass inputs the energy transfer would rise as the square of the mass ratio, because in our previous example at a mass ratio of 1/100 only 1% of the low energy of the incoming particle (neutrino or photon) gets transferred, so the target's increase in absolute energy would be about 1% x 1% = 0.01% of its rest mass energy. For high mass inputs (at same input speed) the recoil energy flattens out, the fall off in transfer efficiency compensated for exactly by the increase in incoming KE. Since max recoil speed is x2 the input speed, recoil energy max is x4 the energy transfer for equal masses.
Why
is energy transfer so efficient for electrons hit by high energy neutrinos?
Why is the energy
transfer so efficient for mismatched, relativistic elastic collisions where
the energy of the speed of light inputs (neutrino or photon) is much higher
than the rest mass energy of the target (electron)? (See right side
of the curve above.) I have seen nothing written on this point, but I have
a (hand waving) theory.
Short answer: The energy transfer is so efficient because the mass of a recoiling electron can increase relativistically to (nearly) match the mass of the incoming particle, this allowing it to absorb nearly all of the energy of the incoming particle.
Consider the elastic collision of two balls the same size, but different mass. A heavy, incoming ball hitting (squarely) a stationary light ball. (This is a staple of freshman physics homework problems). The classical equations show the recoil speed of the ball being hit can be no higher than twice the speed of the incoming ball [v = 2 x (m1/m2) /[1 + (m1/m2)] x v0]. Now consider what happens as the mass of the target drops relative to the input. With 'v' having an upper limit the kinetic energy [KE = (1/2) m v^2] of the recoiling target drops as its mass drops. This is shown on the right side of my sketch above where (black) the transfer of energy falls off as the target gets lighter relative to the incoming mass. Classically a light weight target can only absorb a small fraction of the kinetic energy of a heavy incoming mass.Classical elastic collisionNow consider the case of a high energy neutrino (or photon) (squarely) 'bouncing' off an electron, which is the reaction used in water cherenkov neutrino detectors. Here the electron recoiling at nearly the speed of light can its grow its mass relativistically without limit to the point where it almost matches the mass-energy of the incoming particle. As the target electron accepts energy from the incoming particle it mass grows by the relativity factor [m = m0 /sqrt{1 - (v/c)^2}]. So just as in the classical case where nearly all the energy (and momentum) is transferred when the masses are almost equal, the same thing happens here!
However, while the energy transfer can be 95% (or 98%) it is never 100% because the momentum and energy equations show the target mass can never quite grow to equal the incoming mass. This (small) differential is why these are 'bounce' reactions. To balance everything up a low energy neutrino (or photon) has to recoil backwards (see my neutrino and photon bounce momentum sketches).
100^ energy transfer desk top toyClassical equations
The 100% energy transfer for equal masses is the basis of a popular desk top toy. The toy typically has two to five equal mass balls hanging by strings from a frame. When an end ball is pulled up and dropped, it completely (and surprisingly) stops when it hits the stationary ball(s). The stationary ball at the other end upon acquiring nearly all the energy of the incoming ball, then swings out with about the same speed as the incoming ball. Of course the surprising nature of the toy's action is enhanced when it has more than two balls, the middle balls passing along the energy without any significant movement. (I guess they are just passing along the input impulse (force x time), but I haven't seen an analysis of this.)
v0 pre-collision velocity of m1 known (m2 stationary pre-collision)Table above shows (1st col) the fraction of its incoming speed the mass (m1) retains post collision (v1/v0), so the fraction of the incoming KE that m1 retains post collision must go as the square of its velocity ratio (v1/v2). Hence [1 - (v1/v0)^2] (3rd col above) must be the fraction of the incoming KE of m1 transferred to m2.
v1 post-collision velocity of m1 unknown
v2 post-collision velocity of m2 unknownv1 = [(m1/m2) - 1)]/[(1 + (m1/m2)] x v0 v2 = 2(m1/m2) /[1 + (m1/m2)] x v0
Details of a 5 Mev neutrino-electron bounce
Amazingly
I can't find any reference that show the momentum vector diagram for a
neutrino-electron elastic collision, so I have sketched up the case where
the neutrino energy is 5 Mev. This is neutrino energy x10 the electron
rest mass energy and is typical of a solar neutrino bounce off of an electron,
which is the neutrino detection reaction used by the large water cherenkov
neutrino detectors like Kamiokande (in Japan).
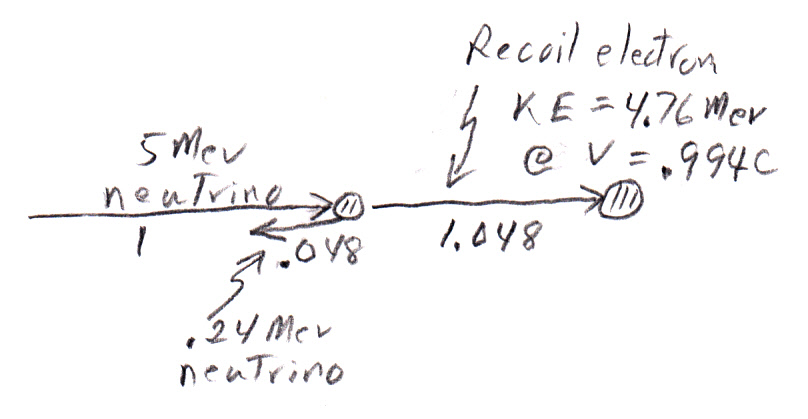
I confirmed the transfer equation I found in the Dutch paper is correct. It can be derived (without using the wavefunction) the same way as the hyperphysics sketch above shows for a photon-electron collision. The result for a 5 Mev incoming neutrino, which is x10 the mass-energy of an electron, squarely hitting a (stationary) electron is a lot like the photon momentum diagrams (above) and for a 5 Mev photon would be (I think) exactly the same.
Solving for relativistic momentum and energy conservation shows that most of the neutrino energy is transferred to the electron (very different from what a classical elastic calculation shows). The electron's output momentum vector (oriented along the neutrino input path) is slightly longer than the incoming neutrino momentum, so to balance up the momentum the momentum of the outgoing neutrino has to be negative, i.e. it has to bounce directly back along the input path. Nowhere have I seen this mentioned for a neutrino scatter collision! The momentum picture for a neutrino-electron bounce is basically the same as a (square hit or 180 degree) photon-electron bounce, which also shows the outgoing photon going directly back along the incoming photon path.
Does the compton formula predict the same energy transfer
for a 5 Mev photon-electron impact?
From above
I could see that the neutrinos and photons colliding (elastically) with
electrons seemed to produce about the same electron recoil, but to be sure
I checked out the compton formula for a 5 Mev photon, a photon with x10
the rest mass energy of the electron. The neutrino analysis above for a
5 Mev neutrino hit shows the the outgoing neutrino has (1/21), or about
4.8%, of the energy of the incoming neutrino. The question is, does the
compton formula also give a (1/21) ratio for the energy of the outgoing
photon over the incoming photon?
Using the notation I used above the constant in the compton formula is lamda0, which is the wavelength of a photon with the rest mass energy of an electron (0.5 Mev). A 5 Mev incoming photon has a wavelength x10 smaller or (lamda0/10). For 180 degree angle of the outgoing photon, i.e. where a photon that bounces directly back, the angle multiplier is two [(1- cos(theta)) = 2]. Hence the compton formula says to find the wavelength of the outgoing photon take the incoming photon wavelength and add twice lamda0 or
wavelength outgoing = wavelength incoming + 2 x lamda0
= lamda0/10 + 2 x lamda0
= lamda0/10 + (20/10) x lamda0
= (21/10) x lamda0
= 21 x lamda0/10
= 21 x wavelength incoming
(check)
Yup, same exact factor of 21 from the compton formula. Compton says the outgoing photon has a wavelength equal to x2 the wavelength of 0.5 Mev photon added to the much shorter wavelength of an incoming 5 Mev photon making the output wavelength x21 longer than the incoming wavelength. Hence the outgoing photon carries away only (1/21) x 5 Mev = 0.24 Mev, so the energy transferred to the electron to start a recoil is 4.76 Mev, which is 95.2% of the incoming photon energy, the same as for a 5 Mev incoming neutrino (or almost exactly the same as the tiny neutrino rest mass energy is ignored).
Obviously the photon-electron (impulse) calculation is important for gamma ray detection, because it shows that a gamma ray photon impact can give a real kick to an electron (in an atom). While the references are not 100% clear, I assume that because the photon energies are much higher than in the photoelectric effect that the electron 'recoil' here, which can approach relativistic speeds, is sufficient not only for an electron to escape from the atom, but also to travel a significant distance and potentially escape from the material.
Free or in an atom?Relativistic collisions
The confusion about whether the electron is free or in an atom may be because it doesn't really matter. Compton scattering applies to high energy photons which typically have energy far above (x10,000 or so) the ev type ionization energy of valence electrons. The Compton calculation assumes the velocity of the electron is zero, ignoring orbiting speed if the electron is in an atom. This is probably a simplifying approximation justified because outer electrons in an atom don't move that fast, so it is likely the momentum of the photon coming in is far higher than the outer orbiting electron.Static electron is a good simplifying assumption
I know the speed of the single electron in hydrogen is well known and would be typical of the speed of the speed of the outer electrons in most atoms, so I checked, and its speed is a little under 1% of the speed of light. Since recoil speeds in the 500 kev range are above 70% of the speed of light, the compton assumption that the electron is not moving looks like a good simplifying assumption.
Eph - Eph' = (Eph x Eph')/E0 x (1 - cos(theta))
where
Eph = energy of incoming photon
Eph' = energy of scattered photon
E0 = rest mass energy of electron
Electron - positron annihilation (footnote)---------------------------------------------------------------------------------------------------------------------------------------------------------
When positrons are released, references almost always say a pair of 0.511 Mev photons result when the positron hits an electron and the two annihilate. Well it appears there's a hidden assumption there, which is that the KE energy of the positron and electron are low compared to their rest mass energy. I wonder how true this is. I read Kamiokande detected the 1987 supernova anti-neutrino triggered positrons because they were moving relativistically. The Yale tutorial calculates the case where an incoming positron has 30 Mev and hits a stationary electron.Clearly post collision the total rest mass energy of missing particles (1.022 Mev) has to be carried off by the (two photons), as these are the only outputs. When the incoming particle is moving slowly, the only (significant) energy for the photons is the rest mass energy of the equal mass particle and anti-particle, and it divides equally between the two photons, so each photon is 511 kev Mev. (Two photons in opposite directions are needed to conserve momentum.) When the input particle comes in relativisticly though, the situation is different. The Yale tutorial shows the rest mass energy does not divide equally between the two photons.
The report works the case where the input particle comes in highly relativistically at 30 Mev (total energy) = [29.5 Mev KE + 0.5 Mev rest mass]. It shows the photon that leaves in the direction of the incoming particle gets 3/4th of mass loss energy 30. 25 Mev = [29.5 Mev KE + .75 x 1 Mev (rest mass)]. The total energy before and after is 30.5 Mev = [29.5 KE + 0.5 Mev + 0.5 Mev], so the backward going photon must have 0.25 Mev = [30.5 Mev total - 30.25 Mev forward photon]. Summarizing: forward photon (effectively) retains all the high KE of the incoming positron and picks up 3/4th of the released mass energy. The backward going photon has as its total energy 1/4th of the released rest mass energy, so it has only half the energy (and frequency) it would have had if the incoming positron had been moving slowly.
Quotes
from the 1979 Noble award ceromony to Glashow, Salam and Weinberg for Electroweak
theory
-- "Electron
and the neutrino belong to the same family of particles; the neutrino is
the electron's little brother
-- It was formerly assumed that weak processes could occur only in connection with a change of identity of the electron to neutrino (or vice versa); such a process is said to proceed by a charged current, since the particle changes its charge. The electroweak theory predicts that there should also be processes connected with a neutral current in which the neutrino - or else the electron - acts without changing identity. (These are 'bounce' reactions.)
-- Infinities were removed after WWII (by Feynman, et al) by assuming the observed value of m and e not with the 'ideal' value (value in Lagrangian), but the calculated value taking into account that fact that the electron and photon are always surrounded by clouds of virtual photons and electron-positron pairs. (This made possible all sorts of calculation, which have been confirmed experimentally with spectular precision.)
-- In 1974
the discovery of J/phi meson made it possible to believe in a system of
four quarks and four leptons. Soon thereafter came two experimental surprises:
evidence for a third charged lepton (tau) and for a -1/3e charged quark
(bottom), which led to a prediction that a top quark must exist (not found
as of 1979). "If it exists at all, must be heavier than 30 Gev." (turned
out to be 173 Gev, found 1995)
-----------------------------------------------------------------------------------------------------------------------------------------------------
Matter and anti-matter
Anti-matter
is often introduced in schools something like this: 'All matter and force
particles have an anti-matter twin'. It would seem from this that by including
the '-anti' world there must exist twice as many matter and force
particles. A close looks shows this is just not true, it is sort
of a semantic game the physicists play.
Sure every particle has an 'anti-' version, but then when you dig in you find at least one particle, the photon, is its own anti-particle. Wikipdia 'photon' puts it this way (equivocating a little), "Seen another way, the photon can be considered as its own antiparticle." What? Seems to me it's more accurate to say there is no anti-photon. Now I suspect that deep in the quantum mechanics something mathematically gets flipped, maybe just a sign, and some particles either don't have the term to be flipped, or maybe it gets double flipped, or when flipped it ends up being another particle.
The later case, i.e. ends up being another particle, is the case with the W particles. In the matter world there are two W particles with opposite charges: W+ and W-. Since what gets flipped is usually identified as charge, surprise, the anti-particle of W+ is W- and vice versa. In other words another semantic game! Including anti-particles in the count does not introduce new W particles, the total number of matter and anti-matter W particles is still two.
What is flipped to make an anti-particle is usually (in popular science texts anyway) is identified as charge.
-- A Scientific American article by two particle phyicists defines anti-matter this way: "The mass of any antiparticle is identical to that of the particle. All the rest of its properties are also closely related, but with the signs of all charges reversed." 'Closely related', what does this mean? Does it mean properties other than charge can also be reversed?
-- Wikipedia (anti-matter) says: "In particle physics, antimatter is material composed of antiparticles, which have the same mass as particles of ordinary matter but have opposite charge and other particle properties such as lepton and baryon number." This sentence is not clear. Does it mean that anti-matter besides having an opposite charge may also have an opposite lepon and/or baryon number? I think it might. In beta- decay an anti-neutrino (-1) is output along with an electron (+1), while in beta+ decay a neutrino (+1) is output along with a positron (-1), which is an anti-electron.
-- If a particle has other attributes (such as an electric charge Q), then the anti-particle has the opposite attributes (or a charge of -Q). The neutron--although electrically neutral--has a magnetic moment opposite that of the anti-neutron. Protons and neutrons have another quantum number called the baryon number, which also has the opposite sign in the corresponding anti-particles. Particles and anti-particles have the exact same mass and equal, but opposite charges and magnetic moments; if they are unstable, they have the same lifetime. (This is much more clear, from a physics doctoral student at Harvard)
We have already seen in the case of the photon, which has no charge, the game is played that it is its own anti-particle. And in the case of W where positive and negative charge versions exist, the game is they become each others anti-particle.
But what about neutrinos? They have no electrical charge. Are they, like the photon, their own anti-particles? Nope, well that is the present understanding, the state of the art as of 2013, but the Scientific American article, and Wikipedia too, suggests a degree of uncertainty, that experiments are being run to see if maybe they are their own anti-particles. In neutrinos something else, a sort of chirality (handedness) substitutes for charge and flips to make an anti-neutrino.
On the one hand it seems remarkable that matter can just 'disappear' when it hits its anti-matter twin, the reaction converting the mass of both into pure radiation (photons). Well, it is not quite so surprising when you look at particle physics reactions where you see all sorts of transformations. For example, neutrino absorbed by a nucleus, appears to convert to an emitted electron while at the same time it 'triggers' a radioactive like (n => p) conversion. But what really makes the conversion of matter and anti-matter into photons not so surprising is that the reaction goes the other way too!
Energy into particles
A high energy photon (E
> 2 x 0.511 Mev) can just spontaneouly convert into matter plus its anti-matter
twin, with them shooting off in oppisite directions to preserve conservation.
This makes it pretty clear that the anti-matter must have the 'opposite'
all the unique properties The most common of these reactions being a photon
converting into electron - positron pair. Particle physicists call this
'pair production'.
-----------------------------------------------------------------------------------------------------------------------------------------------------
Neutron optical
detection
Turns out
experimental phyicists have been working on various ways to detect free
moving neutrons for a long time, probably back to WWII. Wikipedia
has a page on neutron detection. A basic method for neutron detection is
use of a scintillator material. I stumbled on a patent from an Oak Ridge
guy (US 2.719,127 'Neutron-Sensitive Scintillators') for an improved neutron
detecting scintillator, and the date of the patent is 1955! He explains
that a neutron not being charged does not itself ionize material it is
moving through, but a scintillator materical (some sort of phosphor) only
flashes when ionized. The trick to getting this type of optical neutron
detector to work is to add a 'dopant'. This is an element that when it
aborbs a neutron outputs a (moving) charged particle that does the ionizing
of scintillator material.
The neutron absorber here is lithium, and this patent is proposing an improved lithium compound (lithium iodide activated by a small amount of europium) to work with the scintillator. Here's a figure from the 1955 patent showing a clean light pulse that was produced by slow neutrons. (This figure is counts/sec from a stream of slow neutrons, but the patent makes clear that this does work for detecting single neutrons.) The pulse is labelled 4.785 Mev, which may be the frequency of a gamma flash.
Scintillator doped with lithium iodide detecting slow
neutrons
US patent 2,719,127, Fig 1, 1955
I think I see how this works. About 7% of the lithium in lithium iodide would be the isotope lithium 6. It is both a strong absorber of neutrons and when it absorbs a neutron ('neutron activation') it transforms into helium and tritium. It's one of the ways of manufacturing tritium for hydrogen bombs. This is a strongly exothermic reaction so probably some of the electrons get stripped off (either the tritium or helium) becoming ions which excite the scintillator. Wikipedia (tritium) has this equation:
Li6 + n => He4 (2.05 Mev) + T (tritium) (2.75 Mev)
What is puzzling
is that a neutron added to Li6 would make Li7, and Li7 is stable. But on
more reading I see that the reaction goes only when neutrons with energy
above an energy threshold are used, and the description of the reaction
says an 'alpha particle' is ejected. This makes it more likely that the
ionizing particle is the ejected alpha particle.
-----------------------------------------------------------------------------------------------------------------------------------------------------
Periodic
chart isotope decay modes
Here's a very
interesting plot from Wikiedia (beta decay) showing the decay modes for
all (or nearly all) isotopes of all elements, in other words stability
of every possible atom! The big pattern is blue and orange on either side
of the stable black staircase.
Too many neutrons for stability
Isotopes with
too many neutrons for stability (blue) shed a neutron in an (n => p) classic
beta- decay. This increases stability in two ways: one, it moves the element
up the periodic chart one step, where it can support more neutrons, and
two, it has one less neutron.
Too few neutrons for stability
The opposite
happens in the orange zone, where the isotope has fewer neutrons than it
needs for stability. In this case (if energy allows) it creates a neutron
with a (p => n) reaction, either beta+ decay or electron capture, and by
moving down the periodic chart one step less neutrons are needed for stability,
and it has one more neutron.
Note alpha
decay in the yellow zone is a little more problematic. It tends ot occur
in isotopes that are the most neutron poor. While an alpha decay in the
stable element zone (below atomic# 82) helps stability by walking down
the periodic table by two steps, it loses two neutrons in the process.
However, the big alpha yellow zone above element #82 does make sense. There
are no stable isotopes for atoms with 83 or more protons, so protons must
be shed, and an alpha emission is efficient way to do this. My guess is
an alpha decay (by itself) probably doesn't help stability all that much,
so I would expect it to be part of a decay chain with further steps probably
some (p => n) beta+ reactions.
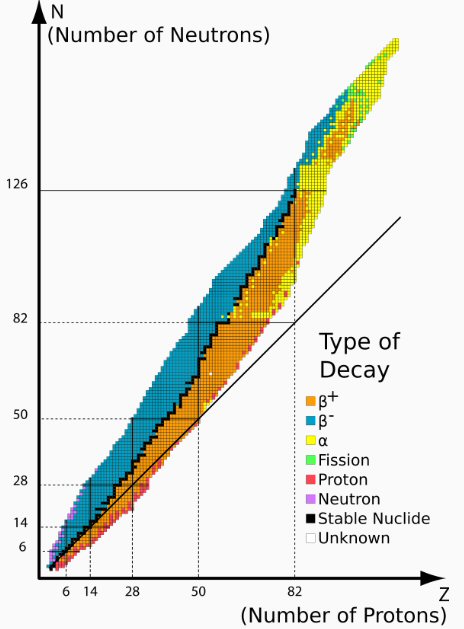
Lead (#82) is the heaviest stable element
(source -- http://en.wikipedia.org/wiki/Beta_decay#.CE.B2.2B_decay)
As a check I looked at the many isotopes of sodium (#11) on Wikipedia, and the pattern is clear and agrees with the plot. The one stable isotope of Na is Na23, which is 11 protons and 12 neutrons. Na's lighter isotopes all decay via beta+ (p => n) moving down one step, and its heavier isotopes all decay via beta- (n => p) moving up one step.
So how do beta+ (p => n) decays occur spontaneously when a neutron has more mass than a proton? Wiki (beta+) explains this can happen only when the daughter's binding energy is higher than the mothers, meaning its mass is lower. I checked this for three lighter isotopes of sodium (Na 22, Na21, Na 20), which decay (respectively) into Ne 22, Ne 21, Ne20 and sure enough the mass of the neon isotopes is less than the corresponding sodium isotope, but by just a hair.
Na 22 21.9944
Na 21 20.9976
Na 20 20.0073
Ne 22 21.9913
Ne 21 20.9938
Ne 20 19.9924
--------------
---------------
-------------
0.0031
0.0038
0.0149
A proton, one atomic unit, is about 938 Mev, so 0.0031 atomic unit difference for Na 22 => Ne 22 provides 2.9 Mev [0.0031 x 938 = 2.9] to drive the reaction. This is enough to provide 0.511 Mev to create the positron leaving 2.4 Mev to split between the kinetic energy of the positron and the emitted neutrino. The Na 20 beta+ decay sees about a x5 times bigger decline in mass, so its decay releases about 15 Mev.
Here's another cool related plot showing the decay times for all isotopes of every element! I found this in a related Wikipedia page (List of elements by stability of isotopes). The big picture is clear. The blue zone, those isotopes far above (too many neutrons) or far below (too few neutrons) the stable weight zone decay the quickest, usually less than a few seconds.
Elements #43 (technetium) and #61 (promethium)
have no stable isotopes
Note there's a little island of almost stability (billion
year lifetimes) at #90 (thorium) and #92 (uranium)
(source --- http://en.wikipedia.org/wiki/List_of_elements_by_stability_of_isotopes)
Is the photon a composite of two neutrinos?--------------------------------------------------------------------------------------------------------------------------------------------------------
Near the end of writing this essay I stumbled onto something new and totally off the wall. A theory called the 'Neutrino speed of light', which postulates that the photon may be a composite particle made up of a neutrino and anti-neutrino. This theory has its own Wikipedia page, very theoretical, nearly all equations that only a physicist could follow, then at the end of the page is this: "Since massless neutrinos are needed to form a massless photon (and it is now pretty clear that neutrinos have mass), a composite photon is not possible." (Whoops)Apparently the reason for the Wikipedia page is historical. The suggestion that the photon might be composite of neutrinos goes back to de Broglie in the 1930's with some scattered interest since, but the discovery of neutrino mass has pretty much killed this theory.
Deep inelastic scattering-----------------------------------------------------------------------------------------------------------------------------------------------------------
Digging deep into particle physics I read that neutral current neutrino quark interactions often described as 'deep inelastic scattering'. (See https://fehcom.net/WA21/written by a CERN researcher) These interactions would show up 20-30 years ago on bubble chamber photographs and were used to probe the inside of protons and neutrons, to get a 'look' at the quarks inside and provided experimental evidence that quarks existed. In books describing the proton-proton collisions at the CERN hadron collider it is pointed out that protons can be though of as bags of quarks and gluons, and when struck hard everything fly apart and reassembles in plethera of short lived particles (pions, kaons, etc). The early neutrino bubble chamber hits are described in these terms, sort of a miniature version of the massive hadron collider collisions.
Neutron decay
Neutron decay
is controlled by the weak force. It's a very simple weak force diagram,
in fact it was my starting point (above) in understanding neutrino detection.
When an isolated neutron decays, it turns into a proton, to maintain charge
balance also an electron, to maintain lepton# also an electron anti-neutrino.
The Scientific American figure shows that the combined spins of the output
products equals the spin of the neutron (+ 1/2). The proton has the same
+1/2 spin as a neutron. The spins of the electron and electron anti-neutrino
are opposite and cancel.
From a deeper perspective the neutron becomes a proton because one of its down quarks turns into an up quark. This quark change is 'mediated' by the weak W- boson that carries off the charge difference in the two quarks, the W- decaying into the an electron and electron anti-neutrino. As usual the energy released by mass decrease is carried away by the kinetic energy of the three output products.

basic (n => p) beta- decay
Quark change (Dn => Up) is mediated by weak W- boson
which decays into an electron and anti-neutrino
(source --- http://www.slideshare.net/asober/feynman-diagrams)
Bottle vs beam
Neutrons are
difficult to bottle up but if the neutrons are very cold and bottle is
very smooth, the neutrons bounce off and remain trapped. So in this experiment
they put some neutrons in a bottle and measure the decay with time of the
number of neutrons in the bottle. The US approach is to build a charge
trap into a neutron beam with the assumption that the protons captured
come from decaying neutrons. Clearly both approaches work because they
give similar answers (14.6 to 14.8 seconds), but these experiments were
intended to be precise, so neither team is happy that there is a 1% difference
(9 sec).
footnote
I found the
spin of the antineutrino to be confusing. Wikipedia (neutrino) shows it
to be +1/2, but the scientific american article shows it to be -1/2. In
the Scientific American article the antineutrino spin (-1/2) and electron
spin (1/2) are shown cancelling so that the spin of the output proton (1/2)
will equal the spin of the input neutron (1/2). All this is consistent.
But Wikipedia shows the spin of W-, from which the electron and antineutrino
come, as +1. Also consistent internally, but not consistent with the Scientific
American article. I find it hard to believe that the authors, who are the
actual neutron researchers, don't know how to describe the spins of its
decay.
I left a note
in the neutrino article about this. It might be there is no convention
to convert right and left handed neutrino spins to plus and minus notation.
-----------------------------------------------------------------------------------------------------------------------------------------------------------
Neutrino references
First are
two fairly technical neutrino reports (20 page or so) from the mid 1990s
that describe the major neutrino detectors of the time. I find this is
the best place to find the neutrino targets for the various detectors (in
the form of the detection equations).
http://www.nap.edu/openbook.php?record_id=9185&page=16
http://www.slac.stanford.edu/econf/C990809/docs/suzuki.pdf
40 min Nobel lecture (2002 physics) on how Kamiokande works by its designer. Tutorial on relativistic elastic collisions by a Yale prof (photon and neutrino collisions with electrons)
http://www.nobelprize.org/mediaplayer/index.php?id=514
http://arxiv.org/pdf/1011.1943.pdf
Neutrino - electron scatter calculation (by a Dutch prof of Nuclear Physics) --- shows maximum energy transfer is same as photon - electron calculation (p 140). 2nd reference is a Yale tutorial on relativistic collisions
https://www.kvi.nl/~loehner/saf_seminar/2010/neutrino-electron-interactions.pdf
http://arxiv.org/pdf/1011.1943.pdf
Atmospheric neutrino talk (2004) (26 slides)
http://www.docstoc.com/docs/122541453/Atmospheric-Neutrinos
Power point presentation about Sudbury detector, and power point style presentation from Nestor (like IceCube, but under ocean) on use of high energy neutrinos for astronomy.
www-pnp.physics.ox.ac.uk/~wilson/presentations/neutrinostory.ppt
http://www.inp.demokritos.gr/nestor/seminar/lkr_presentation.pdf
Wimp detection reference
http://pdg.lbl.gov/2011/reviews/rpp2011-rev-dark-matter.pdf
==================================================================================================
Early notes
This section
I wrote before I knew anything about neutrino detection equations. Here
I was playing around trying to 'derive' a neutrino equation starting with
the classic beta reaction that outputs a neutrino.
Maybe a starting point (for detection) is the equations of beta decay. In negative beta decay an atom gains a proton walking up the periodic chart as a neutron 'changes into' a [proton + electron + anti-neutrino]. The rest mass of a neutron exceeds that of a proton and electron combined so energy is available to be released, so this is an exothermic reaction.
n => p+ + e- + anti-(electron) neutrino exothermic negative beta decay
If we add a neutrino to both sides( and neutrino + anti-neutrino 'cancel'), then we would get below, which is in fact one of the neutrino detection formulas.
n + neutrino => p+ + e- + [anti-neutrino + neutrino]
The above formula agrees with this (possible) neutrino detection diagram I found. The text has this as a neutrino interacting with a neutron (via W+). If above is exothermic, then positive beta decay (below), where an atom loseing a proton walks down the periodic chart, as a proton 'changes into' a [neutron + anti- electron (positoron) + neutrino] must be endothermic. How it acquires energy to drive the reaction at this point I don't know. (I later found out that due to binding energy variations in different elements of the same atomic weight, it can be exothermic too. I show an example later in the essay.)
p+ => n + e+ + (electron) neutrino enothermic positive beta decay
Playing with the above equation, add an anti-neutrino to both sides. The [neutrino + anti-neutrino] do what? cancel or release energy, probably high energy photons.
p+ + anti-neutrino => n + e+ + [neutrino + anti-neutrino]
A textbook gives the equation (below) and says, this is how an anti-neutrino can be detected. The implication is an anti-neutrino interacting with a proton via the weak force, because that is the only way neutrinos interact, yields a positron and (maybe) some energy from canceling neutrinos. My guess is the photodectors lining the vat of liquid are used to detect a flash when the released positron hits an electron and converts to photons. Yup
p+ + anti-neutrino => n + e+
Algebra like modifications on equations with neutrinos(update)
Looks like doing
algebra like modifications on equations like above (canceling neutrinos)
is probably valid. I have been able to draw a valid looking feynman diagram
for both of these equations. Moving the neutrino from one side of the equation
to the other shifts it from being an input (left) to an output (right).
Conservation of leptron numbers in the feynman diagrams shows if the neutrino is an output it must have the opposite lepton number of the particle, i.e. it must be an anti-neutrino (anti-lepton) if the output is an electron (leptron). On the other hand if the neutrino is an input it must have the same lepton number as the particle output, so neutrino (lepton) in pairs with electron (leptron) out, and anti-neutrino (anti-leptron) in pairs with positron (anti-leptron) out.=================================================================================================