CO2 in atmosphere (by volume).
Current level is 383 ppm. The preindustrial baseline
is taken as 280 ppm (by consensus).
My non-technical essay on Global Warming is here: Global
Warming
Go to homepage
How the greenhouse
effect works
Radiative
equilibrium -- no atmosphere
Stefan-Boltzmann
law
Blackbody
calculation trap
Simple
greenhouse model -- with idealized atmosphere
Greenhouse HS
talk (2/9/11)
How does CO2 change the greenhouse effect?
Energy
flows of the real earth atmosphere and surface
Calculating
the two temperatures of the atmosphere
Calculating
temperature increase for doubled CO2
Improving
the greenhouse model
Murky
pond analogy
Notes
on radiative modeling the troposphere
Three
different ways to figure global warming
Mainstream
method --- Hansen & IPCC
Alternative
view #1 (no problem) --- temp rise linear with greenhouse power
Alternative
view #2 (huge problem) --- temp rise linear with CO2 ppm
Alternatvie view #3?
NASA on atmospheric temperature
Hansen's 2004
Scientific American article
Ocean temperature rise
CO2 and oceans
CO2 and ice ages
Global
warming in the old days, or CO2 in early earth
Anti-greenhouse effect
Temperature proxies
How
accurate are paleotemperatures?
Reasons to be skeptical
Reasons to really
worry
Won't
the added CO2 stay in the atmosphere nearly forever?
Greenhouse HS
talk
Global warming overview
There
are three major aspects to the global warming issue:
1) Direct effects --- The greenhouse effect is well enough understood so that a simple perturbation calculation can be made with reasonable accuracy (+/- 25% or so). This provides only the direct effect (power and temperature) of added CO2. These calculations do not include (any) amplification effects of feedbacks and assume the climate responds linearly to the anthropogenic forcing. These calculations yield a relatively small temperature increase of 1.2C for a doubling of CO2.
2) Amplifiers --- Feedbacks within climate system are much more poorly understood. One of the principle ones is water vapor in the atmosphere which is known to increase with temperature because atmospheric water vapor is at saturation levels and the saturation level is strongly temperature sensitive. Amplifying effects are estimated (not calculated). Houghton's book estimates that (principally due to water vapor) direct temperature effects caused by CO2 (or the sun) will (likely) be doubled, meaning a doubling of CO2 with amplifying effects included is expected to cause a temperature rise of (2 x 1.2C) = 2.4C. Feedbacks are assumed to be basically linear and stable, so that they cause a simple scaling (multiplier) of the calculated direct climate effects.
3) Non-linearities --- Detailed, sequential, paleoclimate data (ice cores and sea cores) show the earth's natural climate is very variable. The data shows the climate often does not respond in a slow, linear, way to forcing, it responds with rapid large jumps. The paleoclimate data appears to show that the climate (atmosphere and oceans) have more than one stable mode and that a small forcing can cause the climate to rapidly jump to a new mode (warmer or colder). In other words the climate system may not respond in a linear way (as assumed in #'s 1 and 2 above) to forcings.So there you have it. The risks of global warming are 1) The small effects of CO2 are calculable, 2) The larger amplifying effects can only be estimated, and 3) The risk of really disastrous effect of the climate shifting into a new mode, which has happened repeatedly in the past, are entirely unknown. A further source of uncertainty is that it is unclear to what extent measured increases in earth's temperature in the last 100 years are due to variability in the sun.The large, non-linear responses (popular jargon is 'climate surprises') seen in the climate record causes climate scientists to really worry about the risks of anthropogenic increases in CO2. There is almost no understanding of non-linear climate effects, so no one has any idea when a critical threshold might be crossed. The worry is that anthropogenic increases in atmospheric CO2 might cause a 'climate surprise', but unfortunately with present knowledge it is impossible to figure (or even guess at) what is the risk of this happening.
How firm is the case that CO2?
I think the case for CO2 producing some moderate warming is pretty good,
but the case that it is going to produce a lot of warming is pretty weak.
Here's an overview:
CO2 is known to be greenhouse gas, and measurements show that levels of CO2 in the atmosphere have risen about 33% since the industrial revolution, and as long as fossil fuels continue to be burned it will continue to rise. The observed increases in CO2 are consistent with the amount of carbon released from fossil fuels, so there is little doubt that man is causing the CO2 increase.
The understanding of the atmosphere, greenhouse effect, and earth's power flows is good enough that fairly accurate calculations can be made as to how power radiated to space is reduced as CO2 levels rise and how much the earth's temperature needs to increase to restore power equilibrium. These calculations yield a (final) temperature rise of about 0.5C rise at today's 370 ppm and an additional (final) 0.7C rise (1.2 C total rise) for a doubling of CO2 from its pre-industrial baseline of 280 ppm to 560 ppm. {1.2 C = 0.33 C/w/m2 x 5.35 x ln (560 ppm/280 ppm) = 0.33 C/w/m2 x 3.7 w/m2}
Clouds are an important factor in earth's energy balance, but there is a problem with clouds. Clouds are not well enough understood to make reliable calculations. Some types of clouds cause net warming (high clouds increase the greenhouse effect) and other types cause net cooling (low clouds increase albedo). It is known that warmer air can hold more water vapor, so a warmer earth probably means a cloudier earth. The guessing is that more clouds means a stronger greenhouse effect and that clouds roughly amplify CO2 caused warming by a factor of two. But notice the cloud amplifying factor is basically a guess! Including the cloud amplifying factor the (final) temperature rise for a doubling of CO2 becomes 2 x 1.2C = 2.4C.
There is some evidence that the earth is warming. It's difficult to measure accurately the average earth temperature rise because temperature varies a lot over time and distance. But the generally accepted estimate for today's rise is 0.6C, which is in the ballpark for the rise calculated to be caused by CO2. Of course, earth's temperature could in principle be affected by changes in the sun, and there's historical evidence that the earth's temperature has been all over the place, but accurate measurements of the sun, only available from satellites for about the last 25 years, show only tiny solar variations (0.1% cyclic) with no up or down trend.
Reference CO2 & carbon numbers
There are
a few complications in dealing with CO2 in the atmosphere. One is that
some reference refers to the mass of CO2 and others carbon. Ratio of atomic
weights shows that 12/44 (27.3%) of the weight of CO2 is carbon. Second
is the use of tonne, ton, etc. The best unit to use is tonne, which (I
am pretty sure) is 1,000 kg, sometimes called a metric ton. so a gigatonne
(or a billion tonne) = 10^12 kg. (A kg is about 2.2 lbs, so a tonne is
about 10% heavier than an american/english ton.) Third is ppm of
CO2 in the atmosphere is usually quoted (383 ppm currently) by volume,
the ppm by weight is higher. Most of # below are from Wikipedia,
except for the table below that gives the carbon and energy content (by
weight) of gas, coal and oil.
|
|
|
(kg -C/kg) |
(relative to oil) |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
atmosphere total (current)
CO2 in atmosphere = 383 ppm by volume
(280 ppm preindustrial)
= 582 ppm by weight
mass of atmosphere = 5.14 x 10^18 kg
CO2 mass in atmosphere = 5.14 x 10^18 kg x 0.582 x 10^-3
= 3,000 x 10^12 kg
(3,000 gigatonne)
[CO2/carbon] (by weight) = (12 + 2 x 16)/12 = 44/12
= 3.67
carbon
mass in atmosphere = 0.273 x 3.00 x 10^15 kg
= 820 x 10^12 kg
(820 gigatonne)
27% of CO2 in the atmosphere is due to man
Baseline CO2 in preindustrial times = 0.73 x 3,000 x 10^12 kg
= 2,200 x 10^12 kg
(2,200 gigatonne)
Baseline carbon
in preindustrial times = 0.73 x 820 x 10^12 kg
= 600 x 10^12 kg
(600 gigatonne)
Increase (from baseline)
in CO2 in atmosphere = 0.27 x 3.00 x 10^15 kg
= 807 x 10^12 kg
(807 gigatonne)
Increase (from baseline) in carbon in atmosphere
= 0.27 x 820 x 10^12 kg
= 220 x 10^12 kg
(220 gigatonne)
annual changes in atmosphere
measured increase in CO2 = 0.55% per year (currently) (see below)
annual increase in CO2
mass = 5.5 x 10^-3 x 3.00 x 10^15 kg
= 16.5 x 10^12 kg/yr
(16.5 gigatonne/yr)
annual increase in carbon mass = 5.5 x 10^-3
x 820 x 10^12 kg
= 4.5 x 10^12 kg/yr
(4.5 gigatonne/yr)
estimates (man's CO2 output from fossil fuel and cement)
man's estimated CO2 emission = 27
x 10^12 kg/yr
(27 gigatonne/yr)
estimated atmospheric/ocean CO2 split
2/3 atmosphere
(18 gigatonne/yr)
1/3 ocean
(9 gigatonne/yr)
calculated -- oil
oil production worldwide = 82.5 mil barrels/day x 365 days/yr
= 8.25 x 10^7 x 3.65 x 10^2 barrels/yr
= 3.0. x 10^10 barrel/yr
(30 gigabarrels/yr)
1 barrel oil weight = 0.136 metric ton = 136 kg
[kg carbon/kg oil] = 0.85
carbon in annual oil production = 3.0. x 10^10
barrel/yr x 0.136 x 10^3 kg x (0.85)
= 3.5 x 10^12 kg/yr
(3.5 gigatonne/yr)
CO2 in annual oil production
= 3.67 x 3.5 x 10^12 kg/yr
= 12.8 x 10^12 kg/yr
(12.8 gigatonne/yr)
calculated -- coal
coal production worldwide = 6.2 billion tons/yr x [(2,000 lb) ton
= metric ton/1.1]
= 5.6 billion metric ton/yr
[kg carbon/kg coal] = 0.68
carbon in annual coal production = 5.6 billion
metric ton/yr x (0.68) (3.8 gigatonne/yr)
CO2 in annual coal production = 3.67
x 3.8 gigatonne/yr
(13.9 gigatonne/yr)
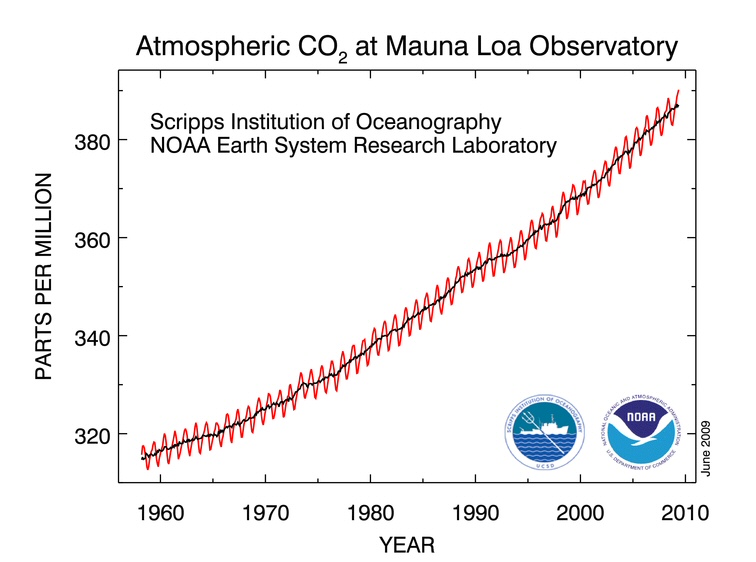
Current rate of CO2 rise
Eye balling
the curve (below), which is a plot of the best data on atmospheric levels
of CO2 (measured at Manua Loa in Hawaii), I estimated (averaging
out seasonal variations) that atmospheric CO2 levels had risen 10 ppm (370
ppm to 380 ppm) in the recent five year period 2000 to 2005. (When I later
dug out tabulated Mauna Loa data, it showed a 10.34 ppm rise in those five
years, confirming that my eyeball estimate was pretty good.) Hence the
current annual increase in atmospheric CO2 (2 ppm/yr) is 0.55%/yr = [10.34
ppm/5)/375ppm], which is also the per cent increase in carbon in the atmosphere.
Since the measured CO2 curve rises pretty smoothly with a gradually increasing
slope, its reasonable to ascribe the increase to man (mostly burning of
fossil fuels), not volcanos, which would produce a lumpy curve. This
is consistent with estimates that put CO2 from volcanos at less than 1%
of the CO2 from burning of fossil fuels.

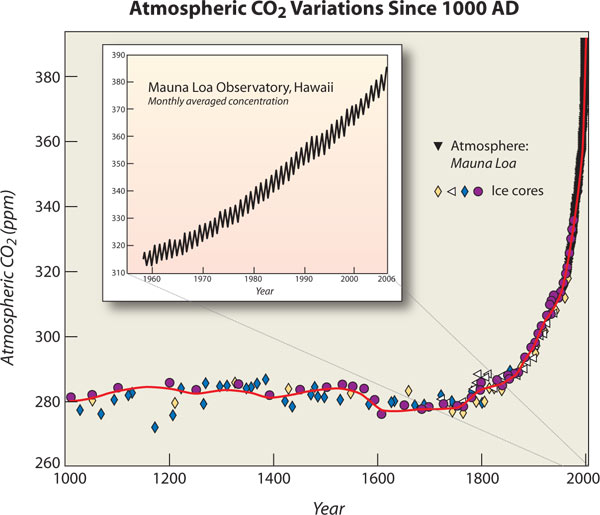
CO2 in atmosphere (by volume).
Current level is 383 ppm. The preindustrial baseline
is taken as 280 ppm (by consensus).
Here's CO2 atmospheric levels for 1,000 years, based on CO2 measured from trapped air in ice cores, showing a clear preindustrial baseline (in modern times) of 280 ppm.
Scott Doney, Woods Hole Oceanographic Institution
How do fossil fuel production numbers match up with
atmospheric CO2 measurements?
Annual
The carbon
content of annual oil production (3.5 gigatonne/yr) fits pretty well within
the measured annual increases in atmospheric carbon (4.5 gigatonne/yr).
From the numbers above we have 61% of man's estimated CO2 output (27 gigatonne)
going into the atmosphere with the remaining 39% (10.5 gigatonne) going
somewhere else, maybe mostly into the the ocean? If we guess that only
85% of oil is burned (remaining 15% going into plastics etc) and apply
the 61% split between atmosphere and ocean, we find 52% (0.52 = 0.85 x
0.61) of the carbon in oil production goes into the atmosphere.
Oil production carbon to atmosphere = 0.52 x 3.5 gigatonne/yr
= 1.8 gigatonne/yr
Fraction of atmospheric carbon increase
due to oil = (1.8 gigatonne/yr)/(4.5 gigatonne/yr)
= 40%
Using the Wikipedia worldwide coal production number (6.2 billion ton/yr) the calculation of annual carbon dug up from coal comes out to be about the same as oil, which seem reasonble. (Actually the calculation for coal comes out to be 10% higher than oil (3.8 gigatonne/yr vs 3.5 gigatonne/yr), but the carbon content of coal (per kg) varies widely (35% to 90%) depending on the type of coal, so it is hard to get a good average number for use in calculations like this.)
Summarizing, we get pretty good agreement between known oil and coal production numbers and measured increases in atmospheric CO2. Without fudge factors they agree within a factor of about two. With the estimated fudge factors we get roughly equal annual increase in CO2 from oil & coal, each in the range of 40-45%, with the balance coming from gas and cement. These are reasonable estimates.
From preindustrial times
World oil
production using a linearized Hubbert curve shows all the oil that will
ever be recovered at 2,200 gigabarrels, and we are now about at the one-half
point of 1,100 gigabarrels having been recovered. How much carbon is in
1,100 gigabarrels?
Carbon (1,100 gigabarrels) =
1.1 x 10^12 barrels x 136 kg/barrel x 0.85 (carbon fraction)
= 127 x 10^12 kg
(127 gigatonne)
This is an reasonable and interesting number. The increase in atmospheric carbon since preindustrial times is 220 gigatonne. If all of the carbon in recovered all is assumed to go into the atmosphere, then it 'explains' 58% of the increase in carbon since preindustrial times. And if assume that only 61% of this oil carbon makes it into the atmosphere (say 39% going into the ocean), then it 'explains' 35%. Since a good fraction of the atmospheric carbon increase since preindustrial times must be due to coal (plus some to gas & a little to cement), a calculated 35% (to 61% limit) coming from oil indicates very good agreement between the known carbon in total oil produced with the known increase in atmospheric carbon since preindustrial times.
How high can CO2 go?
I have
never seen this done, but it seems to be enough information is available
to make a reasonable hip shot estimate of how high CO2 levels in the atmosphere
can go. We figure out how much carbon there is in all the recoverable oil,
gas and coal and assume it all ends up in the atmosphere in the form
of CO2. A key to the argument is the peak oil analysis that says we have
at this point in time recovered (and burned) about half the oil we will
ever recover from the earth, and we know from Mauna Loa data how much CO2
levels in the atmosphere have risen.
For perspective
The baseline
calculations, and general political goal, of the IPCC and most climate
scientists is try and limit the maximum CO2 levels in the atmosphere to
560 ppm, which is a doubling of the preindustrial level of 280 ppm.
Consider the following
We have burned
through half of the earth's oil according to the peak oil theory, which
I think is right, and CO2 levels have only gone up 36.8% (from preindustrial
times). We can make a hip shot guess that this extra manmade CO2 has come
from the burning of oil (40-50%), coal (40-50%) with the balance
gas and cement. Thus as a rough guess burning half the earth's oil
has increased CO2 levels by 16-17% (= 0.45 x 36.8%), so burning up the
remaining half of the oil will only give us a similar rise (roughly another
16-17% ).
Looking at it more accurately (in terms of atmospheric carbon) the IPCC goal is for man to add no more than 600 gigatonne of carbon to the 600 gigatonne originally present in preindustrial times. We now have added 220 gigatonne by burning oil, coal (& a little gas) . With our hip shot estimate that 45% of man's 220 gigatonne contribution, or about 100 gigatonne, has come from burning half the earth's oil, then burning up the remaining oil (with no carbon capture) will add another 100 gigatonne.
From another point of view we have a pretty data that says burning up all the (recoverable) oil on earth (without carbon capture) will only contributed 1/3rd (200 gigatonne) of the 'allowed' (or budgeted) 600 gigatonne increase. So clearly the key question is how much carbon is there in the remaining, recoverable amounts of gas and especially coal?
natural gas
I could find no Hubbert (peak) analysis of recoverable natural gas estimates
worldwide, but Wikipedia says gas production in North America likely peaked
in early 2000 and North Sea gas production peaked in the year 2000 and
is now declining. However, the US has only 3% of worldwide gas reserves,
most gas is in the middle east and in Russia. I did locate a few
natural gas worldwide reserve estimates: 54, 344, 427 trillion meter^3.
Scaling by density (0.72 kg/m^3) and carbon content (76%) we get
Limit of atmospheric carbon from gas = 84, 188, 233 gigatonne
Comparing these to total carbon estimates of oil (254 gigatonne) and since gas has not peaked yet, we can roughly estimate that the final (non sequested) contribution of carbon from gas is on the order of oil.
Hubbert peak method -- coal
Wikipedia
says estimates of recoverable coal have been coming down, and it actually
has a Hubbert Peak estimate of the amount of coal that will ever be mined
of 450 gigatonne. (Wikipedia Coal -- http://en.wikipedia.org/wiki/Coal#_note-19
Ref is David Rutledge of Caltech).) (There are much higher estimates
about. Wikipedia under Hubbert (himself) predicted coal at 2,500 gigatonne.
The International Energy Agency says proven reserves of coal are
909 gigatonne, double the Wikipedia number!) (A tricky issue is coal
can be spread very thin and deep, so how much is recoverable?) Let's use
the Wikipedia 450 gigatonne number and check how much carbon this could
add to the atmosphere.
Limit
of atmospheric carbon from coal = 450 x 10^12 kg x 0.78 (carbon fraction)
= 350 x 10^12 kg
(350 gigatonne)
IPCC goal is CO2 560 ppm (vol) <==> 600 gigatonne
from man
Whoo, 350
gigatonne is a small number!! The general objective of the global warming
is limit the CO2 (& carbon) increase to 200% of preindustrial levels.
This means man's total carbon output needs to be held to 600 gigatonne.
If coal can only contribute 350 oil gigatonne, oil 254 = 2 x 127 gigatonne,
and gas (perhaps) the same as oil, and we apply the fudge factor that about
39% of this carbon goes into the ocean, we find the potential increase
in atmospheric CO2 (& carbon) from burning all the gas, oil
and coal is
0.61(350 + 254 +254) = 523 gigatonne
[600 gigatonne preindustrial + 523 gigatonne from man]/600 = 187% increase
Oceans
CO2 dissolves
easily in water and increased CO2 levels in the atmosphere are raising
the PH of the upper levels of the oceans. Wikipedia (CO2 article) states
about one-third of all human-generated CO2 emissions to date have
been absorbed by the oceans, most of it in the upper levels (1,000 ft or
so) raising the carbon level about 3% (but at another point in the article
the H+ ion level is said to have increased by 25%?). As oceans warm, however,
for several reasons they will be less effective at absorbing CO2.
Ocean pH levels are projected to decrease from 8.2 preindustrial to a little under 8.1 now tending toward 7.8 in 100 years as dissolved CO2 levels triple. (It's not clear from the article or figure if this projection is for the whole ocean or the upper levels.) The Wikipedia reference is a 2006 article in the Woods Hole magazine Oceanus (Doney, Scott C.; Naomi M. Levine (2006-11-29). "How Long Can the Ocean Slow Global Warming?")
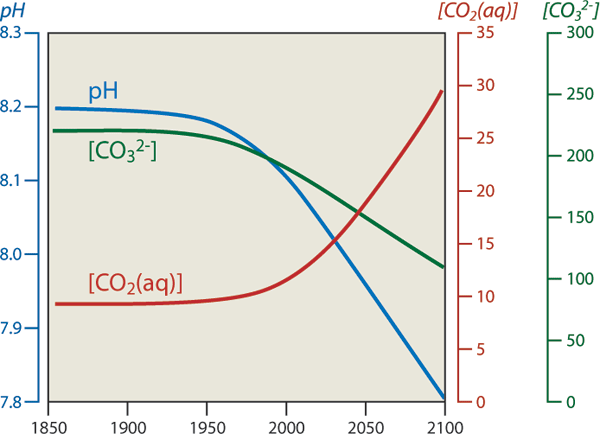
Not clear if this projection is for upper levels of
ocean or whole ocean
Scott Doney, Woods Hole Oceanographic Institution
Good news?
Good news?
With this (low?) estimate of 450 gigatonne recoverable coal and assumed
39% adsorption of (annual) CO2 directly into the ocean the numbers say
it is impossible for CO2 levels in the atmosphere to double, which
is the general goal of IPCC and global warming scientists. IPCC and global
warming goals would be met by doing nothing at all to minimize CO2
outputs!! (Even without any direct ocean absorption the increase is still
only 243% of industrial levels.) Clearly the big uncertainty here is how
much coal will we ever recover!!
In other words if remaining reserves of oil, coal, and gas are not too large, then global warming ceases to be a major problem! The CO2 rise will be self limiting within or near the target level of 560 ppm.. There will not be enough carbon in remaining fossil fuels that will be recovered to go higher. Clearly the global warming debate has a (pretty well) hidden assumption that the (total) reserves of oil, gas, and especially coal are large, so large that carbon sequestration (or removal) is going to be necessary!
Role of the sun
I like stories
of the little guy in science fighting the establishment. The establishment
says ignore the sun, focus on CO2, but Svensmark of Denmark has been the
pioneer of a tiny group that for a long time has been arguing that the
influence of the sun on climate is important (maybe even dominant over
CO2) via an indirect mechanism where the sun's magnetic activity modulates
the cosmic rays hitting earth, which affects cloud cover, which affects
the albedo (reflectivity) of earth.
Enough people are now convinced that this idea has merit that CERN is going to mount a huge, multi-year, 60 scientist, cosmic ray/cloud experiment to check it out with particle beams playing the role of cosmic rays. In a few years we should know a lot more about how clouds form and to what extent this process is affected by cosmic rays.
The abstract of the CERN CLOUD proposal (2000) is interesting. Here it is:
"Paleoclimatic data provide extensive evidence for solar forcing of the climate during the Holocene (last 11.5k years) and the last ice age, but the underlying mechanism remains a mystery. However recent observations suggest that cosmic rays may play a key role. Satellite data have revealed a surprising correlation between cosmic ray intensity and the fraction of the earth covered by low clouds (two references). Since the cosmic ray intensity is modulated by the solar wind, this may be an important clue to the long-sought mechanism for solar-climate variability. In order to test whether cosmic rays and clouds are causally linked and, if so, to understand the microphysical mechanisms, a novel experiment known as CLOUD has been proposed. CLOUD proposes to investigate ion-aerosol-cloud microphysics under controlled laboratory conditions using a beam from a particle accelerator, which provides a precisely adjustable and measurable artificial source of cosmic rays. The heart of the experiment is a precision cloud chamber that recreates cloud conditions throughout the atmosphere."Cosmic ray and cloud numbers
Cosmic rays hitting earth variation (over solar cycle)
10%
Low cloud cover variation (over solar cycle)
1.7%
Cosmic ray change in 20th century
-15%
(due to doubling of solar wind in 20th century)
Cosmic ray change during little ice age
+25%
Forcing due to 1.7% low cloud cover variation
1.2 w/m2
(eq to 1.2 w/m2 /.017 = 70 w/m2 low cloud reflection)
Forcing due to anthropogenic (man caused ) CO2 @ 2004
1.4 w/m2
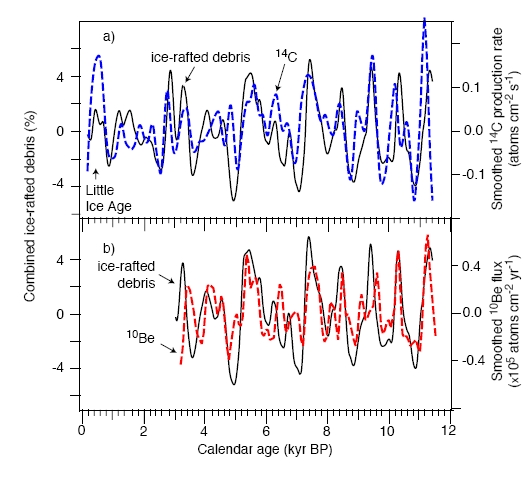
Correlation of climate (ice rafted
debris cold events in North Atlantic during Holocene (last 11k years) with
cosmic rays (C14 and Be10). (from CLOUD CERN documents, 2004)
Most of the numbers in the table above (and the fig above) come from a 2004 CERN memo (link below) written by the CERN CLOUD research team.summarizing recent data on the relationship between cosmic rays and climate.
http://cloud.web.cern.ch/cloud/documents_cloud/cloud_memo.pdf
Critical questions
Over the next
100-1,000 years will the oceans absorb, or outgass, CO2 as they warm?
(Analysis of ice age delays in CO2 response indicate (possible) outgassing)
What does
is the trend in ocean and deep bore hole temperature?
(This data should measure of forcing power/energy.)
Doesn't the
lack of warming of the troposphere indicated big problems with
climate models?
Isn't climate
so naturally variable and chaotic that unless antropogenic CO2 totally
dominates (over solar) isn't prediction hopeless?
Isn't it true
that the forcing concepts used for global warming predictions do not
in any way explain ice age cycles.
Isn't it true
that ice age cycles show how significant local variations can be globally.
Isn't it true
that the cause of ice ages was variations in earth regional solar heating.
Isn't it true
that at least 50% of the predicted temperature changes are due to feedback
and that feedback effects are very poorly understood?
Isn't it true
that the presumed feedback effects multiply any disturbance including solar
variations
Isn't it true
that considering only variations in solar energy output may underestimate
the effect the sun can have on the earth's temperature?
Isn't it true
that the data for the last 15 years (all the data available) shows a remarkably
good
correlation between (low) cloud coverage and cosmic rays (as measured on
the surface)?
Isn't it true
that close, apparently sun-like, stars show significant intensity variations
(up to 0.6%)?
Can it be
shown that deglacation increases in CO2 are, or are not, relevant
to todays anthopodxxxx increases in CO2? If they are, similar future
generations (might be) in big trouble.
Can we develop
far distance satellites to monitor earth albedo and/or temperature?
(We have a bunch of sun monitoring satelites)
Isn't it stupid
to worry about 500 years from now?
(Just a generation ago earth over population was thought to be a disaster.
No one
forsaw the 'green revolution' increases in agricultural productivity that
exceeded population growth.)
Isn't it hugly
hypocritical to politically worry about contaminating the earth's atmosphere
for
future generations while we are proceed to strip mine the earth bare of
energy and mineral
resources?
How the greenhouse
effect works
Imagine a
sphere is surrounded by a thin, isothermal shell of absorbing material.
Each square meter of the sphere's surface is radiating out power, say 2Po.
Each square meter of the shell absorbs the power from the sphere and reradiates
it, but because the shell has two radiating surfaces it radiates
half the power (Po) outward and the other half of the power (Po) inward
back toward the sphere.
In other words the shell radiates back to the sphere half of the power the sphere radiates out, so for the sphere/shell combo to radiate Po into space, the sphere surface must be hot enough to radiate 2Po to the shell. This is the greenhouse effect.The law of blackbody radiation (power = k x temp^4) tells us that to double the power radiated the absolute temperature of the body (or surface) must be higher by the fourth root of 2, which is an increase of 18.9% (2^1/4 = 1.189).
Applied to the earth this idealized greenhouse effect would mean an earth surface temperature of 303K, or 48C (87F) higher than the earth's radiative equilibrium temperature of 255K. While this is basically how the real atmosphere warms the earth surface, the real atmosphere differs enough from the a fully absorbing, thin shell to lower the greenhouse temperature rise from 48C to 33C, resulting in a surface temperature of 288K.
Here's a link to a cool animation showing CO2 molecules doing their thing. Note in the animation each CO2 molecule is shown as radiating in all directions. This is correct, but is still consistent with our two surface model when the whole atmosphere is considered, because it is only the radiation that 'leaks out' of the atmosphere that matters.
http://www.seed.slb.com/en/scictr/watch/climate_change/anim/greenhouse/index.htm
Radiative
equilibrium -- no atmosphere
update --- More
intuitively the 'no atmosphere' argument applies also for earth with an
atmosphere that is 99+% same was we have, an atmosphere of nitrogen, oxygen,
and argon, where only the greenhouse gases water vapor (0.4%), CO2 (390
ppm), methane (2 ppm), and a few other trace gases have been removed.
-------------------------------------
For an earth with
no atmosphere the calculation of the earth's surface temperature is simple.
By assuming that the earth reradiates the power it absorbs like a blackbody,
we can calculate the earth's surface temperature from the blackbody radiation
law and the absorbed fraction of incident solar power hitting the earth.
The latter is found by using the earth's size and distance from the sun
to figure what fraction of the sun's total power we intercept.
A fraction of the incoming solar energy hitting the earth's surface is assumed to be reflected rather than being absorbed. The reflected fraction, called the albedo, goes out at the same (high) visible frequencies as which it comes in, whereas absorbed power is reradiated out at a much lower (infrared) frequencies since the earth is a lot cooler than the sun. We set the earth's albedo to 0.3 in this no-atmosphere model (and in the following simple model with atmosphere), because the real (earth + atmosphere) is thought to reflect 30% of incident solar radiation.
The incident solar radiation hitting earth (per sq meter of earth's surface) is known to be (1/4) x 1,370 w/m2. The (1/4) comes from the ratio of a earth's spherical surface area (4 pi r^2) to the area of the earth's disk (pi r^2) that intercepts sunlight. The absorbed power (per sq meter of earth's surface) is
Absorbed solar power = (1- albedo) x (1/4) x 1,370 w/m2
= (1- 0.3) x (1/4) x 1,370 w/m2
= 240 w/m2
(range of 235 to 240)
Stefan-Boltzmann law
The earth
is assumed to radiate as a blackbody. The total radiated power under
the black body radiation curve is exactly proportional to the fourth
power of absolute temperature (measured in degrees Kelvin) scaled by the
Stefan-Boltzmann constant. The Stefan-Boltzmann constant is known with
high precision because it is a combination of fundamental constants like
planck's constant and speed of light. Here is the formula:
Power radiated (blackbody, total) = k x T^4
(Stefan-Boltzmann law)
where
T = absolute temperature (in K)
(-273 K = 0C)
k = 5.6704 x 10^-8 w/m2-K4
(Stefan-Boltzmann constant)
This is the key equation that allow the earth's black body temperature (radiative balance temperature) to be calculated. The calculation (with k defined using w/m2) uses for power the av power absorbed by each meter^2 on the earth's surface (240 w/m2). Since (power out = power in) at equilibrium, the power radiated out to space by (earth + atmosphere) must be (almost) the same as the power the (earth + atmosphere) absorbs from the sun.
Geothermal power flow to surfaceConducted geothermal power (0.06 w/m2) is 4,000 to 8,000 times less (< 0.025%) than absorbed solar power (240 w/m2), so it can be neglected in calculating the earth's radiative equilibrium temperature. (I don't have a number for heat to the surface from volcanos).
The caveat 'almost' is because the earth's surface receives a small amount of heat from inside the earth (combination of primal heat and heat from decay of a few unstable isotopes). This heat comes up via conduction through the crust and volcanos. Using the earth's crust thermal gradient (20C/km) and the av conductivity of rocks (1.5 to 3 w/m-K) the calculated geothermal heat conducted to the surface is(geothermal power conducted to surface) = 3 w/m-K x 0.02 C/m
= 0.06 w/m2
(radiated power) = k x T^4
so
T = (power/k) ^ 1/4
= {(240 w/m2)/(5.67 x 10^-8 w/m2-K4)}^1/4
= 255 K
255K (-18C) is the radiative equilibrium temperature of earth (as seen from outer space). In this no-atmosphere model 255K is the earth's surface temperature.
A good introduction
to blackbodies can be found here ---
http://hyperphysics.phy-astr.gsu.edu/hbase/mod6.html#c1
More on Stefan-Boltzmann law
The key to
the greenhouse effect is the stefan-boltzmann law [power = kT^4], but how
does this work really and how was it figured out? When researching my HS
talk on the 'History of the 'Atom and an Introduction to Quantum Mechanics',
I read that it was Plank in 1900 who figured out that energy in light was
proportional to frequency [E = h x freq], where h is plank constant and
] and quantized to whole cycles. Plank determined h (to within 2%) by matching
his formula to the data.
But the stefan-boltzmann law is formulated in the 1870's long before (presumably) the linear relation of light energy to frequency was understood, so herein lies a small mystery. How was the [total power = kT^4] law figured out? My initial guess is that this was done thermally, that heat radiated from a hot object was measured and it was found to follow a 4th law curve and yielded the proportionality constant.
Here are the related spectrum laws
1879 stefan boltzmann law
[total power = kT^4]
1896 wein's law
high freqency (power vs freqency) formula
1900 plank's law
1900 -1905 rayleigh (1900)
power varies as (1/wavelength)^4
rayleigh + jeans (1905)
(improved, obtain a proportionality constant)
From the dates it is clear that total power goes as the 4th power of (absolute) temperature was figured out much earlier (1879). Work on the spectrum power (how power varies with frequency) was done 20 to 30 years later (1896 to 1905) by Wein, Rayleigh, and Jeans, who came up with two approximate formulas, and Planck who came up with the exact formula.
Here's an interesting set of curves. Two approximate curves from models of Wein and Rayleigh-Jeans matched black body radiation spectrum (energy vs frequency) at high and low frequency respectively. In 1900 Plank's resonant oscillators model yields a formula that matches the whole spectrum by assuming:
1) Light power = h x frequency
2) Freqency is quantized (partial cycles don't count)
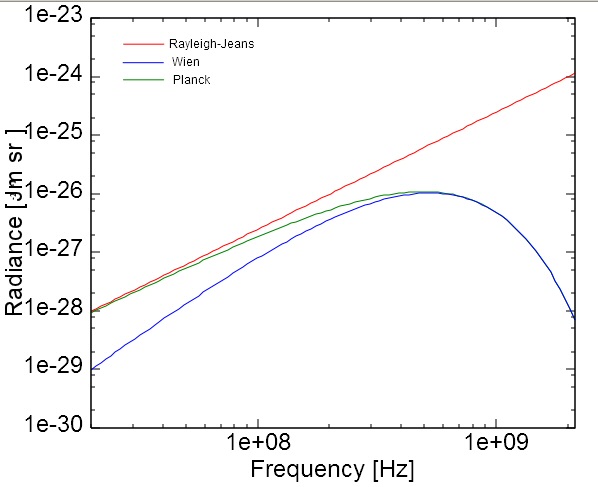
Black body radiation spectrum curves
source -- Wikipedia 'Wien approximation'
Taylor series expansion of e^x
e^x = 1 + x + x^2/2 factorial + x^3/3 factorial .... (for all x)
e^x = 1 + x (for x<< 1)
In plank's formula
x = hc/wavelength kT = h freq/kT = E/kT = eV/kT
From electronics I know r = 25 mV = kT/q at room temperature, so is k boltmann constant? Yes.
k = 25 x 10^-3 volt x 1.6 x 10^-19 coulomb/298 kelvin (25C)
= 1.34 x 10^-23 joule/kelvin check
(1.38 x 10^-23 joule/kelvin)
So at room temperature [kT = 25 meV]. Thus x in plank's formula [eV/kT] is < 1 in the infrared at room temperature (visible light energy is about 1.5 to 3 eV) and at higher temperatures at frequencies less then ultraviolet. In other words the e^x = 1 + x approximation can be used at lower frequencies.
Plank's formula (as given in Wikipedia 'Rayleigh–Jeans law')
Power (freq) = (2c^2/wavelength^5) x h/[e^(hc/wavelength kT)-1]
= (2c^2/wavelength^5) x h/[e^(eV/kT)-1]
or
Power (freq) = 2c^-3 freq^5 x h/[e^(h freq/kT)-1]
low frequency approximation (shows radiated power rising very rapidly with frequency)
Power (freq) = 2c^-3 freq^5 x h/[1 + (h freq/kT) -1]
= 2c^-3 freq^5 x h/[h freq/kT]
= 2c^-3 freq^5 x h/[h freq/kT]
= 2c^-3 freq^5 x kT/freq
= 2kTc^-3 freq^4 or
2kTc/wavelength^4
This is identical to the equation that Rayleigh and Jeans derived classically, the low frequency assymptote in the figure above.
Frequency roll off
When photon energy
(eV) becomes greater than 25 mv (@room temp, 300 kelvin) or 250 mv @(3,000
kelvin), both below visible light frequencies (1.5 to 3 eV approx), then
(eV/kT) is > 1 and the exponential in the denominator begins to grow with
frequency. Soon it is growing faster than freq^4 causing the distribution
to maximize and begin to roll off at high frequency. Check: black body
spectra (below) show the curve maximum at 3,500 kelvin is about 800 nm
(in the infrared just below the visible red at 700 nm) and at 5,500 kelvin
is at 500 nm (aprox visible blue).

black body radiation spectrum (power per nm) vs (absolute)
temperature
peak goes from slightly in infrared (800 nm) to visible
blue (500 nm) as temperature goes
from 3,500 to 5,500 kelvin
source -- Wikipedia 'Planck's law'
Blackbody calculation
trap
There is a
potential trap in doing blackbody calculations, a trap that I (briefly)
fell into. The atmosphere has two radiating 'surfaces' (up and down) whereas
the earth's surface has only one (up). When calculating the temperature
of the atmosphere, it's tempting to plug total w/m2, meaning power
radiation up + radiation down, into the Stefan-Boltzmann blackbody equation.
Total power gives the right answer with the earth, because there's just
one
surface,
but it gives the wrong answer with the atmosphere, which has two radiating
surfaces. The blackbody power radiated from a surface depends only on the
temperature of that surface. To calculate the upper (top side surface)
temperature of the atmosphere you use only the power radiated up,
and for the lower (bottom side surface) temperature
only
the power
radiated down.
Simple greenhouse
model -- with idealized atmosphere
Here is where
things get interesting. When an atmosphere is added to earth, we have two
blackbodies, the earth's surface and the atmosphere, and they can radiated
into each other. The simple model below (constructed by me) of an earth/atmosphere
system will be shown to produce a greenhouse effect, i.e. the equilibrium
temperature of the earth's surface will be found to be (significantly)
higher than the radiative equilibrium temperature of the whole system.
Our simple model of an earth/atmosphere system makes the following assumptions:
* Atmosphere is assumed to be thin, isothermal, shell of gas
* Atmosphere is 100% transparent to solar frequencies, and
100% opaque to the lower (infrared) frequencies radiated by earth
* Earth's surface and the atmosphere both radiate as blackbodies, and
earth and atmosphere can transfer power between themselves only
by
radiation
* Earth's surface has an albedo, i.e. it reflects a fraction of high
frequency solar radiation,
but absorbs 100% of infrared radiation it receives from the atmosphere
The assumption that the atmosphere is 100% transparent to solar frequencies and 100% opaque to earth radiated infrared frequencies means that the albedo of the earth/atmosphere system is unchanged from our no-atmosphere earth. Since our simple model with atmosphere is absorbing (and reflecting) the same power as before when there was no atmosphere., our earth/atmosphere system must, when viewed from space, must appear to be at exactly the same radiative equilibrium temperature we calculated previously, 255K.
The fraction of solar energy that is absorbed by the earth's surface (1 - albedo) is blackbody reradiated at lower frequencies where the model atmosphere is assumed to be 100% opaque. So all radiation from the surface is captured (absorbed) by the atmosphere. Looking from space we see all the radiated power from the earth/atmosphere system as being radiated from the atmosphere. Hence in this simple model the idealized, isothermal atmosphere must be at 255K, the earth/atmosphere system radiative equilibrium temperature, and the same temperature that we previously calculated for the earth's surface with no atmosphere.
The model atmosphere radiates as a black body, and in general black bodies radiate equally in all directions. In our model the atmosphere is idealized as a thin, isothermal layer around earth, which means (and here is the key) it radiates half its power up (into space) and half down (back to earth). The power radiated out to space from the atmosphere must (for the earth to be in radiative equilibrium) be exactly what we calculated for our no-atmosphere earth , 240 w/m2. Because our idealized (thin, isothermal) atmosphere radiates the same power down as up, the power radiated from the atmosphere back to earth must also be 240 w/m2.
What is the temperature of our idealized, isothermal shell atmosphere?We see that adding the atmosphere has increased the power absorbed by the earth. The earth's surface receives power radiated from the sun and additional power radiated down from the atmosphere. This is the greenhouse effect. In our simple model the infrared radiation from the atmosphere down to the earth is assumed to be all absorbed by earth. Note everything is in power balance. The surface absorbs 240 w/m2 from the sun and 240 w/m2 from the atmosphere and radiates out 480 w/m2. The atmosphere absorbs all 480 w/m2 the earth radiates (and no power directly from the sun in this model) and radiates out 240 w/m2 down to earth and 240 w/m2 up to space.
Well, each atmosphere surface (top and bottom) is radiating 240 w/m2. We already calculated (using the Stefan-Boltzmann equation) that a blackbody surface radiating 240 w/m2 is at 255K. Hence our isothermal shell atmosphere is at the earth's radiative temperature (255K), and it is 48C cooler than the surface.
We find that adding a simple, idealized, atmosphere to our earth (an atmosphere that radiates half its power up and half down) causes the power absorbed and reradiated by the earth's surface to be doubled. Doubling the power the earth's surface must radiate means (using the blackbody radiation formula) that it must get hotter by the fourth root of 2. This is an increase in absolute temperature of 18.9% from 255K to 303K, since (2^1/4 x 255K = 1.189 x 255K = 303K). The idealized, isothermal shell atmosphere sits at earth's radiative balance temperature (255K), since each surface (direction) of the atmosphere is radiating 240 w/m2. Hence, our simple model has a surface greenhouse temperature rise (above radiative equilibrium) of 48C = (303K - 255K).
Summary -- simple greenhouse model
Total power absorbed by surface = 240 w/m2 (from sun) + 240 w/m2 (from
atmosphere)
= 480 w/m2 (total)
Surface temp (@ 480 w/m2) = (480/240)^1/4
x 255K
= 1.189 x 255K
= 303K (48C greenhouse rise)
Atmosphere temp (@ 240 w/m2) = (240/240)^1/4 x 255K
= 255K (earth's radiative balance temperature
and 48C cooler than surface)
How well does
our simple model match the real earth? Well, our answer is in the ballpark,
but off on the high side by 30% or so. The observed earth surface temperature
is 288K. This is rise above radiative equilibrium of 33C vs the 48C the
simple model predicts. However, by looking at details of how the earth
surface and atmosphere absorb and reflect, we see how to improve our earth/atmosphere
model.
How
does CO2 change the greenhouse effect?
The greenhouse
effect explains the temperature rise of the earth's surface above radiative
equilibrium, but predicting how temperature changes as CO2 levels change
is a whole other thing. Here the issue is figuring out (quantitatively)
how the greenhouse effect is altered for different levels of CO2. The mainstream
guys do this in three steps:
1) Forcing --- Power flow (in w/m2) into the atmosphere/earth. Zero normally taken at 280 ppm, which was (about) the CO2 level for 1,000 years prior to industrial revolution. Most forcing is considered to be man made, but changes in the sun are included. Climate sensitivities are often evaluated for a doubling of CO2 from pre-industrial times (280 ppm to 560 ppm).
Much of the (surface) radiation that can be absorbed by CO2 is already being absorbed, as a consequence the forcing vs CO2 ppm curve shows signs of flattening. The forcing of CO2 is modeled by the natural logrithmic function below (IPCC).
Forcing (w/m2) = 5.3 x ln (CO2 in ppm/280 ppm)
| CO2 (ppm) | Forcing (w/m2) | Comment |
| 140 | -3.67 | x 0.50 |
| 185 | -2.20 | x 0.66 (ice age min) |
| 280 | 0 | baseline |
| 383 | 1.66 | x1.368 (current) |
| 560 | 3.67 | x2 (target max) |
|
|
|
|
2) Sensitivity --- Linear scaling factor that translates forcing power to (final) surface temperature change (deg C/w/m2).
3) Lag --- Time required for the temperature to (fully) respond. This time is (roughly) proportional to (temperature change) x (heat capacity of ocean + crust) and inverse proportional to the forcing power.
Energy
flows of the real earth atmosphere and surface
The figure
below (Kiehl et al, 1997) is the most widely referenced (textbook) figure
showing details of earth/atmosphere energy flows. A lot of very useful
information can be teased out of this figure about the magnitude of the
greenhouse effect and how the earth's temperature is likely to respond
to changes in forcing power.
Calculating
the effective up and down radiative temperatures of the atmosphere
The real (atmosphere
+ clouds) as shown below radiates more power downward (324 w/m2) than upward
(195 w/m2), hence the bottom radiative 'surface' is hotter than the top
radiative 'surface'. Also the atmosphere radiation downward (324
w/m2) is less than the surface radiation upward (390 w/m2), so the atmosphere
is cooler than the surface (it had better be!) Troposphere temperatures
drop (approx) linearly at (about) 6.5 C/km, starting near the surface temperature
(288K) with a minimum of about 220K at 10km.
Note the atmospheric temperatures calculated below are (sort of) equivalent blackbody temperatures. The atmosphere is not a single/true blackbody, it radiates more like a weighted sum of blackbodies. While this methodology may seem suspect, the results in terms of temperature and equivalent altitudes look pretty reasonable. Here's the calculations (scaling from the earth's radiative balance baseline, 255K @ 240 w/m2):
Earth's
surface
(390/240)^1/4 x 255K = 288K (+33C greenhouse rise)
Atmosphere
bottom (324/240)^1/4 x 255K =
275K (-13C vs surface) (2 km eq)
Atmosphere
top
(195/240)^1/4 x 255K = 242K (-46C vs surface) (7
km eq)
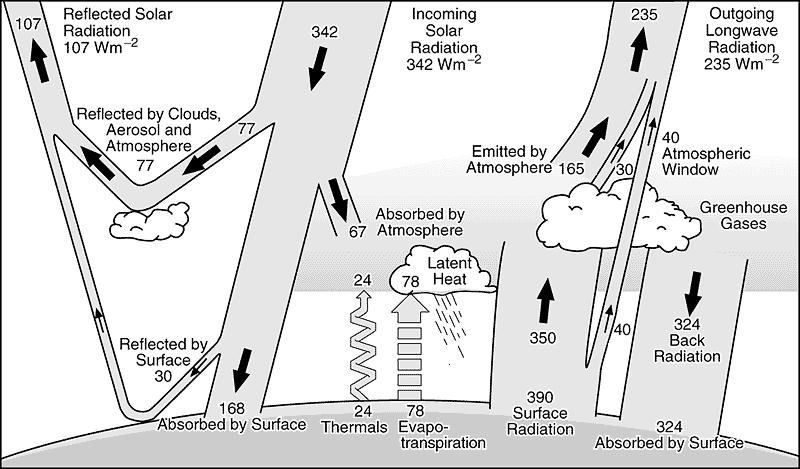
Kiehl, J. T. and Trenberth, K. E., 1997, Earth's
Annual Global Mean Energy Budget, Bull Amer Meteo Soc
(Info about this fig here:http://assets.cambridge.org/97805218/71075/excerpt/9780521871075_excerpt.pdf)
Calculating
temperature increase for doubled CO2
When
writing for the non-specialist the climate guys tend to just give the climate
sensitivity factor, which is the expected change in surface temperature
for a small change in forcing power, without any justification or derivation.
The values often quoted for a doubling of CO2 as a change of 1.2C for forcing
power of 3.7 w/m2 (about 0.33C/w/m2). The 3.7w/m2 is calculated as additional
radiative power from the surface that would be absorbed (no temperature
change) based on doubled CO2 levels widening (& slightly deepening)
the absorption notch in infrared frequencies.
Houghton in his book goes so far as to say, "This (1.2C rise for doubled CO2) is not disputed among scientists." (p 216) The 0.33C/w/2 value assumes no feedback effects, or as Houghton puts it, "nothing else changes apart from atmospheric temperature".
Even though the numbers in the Kiehl figure for the atmosphere are just averages, I have discovered that tweaking the numbers a little to reflect more CO2 allows a value for climate sensitivity to be calculated that is surprisingly close to the 'real' value, meaning the value calculated by the atmospheric scientists who take into account lots of detail about how various levels of the the atmosphere are affected.
The baseline 'official' numbers for a doubling of CO2 (280 ppm to 560 ppm) are these (Houghton says they are agreed to by all scientists):
3.7 w/m2 forcing
1.2 C rise (no feedback, i.e. no cloud changes)
Aside --- I have looked through two textbooks on atmospheric physics, including Houghton's (he is a professor of atmospheric physics at Oxford) and find a disappointingly brief treatment of how increasing CO2 affects the atmosphere and temperature. It's like one page in a 300 page book. However, I have found online a Univ of Chicago applet that allow greenhouse gases, etc, to be varied and shows absorption spectrums and power (see below).Here is my own simple analysis for the effects of a CO2 doubling using the Kiehl figure.
a) Introduction
The Kiehl
figure shows that only about 10% of surface radiation at present is able
to escape absorption by greenhouse gases, the other 90% being absorbed
by the atmosphere and reradiated up & down. CO2 in the atmosphere at
present notches out (captures) a fairly wide band of frequencies near the
peak of earth's infrared radiation. In most of this notch nearly all the
energy is already absorbed, so more CO2 in the atmosphere can only increases
absorption of surface radiation by (moderately) widening the absorption
notch (in the "wings" Houghton says). Calculating over the frequency range
the climate guys find a doubling of CO2 is expected capture 3.7w/m2 that
was previously radiated directly to space. The jargon is that a doubling
of CO2 causing a forcing power of 3.7w/m2. IPCC approx CO2 forcing
power with this equation:
CO2 forcing power (in w/m2) = 5.35 x ln (CO2 concentration in ppm/280 ppm)
= 5.35 x ln (560 ppm/280 ppm)
= 5.35 x 0.693
= 3.7 w/m2
Footnotes --- There has been a downward trend in time in the forcing number. In the first IPCC report of the early 90's the CO2 formula scaling factor was 15% higher than now. Univ of Chicago's David Archer, author of a book on global warming, and provider of spectrum applet (see below) uses the number 3.2 w/m2 (15% lower than current IPCC) for a doubling of CO2.
b) Factor in doubled CO2 forcing (3.7 w/m2)
Let's increase
the greenhouse absorption in the figure by 3.7w/m2, which reduces direct
radiation to space by 3.7 w/m2 (forcing), and calculate how much the surface
temperature must rise to compensate. Note since from the Kiehl figure the
surface is radiating 390 w/m2, a 3.7 w/m2 change is equivalent to
increasing the percent of surface radiation absorbed by the atmosphere
from about 90% to 91% and reducing the percent of surface radiation escaping
direct to space from 10% to 9%.
c) Tweaking the earth's energy budget (Kiehl figure)
Since in steady state the earth is in radiative balance with space, a reduction
in energy flow in the direct surface-to-space window radiation (due to
more CO2) must be made up by an equal increase in energy flow from the
atmosphere to space. But the atmosphere currently only radiates into space
about 37.6% (195/519) of the (av) power it absorbs. If we assume
this
ratio stays constant, then the atmosphere needs an added input of 9.84
w/m2 for it to radiate an extra 3.7 w/m2 to space. (The ratio depends on
the difference in the top/bottom radiating heights in the troposphere,
so the ratio may not stay exactly constant, but there's a good chance that
ratio changes are a second order effect.) We fill further
assume
that
the other power inputs to the atmosphere (169 w/m2) stay constant. This
is (I think) the equivalent of saying no feedbacks, meaning no increase
in evaporation or clouds.
About a third of the extra 9.84 w/m2 the atmosphere needs comes from the added 3.7 w/m2 captured by the doubled CO2, the balance comes from a surface that warms enough to radiate an additional 6.14 w/m2 = (9.84 w/m2 - 3.7 w/m2) to the atmosphere. This is an increase in surface radiated power of 1.574% (from 390 to 396.14), which since power goes as temp^4, translates to an increase in surface absolute temperature of 1/4 x 1.574% x 288K = 1.13 C.
Baseline w/m2 (now)
Tweak w/m2 (double CO2)
delta
--------------------------
------------------------------
------
surface to space
40 (10.25%)
36.3 (9.16%)
-3.7
atmos to space
195
198.7
+3.7
power to space (total)
235
235
0
increase in surf rad
---
6.14
percent incr in surf rad
---
1.574%
surface radiation
390
396.14
+6.14
surf rad absorbed by atmos 350 (89.75%)
359.84 (90.83%)
+9.84
other power to atmos
169 = (67 +24 +78)
169 = (67 +24 +78)
0
atmos power (total)
519 = (169 + 350)
528.84 = (169 + 359.84) +9.84
fract atmos to space
0.376 = (195/519)
0.376 = (198.7/528.84)
percent sur temp increase ---
0.394% = (1/4 x 1.574%)
surface temp
288K
289.13K = (1.00394 x 288K)
surface temp rise
---
1.13C (for 3.7 forcing <=> double CO2)
sensitivity factor
---
0.307 C/w/m2 = (1.13C/3.7)
This simple analysis gives a temp rise of 1.13C that is pretty damn close (within 10%) to the 'official' textbook value of 1.2C for a doubling of CO2. It also gives a sensitivity factor (0.307 C/w/m2) that is within 10% of the 'official' value of 0.33 C/w/m2. The fact that both values come out so close to the official numbers probably indicates that the official numbers come from a very similar 'perturbation analysis' of the earth's energy budget. Hence the numbers tabulated above are probably indicative of what a doubling of CO2 will do (steady state, no feedbacks) to the earth energy budget.
Improving the greenhouse
model
The best available (textbook) figure on earth/atmosphere energy flows (Kiehl
fig) looks pretty complicated with energy flows all over the place, but
if we compare it carefully with our simple (idealized) model we can identify
four ways in which our simple model has the physics a little wrong.
a) Simple model review
The simple
model with its idealized atmosphere (thin shell, isothermal, 100% transparent
to sunlight, 100% opaque to infrared) absorbs all the power radiated from
earth, radiating half to space and half back to earth. This
atmosphere doubles the power the surface must radiate, because all
the radiation to space comes from the atmosphere. The isothermal, shell
atmosphere is at the earth's radiative equilibrium temperature (255K).
For the surface to double its radiation it must rise in temperature (using
the equation for blackbody radiation) by 1.189 = 2^1/4. The increases in
surface temperature above the radiative equilibrium temperature (255K)
is 48 C = (2^1/4 -1) x 255K.
b) Strengthening terms
* The real atmosphere is quite a bit more complex than the (thin, isothermal)
atmosphere assumed in our simple model. The real atmosphere is not isothermal
and does not radiate equally up and down; it radiates 37.6% up (165 +30)/(165
+ 30 +324) and 62.4% down (see Kiehl figure), a ratio of 62.4%/37.6% =
1.66.
The reason for the unequal radiation power split is that effective atmospheric radiating layer seen by the surface is a lot warmer than the radiating layer seen by space (see murky pond analogy). Temperature in the troposphere (due to expanding, cooling gas) drops about 60C as you go up from the surface to 10 km (280K to 220K). Using the relationship that radiated power goes as the temperature of gas (Kelvin) to the fourth power we can estimate that the effective atmospheric radiating layer seen by the surface is about 33C warmer [(1 + 33K/255K)^4 <=> 1.66] than the radiating layer seen by space.
From the calculations above we see the effective lower radiating surface of the atmosphere is 13C cooler than the surface (equivalent to radiating from a height of 2km), whereas the effective upper radiating surface (which radiates to space) is 46C cooler than the surface (equivalent to radiating from a height of 7km). More downward radiation than upward radiation by the atmosphere substantially strengthens greenhouse effect. Compared to our idealized, isothermal atmosphere, about 33% more power must be radiated from the surface to get the atmosphere to radiate the required amount of power up to space.
Atmosphere split (power) correction term: 1.33 (+33% term = 0.50/.376)
c) Weakening terms
* In our simple model we assumed 100% of the power transferred from the
surface to the atmosphere is transferred via radiation, but in the real
earth about 77% is transferred via radiation and about 23% via mass. The
non-radiative heat transfer is associated with water vapor evaporated off
the surface (cools surface) and condensing in clouds (heats clouds) plus
some direct heating of air from the surface (thermals). This reduces the
greenhouse effect because the surface only has to radiate about 80% of
what we initially assumed.
Mass heat transfer (power) correction term: 0.803 (-20% term = (24 +78)/(519)
* In our simple model we assumed the atmosphere directly absorbs no power directly from the sun, but the real atmosphere absorbs 28.5% of the solar radiation absorbed by the earth/atmosphere system. This reduces the greenhouse effect because the atmosphere gets about 13% of the heat it needs to radiate to space without it having to be radiated off earth.
Direct atmosphere solar absorption power correction term: 0.871 (-13% term = 67/519)
* In our simple model we assumed that 100% of the power from the surface is absorbed by the atmosphere, that no power is directly radiated into space from the surface. But the real atmosphere only absorbs about 90% of the surface radiation allowing about 10% to leak through into space. This reduces the greenhouse effect because this component of the surface radiation is not multiplied by the atmosphere power splitting factor of 2.65 =(1/.0.376)
Leakage of surface power to space (power) correction term: 0.873 (-13% term = 1.65 x 40/519)
d) Combining our correction factors
In our simple model (with its idealized atmosphere) the radiated power
from the surface was multiplied by x2.00 leading to an increase in absolute
temp above radiative equilibrium of 48C = (2^1/4 - 1) 255K, which is about
15C higher than observed real world rise of 33C.
Applying our four correction factors we find the heat to be radiated off the surface (relative to the power radiated with no atmosphere) is (1.33 x 0.803 x 0.871 x 0.873) x 2 = (0.83) x 2 = 1.624. Our simple model overestimates the greenhouse increase (ratio) of surface radiation power by about 23% = (x2.00/x1.624), which explains why the simple model predicts a surface temperature rise (above radiative equilibrium temperature) that is 15C too high.
In our patched simple model the increase in absolute temp above radiative equilibrium is 32.9C = (1.624^1/4 - 1) 255K, very close to the observed real world rise of 33C.
Notes on molecule photon absorption
Molecules
store thermal/kinetic energy in several ways. The bonds between atoms in
molecules are somewhat like little springs. They can stretch and bend near
certain natural frequencies, and when they do they store additional (kinetic)
energy. Molecules in gases (liquids too?) are free to rotate, and this
stores additional thermal/kinetic energy.
What is important from a photon absorption perspective is that only vibration (or rotation) modes that change the dipole of the molecule (so called 'active' modes) cause the molecule to couple to photons. A molecule with a (permanent) dipole moment has some separation between its positive and negative charges, with the result that from 'some moderate distance outside' some net charge is sensed. In an active molecule the physical movement of the net charge (as seen outside), due to atoms in the molecule vibrating or the molecule rotating, causes the molecule to emit and absorb photons that correspond to the frequencies at which the charges move. Technically a dipole moment is equal to a (plus/minus charge pair) x (distance between the charges).
Molecules only absorb via vibration-rotational absorption if they have electric dipole moments. Symmetric molecules like N2 and O2 with covalent bonds that make up most of the atmosphere have no electric dipole moments and thus don't absorb infrared radiation. The reason symmetric molecules (and isolated atoms) have no dipole moment is that their electron cloud is symmetric around the nucleus. In other words the effective center of the shell of negative electron charge is at the positive nucleus. With no (average, permanent) separation between the positive and negative charges there is no dipole moment. CO2 and H2O are assymetrical and do have a dipole moment.
Width of absorption band is broadened by kinetic energy of the atoms. Gas at higher pressure has more collisions so has a wider absorption band, and effect called 'pressure broadening' Solids have atoms so close together (high pressure) that 'mutual interactions give multitudes of adjacent quantum states, so absorption and emission is essentially continuous.'
Low energy absorption (tens of um) is rotational bands. In the near infrared (7 um and less) vibrational-rotational states are excited. On the high frequency/high energy side there is the "ionization continuum". When an electron absorbs a photon and is kicked free of the molecule it carries the excess energy with it in the form of kinetic energy. Therefore above the ionization energy the absorption spectra is continuous.
Ozone absorbs energy via "disassociation energy", which is the vibrational energy getting so high the two oxygen molecules break apart. This happens with absorption of ultraviolet, leading to ozone absorbing nearly all ultraviolet.
CO2 and H2O absorption spectra
Prior to 1950
it was thought that CO2 in the atmosphere was probably absorbing very close
to 100% of the energy in the frequency bands where CO2 could absorb. The
corollary of this is more CO2 in the atmosphere would likely make no difference
as you can't absorb more than 100%.
However, when looked at closely CO2 absorption at different pressures is a mix of narrow and wide pulses spread over a wide frequency range. Prior to computers it was not possible to calculate accurately the energy absorption at all these frequencies. In the 1950's precision CO2 absorption measurements at low pressure, backed up by lengthy computations, showed that adding more CO2 really would change how the atmosphere absorbed radiation. CO2 with three atoms and four bonds has four vibration modes, three of which are active with absorption bands centered at 2.7um, 4.3 um and 15.00 um (12-17um), all of which fall within the range of infrared frequencies radiated by the earth.
Absorption/emission CO2
H20 type
2.66 um
* asymmetric
stretching
4.26 um
*
asymmetric stretching
6.3 um
* vibration-rotation
bending
14.7 um
*
bending
25+ um
* pure rotation
Earth's blackbody radiation
What's important
from CO2/global warming point of view is the CO2 absorption notches that
fall under the earth's blackbody radiation curve. 95% of the earth's blackbody
radiation energy is emitted between 2.5 um and 25 um with a peak at 10
um. The blackbody curve is only moderately peaky, with the response at
2.5 and 25 at about 20% of the peak at 10 um.
From 275K curve below most energy from surface between (100 and 1500 with peak at 550 wavenumber) (6.7um to 100um with peak at 18um).
The CO2 absorption/emission band that is by far the most important to the greenhouse effect is the one at 15 um (12-17 um). This creates the wide, deep, 50-60C notch (@ 600-800 wavenumber) that is almost at the peak of the surface radiation (see figure below).
There's a window to space right under the earth's (288K) peak of 10um (except for a narrow ozone notch in the dead center). CO2 absorbs strongly in zones on both sides of the window as does H2O. H2O also takes out everything on the lower end below 29 um in the far infrared with rotational absorption. There are also some partially transparent gaps (of CO2 and H2) in the 2.5 to 25 um region. If more CO2 (or H2O) is added to the atmosphere the deep notches widen a little and the partially transparent regions get a little less transparent.
The earth's energy budget (Kiehl figure) shows that currently about 10% of the earth radiation gets through windows (40 of 390 w/m2). Calculations show that double CO2 (280 ppm to 560 ppm) has a forcing of 3.7 w/m2 [= 5.35 x ln(560/280)] equivalent to about 10% of the window power. Since current CO2 concentration of 380 ppm (relative to pre-industrial 280 ppm) has a forcing of 1.6 w/m2 [= 5.35 x ln(380/280)], increasing CO2 to 560 ppm will only close the radiation window to space from current levels by a small amount, about 5% (= 2.1 w/m2/40 w/m2). (Note the original IPCC 1990 forcing formula was 6.3 x ln(C/Co), which is 18% higher, which gives a feeling for the uncertainty level.)
Proposal to reduce sunlight
A Scientific
American article (Oct 2008) discusses a recent proposal by Roger Angel
at Univ of Arizona, who is famous in astronomical circle for hugely improved
methods of making large telescope mirrors, for a solar shield placed at
the L1 Lagrangian point between the earth and sun. The idea is a 1.8% reduction
in solar power hitting earth could cancel all the temperature rise due
to a doubling of CO2. The value of 1.8% comes from a computer study done
in 2000 by Lawrence Livermore scientists Bala Govindasamy and Ken Caldeira.
My first thought was 1.8% seemed way too big. 1.8% in power terms is [24.7 w/m2 = 1.8% x 1,370 w/m2], which is far higher than the usual forcing numbers. But the key here is that the power the earth radiates into space is proportional to T^4 (4th power of absolute temperature). So a 1.8% reduction of input solar power means a reduction of the current earth temperature of 288K (including greenhouse effect multiplier) by [0.45% = 1.8%/4]. This translates into a temperature decrease of [1.3C = 0.45% x 288K], which is the ballpark for the expected temp rise due to a doubling of CO2 (280 ppm to 560 ppm).
Earth's infrared radiation spectrum
The figures
below show how the earth + atmosphere radiates to space. In our idealized
greenhouse models the atmosphere was assumed to radiate as a single/true
black body, but the figures below shows this is not really true. The atmosphere
radiates more like a weighted sum of blackbodies with temperatures ranging
from (about) 220K to 280K, which is the temperature range of the troposphere.
The first figure is (supposedly) real data from a satellite (superimposed on a set of blackbody curves). The following three figures are from a Univ of Chicago computer model that, as you can see, matches the data very well. The computer model allows the frequencies and power absorbed by the various greenhouse gases to be teased apart. In the first model figure all the greenhouse gases are shown. In the 2nd figure water vapor is removed (showing the absorption notches of CO2, ozone, and methane clearly). The 3rd model figure shows the absorption of water vapor only (no clouds). Note, all these infrared radiation curves are for particular weather conditions and latitudes, not universal earth averages.
I generated the model curves from on online radiation model of David Archer of Univ of Chicago who is author of a book on Global Warming. I set its Locality setting to 'subarctic summer' because it gave a surface temp close to the average earth temp of 288K. Weather was set to default value of 'no clouds', and CO2 at default value of 375 ppm (current value). This model can be used to look at how the CO2 notch area changes as CO2 concentration changes. Since the CO2 notch area is currently about 30 w/m2, I expected it should change about 10% when I compared 280 ppm and 560 ppm, since the (standard) forcing for CO2 doubling is 3.7 w/m2. (This model watt total in the upper right hand corner, however, shows 3.2 w/m2 for CO2 doubling.) However, the area under the CO2 notch changed a lot less than 10% for CO2 doubling. I would guess more like 3% or 1 w/m2!
Here is a link to Archer's book and the Univ of Chicago radiation model:
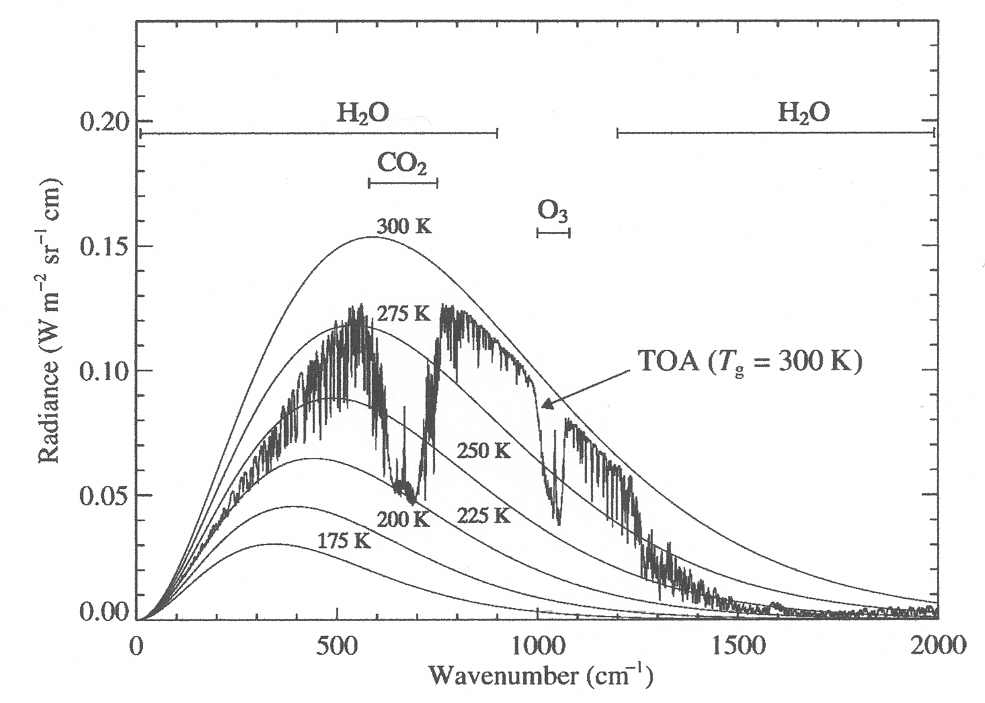
Measured upwelling infrared radiance at 60 km for
a clear sky, mid latitude, summer atmosphere (TOA = Top of Atmosphere)
(wavelength in microns = (10,000/wavenumber in cm^-1), CO2 notch
<=> 15 micron, O3 notch <=> 9.4 micron)
(from the book, "Radiation in the Atmosphere, A Course
in Theoretical Meterology)
Univ of Chicago model spectrum above shows the radation
absorption of all the greenhouse gases
As a reasonableness check I estimate the area under the 288K curve, which the model follows sans all greenhouse gases, (using a triangle) and find it to be 336 w/m2. OK, this is about 14% less than the exact value of (288/255)^4 x 240 w/m2 = 390 w/m2. (This was a useful check, because when I did this check on a version of this figure from David Archer's book, I found the scaling of the vertical axis off by a factor of 2.)
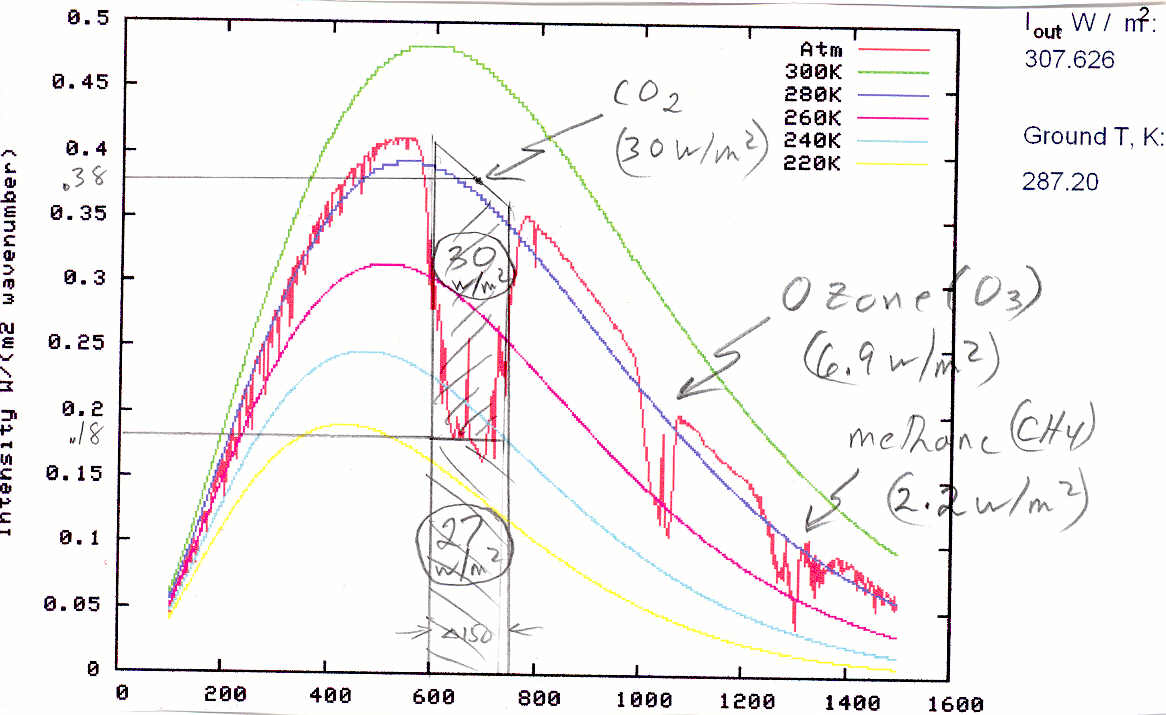
Univ of
Chicago model spectrum above shows the radation absorption of CO2, O3,
Ch4, but not water vapor
When water vapor is excluded, the frequency notches causes by the other three major greenhouse gases standout clearly. In isolation the (net) absorption of CO2 is 30 W/m2, ozone is 6.9 w/m2 and methane is 2.2 w/m2.
Note, what CO2 really does (in isolation) is absorb all the 57 W/m2 the surface attempts to radiate to space in the narrow frequency range 600-750 wavenumber, and replace it in the output spectrum to space with a much smaller value of 27 w/m2. The radiated power to space at these frequencies is lower than the surface radiation because the CO2 radiating to space is near the top of the troposphere at a temperature of 220K to 230K, and gas radiations fall on a blackbody curve that corresponds to the temperature of the gas.
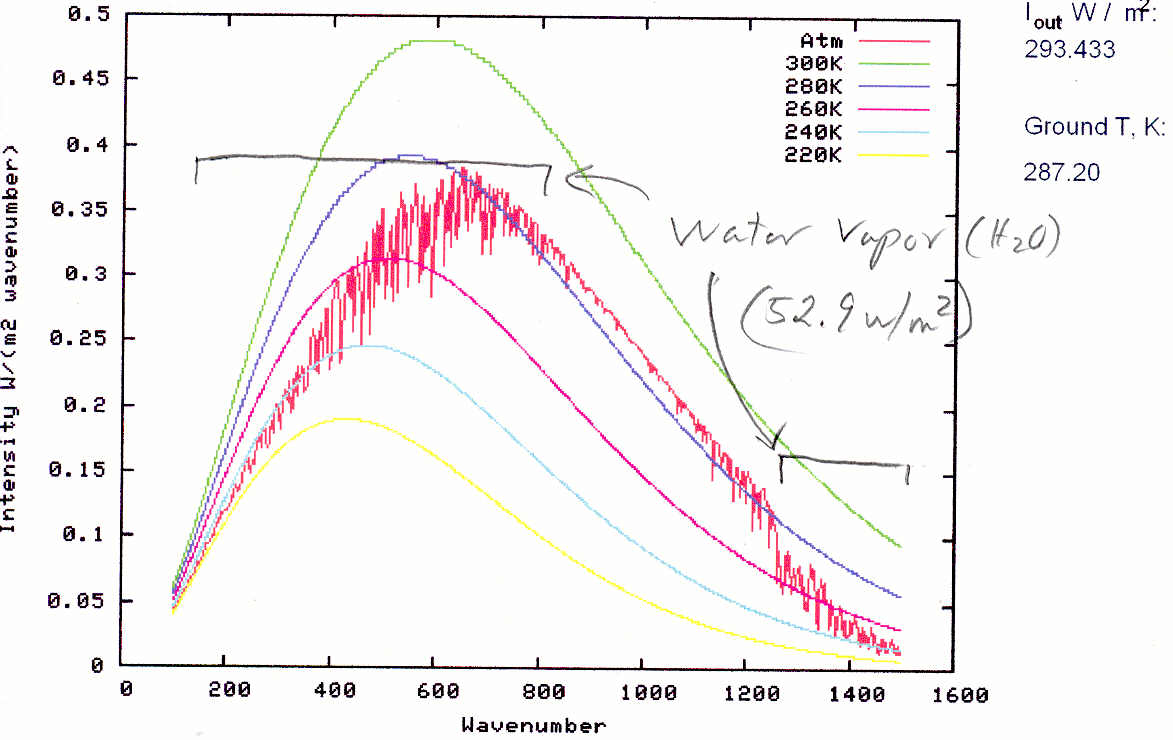
Univ of
Chicago model spectrum above shows the radation absorption of water vapor
only (no clouds)
Water vapor has an isolated absorption of 52.9 w/m2, nearly double that of CO2. As you can see comparing the two curves above, the ozone and water vapor absorption frequencies basically don't overlap, CO2 and water vapor overlap a little bit, and methane and water vapor overlap strongly.
Atmospheric window only passes 10% of surface radiation?
Note the window
width in both the satellite and Univ of Chicago model spectrums above does
not seem consistent with the Kiehl energy budget figure. Kiehl says that
only about 10% of surface radiation (40 w/m2 of 390 w/m2) makes it through
the infrared window in the atmosphere to space. But the window-to-space
above looks quite wide, from about 750 to 1250 wavenumber, with an area
underneath of 85 w/m2, or about 20-25% of the total radiated power. This
is about double what it should be (on average) according to Kiehl. What
is going on here? Is this a cloud effect? (more playing with the radiation
model might clear this up)
Solar spectrum on earth's surface
Here's an
interesting figure showing the sun' power spectrum and how it's modified
at the earth's surface by absorption regions in the atmosphere. This spectrum
is important to those designing solar power collectors. Eyeballing this
figure it appears that about half the sun's power (area under red curve)
is just below the visible in the near infrared (800 to 2500 nm).
Note that incoming solar power spectrum peaks around 500 nm, whereas the curves above show the outgoing (upwelling radiation from the earth's surface) spectrum peaks about wavenumber 550 cm^-1 (550 cycles in one cm) for a wavelength of 18 microns. 18 microns is in the lowest infrared region (IR-C) which runs from 3 to 1,000 microns. Thus outgoing radiation from earth peaks at a frequency about 36 times lower than incoming solar radiation.
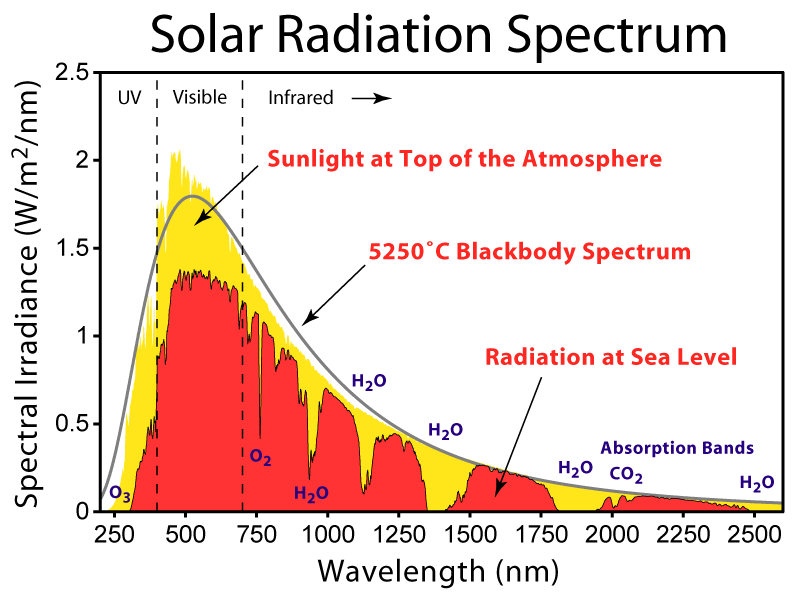
(Credit Wikipedia -- Solar Irradiation)
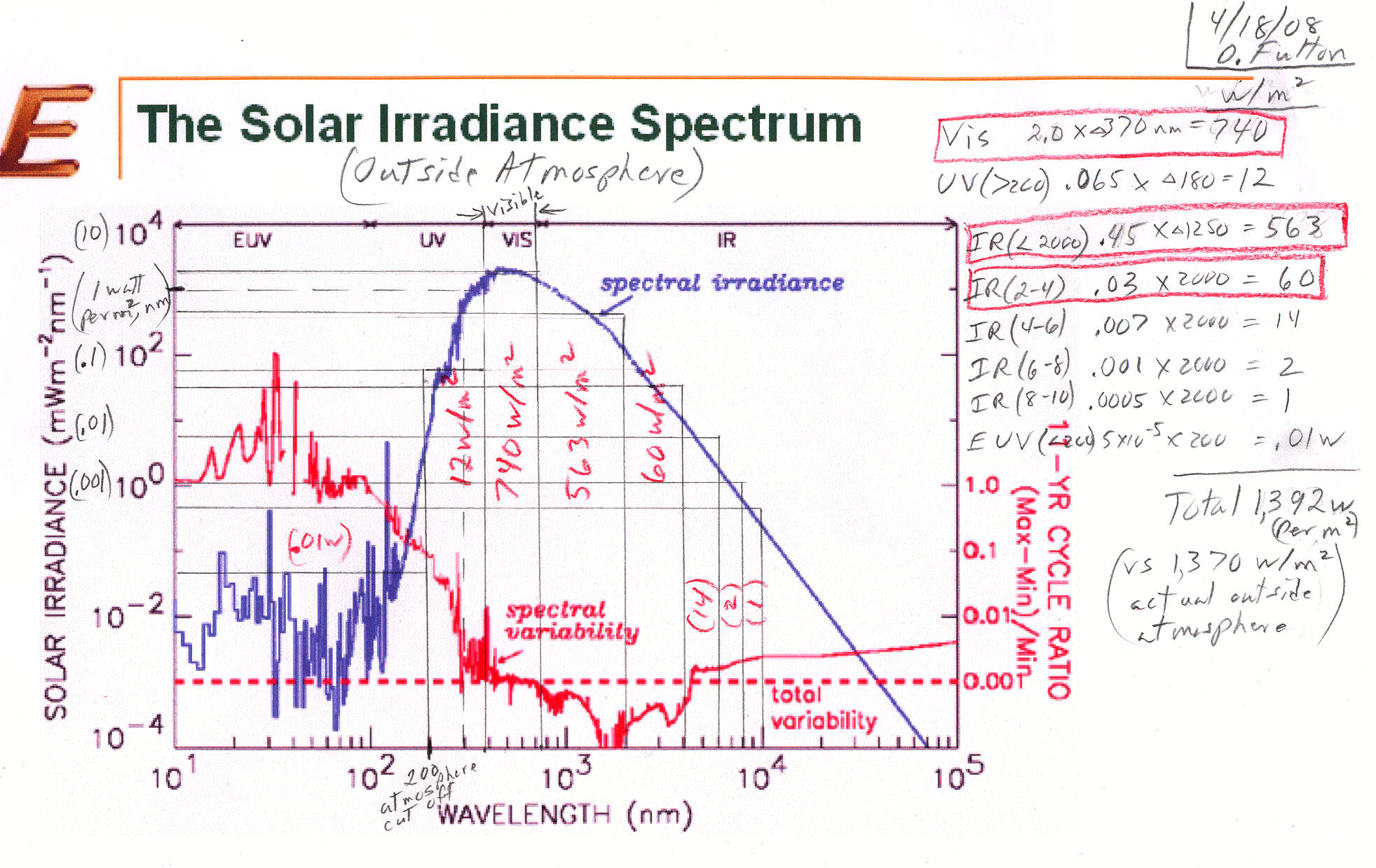
Where in the spectrum is the sun's power?
A solar irradiance
curve, like the one above, is technically a power spectral density curve.
If you want to know how the sun's power is divided among the visible, UV
& IR you need to find areas under the curve since (w/m^2/nm x
nm = w/m^2). Eyeballing the curve above it looks like the reduction of
27% from 1,370 watt/m^2 total, outside the atmosphere, to (approx) 1,000
watt/m^2 on the earth's surface occurs all across the spectrum.
I found another solar irradiance curve (below) that was plotted logarithmically and covered most of the spectrum. Printing it out and drawing a few rectangles on it with pencil and ruler allowed me to estimate (with maybe 10-20% accuracy) where in the spectrum the sun's power is located. The results are interesting. In round numbers I find (outside the atmosphere)
Visible
55% 740 watt/m^2
Infrared
44% 640 watt/m^2
Ultraviolet 1%
12 watt/m^2
For the designers of solar energy systems the key result is that about 94% of the sun's power is in the visible octave (380 nm to 750 nm) and the octave and a half of infrared just below the visible (750 nm to 1,800 nm).
Murky pond analogy
Suppose you
are outside the earth and could see the infrared radiation the earth radiates.
(After all, infrared radiation is electromagnetic radiation like light,
only at a lower frequency.) Where do you see the radiation coming from,
the surface or the atmosphere?
Well, a good analogy is looking from a pier down into a shallow, murky pond. If the water is pretty clear, much of the light you see is reflected from the bottom of the pond. You see the bottom clearly. If the water is a fairly murky, maybe you can see into the water a few feet and barely see the bottom. If the water is really murky, you might only be able to see into the water a few inches, the bottom invisible.
At present 40 w/m2 of 240 w/m2 (total), or 1/6th, of the earth's radiation is coming off the surface and the rest from the atmosphere, so in our analogy CO2 in the atmosphere makes the atmosphere 'look' fairly murky in the infrared. In our pond as the concentration of murk increases the distance that light can penetrate is reduced. From outside you see the light reflected (up) from closer to the surface. From the bottom of the pond you see light reflected (down) from lower down in the water.
What this analogy makes clear is that more CO2 in the atmosphere causes the 'effective' height of infrared radiation (to space) to increase, and the 'effective' height of radiation (back to the surface) to decrease. These heights are in the troposphere, the lower 10 km of the atmosphere, which has an (approx) linear temperature gradient from 288K to about 220K at its top (10 km). So adding CO2 in the atmosphere means radiation to space will originate from a higher and colder level in the atmosphere. Similarly, the radiation from the atmosphere back to earth will originate from a lower and warmer level in the atmosphere.
If the effective atmospheric level radiating to space is colder, meaning less than 255K (radiative equilibrium temperature), then less energy is radiated to space. So over time the surface warms and pushing up all the temperatures of the (approx) linear troposphere thermal gradient until the effective level radiating to space is again at 255K While this restores the power radiated to space to equilibrium levels, the power radiated back to earth is higher because it come from a lower and warmer region of the atmosphere.
Bottom line --- more CO2 in the atmosphere causes a larger fraction of surface radiated power to be reradiated back to the surface, because it comes from lower and warmer region of the atmosphere, and a smaller fraction of surface radiated power to make it directly to space. The result is a stronger greenhouse effect. The surface must get warmer and radiate more to bring the power radiated to space up to the earth's radiative equilibrium power of 240 w/m2.
Notes
on radiative modeling the troposphere
Is there a
simple way to model the (real) earth's atmosphere from a radiative viewpoint?
I think there might be. From my reading about the atmosphere I have found
some simple and suggestive relationships between the temperature and density
of the lower atmosphere (troposphere) and the radiative equilibrium temperature.
The temperature of the troposphere (lowest 10 km) is modeled as falling linearly with height from 288K at the surface (earth's surface temp) to (about) 220 K at 10km. Temp then stays (approx) constant at 220K from 10 km to 20 km (into the lower stratosphere). The average of these two temperatures is 254K = (220K +288K)/2, which is very close to the radiative equilibrium temperature of the earth (255K). This is unlikely to be a coincidence.
Pressure (and mass) in the atmosphere fall (approx) exponentially with height. Wikipedia gives 5.6 km as the height where the pressure has dropped to 50%, meaning that 50% of the mass of the atmosphere is below 5.6 km and 50% above. Since 20 km is roughly x3 higher than 5.6 km, by assuming an exponential fall in pressure and mass with height, we can calculate that nearly all the mass (97% to 99%) of the atmosphere is below 20 km. Since it takes mass to radiate, from a radiative viewpoint we probably can ignore all air above 20 km.
Houghton's book on global warming says, "average height from which thermal radiation leaving the atmosphere originates is (about) 6 km". Above I calculated, the effective upper radiation level of the atmosphere is about 7 km. Another coincidence, I think not?
Put this all together and we have (sort of) a 'magic' height of 5 to 7 km. The atmosphere at this height is at its 50% point in gas pressure (& mass), and its temperature is at the radiative equilibrium temperature (255K) that the earth presents to outer space.
Baseline numbers
There is a lot of disagreement about many aspects of global warming, but
some values seem to be generally accepted (if not exactly, then close)
by most researchers, as such they form a set of baseline numbers.
(Some change in these numbers over time is, of course, to be expected.)
Solar flux at earth's distance
1,370 w/m2 (solar constant)
Earth/atmosphere albedo
0.30
Energy absorbed (@ albedo = 0.3)
240 w/m2 (235 to 240)
Earth's black body temp (@ albedo =0.3)
255K (-18C)
Earth current temperature
288K (15C)
Greenhouse effect rise (288K - 255K)
33C
Earth's (av) temp rise (from 1860)
0.6C
Expected rise for doubled CO2 (no feedbacks) 1.2C
(280 ppm to 560 ppm, steady state rise)
Expected rise for doubled CO2 (w/feedbacks) 2 x 1.2C
= 2.5C (IPCC guessimate)
Three
different ways to figure global warming
There are
three
totally different ways to calculate global warming. People feed the data
into different models, all of which (at least initially to me) appear plausible.
The difference between these models is not a matter of a second order effects
or feedback mechanisms. These models are funamendally different, and critically,
they give wildly different predictions: basically no problem, moderate
problem, and life on earth is doomed!!
Hence a key aspect of the global warming 'debate' is verify the right model is being used. Here's a list of the three models.
1) Mainstream method --- Hansen & IPCC
2) Alternative view #1 (no problem) --- temp rise linear with greenhouse
power
3) Alternative view #2 (huge problem) --- temp rise linear with CO2 ppm
?? 4) Alternative view #3 -- temp rise caused by
fuel energy release
Note, in my reading so far I have found zero support among climate scientics for any of the 'Alternative views'. So while these may just be the views of cranks (or the uninformed), where and how they have their physics wrong (if they do!) is I think worth discussing.
Mainstream method
--- Hansen & IPCC
This method
is based on work by Hansen & IPCC (Intergovernmental Panel on Climate
Change). Hansen analysis (seems to be) based mainly on ice age data. Hansen
has studied the variations in CO2 and temperature over several ice age
cycles. He believes at this time the earth (averaging over several ice
age cycles) was in radiative balance. This is his baseline.
He then tries to figure out what fraction of the ice age temperature variation was due to CO2 and what fraction to other causes. He assigns only about 1/3 to 1/2 of the ice age temperature variation to CO2, and he derives ice age forcing power caused by CO2. Based on this analysis he concludes that at present CO2 levels the earth is now not in radiative balance. His latest value is that each square meter of the earth is (continuously) absorbing 0.85 w/m2 more power than it radiates. His sensitivity factor is 0.75 C/w/m2 that he derives from a 5C ice age variation that he attributes to a 6.5 watt forcing (5C /6.5 w/m2 = 0.75 C/w/m2).
This method is predicting temperature increases that are higher than is now now measured. For example 3.7 w/m2 x 0.75 C/w/m2 = 2.78C predicted final temperature change for 'doubling of CO2. So for the calculations of this method to be brought into agreement with measured values, the excess power must be going somewhere. There is only really one possible energy sink, this is the earth (sea and/or crust). Hansen in his Scientific American article says the sea is absorbing much of this CO2 excess power and can do so for about a century, thus delaying the final temperature change until then.
Alternative view
#1 (no problem) --- temp rise linear with greenhouse power
This method
focuses on the proportional increase in greenhouse power caused
by higher levels of CO2 and assume that the greenhouse effect temperature
rise will increase by this same proportion. For example, if 'doubling
of CO2' (an increase from pre-inductrial levels of 280 ppm to 560 ppm)
causes a power increase (to the surface of) 3.7 w/m2, then this is
a 1.14% increase in greenhouse power (power radiated to the surface from
the atmosphere), so the expected final temperature rise from this
additional 3.7 w/m2 is 1.14% x 33C greenhouse rise = 0.38C. This is a sensitivity
factor of 0.38C/3.7 w/m2 = 0.1 C/w/m2. This is 7.5 times lower than Hansen's
figure!
This method seems eminently reasonable to me. It's virtue is that its a simple scaling, based on the physics, and it's not necessary to know the details of the many complex and poorly understood feedback mechanisms. It's only necessary that they continue to work about as they do now.
This method predicts that final CO2 temperature rises are quite small. A real plus for this method is that its predictions match up well with what is measured! CO2 levels have increased 39% from pre-industrial times (280 ppm to 390 ppm), but the measured temperature increase is about 0.6C. This agreement with observed temperature is achieved without need for assuming that the earth is acting as a big heat sink.
Alternative
view #2 (huge problem) --- temp rise linear with CO2 ppm
This method echews complex physics. It simply notes that empirically (by
observation) there appears to be a simple, nearly linear, relationship
between CO2 levels and temperature rise. The pre-industrial 280 ppm of
CO2 caused a greenhouse rise of 33C. If this relationship was linear it
is equivalent to an an 11.8C rise per 100 ppm of CO2. What is very
interesting in that during the ice age when CO2 and temperature cycled
up and down the variation was just about 10 C variation for 100 ppm change
in CO2.
So this theory just says, look don't sweat the details of the physics, the data shows that CO2 final temperatures are pretty much linear with CO2 levels. It could be just a coincidence that the ice age scale factors is very close to the total scale factor. But it might also be that this method is giving a good prediction. (I have only found one site that uses this method). So unless there is a strong physics case against it, I would consider this method as viable.
This method is predicting that life on earth is (basically) doomed!! A doubling of CO2 levels from pre-industrial times increases the greenhouse rise from 33C to 66C. This is an extra 33C that will cook the planet. Average temperature will rise from 59F to 118F. Almost no plants can survive this. The only hope here would be global engineering on a massive scale to pull the CO2 out of the atmosphere.
Note this method requires that the earth (land and/or sea) now be absorbing huge amounts of power, several times what Hansen thinks. This is because the final temperature rise caused by today's CO2 level of 390 ppm CO2 (39% above the pre-industrial level of 280 ppm) is 39% x 33C = 13C, whereas only 0.6C is observed to date. The author of the site that discusses this thinks the earth's crust is absorbing the power and could do so for about a century. If this view is right, significant and measurable temperature rises in land and/or sea temperatures should now be visible. What does this data show?
(Thhis is also view of Tom Goreau, see 'reasons to really worry', below)
Alternatvie view
#3?
Aubrey Banner
argues (& calculates) that it is the energy released by burning
from fossil (& nuclear) fuels alone that are the cause of global
warming. CO2 is just an unimportnat byproduct. He also calculates the energy
that has been absorbed by the estimated melting of sea and artic ice and
gets a number comperable to enegy released. Why does no one else talk about
ice melt as a sink? His says primary energy is 4.4 x 10^20
joules/yr.
Below I convert his primary energy number (not checked) to w/m2. The result is a very small value of 0.028 w/m2. This is not totally neglible, but it is only 3% of Hansen 0.85w/m2 forcing.
{(4.4 x 10^20 joules/yr)/(3.16 x 10^7 sec/yr)} /5.09 x 10^14 m2
= 1.4 x 10^13 w/5.09 x 10^14 m2
= 0.028 w/m2
http://lofi.forum.physorg.com/Melting-the-World&%2339%3Bs-Ice_13054.html.
NASA on atmospheric
temperature
Interesting
---- Below is a somewhat skeptic global warming site from NASA. They defend
the accuracy of their satellite atmosphere temperature models (satellites
agree within 0.02C and agree well with ballon measurements).
http://science.nasa.gov/NEWHOME/headlines/essd06oct97_1.htm
The key point they make is that in the last 20 years the (av) temperature of the troposphere (lowest 10 km) has gone down slightly. Yet in all models the low troposphere is assumed to be well coupled in temperature with the surface (generally modeled as declining linearly from 288K to about 220K at 10km). (Whoops -- site is dated 1997, but a linked site for satellite data shows maybe a tiny (0.05C) rise total in 20 years (1982 to 2002)
Does the sun control earth's climate?
It had long
been assumed (quite reasonably) that the only way the sun could (or was
likely to) affect the earth's climate was by variations in the sun total
energy output. The variation in the sun's energy output has been accurately
measured by satellite for about the last 20 years. While some variation
has been seen over this time, its quite small, about 0.1% (eq to 1% x 240
w/m2 = 0.24 w/m2 forcing) basically following the 11 year sunspot cycle
with no long term trend up or down. Since 0.24 w/m2 is a small compared
to calculated CO2 forcing levels, the IPCC starting in 1990 has tended
to dismiss solar variations as having any major impact on earth's climate.
However, it was known there were one or two other ways that the sun might have some influence on earth's climate. One was by variations in ultraviolet energy (which are absorbed in the stratosphere), and the other was by an indirect mechanism where the sun's solar wind affects cosmic rays hitting the earth.
Svensmark is a lonely voice
In 1996 Danish
scientist Svensmark showed there was a remarkable correlation between earth's
temperature and the intensity of (intergalactic) cosmic rays hitting the
earth over the last few hundred years. While this was tantalizing, at the
time there was little understanding of how cosmic rays could affect earth's
climate, so most scientists paid little attention was paid to Svensmark's
paper. Svensmark struggled to get funding, but continued to work on the
problem.
No doubt the lack of attention to the sun/climate link was partly due to political reasons related to global warming. One prominant scientist called Svensmark's work "dangerous", meaning, of course, politically dangerous because it could potentially undermine the (supposed) scientific consensus that excess CO2 from fossil fuel burning is causing global warming.
A new view of the sun/climate connection
In recent
years the relatively small group of scientist working on the on the sun/climate
connection has made big progress. They have assembled an increasingly convincing
case that the sun may very well be substantially influencing the earth's
climate and temperature.
First, new work has shown that historical earth temperatures measured several different ways show tight correlation with with solar activity.
Second, how
the sun can affect earth's climate through modulation of cosmic rays has
come to be much better understood. The level of cosmic rays hitting earth
have been shown to vary quite a bit (10%) as the intensity level of the
sun's solar wind changes. More solar activity means a stronger solar wind,
and a stronger solar wind shields the earth from galactic comic rays more
effectively. Newly available data from satellites shows more cosmic
rays correlate closely with more low attitude (< 3 km) cloud cover.
(This would make sense if cosmic rays were in some way seeding the clouds.)
An increase in low attitude clouds is known to cause a cooling effect by
increasing the earth's albedo (overwhelming increases in the greenhouse
effect). Summing up:
More solar activity => stronger solar wind => less cosmic rays hit earth
=>
less (low) clouds => lower albedo => warmer earth
Less solar activity => weaker solar wind => more cosmic rays hit earth
=>
more (low) clouds => higher albedo => cooler earth
WhoopsThird experiments are now under way to understand how cosmic rays might affect clouds. This includes a major experiment planned at CERN (called CLOUD) and 'basement' experiment completed by Svensmark (called SKY). The cosmic ray/cloud relationship has gone big time with CERN involved. CLOUD is a new, large, flexible cloud chamber to be built at CERN where the effect of particle beams (simulating cosmic rays) on cloud formation can be studied. This is viewed as multi-year series of experiments. The CERN CLOUD proposal says,
Wikipedia (Sun) under 'solar anomolies' saysSun's magnetic field is at less than half strength compared to the minimum of 22 years ago. The entire heliosphere, which fills the Solar System, has shrunk as a result, resulting in an increase in the level of cosmic radiation striking the Earth and its atmosphere. [Translation: via the Svensmark effect this should result in more (low) clouds, higher albedo, and earth cooling (not warming).]
" The primary task of CLOUD is to investigate how galactic cosmic rays may influence cloud formation."Water droplets in clouds form around tiny dust particles or aerosols (liquid droplets). The more dust or aerosols in the air the more clouds and the smaller the droplets and the brighter the clouds. Over the oceans where dust is rare cloud formation is strongly dependent on the amount of sulfuric acid aerosols in the air. A recent 'basement' experiment (by Svensmark) shows that ionization of the air caused by (real) cosmic rays strongly affects the formation (by clumping) of sulfuric acid aerosols around which cloud water droplets form.
Here's a link to a 2006 CERN press relese on CLOUD. The first results are expected in summer 2007.
http://press.web.cern.ch/press/PressReleases/Releases2006/PR14.06E.html
In summary the primary way the sun affects the earth's climate is thought to be this:
The sun's magnetic activity modulates the amount of galactic cosmic rays hitting earth (via solar wind), which in turn affects the amount of potential cloud forming sulfuric aerosols in the air (via ionization of the air), which in turn affects the amount of low attitude cloud cover on earth (especially over the ocean), which in turn affects the earth's albedo, which in turn affects the earth's temperature.So while it's still a minority position, with the new experimental, historical, and theoretical evidence rapidly piling up, quite a few scientists are coming to believe that the sun, not CO2, may very well be the dominant force in the control of earth's climate.
Correlation of clouds with cosmic rays
Measurement
of the extent of earth's cloud cover can only reliably be done from space,
so no good cloud data exists before about 1980. Here's the variation in
low attitude cloud coverage (from satellite data) and cosmic ray intensity
(measured at ground level) since 1984. During this time interval cosmic
ray intensity has varied about 10% and cloud coverage about 2%. Clearly
the correlation is remarkably good.
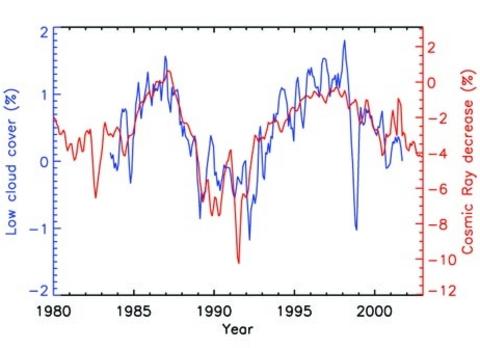
"The correlation between cosmic ray flux (red) as
measured in Neutron count monitors in low magnetic latitudes, and the low
altitude cloud cover (blue) using ISCCP satellite data set, following Marsh
& Svensmark, 2000 (used with permission)" as included in Cosmic Rays
and Climate by Nir J. Shaviv, PhysicaPlus, 2005, online Israel physics
journal).
Correlation of temperature with solar activity
Here's a figure
showing variations in oxygen O18/O16 ratios (from stalagmites in a cave)
and C14 levels (from tree rings) on a hundred year time scale for three
thousand years (from 6,000 to 9,000 years ago). O18/O16 ratio is the most
common proxy for temperature and C14 for solar activity. The interpretation
of this figure is that it is a plot of (Indian) ocean temperature (used
to form the stalagmites) vs solar activity. Clearly it shows a remarkably
good correlation.
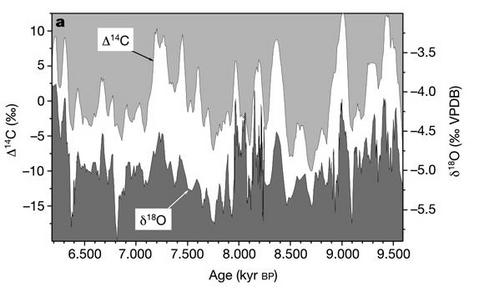
"The correlation between solar activity, as mirrored
in the 14C flux and a climate sensitivity variable, the 18O/16O isotope
ratio from stalagmites in a cave in Oman, on a centennial to millennial
time scale. The 14C is reconstructed from tree rings. It is a proxy of
solar activity since a more active sun has a stronger solar wind, which
reduces the flux of cosmic rays reaching the Earth from outside the solar
system. A reduced cosmic ray flux will in turn reduce the spallation of
nitrogen and oxygen, and with it the formation of 14C. On the other hand,
the 18O/16O ratio reflects the temperature of the Indian ocean, the source
of the water that formed the stalagmites. (Graph from Neff et al., 2001,
copywrite by Nature, used with permission", as included in Cosmic Rays
and Climate by Nir J. Shaviv, PhysicaPlus, 2005, online Israel physics
journal)
Sun/climate references
A good few
page overview of the the sun/climate theory and recent work written for
the general public is in an online Israeli physics journal, by the Israeli
scientist Shaviv, at the link below. The two figures above are from this
link. Shiviv has also done original work showing that the relationship
between earth's temperature and cosmic rays (measured from meteorite surfaces)
may very well extend back 500 million years (more than 10% the lifetime
of the earth).
http://physicaplus.org.il/zope/home/en/1105389911/1113511992_en/
A highly recommended reference on the sun/climate connection is a new book by Svensmark & Calder:
The Chilling Stars: The New Theory of Climate Change, by Svensmark & Calder, 2007
I have nearly finished reading this very well written book, written apparently mostly by Calder who is a writer. At the end it contains a more speculative, but very interesting section, about how the major climate variations over billions of years (snowball earth and no ice for a billion years) may be explainable by the earth passing in and out of arms of the galaxy as it rotates about the galactic center. Cosmic rays in arms would be a lot higher than between arms, since in arms active star formation produces a lot of short lived stars and supernova that are the primary source of cosmic rays. It turns out the galaxy arms and earth/sun don't rotate as the same rate about the center of the galaxy. Who knew? Good stuff! I just checked the books Amazon customer ratings, and it's five stars, based on 12 customer reviews.
Sun -- miscellaneous
As the earth
travels on its elliptical orbit around the sun, the earth-to-sun distance
varies about 3.74%. Solar intensity varies as the square of distance, so
it varies 7.6% (max to min) during the year. Presently the earth-to-sun
distance is minimum (and solar energy maximum) in Jan. Albedo of earth
also varies during the year ranging between .30 to .32. When albedo and
solar variations are combined, earth's absorbed solar power varies between
232 to 246 w/m2 during the year. In the northern hemisphere energy is 10
w/m2 higher than av in winter and 10 w/m2 lower in summer. Hence our present
solar cycle tends to reduce winter/summer temperature difference in the
northern hemisphere. (Southern hemisphere summer/winter differences
are moderated by all the ocean water in the southern hemisphere.)
Historical carbon 14 levels provide a measure of solar activity. "Stuiver and others confirmed the connection between solar activity and carbon-14, and this became a standard tool in later solar-climate studies."
The John-Daly site (below) hosts dozens of outside weird science paper (many focusing on the sun), some well written, some not. One of the most interesting papers there, saying the sun is responsible for most of earth's climate variations, is by Dr Theodor Landscheidt. He is an independent researcher, but he appears to have published a lot of peer reviewed papers and good credentials.
http://www.john-daly.com/solar/solar.htm
How much energy are
the oceans absorbing?
The
consensus of climate people is that the oceans are the dominant sink for
any earth (non-equilibrium) forcing power. Clearly changes in thermal energy
stored in the ocean are very important to quantifying global warming. Ocean
temperature changes (in principle) can be used to measure earth's forcing
power, and (via forcing power and climate sensitivity) to estimate the
future temperature changes already in the 'pipeline'.
A co-author with Hansen has a global warming site (link below). He shows a plot of ocean heat vs time (1993 to 2003) that is pretty much a straight line with a slope indicating power absorption of 0.6 w/m2, in the ballpark of Hansen's' most recent forcing value of 0.85 w/m2.. He also comments that measured ocean temp data is of high quality.
http://www.realclimate.org/index.php/archives/2005/05/planetary-energy-imbalance/
However, a very different view of ocean energy/temperature is a recent paper by Lyman et al. This appears to be a major paper in a major journal reporting on huge numbers of ocean measurements (Lyman et al, Recent Cooling of the Upper Ocean, Geophysical Research Letters, May 2006). Firstly, the data (over 13 years) shows a lot of variability. Secondly, av warming rate is fairly low, equivalent to 0.33 w/m2. Most interesting of all is that a few months after the paper was published (May 2006) it was withdrawn when it was found that (both!) of the new temperature sensors (deployed in 2003) were in error. You would think this would be an area of research that would be adequately funded, but based on recent evidence that does not appear to be the case.
So there, you have it. One set of researchers saying measured ocean temperature rise nicely confirms the climate models, with others saying whoa... An inherent problem is that there is a huge amount of 'noise' in ocean temperature measurements. Weather and circulation changes, like El Nino, make huge temperature variations (many degrees) compared to the tiny changes (hundred's of a degree/yr) expected from global warming. At this point I don't know what to believe about ocean temperature data.
Reasons to be skeptical?
Did CO2 lag temperature changes in ice ages?
This high resolution ice age ice core data has three slope changes marked
that look pretty convincing that CO2 lags temperature change. All
three delays are about the same time and its a reasonable value if the
oceans are responsible.
Excellent historical review site (http://www.aip.org/history/climate/co2.htm) says this, "Most of the evidence pointed to a slight lag (in CO2 concentration following temperature) of a few centuries. It seemed that rises or falls in carbon dioxide levels had not initiated the glacial cycles." However, this is still consistent with CO2 causing (most) of the ice age temperature change. CO2 is viewed as an amplifier.
Ice age data also yield a value for climate sensitivity factor. Where does the forcing power come from? I think it is calculated from CO2 levels. Since forcing is logarithmic, 140 ppm to 280 ppm is the same forcing as 280 ppm to 560 ppm (3.7 w/m2).
Figure below is from this site
http://motls.blogspot.com/2006/07/carbon-dioxide-and-temperatures-ice.html
The supposed
mechansim for the drop in CO2 is absorption by the oceans since cold water
holds more gas (fizz) than warm water. 2003 Science paper on CO2
lag
http://icebubbles.ucsd.edu/Publications/CaillonTermIII.pdf
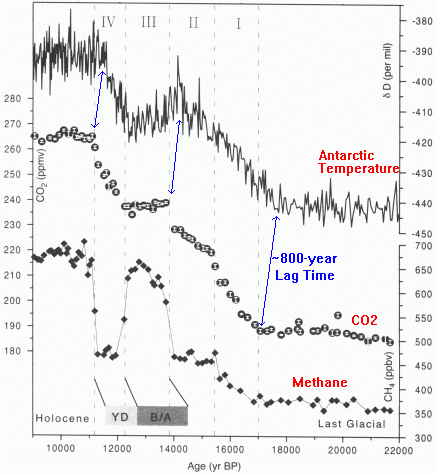
Suppose feedback effects are neutral or negative
Skeptic links
Interesting skeptic
link (extensive quote of MIT scientist Linzen that feedback will not
double and may even be negative
http://motls.blogspot.com/2006/07/carbon-dioxide-and-temperatures-ice.html
Info on scientist who have converted from pro global warming to being skeptics. Interestingly this is a press release from the US Senate Environment and Public Works committee.
US Senate Environment and Public Works committee link
Reasons to really
worry
A simple scaling from ice age data says the earth (long term) is in big
trouble.
Is this another crank? "I have published some papers on this, a few
that have been banned from publication by malign reviewers" Tom Goreau
Link below
(purports to be a briefing paper for Kyoto from the Global Coral Reef Aliance),
but the president (& maybe the whole aliance) appears to be an unaffiliated
guy (Thomas J. Goreau, Ph.D.) based in Cambridge MA. His bio says
Phd. Biogeochemistry, 1981, Harvard University
M.S, Planetary Astronomy, 1972, Caltech
B. S, Planetary Physics, 1970, MIT
1 ) Sensitivity of temperature to carbon dioxide in the observed record is around 10.7 times greater than that of the IPCC projections,
2) Sensitivity of sea level to temperature in the observed record is around 99.7 times greater than that of the IPCC projections,
3) Sensitivity of sea level to carbon dioxide in the observed record is around 1250 times greater than that of the IPCC projections.
"These comparisons suggest that the projections which are now being used to assess acceptable rates of climate change appear to greatly underestimate the ultimate extent of potential changes in global climate variables based on those which have actually taken place in the past. One reason for this large discrepancy could be that the models do not include all of the major feedback mechanisms by which changes in one climate variable affect another, such as changes in global ocean circulation and changes in the metabolism of the biosphere."
"Strong feedback mechanisms must exist in the climate system which greatly amplify small changes in sunlight reaching the earth, because otherwise the earth's climate would not show such large changes between ice age and warm conditions which vary in synchrony with changes in the earth's orbit around the sun, but these feedback mechanisms have not yet been adequately understood, characterized, or included in existing climate models. It is no criticism of the sophistication of the climate models to say that if they oversimplify reality and underestimate the actual past variations we should use actually observed changes rather than theoretical model results as our guide."
http://www.globalcoral.org/climate_change_impacts_have_been.htm
http://www.opendemocracy.net/globalization-climate_change_debate/2558.jsp
During ice ages a 100 ppm change in CO2 is associated with a 10C change of (arctic or average?) temp and huge changes in sea level. These big effects have to be (somehow) explained away, but I see (so far) almost nothing on this. Did the albedo change big time? Isn't this just a feedback effect.
But Hansen looks at ice age data and says its the best data we have and no real problem, it confirms the mainstream analysis. But he doesn't really explain why projects looks so low compared to ice age changes.
Won't
the added CO2 stay in the atmosphere nearly forever?
The record shows CO2 levels virtually constant (at 280 ppm) for the last
1,000 years. This means that (at 280 ppm) carbon sinks and sources must
be in balance. So when we burn fossil fuels that put extra CO2 in the atmosphere,
where are the carbon sinks that are going to take it out? Sure there is
a sink in little sea critter making shells that sink to the bottom of the
ocean and get buried, but Houghton says this is 0.1 Gton/yr which is only
about 1% of CO2 being added to atmosphere every year. But note, there this
ocean carbon sequestration must be balance by CO2 sources since CO2 levels
in atmosphere have been stable. There is no net carbon sink, at
least at 280 ppm. Houghton briefly talks about this at the end of his book.
The only real CO2 'sink' in coming generations that I am aware of is the ocean. Have seen no real data on this, but people talk vaguely of the ocean over one to a few centuries the ocean maybe removing the CO2 from the air as the deep ocean rolls over. But of course this causes another problem by changing the PH of the ocean, possibly interfering with the formation of shells! Wikipedia notes, though, that as the oceans warm they are able to absorb less gas.
This whole aspect of global warming , absolutely critical for the long term, seems to be wildly under reported and (maybe) wildly under researched!
Hansen's
2004 Scientific American article
2005
update, Hansen new #'s
0.85 w/m2
forcing (existing)
0.67 C/w/m2 sensitivity
(to forcing)
Leading US climate researcher James Hansen summarized his views on global
warming in a 2004 Scientific American article. Here is my summary of the
key points of the article:
* 2 w/m2 is
current forcing. A couple of pages later he says the earth is now out of
balance between 0.5 to 1.0 w/m2 ????
* at 2004
CO2 levels an additional 0.4 to 0.7 C surface increase is in pipeline
* lag is due
to ocean ("about a century to approach a new balance")
* best knowledge
of climate sensitivity comes from data on earth's history
* ice age
climate swings provide an empirical measure of climate sensitivity (critical
insight)
* Antartic
ice sheet (except for fringes) never melted in interglacia periods
* Slow earth's
orbital changes were responsible for ice ages. Total solar energy hardly
varied
but there were substantial geographical and seasonal variations
* 6.5 w/m2
is difference in climate forcing between ice age and today
and it causes a 5C difference in temperature.
* 0.75 +/-
0.25 C/w/m2 sensitivity obtained from ice age data (5C /6.5 w/m2)
* Climate
models yield a similar climate sensitivity, but ice age data best because
it includes all mechanism (even those not understood)
* Human made
non-CO2 gases are "comperable" to CO2
* Sun appears
to have brighten (few tenths of watt forcing) over last 150 years
* Ice core
data shows almost no change in CO2 over last 1,000 years
(Are solar variations only then the cause of the medieval warm period and
the little ice age?)
* 0.75C has
been surface temp increase since late 1800's
* ocean thermal
energy has increased 10 w-yr/m2 in last 50 yr (0.2w/m2 av)
(inconsisent with his 0.5 to 2 w/m2, most of which is going into ocean!)
* 1 meter
of sea rise from melted ice required 12 w-yr of energy (which would take
only 12 years at 1 w/m2)
Comments
The only (supposed)
explanation in the article of where the ice age 6.5 w/m2 forcing comes
from is this, "geographical distribution of ice sheets, vegation cover,
and coastlines during the ice age were well mapped" (How is this
an explanation of forcing power??)
Ice
age temperature variations (each cycle) were about 10C from warmest to
coldest. Hansen talks of 5C. I think the reason is that Hansen takes the
ice age mean temperature to be his baseline termperature. From that
baseline the current interglacial period is 5C warmer.
His
off hand mention that in only 12 years that 1 w/m2 provides enough energy
to melt enough ice to raise sea level one meter, is a real nut-job
comment. He apparently is referring here only to the latent melt
heat of ice, which is the addition enegy needed to melt after
the ice has been heated to 0C.
Temperature proxies
Plots
of ice age climate often show gas concentrations and temperature. Ice cores
have trapped air bubbles that provide samples of ancient air that can be
directly analyzed to find ancient concentrations of CO2 and methane. But
where does ancient temperatures come from? Paleotemperature is reconstructed
from various 'proxies':
oxygen 18/oxygen 16 (H2O of ice
in ice cores)
oxygen 18/oxygen 16 (CaCO in
shells of foraminifera is sea sediments)
argon 40/argon xx
(air bubbles in ice cores)
The most common proxy appears to be ratio of oxygen isotopes (O18 to O16) (invented in 1947 by Harold Urey). With ice cores the oxygen is obtained from the ice and in sediments is obtained from the shells (calcium carbonate) of small sea critters (foraminifera). Water with O18 is about 10% heavier than water with normal O16, so it doesn't evaporate or condense quite as easily, nor is it taken up as readily by biological proceesses. This referred to as isotopic fractionation. What makes oxygen isotopic fractionation a proxy for temperature is that it the fractionation rates are known to vary with temperature, so in princple one can convert O18 to O16 ratios in ice or shell to temperature.
People doing time lag studies of CO2 vs temperature use argon 40 as a proxy. This is more accurate in time because they can obtain both gases from the same air bubbles. How does argon work as a temperature proxy?
Baseline problem
So O18 to O16 ratios can be scaled directly (perhaps with different scale
factors for ice and shells) into temperature? Nope, there's a (serious)
complication. The final isotope ratio depends not only on the (average)
temperature when the water evaporated or the animal lived, but also on
the baseline ratio of O18 to O16 in the ocean at the time. The ratio
of O18 to O16 in the ocean varies with amount of water on earth's surface
tied up in ice. Water in ice is deficient in O18, so a lot of ice means
the oceans contain more O18. Historically, it took decades, after
O18/O16 ratios in forams in ocean sediments began to be measured in the
50's, to tease apart the ocean baseline and temperature signals.
Recent raw data
I found a
paper in a technical atmospheric physics book where recent glacier snows'
oxygen ratios were plotted aginst measured temperatures for several
years. The data shows a clear correlation between oxgyen and temperature,
but the points certainly don't fall on straight. There is quite a bit of
scatter in the data (1 to 2 C). Worse, the researchers drew two (oxygen
vs temp) straight lines with substantially different slopes through their
scatter, the difference being how the snow was collected. I did not see
where they compared their slopes to others (I might have missed it). And,
note, the uncertainty and noise shown in this paper does not include any
of the uncertainty in ocean baseline that faces paleo-researchers.
Other proxies
O18/O16 in
ocean sediments & corals proxy for (more land ice & lower ocean
levels)
H2/H1 in ice
cores proxy for temperature
Be10 in ice
cores proxy for solar activity
Sulfuric acid
in ice cores proxy for volcanic activity
How important are paleotemperatures?
Very -- paleotemperatures
play an important role in the science of global warming. They are used
to understant/verify relationship between temperature and various forcing
factors like CO2. Hansen says ice age variations is the best data we have
as to how the atmosphere responds in temperature to changes in CO2 and
methane.
Scale factor and offset
The uncertainities converting proxy concentration to temperature can be
broken down into several categories. Generally a simple linear model is
assumed,
meaning if temperature is plotted vs proxy concentration the result would
be a staight line. (In the real world, of course, this will not be exactly
true, but if the proxy is well chosen will be true enough.) The equation
of a straight line is y =ax + b, where y is the vertical and x horizontal.
'a' is the slope of the line and b moves the straight line up and down
on the vertial axis.
In the world of measurment 'a' is often called the scale factor and 'b' the offset. The scale factor (alone) allows a change in concentration to be converted to a change in temperature. To convert a specific concentration to a specific temperature the offset must also be known. Generally a calibration is done at one point, meaning (specific concentration <=> specific temperature), so in general to convert concentrations to specific temperatures both scale factor and offset must be estimated.
How
accurate are paleotemperatures?
A lot of papers
plot vs proxies. This is clean. (It's similar to astromomers plotting distant
objects vs redshift.) Converting proxies to real temperatures (in deg C)
is far from simple. There's the problem of noise (scatter) in the data.
Technical problems (of which I know nothing) likely abound. Temperature
changes are affected by uncertainties in gain (slope of proxy concentration
vs temp). If absolute tempeerature is to be assigned to a specific proxy
concentration, there are also uncertainities in offset. The baseline
ocean oxygen concentration falls into this category.
My look at some recent raw glacier data and all these error sources makes me believe that paleotemperature plots (in deg C) need to be taken with a big grain of salt. My guess is that uncertainties in (delta T/delta concentration) are in the range of 1.5 to 2. Absolute temperatures have even more error, due to calibration and baseline errors. I'm betting the uncertainity in gains, which affect temperature difference plots, might be a factor of 1.5 to calibration and baseline problems. On top of this the raw data may has some scatter and nise. (Ice core proxy data tens to look continuous (no jumps). I wonder how much filtering is done on this published data?
So clearly an important question (one that I rarely, if ever, see asked) is how accurate are paleotemperatures? How big is the correction factor due to ocean isotopic ratios, and how well known is it? Hansen implies it is about a 15% factor for ice age data, but I have seen other reference that say it is much larger.
The O18/O16 ratio of snow depends not only on the temperature, but on the baseline O18/O16 ratio in the oceans. This in turn varies with the amount of water tied up in ice. According to Houghton the ocean O18/O16 ratio is about a 15% correction factor when converting oxygen rations into temperature. Ocean sediments are used to estimate this correction term. Also the difference in O18 and O16 evaporation rates are known to vary with temperature (about 0.7 part per thousand/deg C).
Global
warming in the old days, or CO2 in early earth
Here is Wikipedia
(Paleoclimatology) on CO2 in the early earth:
The very early atmosphere of the earth contained mostly carbon dioxide (CO2): about 80%. This gradually dropped to about 20% by 3,500 Ma. This coincides with the development of the first bacteria about 3,500 Ma. By the time of the development of photosynthesis (2,700 Ma), CO2 levels in the atmosphere were in the range of 15%. During the period from about 2,700 Ma to about 2,000 Ma, photosynthesis dropped the CO2 concentrations from about 15% to about 8%. By about 2,000 Ma free O2 was beginning to accumulate. This gradual reduction in CO2 levels continued to about 600 Ma at which point CO2 levels were below 1% and O2 levels had risen to more than 15%. 600Ma corresponds to the end of the Precambrian and the beginning of the Cambrian, the end of the cryptozoic and the beginning of the Phanerozic, and the beginning of oxygen-breathing life.Anti-greenhouse effect
But suppose a component of the atmosphere had the opposite properties, meaning it was opaque to solar radiation and transparent to infrared radiation. What would be the effect? The effect would be to cool the surface. This is the anti-greenhouse effect. It happens transiently on earth when a volcano spews lots of dust into the high atmosphere, but it's really important only on Saturn's moon titan.
Titan has a lot of methane in its atmosphere, and the methane gas particles clump together to make large particles that make a haze in its atmosphere. (Titan's atmospheric haze was measured by the Huygens probe that landed on its surface.) Titan's methane haze absorbs the incoming solar radiation and reradiates it, some back out to space and some down to the surface, while being transparent to the infrared radiation from the surface. Since only a fraction (ideally half if the absorbing layer is thin) of the sun's energy reaches Titan's surface, this anti-greenhouse effect lowers Titan surface temperature by about 8C.
CO2 and oceans
It is important
to keep in mind the following distinction
During the ice ages the av ocean temperature probably went up and down by 5-10C degrees. Since cold water can hold more CO2 in solution than warm water, CO2/ocean exchanges during ice ages were likely influenced (strongly?) by the ocean temperature changes. Our current global warming concern is different. What is important in the near future is how CO2/ocean exchanges work when there little to no ocean temperature change, just a buildup of CO2 in the atmosphere.Hydrogen bomb tests in the Pacific doubled the concentration of radioactive C14 in the atmosphere as well as putting out a lot of other radioactive materials. This 'experiment' was extremely useful (from a global warming perspective) as it allowed direct measurements of how gases from the atmosphere are absorbed into the ocean. Prior to the 1950's it was generally assumed that CO2 from fossil fuel burning was not not going to build up in the atmosphere due to the oceans.
Firstly, it was found that radioactive material did not dissolve throughout the ocean water it remained in the top meter or so. Secondly, the (net) absorption rate of CO2 into the oceans was found to be about ten time lower than expected. In technical terms, sea water is a "buffered" solution, it resists the change in acidity that an uptake of CO2 would involve. (CO2 in solution makes carbonic acid, a weak acid.) 90% of CO2 molecules from the atmosphere that wind up in the oceans soon get evaporated out. Bottom line, removal of CO2 from the atmosphere by the sea is very slow (centuries?) as confirmed by the buildup of CO2 in the atmosphere over the last 30 years.
Here is Wikipedia on CO2 and oceans --- "The vast majority of CO2 added to the atmosphere will eventually be absorbed by the oceans and become bicarbonate ion, but the process takes on the order of a hundred years because most seawater rarely comes near the surface. (In the 1950's three studies using carbon-14 estimated the oceans turned over completely in several hundred years.) As the oceans warm, carbon dioxide solubility in the surface waters decreases markedly. However, the overall system is quite complex. (see the article on the carbon solubility pump)."
The Science article (below) says this about CO2 and oceans during ice ages --- "CO2 (in the atmosphere) may be controlled in large part by the climate of the south ocean. ... A delay of about 800 years seems to be a reasonable time period to transform an initial Antarctic temperature increase into a CO2 atmospheric increase through oceanic processes.
Excellent reference: (includes a 25k book)
http://www.aip.org/history/climate/Radmath.htm#L_0623
CO2 and ice ages
A recent paper, which I have read, in the journal Science (Caillon, et
al) argues that during the ice ages CO2 increases, while clearly preceding
deglaciation which takes 5,000 years or so, tended to lag temperature changes
by about 800 years. They conclude (page 1730):
"This confirms that CO2 is not the forcing that initially drives the climatic system during a deglaciation. Rather, deglaciation is probably initiated by some insolation forcing (more sunlight in northern hemisphere)."
"This sequence of events is still in full agreement with the idea that CO2 plays, through its greenhouse effect, a key role in amplifying the initial orbital forcing."Note they are not arguing that if CO2 lags temperature it proves that CO2 is not responsible for the temperature change. Just the opposite, they argue only that the lag indicates that CO2 is not the trigger, but CO2 is still (to some unspecified extent) 'responsible' for the temperature changes due to its greenhouse amplifying effects.
Here is the 2003 Caillon (et al) Science paper:
http://icebubbles.ucsd.edu/Publications/CaillonTermIII.pdf
CO2 and snowball earth
The snowball
earth hypothesis posits that the earth completely frozen over (oceans everywhere
frozen a km thick and glaciers at the equator) a few times between 650M
and 800M years ago. It explains why glacial dropping of this age are found
at sea level on continents that at the time were near the equator, and
why immediately above them is always a carbonate cap (layer of carbonate
rocks). The argument is that in the very long term (million of years)
CO2 levels in the atmosphere are set by a balance between CO2 removed by
weathering of rocks (bicarbonate ions from weathered rocks are washed into
the sea and combined with calcium and buried in the sea floor as carbonate
rocks) and CO2 from volcanos (carbon is recycled from melted, subducted
ocean floor).
The trigger for the snowballs is not known for sure, but may it may have been that all the continents were relatively near the equator. This meant initially no arctic or antarctic glaciers, so the rock weathering CO2 removal rate stayed high as CO2 and temperatures levels dropped. Also the sun was 6% weaker then than now. Ocean ice has a much higher albedo than open water, so an expansion of ocean ice increases the earth's albedo causing more cooling which causes more ocean ice. It's positive feedback, and snowball earth is the end point of a runaway condition. The global earth temperature is thought to have dropped to an amazing -50C.
At first the snowball theory had little support because no one could figure out how if the earth got into this state it could get out. The snowball recovery is now posited to be a huge build up of CO2 in the atmosphere to x300 to x1,000 times higher than now (10% to 30% of the atmosphere). The idea is that with the earth covered in ice the rock weathering CO2 loss mechanism shut down, but volcanos kept putting out CO2. It took about 10 million years for CO2 levels to get high enough to melt the ice, but when the ice melted it melted very fast. The very high CO2 levels then roasted the earth with the global temperature rising from an amazingly cold -50C to an amazingly hot +50C (a 100C change!!) in only few hundred to 1,000 years. (For perspective the temperature change over an ice age cycle was only about 10C or so.)
Since the snowballs
must have killed off most of early life (all single cell life) and put
surviving life under great stress, and since this happened just before
the Cambrian explosion, it's a good guess that snowball earths played a
important role in advancing life beyond the single cell stage.
------------------------------------
Medieval Warm Period
A number of
researchers are looking carefully at the Medieval Warm period. If it could
be shown (as some suspect) that it was warmer then than now, it would be
strong piece of data that at the very least the 'noise' level of climate
variation is larger than generally believed. If the Medieval Warm period
does turn out to be quite warm, then it has direct bearing on the global
warming as a political issue because a key issues is whether (or not) recent
warming rises above the noise of natural climate variation.
Global Dimming
Dimming as a 'hot' topic is apparently new. Nova recently did a whole hour
on it, it what appeared to be a scientifically credible show. I don't think
the term 'Global Dimming' even appears in Hansen or Houghton.
(most of the following from my memory of the Nova show. It needs to be researched)
--- Apparently there is a long (100 yr?) record kept (by farmers?) of 'pan evaporation rates'. This is a measure of how long it takes a thin layer of water in a pan outdoors to evaporate. This record shows a large (10% of so) decline in recent years.
--- Pan evaporation rates depend on a lot of things (temp, wind, sunlight), but analysis by two guys in the film finds the amount of sunlight is dominant factor. (One is quoted as saying the photons pop the water molecules out of the pan.) So interpretation of slower pan evaporation rates says that less sunlight is reaching earth's surface.
--- We know from solar satellites that the sun's energy output shows no downward trend in the 20-30 years for which we have satellite data. So interpretation is that lower pan evaporation rates mean more cloudiness.
--- A large experiment run in Maldives Islands, comparing southern islands (far from India) with northern islands (near India), shows (I think?) that particulate pollution from man is causing more cloudiness, and this effect is x10 larger !! than was thought.
--- (somehow) This is all tied in with data taken during the 9/11 plane groundings. The 9/11 data showed that the wispy clouds from plane contrails do have a measurable effect on earth's surface temperature.
Conclusions ---- The show's theme is that recent research has shown that 'Global Dimming' caused by particulate associated cloudiness is an important factor in earth's energy balance. In fact 'Global Dimming' is probably canceling 1/2 the power imbalance due to increased greenhouse gases! Presumably (though the show didn't say so) this effect is due to an increase in the earth's albedo. (Obviously, this is very important to the Global Warming debate, if it is true/correct.) So is this good news or bad news?
Bad news? --- Nova, of course, concludes that Global Dimming is bad news because the underlying global warming may be twice as great as we have thought. That is, if we lower air pollution, then the global warming effects will strengthen. Fair enough, but I don't think that's the whole story.
Good news? --- A manmade effect (recently thought insignificant) is now shown? to be canceling half of the effect of global warming. In other works man can do something about global warming (besides reducing greenhouse gases), because he already done it. It's a 2nd unplanned experiment man has run on the earth. If man has been able to dump stuff into the atmosphere to cancel half the greenhouse effect in a totally unplanned way, doesn't this demonstrate that man has the capacity to compensate for more greenhouse gases by (on purpose) putting stuff into the atmosphere to increase albedo?
Finally, a note of caution. If Global Dimming is true, it shows the extremely poor understanding of clouds and what effects them. According the experts on the Nova show, the importance of particulate pollution to global warming had been underestimated by a factor of 10!! (It's a 50% term, not a 5% term.)
It seems to me that our new understanding of the role of clouds in Global Dimming, also tends to strengthen Svenmark's claim that cloud changes caused by solar modulated cosmic rays are important. When we know more of how clouds form, we may find that the sun plays a much bigger role than we think now.
Circuit analog thermal models
Engineers
often make circuit models of simple thermal systems. This involves modeling
heat flows and lags (whose details are complex) with resistors and capacitors.
Resistors model conductors with temperataure assumed to be proportional
to the power flowing through the conductor (units are deg C/watt).
Capacitors model heat capacity with capacitance assumed proportional to
the energy the material can absorb per degree of temperature rise (units
are joule/deg C). This type of modeling is used for the design of
transitor cooling systems. It relatively simple, and has the advantage
that it allows the large knowledge and intuition of electrical circuits
to be applied to thermal problems.
An electrical model can also be used to model the dynamics of heat flow. The rate at which heat will flow into a large body by conduction (when the temperature at the surface increases) can be modeled with an RC ladder model. This is analogous to LC ladder models, which are used to model the high speed propagation of signals (at near speed of light) down long cables.
Electrical
Thermal
---------------
--------------
voltage <=>
temperature
current <=>
power
resistor <=>
temp/power (thermal resistance)
capacitor <=>
energy/temp (thermal capacity)
Can this type of modeling be used for the grreenhouse effect? Don't know yet, but there is reason to think that they don't apply. These methods are used with solid materials where the dominatnt method of heat flow is conduction. In contrast heat flows in the greenhouse effect are radiative.
Data from weird science site #1 -- Distaster coming
This site
claims the earth is already headed for disaster with a coming 14C rise
(in the next 140 years) due to CO2 already in the atmosphere. This result
he is derived from a simple, linear model that CO2 only
controls
temperature rise (above radiative equilibrium temp). Since present CO2
levels (390 ppm) are already about 139% of pre-industrial levels (280 ppm),
his prediction is that current CO2 levels will eventually cause a 0.39
x 33C rise = 13C (author gets 14.4 C because he uses a high albedo of 0.34
vs the accepted value of 0.30). He believes excess energy is now
going into the earth's crust, and he calculates its energy absorption limit
will be reached in about 140 years.
This internet guy is a stove designer, and says he has a degree in nuclear physics from Univ of Chicago. Here are numbers from his site.
Equilibrium surface temp (no CO2) ( note 1)
-9 F (-23C) (250K)
CO2 temp rise (steady state, above equilibrium temp) (note 2)
before 1800 (280 ppm CO2)
66 F (36.7C) rise (57 F) earth
'final' at current CO2 level (390 ppm)
92 F (51.1C) rise (83 F) earth
(an extra 51.1C -36.7C =14.4 C rise 'in pipeline')
Response time (due to thermal mass of upper crust) (note 3)
143 yr
Current world temp
15C, 59 F, 288K
CO2 total in atmosphere
1,760 bil ton
CO2 added to atmosphere (by respiration & burning)
300 bil ton/yr
CO2 removed from atmosphere (by photosynthesis)
300 bil ton/yr
CO2 added to atmosphere by man (burning fossil fuels)
8 bil ton/yr
CO2 removed from atmosphere (by ocean)
6 bil ton/yr ??
CO2 removed from atmosphere (by weathering of rock)
? bil ton/yr ??
CO2 eq added by outgassing (CO2 from volcanos and CH4)
6 bil ton/yr??
Notes
1) This calculation
is actually very simple equating incoming power from the sun (and heat
flows from inside earth) to radiated outflows calculated from the Stefan-Boltzmann
equation (black body radiation). His calculation is confirmed by another
site that using the same albedo gets -21C (2 C lower). He uses the following
values:
albedo = 0.34 (meaning 34% of solar energy is reflected)
av heat flow to surface from sun = 283.6 Btu/sqft/hr (894 w/m2)
?
(btu/hr x 0.293 = watt) (1 sqft = .0929 m2)
av
heat flow to surface from inside earth = 0.02 Btu/sqft/hr (negligible)
2) Confirming
data for a linear model is from ice age temperates using ice cores:
ice core data (188 ppm CO2)
44 F (24.4C) rise (35 F) earth
3) The response time calculation is based on heat flow from the atmosphere needed to heat the topmost 2,900 feet of crustal rock to 83F. At depths below 2,900 feet the rock is already hotter than 83F. (Note his calculated response time does not seem to include the ocean! The ocean absorbs CO2 from the air over (approx) 100 yr (?) lifetime, and it seems to me ocean temperature must climb if air temperature is to climb, and water also has a large thermal inertia.)
Most of the data above is from this link
This site
is interesting and has a lot what seems to be original stuff.
http://mb-soft.com/public3/global.html
Data from weird science site #2 -- No problem
My initial reaction is that this is a terrific site. They calculate different
ways quoting always their sources (which appear reputible) and detail their
calculations. They comment intelligently and consider 2nd order effects.
Their baseline forcing watt/m2 come from an IPCC report formula: F = k x ln(C/Co) where Co is pre-industrial concentrations of CO2 and k = 5.35. For a 'doubling' of CO2 (from pre-industrial levels) the formula gives 5.35 x ln (560 ppm/280 ppm) = 3.7 w/m2. (This looks clean.) National Accademy in 2001 was using about the same value (4 w/m2).
This site figures the {temperature rise per w/m2} scale factor by dividing the 33C surface rise by the 324 w/m2 shown radiated down from the atmosphere in the Kiehl figure below, which gives a (linear) scale factor of 0.1 C per w/m2. Scaling by their baseline forcing of 3.7 w/m2, they get a steadystate rise of 0.37C. At first glance this analysis looks very reasonable.
The note the work of Sherwood B. Idso (CO2-induced global warming: a skeptic’s view of potential climate change, 1008) who describes 8 natural experiments from which he arrives at an av surface air temperature sensitivity factor of 0.1 C/wm-2.
The IPCC's estimate of additional forcing from all added CO2 since the Industrial Revolution is 1.5 Wm-2. They quote Professor Roger Pielke, Sr, who thinks that only 28% of warming come from CO2.
They do other calulations that yield 0.25 C per w/m2, yielding 0.25 x 3.7 w/m2 = 0.93 C final rise. They quote National Academie (2001) "If there were no climate feedbacks, the response of Earth's mean temperature to a forcing of 4 W/m2 (the forcing for a doubled atmospheric CO2) would be an increase of about 1.2 °C ."
Their max upper final figure is 1.6 C rise (from pre-industrial baseline) for doubling of C02 (@ 6.3 w/m2 forcing), which is +1C more than today. The higher figure assumes other greenhouse gases rise when CO2 rise and they argue it is very conservative, since it includes solar variations and ignores the fact is unlikely to increase further.
They ask the question, "Why are numbers so low?" They speculate (reasonably) that small increases in warming may be increasing albedo slightly. This would be a real world negative feedback effect.
They comment on Hansen, "Climate models usually work on 0.5 - 1.0 °C per Wm-2, which is how they come up with such fantastic warming projections. These numbers are apparently used based on this estimate by James Hansen: Global climate forcing was about 6 1/2 W/m2 less than in the current interglacial period. This forcing maintained a planet 5 °C colder than today." (5C/6.5 w/m2 = 0.75 C/m2)
Hansen's Scientific 2003 American article article (on Junk Science site)
"In Earth's Energy Imbalance: Confirmation and Implications Hansen, et al, state: Our climate model, driven mainly by increasing human-made greenhouse gases and aerosols, among other forcings, calculates that Earth is now absorbing 0.85 ± 0.15 watts per square meter more energy from the Sun than it is emitting to space. This imbalance is confirmed by precise measurements of increasing ocean heat content over the past 10 years." But a 2006 paper using data from the same source says ocean has not warmed at all in the last few years. These athors (Lyman) conclude forcing for last 13 years is only 0.33 w/m2.
Conclusion --- Real-world measures suggest moderate to strong negative feedback, currently unnamed and un-quantified, mitigates the Earth's thermal response to additional radiative forcing from both human activity and natural variation.
On balance of available evidence then the current model-estimated range of warming from a doubling of atmospheric carbon dioxide should probably be reduced from 1.4 - 5.8 °C to about 0.4 °C to suit observations or 0.8 °C to accommodate theoretical warming -- and that's including Forcing of 3.7 Wm-2 from a doubling of pre-Industrial Revolution atmospheric carbon dioxide levels, a figure we suspect is also inflated.
Here is his summary of key points:
* the textbook derivation of globally averaged greenhouse, using Stefan's
Constant, evaluates to roughly 33 °C and 150 Wm-2
* the IPCC Third Assessment Report alt: Third Assessment Report (Equation
6.1) states: "The climate sensitivity parameter (global mean surface temperature
response delta Ts to the radiative forcing delta F) is defined as:
delta Ts / delta F = lamda"
*a blackbody-equivalent Earth climate sensitivity parameter (lamda) would
be 33 / 150 = 0.22 °C per Wm-2
* substituting the values from Earth’s Annual Global Mean Energy Budget
(Kiehl and Trenberth, 1997) produces a lamda value of ~0.1 K per Wm-2,
as does using values derived by Professor Roger Pielke, Sr. and this is
in agreement with the sensitivity derived by Idso in eight natural experiments
described in CO2-induced global warming: a skeptic’s view of potential
climate change
* Earth then responds at less than half the rate of a perfect blackbody
due to a preponderance of negative feedbacks
* climate models use lamda values of 0.75 ± 0.25 °C per
Wm-2, 5-10 times greater than empirical measures support
* Hansen (and GISS model E) prematurely claimed support for these extreme
values in Earth's Energy Imbalance: Confirmation and Implications, releasing
Earth’s Energy Out of Balance: The Smoking Gun for Global Warming
* continued measurement showed model E was incorrectly dumping heat into
the modeled oceans at a rate of more than 0.8 Wm-2 when it should have
been removing it at about -1.0 Wm-2, destroying the claim of agreement
between model and real world
* climate models produce excessive future climate warming estimates due
to erroneously large lamda values
* a realistic value for estimated warming induced by a doubling of atmospheric
carbon dioxide is about 0.4 °C.
http://www.junkscience.com/Greenhouse/What_Watt.htm
Greenhouse rise does apear to be (approx) linear
with CO2
From
my ice core data (above) -- Approx 10C difference <=> 100 ppm
of CO2
Simple (linear) scaling says todays levels that are about 110 ppm higer
than baseline (390 vs 280 ppm) is equivalent to an extra 11C rise in the
'pipeline'. Of course, this ignoring albedo differences between ice age
and now. Dividing the total global warming above radiative balance (33C)
(albedo = 0.3) by baseline (preindustrial CO2 (280 ppm) we get 100 ppm
= 11.8C rise (=33/2.8). This look pretty linear and is the model Johnson
(weird science) is using. Is there any science behind this, or is it just
empirical?
But Johnson uses a higher albedo (.34 vs .30) which should lead to a 3.08% x 255K =7.8 C {(.30/.34)^0.25 = 0.979} lower thermal equilibrium temp and a higher baseline greenhouse effect. Johnson gets an equilibrium temp 5C lower 23C (250K vs 255K for UC) and figures baseline greenhouse rise at 36.7C (vs 33C UC), coming up with an extra 14.4C in the pipeline (390 ppm).
A key point is that Hansen (in 1993) allocates only about 32% (=.0.8 x 2.6 w/m2/6.6w/m2) of ice core temperature variation to CO2 (rest to albdo differences and aerosls). With Hansen albedo 32% fudge reduction is applied to Johnson's 14.4 extra pipeline rise it is reduced to 0.32 x 14.4 = 4.6C. This is roughly consistent with Hansen claim that
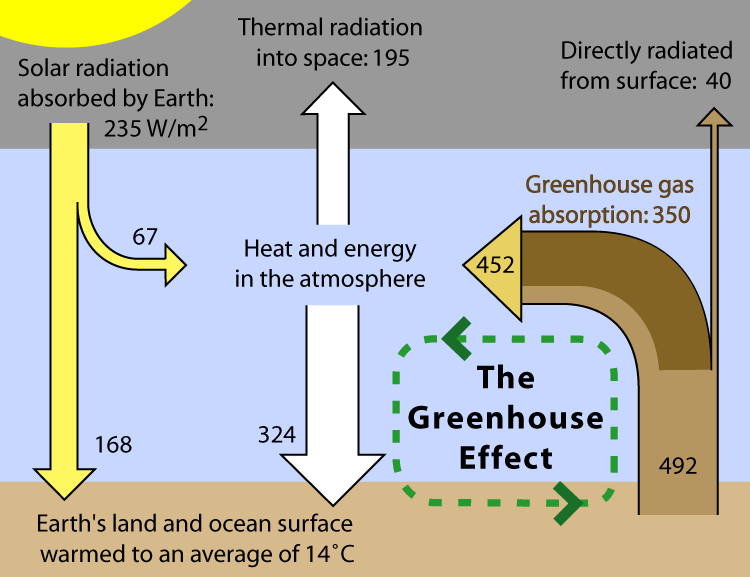
Is this Wikipedia figure right?
Yes, but it
is misleading. The problem I have with this figure is that it appears
that the earth's surface is not radiating as a blackbody. The calculated
earth temperature with no greenhouse effect (albedo =0.30) at radiated
in/out power of 240 w/m2 (235 w/m2 here). The av surface temp is
288K, which is rise above radiated equilibrium of 33C. Since blackbody
radiated power varies as the forth power of absolute temperature, if the
surface radiates as a blackbody its radiated power at 288K should be {(255
+33)/255}^4 = 1.627 times incoming power (1.627 x 240 w/m2 = 390 w/m2),
whereas the figure above shows 492 w/m2 coming up from the earth.
I found a more nuanced version of the above figure in a technical book on atmospheric dynamics, and it is the Kiehl figure near the top of this essay. The upper figure is a simplified version of the figure below, the numbers agree exactly. Note in the figure below the surface radiated power is shown as 390 w/m2, which is exactly what we calculated above. The surface does radiate as a blackbody! Notice about 21% {(102 w/m2)/(492 w/m2)} of the power from the surface to the atmosphere is done by mass transfer, not radiation. Most of this appears to be heat transfer in water vapor. Water evaporated on the surface cools the surface, and when the water vapor condenses in clouds, it releases the heat it took from the surface into the atmosphere. (I'm surprised the figure doesn't show heat transfer in mass downward. Does rain transfer heat from the atmosphere to the surface?)
Notice how the atmosphere radiates in the Kiehl figure: about 38% goes up and 62% down to the surface. At this point I don't know what controls this split, but it's obviously important as it directly affects the power to the surface and hence the surface temperature.
Textbook earth climate sensitivity parameter
A textbook calculation of the climate sensitivity parameter (scale factor
you multiply by forcing power to get temp rise) goes like this. All feedback
factors are ignored. The greenhouse effect power is calculated by assuming
that all power leaving the surface is blackbody radiation
(this differs markedly from Kiehl in fig above!). The standard values of
temperture and absorbed power are used: albedo of 0.3, 33C rise above a
radiative baseline of 255C, and absorbed solar power of 240 w/m2.
greenhouse power = [1 - {(255 +33)/255}^4] x 240 w/m2
= (1 - 1.627) x 240 w/m2
= 150 w/m2
climate sensitivity parameter = 33C rise/150 w/m2
= 0.22 C per w/m2
Note, the widely quoted Kiehl numbers (Widipedia & thermodynamic textbook) have the greenhouse power, i.e. the 'extra' power coming to the surface from the atmosphere, at 324 w/m2 (see fig above). This reduces the 'climate sensitivity parameter' to less than half the textbook value (0.1 C per w/m2 = 33C rise/ 324 w/m2).
Global energy balance
The numbers below
are my baseline for global warming. They were obtained from Wikipedia and/or
a lecture on global energy balance from a prof at UC (Univ Calif at Irvine)
at link below.
Stefan-Boltzmann constant
5.67 x 10^-8 w/m2-K4
Earth radius
6.367 x 10^6 m
Earth surface area (4 pi r^2)
5.09 x 10^14 m2
Earth's disk area (pi x r^2)
1.274 x 10^14 m2
Hydrosphere mass (total) (oceans + ice)
1.4 x 10^21 kg
Ocean volume
1.34 x 10^18 m3
Ocean surface area
3.61 x 10^14 m2
Water (liquid) heat capacity
4,180 joule/kg-K
Seawater thermal conductivity
0.65 w/m-K
Earth's crust thermal conductivity
1.7 w/m-K
Earth's crust thermal capacity
800 joule/kg-K
Atmosphere mass (total)
5.15 x 10^18 kg
Air heat capacity
1,000 joule/kg-K
Solar flux (total luminosity)
3.9 x 10^26 w
Distance sun to earth (av)
1.5 x 10^11 m
Solar flux at earth's distance
1,370 w/m2 (solar constant)
3.9 x 10^26 w)/ (4 x pi x (1.5 x 10^11)^2
Energy absorbed by earth (albedo=0.3)
1.222 x 10^17 watt
solar constant x disk area x (1- albedo)
1,370 w/m2 x 1.274 x 10^14 m2 x (1-0.3)
Energy absorbed per m2 (sphere area, daily average) 240
w/m2
= 1.222 x 10^17 watt/ 5.09 x 10^14 m2
= solar constant x (disk area/sphere area) x (1-albedo)
= 1,370 w/m2 x (1/4) x (1-0.3)
Earth's black body temp (calculated, albedo =0.3)
255 K
(255K = -18C = -0.4 F)
(for albedo = 0.34, then 251.3 K (-21.7 C, -7F)
Geenhouse heating (albedo =0.3)
33 C (59.4F)
measured temp - calculated black body temp
288K - 255K
Energy emitted by earth (black body)
1.220 x 10^17 watt
= 5.67 x 10^-8 w/m2-K4 x (4 pi r^2 of earth) x Temp (K)^4
= 5.67 x 10^-8 w/m2-K4 x 5.09 x 10^14 m2 x (255K)^4
= 1,220 x 10^14 watt
Earth's mean surface temp (measured)
288 K (15C, 59F)
(288 K = 15C = 59F)
blackbody power = k T^4, so on this basis
an extra 33C rise is equivalent to 390 w/m2 vs 240 w/m2,
162.5% increse , an extra 150 w/m2
(150 w/m2 is textbook value, Wikipedia has an even higher number!)
Incoming solar radiation (100 total, albedo =0.3)
70 % absorbed (50% by surface, 20% by atmosphere & clouds)
30%
reflected (20% by clouds, 6% by atmosphere, 4% by surface)
Radiation back to space (70 total)
(60% from atmosphere, 10% from surface)
Mass of hydrosphere column (av over whole earth surface)
2.75 x 10^6 kg/m2
1.4 x 10^21 kg/5.09 x 10^14 m2
Depth of ocean (av over whole earth surface)
2.63 x 10^3 m
(% of surface covered by oceans) x (av ocean depth)
(3.61 x 10^14 m2/5.09 x 10^14 m2) x 3,711 m
0.709 x 3.71 x 10^3
Density of ocean (references => 1.03 x 10^3 kg/m3)
1.04 x 10^3 kg/m3
(mass of ocean)/(volume of ocean)
1.4 x 10^21 kg/1.34 x 10^18 m3
Heat
capacity of hydrosphere (total)
5.8 x 10^24 joule/deg C
4,180 joule/kg-K x 1.4 x 10^21 kg
Heat capacity of hydrosphere (per m^2, average)
1.15 x 10^10 joule/m2-deg C
4,180 joule/kg-K x 2.75 x 10^6 kg/m2
Heat capacity of cubic meter seawater
4.35 x 10^6 joule-m3/deg C
4,180 joule/kg-K x 1.04 x 10^3 kg/m3
Earth's total heat absorbed (@ 1 w/m2 unbalance)
5.09 x 10^14 joule/sec (w)
1 w/m2 x 5.09 x 10^14 m2
Time to heat hydrosphere 1C @ 1 w/m2
361yr
(5.8 x 10^24 joule/5.09 x 10^14 joule/sec)
x 3.16 x 10^7 sec/yr
Seconds in a year
3.16 x 10^7 sec/yr
3.6 x 10^3 sec/hr x 24 hr/day
x 3.6525 x 10^2 day/yr
UC lecture on radiative balance
http://www.ess.uci.edu/~yu/class/ess200a/lecture.2.global.energy_cycle.pdf
Heat flow to the surface of earth's internal heat
thermal conductivity rocks (earth's crust)
1.5 to 3 w/m-K
geothermal temperature gradient in earth's crust
20C/km or 0.02 C/m
geothermal power flow = temp gradient x thermal conductivity
= temp gradient/thermal resistance
= (0.02 C/m x 3 w/m-K)
= 0.06 w/m2
Putting the
numbers together we find the geothermal power flow (upward) through the
crust to the surface is small: 0.06 w/m2 (= 0.02 C/m x 3 w/m-K).
There must also be a TBD term due to heat released by volcanos. 0.06
w/m2 is 4,000 times (0.025%) smaller than the 240 w/m2 solar power absorbed
by (earth + atmosphere), so it can be neglected in calculating earth radiative
equilibrium temperature.
======================================================
Toy Model 1 --- An isothermal ocean closely coupled to the atmosphere
Supposed the sun shut off!
A useful bench
mark to get a feeling for how much thermal energy is stored in the oceans
is to ask what would happen if the sun suddenly shut off. Let's make the
(unrealistic) assumption that the heat flow and/or circulation within the
oceans are good enough to keep ocean temperture (approx) isothermal. How
fast would the ocean temperature decline? The heat energy stored in the
atmosphere is about 1,000 times less than the oceans, so can be neglected.
I also assume the heat stored in the interior of earth is isolated (in
the medium term) from the surface by low conductivity rocks.
The result is that if the sun shut off and all radiation of the earth came from heat in (isothermal) oceans, then the ocean temperature would fall (initially) at the rate of 0.667 C/yr.
Scaling from no sun radiation to a small radiative
unbalance
An oft quoted
number (Hansen 2005) is that the earth has an energy unbalance of 0.85
w/m2. This is a power unbalance of 0.345%. (=0.85 w/m2/240 w/m2)
of the energy being absorbed and radiated. Again using our toy model that
the oceans are approx isothermal and closely coupled with the atmosphere,
then the rate of ocean temperature increase at 0.85 w/m2 is 0.00345 x 0.667
deg C/yr = .0023 deg/yr or 434 years to change one degree C!
Data from above
Energy absorbed/radiated by earth (total)
1.222 x 10^17 w (joule/sec)
Heat
capacity of hydrosphere (total)
5.8 x 10^24 joule/deg C
Heat capacity of hydrosphere (per m^2, average)
1.15 x 10^10 joule/m2-deg C
Energy absorbed per m2 (sphere area, daily average) 240
w/m2 (joule/sec-m2)
Seconds in a year
3.16 x 10^7 sec/yr
calculation
[(5.8 x 10^24 joule/deg C)/(1.222 x 10^17 joule/sec)]/3.16 x 10^7 sec/yr
= 4.74 x 10^7 sec/deg C/3.16 x 10^7 sec/yr
= 1.5 yr/deg C
check (same calculation on m2 basis)
[(1.15 x 10^10 joule/m2-deg C)/ (240 joule/sec-m2)]/3.16 x 10^7 sec/yr
= 4.79 x 10^7 sec/deg C/3.16 x 10^7 sec/yr
= 1.5 yr/dec C
How realistic is this? My guess is that the atmophere is closely coupled to the top layer of the ocean, but that temperature gradeints in the ocean are huge and long standing. So the thermal capacity available to absorb greenhouse power imbalances is likely much, much less, than the above total hydrosphere numbers indicate. However, the ocean does turn over in a hundred years or so (?), so over a long time scale more water is available to absorb heat.
Questions --- Why is it when you you deeper in the ocean it gets colder, whereas when you go deeper into the the earth it gets warmer? And the crust of the earth is a lot thinner under the oceans than the continents.
Guess --- It's probably because the dominant heat flow in the ocean is
convection (and water conduction is relatively poor?), so the ocean bottom
is cold water flowing down from the poles.
-------------------------------------
What is badly needed
in the global warming issue is the equivalent of a 'Peak Oil Theory'.
A simple (approx) model that would make clear (with numbers) how earth's
the temperature is affected by CO2. (maybe it exist, I am looking)
======================================================
Thermal resistance model
Consider
1) Blackbody radiative surface of the earth is around 5-6 km where the
temperature is 255K (blackbody temperature of earth). This is 33
C cooler than the surface.
2) Models show most heat radiated from earth to space is radiated from
the atmosphere. On the other hand most of the solar radiation is absorbed
by the surface.
3) The atmposphere warms when CO2 and water vapor absorb heat radiated
from earth.
Can we not consider that greenhouse gases create a thermal resistance between the real surface and the blackbody radiative surface? The value of that resistance (per m2) is 33C/195 w/m2=0.18 degC/w/m2. (195 w/m2 is power radiated to space from atmosphere shown in Wikipedia fig) Greenhouse gases then (in some way) modulate this resistance, possibly by abosrbing lower in the atmposphere and/or pushing the radiative surface up (it is reported to be rising).
Caveats
a) Resistance? Well the atmosphere is on obstacle to heat flow from
the surface to space, but it may very well not be linear (with power) since
the mechanism is absorption/reradiation.
b) How does CO2 modulate this thermal resistance (thermal obstacle)? Note,
the Wikipedia figure shows 90+% of real surface radiation is already absorbed
by the atmosphere (70% by greenhouse gases), so more CO2 cannot do a whole
lot to increase absorption. However, CO2 might be pushing the blackbody
radiative surface higher, or in some way affecting the up/down radiative
split. (Note if the up/down radiative split went from
Questions
a) Would not the earth's real surface (in this scenario) also radiate as
a black body? (how can it not?)
b) What is the mechanism by which more CO2 increases the thermal resistance?
c) Might the relation between thermal resistance & CO2 ppm be (approx)
linear?
======================================================
Weathering --- negative feedback
" Higher temperature (& precipitation) cause more weathering of rocks.
This increases sequestering of CO2 from atmosphere (tending to slow the
temperature increase)." Need to translate this into specifics.
Diurnal (day/night) temperature
Curious fact
Most global warming occurs at night
(meaning the av temp rises prinmarily because it does not cool off as much
at night).
Greenhouse effect reduces (substantially) the day to night variation in temperature.
"Tyndall had pointed out more than a century back that basic physics declared that the greenhouse effect would act most effectively at night, as the gases impeded radiation from escaping into space. Statistics did show that it was especially at night that the world was warmer."
Natural solution to global warming?
The
weird science site (above) says the following:
"If the world-wide productivity of plants would (NATURALLY) increase by just three percent, then the current consumption of 300 billion tons of carbon dioxide would increase to 309 billion tons and a new equilibrium situation (temperature) would occur... Phytoplankton in the oceans would probably not react very quickly at all. It would probably only significantly begin after the ocean temperature had risen by a number of degrees, probably meaning decades." (Measured data on soybeans indicates that when CO2 is increased from 400 ppm to 500 ppm their carbon fixing goes up about 18%. Growth rate at higher CO2 levels appears to substantially flatten.)Is this right? Nope. (It might help a little short term.) What happens where these more active plants die? The answer is almost all this carbon goes right back into the atmosphere. It make little difference how much carbon plants are recycling through the atmosphere each year. It also makes little difference what fraction of carbon is naturally sequested. Think about it. A steady state level of CO2 in the atmosphere (say, before the industrial revolution) requires that whatever carbon gets sequested must somehow be replaced from natural sources (very likely outgassing of primal hydrocarbons and CO2).
The only answer
I see is this --- If man is going to burn up all the fossil fuel adding,
say, 8 bil/ton of CO2/yr to the atmosphere, then to prevent CO2 levels
in the atmosphere from rising further an extra 8 bil ton/yr of CO2
needs to be sequested. It's as simple as that. Solving global warming is
(plain & simple) a matter of carbon sequestration.
--------------------------------------
Copy of an email I sent him (4/21/07)
C Johnson
Just found your very interesting
global warming site. (I like your writing style; you make your key points
pretty clearly.) I have two question/comments.
I have read a lot about global warming (I have my own global warming home page), but have not seen before your (seemingly powerful) argument that the earth's steady state, surface temp rise above radiative equilibrium is likely to be approximately proportional to atmospheric CO2 level. It's certainly seems like a good first order model to me (I am an engineer), and if true, it's scary as hell. So my question is this: Is there another side to this story? What do the climate modelers have to say about this model?
My second point is relates to your discussion of plants taking in more than 300 bil tons/yr of CO2, either because there are more plants or because they are more efficient fixing carbon at higer temp & CO2. You said this:
"In this case, if the world-wide productivity of plants would (NATURALLY) increase by just three percent, then the current consumption of 300 billion tons of carbon dioxide would increase to 309 billion tons and a new equilibrium situation would occur."
Really? I don't think so. If more efficient plants take in, say, 309 bil tons, then when they die and are oxidized they put out (about) 309 bil tons. I don't see how an increasing roll over of natural carbon through photosynthesis does anything significant (in the long term) to blunt the rise in CO2 levels from the burning of fossil fuels.
Seems to me global warming is at root an issue of carbon sequestration. Either leave the carbon in the ground, or if we take it we need to capture the carbon after we burn it and bury it.
Don Fulton
----------------------------------------
Random web notes CO2 vs forcing power flows
Wikipedia -- "Recent measurements indicate that the Earth is presently
absorbing 0.85 ± 0.15 W/m2 more than it emits into space (Hansen
et al. 2005). This increase, associated with global warming, is believed
to have been caused by the recent increase in greenhouse gas concentrations."
Hanson (1993) " The Earth must be in radiative (energy) balance within
a very small fraction of 1 W/m2 averaged over the current interglacial
period as well as during the peak of the last ice age 20,000 years ago."
What does this mean? It sounds like he is averaging over the 100k years
of an ice cycle, taking the mean value.
Ice core data (above)
min ice age temp -8 C to -10 C (relative to today)
Hansen uses 5C (looks like average of ice age temp cycles)
equates -5C with -6.6 w/m2 forcing (compare to 240 w/m2 baseline)
-6.6 w/m2 breaks down
-3.5 w/m2 albebo (only 1% change in albedo in ice age???)
- 2.6 w/m2 (greenhouse gases, 75-80% CO2)
- 0.5 w/m2 aerosols
Hansen's figure says 0.75C change for change of 1 w/m2
hansen text says, climate sensitivity is 3/4°C per W/m2, which
corresponds to 3±1°C for a doubled CO2 forcing of 4 W/m2 (I
don't understand this at all!)
------------------------------------------------
-- We have presented evidence (Hansen
et al. 1997) of a disequilibrium of at least 0.5 W/m2.
This disequilibrium could be measured as the sum of the rate of heat storage
in the ocean plus the net energy going into the melting of ice. (old
#'s, 1999)
-- Hansen: removing CO2, with water vapor kept fixed, would cool the Earth 5-10°C; removing CO2 and trace gases with water vapor allowed to respond would remove most of the natural greenhouse effect.
-- The key to understanding this apparent contradiction is to remember that we live at the bottom of the gaseous sea called ?atmosphere.? As far as the Earth?s radiation balance is concerned, the lower atmosphere and the surface of Earth form part of a ?warm interior? of the planet.
-- The relevant surface for re-radiation of heat that we see from space is located well above the real surface of the Earth where we live. The Earth?s heat radiation zone is centered about 5000 meters up (17,000 feet) within the atmosphere. To get a better handle on this concept consider the following: the difference in elevation between 0 meters and 5,000 meters corresponds to a difference in temperature of about 60°F
-- The black-body (radiative) surface lies 5000m up in the atmosphere from the land surface. In the past 100 years this surface has been rising.
-- Estimates of overall warming from a doubling of carbon dioxide fall largely within the range of between 1.5 and 4.5 degrees Celsius
-- The consensus, which is monitored and published by the Intergovernmental Panel on Climate Change, is that global average surface air temperature will rise by about 0.2° C per decade (if the carbon dioxide concentration continues rising at its current rate), global sea level will rise by about 0.2 to 0.8 meters over the next 100 years, and precipitation will increase over the high latitudes. The near-surface warming is expected to be greater over continents than over oceans.
-- Instead the CO2 molecule (just like all other molecules) is a Rayleigh (and Raman) scatterer with a larger scattering cross-section at IR wavelengths than N2 and O2.
Intesting climate sites (to review)
Skeptical
comments on modeling, uncertainity, etc.
http://www.climateaudit.org/?p=775
Lots of info
with skeptical bent. Good stuff on Medieval warm period.
http://www.co2science.org/scripts/CO2ScienceB2C/data/mwp/mwpp.jsp
References (check)
--Global Warming:
The Complete Briefing (Paperback) by John Houghton $44 Amazon (revised
2004, well reviewed) (can search inside) discusses energy balances. (I
ordered it)
Cess, R.D., and 29 others, 1993. Uncertainties in Carbon Dioxide Radiative Forcing in Atmospheric General Circulation Models. Science 262, 1252-1255
Kiehl, J.T. and R.E. Dickinson, 1987 Study of the Radiative Effects of Enhanced Atmospheric CO2 and CH4 on Early Earth Surface Temperatures. J. Geophys. Res.92 2991-2998
Myhre, G., E.J. Highwood, K. Shine and F. Stordal, 1998.
New estimates of radiative forcing due to well mixed greenhouse gases.
Geophys Res Letters 25 (14) 2715-2718
------------------------
(from Houghton's technical book on Atmospheric physics)
-- 20-60
km band (upper stratosphere & lower mesosphere) temp distribution determined
by balance between radiative cooling "from this CO2 band" and heating due
to solar absorption of ozone
-- CO2 cooling
(in band above) is independent of height
-- lower atmosphere
radiates like a black body because it is in local thermodynamical equilibrium
-- upper atmosphere
is not in local thermodynamical equilibrium
-- troposphere
is dominated by convection (because it has a steep unstable decrease in
temp with height
causing lower, warmer air to rise
-- stratosphere
is in approx radiative equilibrium and radiative transfer is dominant
(temp is constant with height)
-- radiative
time constant for atmosphere is 6 day (can be neglected for short term
changes, but in long term are dominant)
-- when a
planet first forms with no atmosphere the surfacecan be assumed to be in
radiative equilibrium. As outgassing occurs and water vapor begins to accumulate
in the forming atmosphere, the greenhouse effect begins causing a warmer
surface and more evaoporation. This process continues until atmosphere
become saturated with water vapor (water then condenses out (as rain) as
fast as it go in by evaporation).
-- on Venus
the saturation point was never reached.
-- 50% of
earth is cloud covered
-- clouds
reflect 50% (albedo = 0.5), transmit to surface 30% and absorb 20% of incident
solar radiation
** -- Read page 256 --- shows how more CO2
effect radiation from atmosphere!
radiation
from CO2 leaves atmosphere from statosphere and mesophere (see fig 12.7)
temp at 50km = 280K
double CO2 (in equation 4.26) then cooling rate (equation 4.27) increases
and temp at
50km decreases by 20C. Therefore the effect of more CO2 is to cool strasosphere
-- In troposphere
when CO2 is doubled the "origin of the radiation" is 3km higher, -18C cooler
(232k instead of 250K) (a drop roughly of 3w/m2 in notch)
------------------------------------------------------------
Informed skeptics
MIT's
Richard Lindzen --- Lindzen recently published a bombshell paper in the
Bulletin of the American Meteorological Society demonstrating there is
a huge tropical "thermostat" that regulates planetary warming. It reduces
the likely warming in the next century to, at warmest, somewhere around
1.6ºC, or the lowest end of the National Academy's range.
John ("Mike")
Wallace, who chairs the Atmospheric Science Department at University of
Washington
S. Fred Singer,
Professor emeritus of Environmental Sciences, University of Virginia
A list I found
(check out)
Robert White (former head of the US Weather Bureau)
Richard Lindzen (Prof. of Meteorology at the MIT)
Willie Soon (Harvard-Smithsonian Center for Astrophysics)
Sallie Baliunas (Harvard-Smithsonian Center for Astrophysics)
Robert Balling Jr. (Director of the Office of Climatology,
Prof. of Geography at Arizona State University)
Fred Singer (President of The Science & Environmental
Policy Project)
Zbigniew Jaworowski (Chair of the Scientific council
of the Warsaw Central Laboratory for Radiological Protection, CLOR)
Eric S. Posmentier (Department of Physics and Mathematics
at Long Island University, Brooklyn)
Michael Jorgensen (Paleoclimatologist)
Theodor Landscheidt (Schroeter Institute for Research
in Cycles of Solar Activity, Nova Scotia)
Frederick Seitz (Former president of the National Academy
of Sciences)
Robert E. Stevenson (Oceanographer, previously with the
ONR and Secretary General of The International Association for the Physical
Science of the Oceans)
Craig Idso (Center for the Study of carbon Dioxide and
Global Change, Arizona)
Sherwood Idso (Center for the Study of carbon Dioxide
and Global Change, Arizona)
David Legates (Center for Climactic Research, Delaware)
Chauncey Starr (Former Dean of Engineering at UCLA and
founder of EPRI)
Add to this
scientists who have reversed their originally pro-'global-warming' views,
such as:
Roger Revelle (Prof. of Ocean Science at Scripps Institute
of Oceanography)
Michael McElroy (Head of the Department of Earth and
Planetary Sciences at Harvard)
Positive climate feedbacks
water vapor-greenhouse
-snow-albedo
----------------------------------------------------
Petition Project
Not sure what
to make of this -- An anti-global warming petition (now with 10,000+ signatures,
mostly technical people) pushed by Frederick Seitz, Past President, National
Academy of Sciences, U.S.A.,President Emeritus, Rockefeller University
(pretty good credentials). But maybe 10 years old. Based on a 1998 paper
it says is peer reviewed, but no journal name is shown on paper.
From a 1998 (peer reviewed?) paper on this site -- http://www.oism.org/pproject/s33p36.htm
-- "The hypotheses that the IPCC has chosen to adopt predict that the effect of CO2 is amplified by the atmosphere (especially water vapor) to produce a large temperature increase (14). Other hypotheses, shown as hypothesis 2, predict the opposite that the atmospheric response will counteract the CO2 increase and result in insignificant changes in global temperature (25-27). The empirical evidence of figures 5-7 favors hypothesis 2. "
-- The global warming hypothesis is not based upon the radiative properties of the GHGs themselves. It is based entirely upon a small initial increase in temperature caused by GHGs and a large theoretical amplification of that temperature change. Any comparable temperature increase from another cause would produce the same outcome from the calculations.
-- The
CO2 greenhouse effect and the thermal history of the atmosphere, 1980,
Geophysics
Abstract (partial) --- The atmospheric absorption
of thick CO2 layers was determined in order to understand the thermal stability
on the earth's surface in spite of atmospheric composition changes (the
mean chemical composition changed from CO2 to O2 to N2 in the last 0.75
billion years). The calculated greenhouse effect was then combined with
other long-term phenomena which influenced the temperature. It was estimated
that the surface temperature increase did not reach the boiling
point of water for CO2 concentrations that were thousands of times larger
than the present concentrations; however, higher energy CO2 bands and/or
an increase in atmospheric H2O may have changed the greenhouse effect.
** -- In a sense, raising or lowering CO2 acted mainly as a throttle to raise or lower the really important greenhouse gas, H2O. (Arrhenius in 1896 included water vapor feedback in his model.)
-- Re: question of what happens to the heat abrobed by greenhouse gases --- quote from 'Sir' John Houghton: "Absorption and emission of radiation in the atmosphere ... can only occur effectively if the collisional deactivation time of the relevant molecular vibrations is substantially longer than the radiation lifetime."
-- As an IPCC lead author, I believe I have a responsibility to respond to serious questions about global warming. Dr. Hug seems to be genuinely puzzled at his results. Although I'm no expert on radiation transport, I did a little work on the subject ten years ago. As I recall, the value of ~4 Watts per square meter for doubled CO2 ultimately derives from a long series of laboratory measurements, e.g., as published by D. K. Edwards in the Journal of the Optical Society of America (v. 50, p. 617, June 1960), which were later fitted to empirical formulas by V. Ramanathan (Journal of Quantitative Spectroscopy and Radiative Transfer, v. 12, p. 933, May 1972; Journal of the Atmospheric Sciences, v. 33, p. 1330, 1976).
-- After dawn
there is warming of the surface which then emits more radiation as it is
warmer than the atmosphere. Some of that radiation is lost to space via
the infra-red window. Most of the emitted radiation is absorbed by the
lowest 100 metres of the atmosphere. The absorption causes the atmosphere
to become warmer. This occurs mainly by excited molecules colliding with
molecules of oxygen and nitrogen. (suspect source)
====================================================================
Greenhouse
HS talk (3/8/11)
Greenhouse Effect
----------------------------
Key numbers
Earth's radiative
balance
255K (-18C, 0F)
Earth's av
surface temp
288K (15C, 59F)
----------------
Actual greenhouse
effect temp rise
33K (33C, 59F)
Max greenhouse
rise
(100% absorbing 'thin' atmosphere)
48K (48C, 86F)
temp formulas
Kelvin used for absolute temp (0K = -273C)
Fahrenheit = (9/5) C + 32
----------------------------
Key to understanding the greenhouse effect
1) Atmosphere
absorbs much of the (infrared) radiation from the surface, radiating about
half out to space and half back to the surface.
2) Radiated
power from earth (or any blackbody) depends of the temperature to the 4th
power, or a 1% increase in temperature means a 4% increase in radiated
power.
Radiated power = k x T^4
where T is absolute temperature (0K = - 273C)
The combined effect of 1) and 2) is that the surface must radiate about x2 (twice) the power it would if earth had an atmosphere with no greenhouse gases, i.e. just nitrogen, oxygen and argon. The 4th root relation between temperature and power predicts the surface temperature must warm by [4th root of 2 = 1.189], and [18.9% x 255K = 48K (86F)].
In reality not all the surface radiated power is absorbed by the atmosphere, so the surface must radiate about x1.63 the power so the actual greenhouse temperature rise is reduced to 33K (59F), about 2/3rd of the ideal rise, since [4th root of 1.63 = 1.130]
Global warming
is a strengthening of the greenhouse effect. With more CO2 in the atmosphere
the range of frequencies absorbed by CO2 expands. The atmosphere becomes
a little more opaque to the earth's radiation. Over your lifetime
it is possible the greenhouse effect will strengthen by 7.5% (+/- 2.5%),
which means 0.075 x 33K (59F) = 2.5K (4.5F) warmer temperatures.
----------------------------------------------------------------
Figures of GreenHouse Power Point format
greenhouse_effect_HS_talk_fulton.ppt

Earth's surface is much warmer, by 33K (59 F), than
would be predicted from radiation physics
Why? Ans:
earth's atmosphere creates a greenhouse effect.
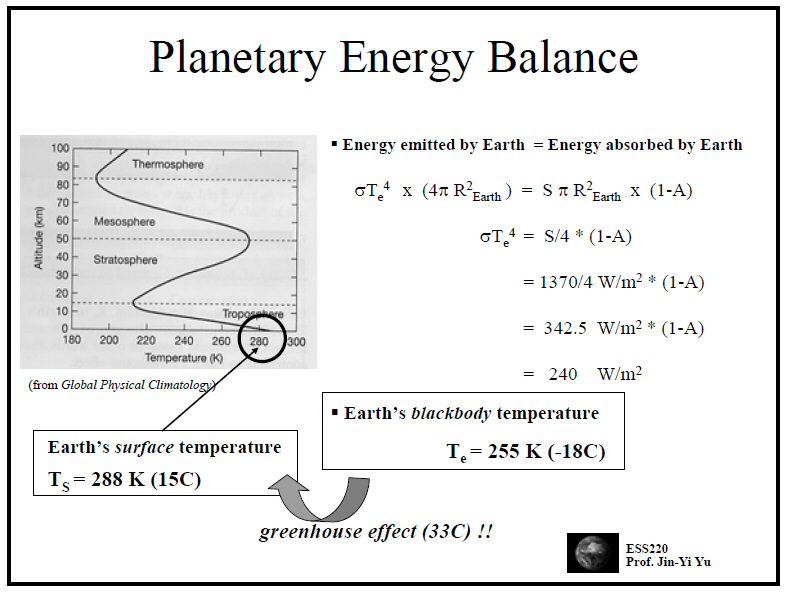
average earth temperature
no greenhouse -- 255K (0F)
with atmosphere greenhouse -- 288K (59F)
A (albedo) = 0.3 (30% of solar energy is reflected,
70% absorbed)
Earth's temperature (as seen from space) has long been
constant
The earth
is in thermal equilibrium. All the power the earth absorbs
from the sun (30% of solar power is reflected and can be ignored) is continually
radiated away to space. The earth radiates in the infrared, about 20 times
lower in frequency than sunlight, because the earth (as seen from space,
say the moon) is 20 times cooler than the sun's surface.
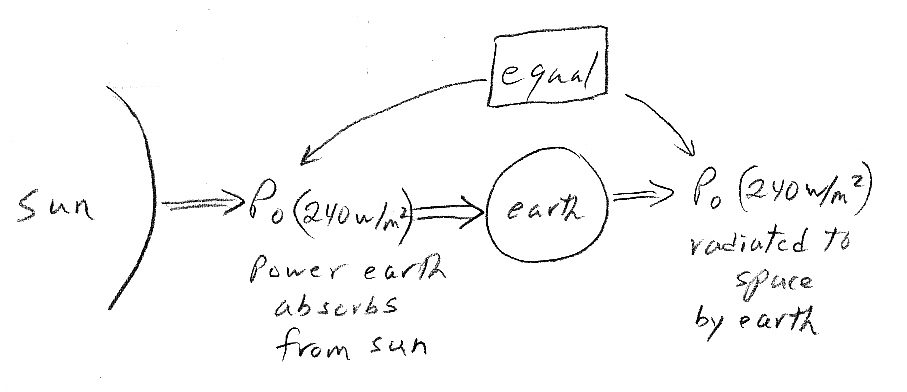
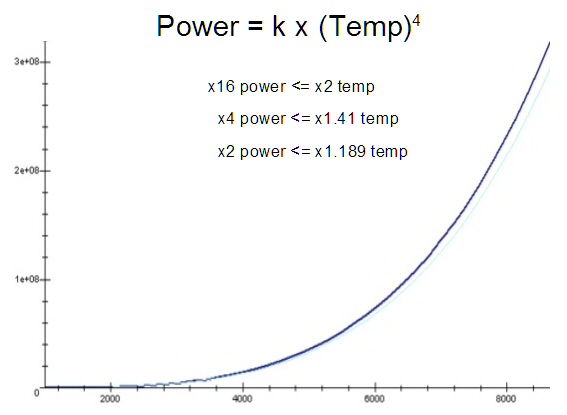
Power (black body radiated) vs Temperature (kelvin)
Power (radiated per unit area) varies as T^4
-- Stefan-Boltzmann law
Hor: x2 (4,300K to 8,600K)
Vert: x16 (1 unit to 16 unit)
(source --- Wikipedia 'Stefan–Boltzmann law')
Idealized atmosphere (totally opaque to infrared)
Simple model
(below) that demonstrates how the atmosphere raises the surface temperature.
Key --- Molecules of atmospheric greenhouse gases (H2O, CO2, Ch4) absorb outgoing infrared photons from the earth's surface and reradiate them in a 'random' direction. Since (compared to earth) the atmosphere is a thin shell this means that it radiates 'half' its power upward (to space) and 'half' downward (back to the surface).The resultant half up/half down radiation split by the atmosphere is that power the surface radiates has to double to force the right amount of power (240 w/m^2) to flow out into space. Since radiant power goes as 4th power of temperature (stefan boltzmann law P = k x T^4), power doubling means the (absolute) temperature of the surface must be hotter by 4th root of T [2^(0.25) = 1.189] or 18.9% This simple model a greenhouse rise of 18.9% of 255K (no atmosphere temperature) = 48K (86F), which is in the ballpark on the high side.

Shows in principle how atmosphere raises surface temperature
by radiating to space
only 'half' of the power it absorbs from surface.
The greenhouse effect of this simple model come out
a little high (48K vs actual 33K)
because the real atmosphere is neither thin, isothermal,
nor 100% opaque to infrared.
Notes
Greenhouse
gases asymmetrical
molecules with a dipole moment
are torqued by the IR E field
H2O
rotation mode absorbs IR
CO2
bending mode absorbs IR (15 um band)
Microwave
ovens
2.5 Ghz (0.12 meter wavelength) microwave region,
about 10,000 lower frequency than earth radiated IR

.
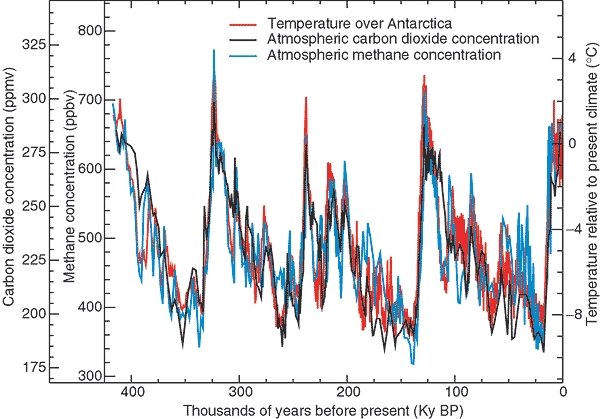
Atmospheric absorption spectra (H2O and CO2)
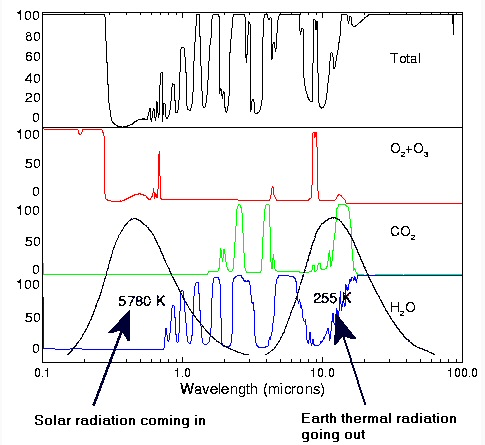
(source -- http://chriscolose.wordpress.com/2008/03/09/physics-of-the-greenhouse-effect-pt-1/)
Planetary greenhouse data
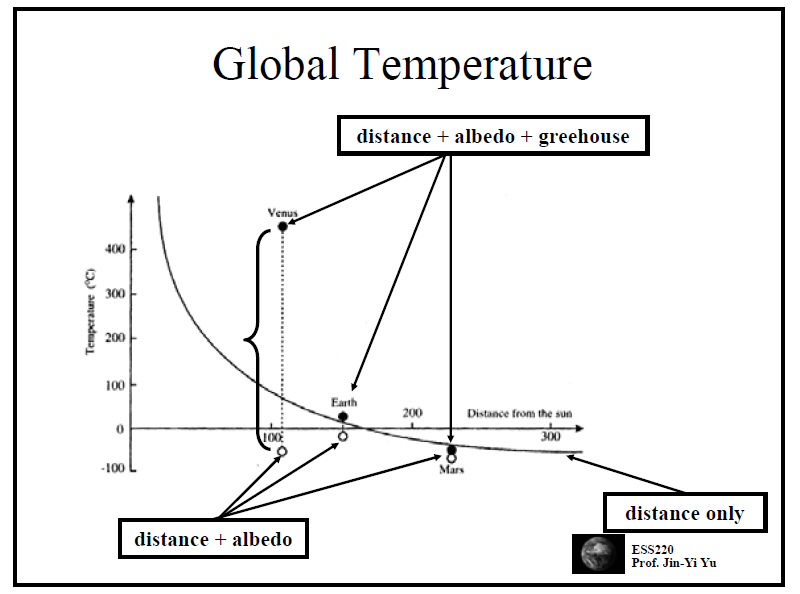
Sources Wikipedia (Idealized greenhouse model)
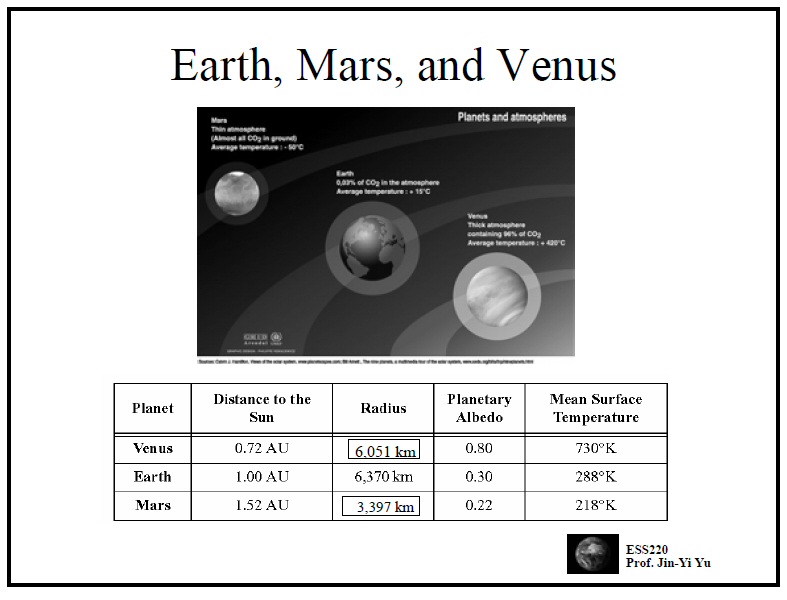
Where does 4th root come from?
Ans: Stefan Boltzmann law
Earth radiation
varies as the 4th power of absolute temperature from Stefan Boltzmann law
(figure below). Earth must radiate away (exactly) all the power it absorbs
from the sun, which on average is 240 watts for every square meter of earth's
surface. (Averaging smooths out effects of earth's rotation and latitude.)
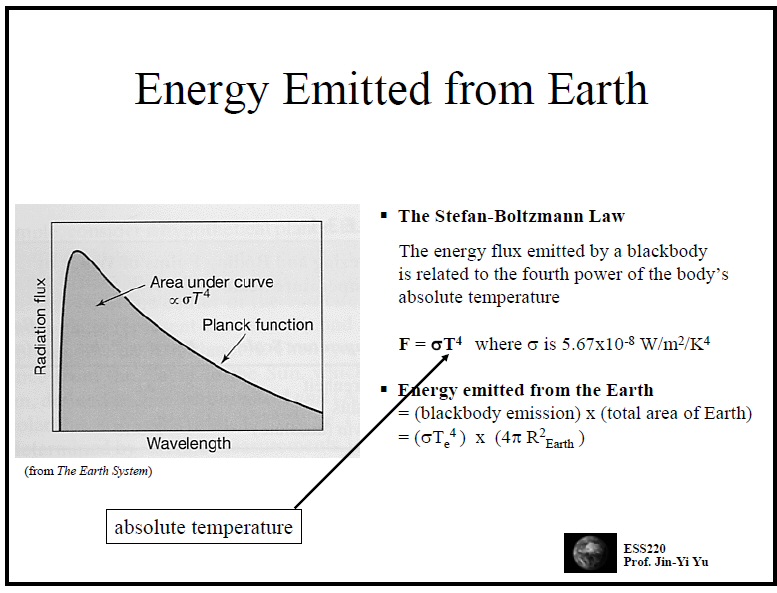
Radiated energy varies as (absolute) temperature to
the 4th power (Stefan-Boltmann law)
T (absolute or kelvin) = T(centagrade) + 273
Power radiated
to space from earth = [Stefan Boltzmann constant x T^4]
T(absolute) = 4th root {radiated power/Stefan Boltzmann constant}
= 4th root {240 (w/m^2)/5.67 x 10^-8 (w/m^2)}K
= 255K (kelvin)
Radiation physics
The effective
(technically black body) temperature of the sun is pretty close to x20
times higher than the earth's temperature (5,800 K vs 290 K). Via Wein's
law this makes the wavelengths of the earth's radiation x20 times longer
than those of the sun. The sun's energy is mostly in the visible range
of frequencies whereas the radiation from the earth is x20 lower in frequency,
mostly in the deep infrared (invisible to human).
A key fact that makes the greenhouse work is that 'all' the gases of the atmosphere are virtually transparent to solar frequencies, so almost none of the incoming solar power is absorbed. (However, 30% is reflected by the atmosphere, clouds and the surface.) But greenhouse gases in the atmosphere (not the main atmospheric gases: O2, H2 and Ar) are opaque (absorb) over a substantial fraction of the infrared frequencies where the earth strongly radiates. Hence the atmosphere absorbs a substantial fraction (80-90%) of the earth's surface outgoing radiant power, and its molecules reradiate it random directions, which results in half the outgoing power going to space and half back to the surface.

Earth's temperature is x20 lower than sun (surface)
temperature
Atmosphere is transparent to visible light,
but at x20 lower freq (in infrared) the atmosphere
is pretty opaque
Idealized greenhouse (ratio) model (100 = sun power
@ earth)
1)
No atmosphere
2) Idealized atmosphere (totally opaque to infrared)
3) More realistic atmosphere (390 ppm CO2, 2011)
4) Atmosphere tweaked for higher CO2 (560 ppm CO2)
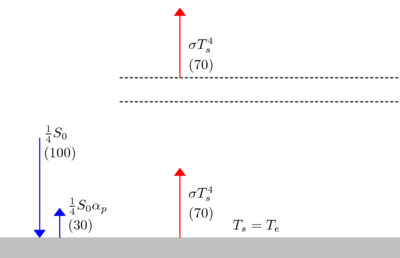
earth with no atmosphere (no greenhouse)
Ts = 255K @ radiative balance (albedo = 0.3)
More realistic atmosphere (390 ppm CO2, 2011)
Reducing the
atmospheric infrared absorption from 100% to 78% gives the correct greenhouse
temperature rise of 33K (59F). (Note, this model of the atmosphere is still
idealized because it assumes the atmosphere is thin and isothermal, neither
of which is true.)
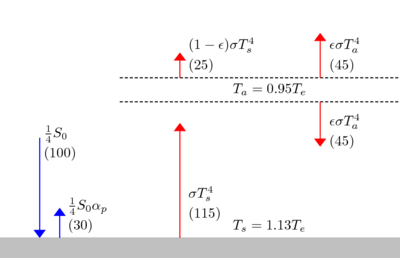
earth with (idealized, thin, isothermal) atmosphere
greenhouse
atmosphere absorbs 78% =(90/115) of surface radiation
Surface radiated power increased by (115/70) = 1.64
Ts = 4th root{1.64} x 255K (0F)
Ts = 1.13 x 255K = 288K (59F)
Ts = 288K (actual earth surface temperature)
Atmosphere tweaked for higher CO2 (560 ppm, twice the
pre-industrial baseline of 280 ppm)
Below tweaks
the model above to get an added 2.5K (4.5F) added greenhouse rise, roughly
equivalent to increasing CO2 to 560 ppm (vs 390 ppm today). In this simple
model raising absorption of the atmosphere from 78% to 82% raises the greenhouse
rise from 13% to 14% of 255K (an added 1% of 255K, or 2.5K).
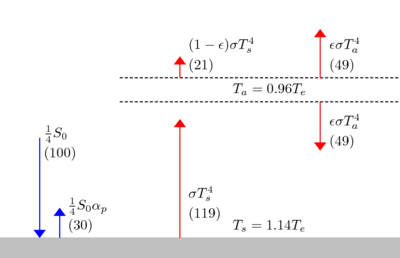
x2 CO2 (280 ppm => 560 ppm)
atmosphere absorbs 82% =(98/119) of surface radiation
Surface radiated power increased by (119/70) = 1.70
Ts = 4th root{1.70} x 255K
Ts = 1.14 x 255K = 288K + 2.5K (4.5F)
Approx: 7% strengthing of greenhouse effect
and 1% increase in earth's absolute temperature