My plot of electron potential energy & kinetic
energy vs orbital radius
Size of an electron
Derivation of Bohr
radius
Minimum
energy radius
Fine structure constant
-------------------------------------------------------------------------
Reference
1 )
wavelength = h/p
where p= Mv = mv/sqrt{1-(v/c)^2}
2)
delta pos > (hbar/2)/delta p or
delta E x delta t > hbar/2
3)
E = h x freq
4)
alpha = e^2/(4 pi e0 hbar c) = e^2/(2 e0 hc) = 1/137.036
5)
hbar = h/2pi = 1.0546 x 10^-34 J-sec
6)
m = 9.109 x 10^-31 kg
7)
e = 1.6022 x 10^-19 coulomb
8)
e0 = 8.8542 x 10^-12
-------------------------------------------------------------------------
An essay on
the electron?
Of the elementary particles that make up ordinary matter the electron is the simplest. It has no internal structure and is totally stable. This is unlike protons and neutrons, both which are made of up & down quarks, and unlike free neutrons, which are unstable, decaying in 15 minutes. It is characterized by only a few numbers (charge, mass and magnetic moment), the values of which are known to 9 or 10 decimal places. It was the first of the elementary particles to be experimentally discovered, in 1897 by J.J. Thompson.
The electron has an anti-particle, called the positron, which has the same properties as the electron except its charge is reversed. Positrons exist in the real world because they are emitted by radioactive isotopes that decay via positive beta decay. The positrons created in the body (from radioactive sugars incorporating fluorine 18, which are used in 'positron emission tomography') typically travel a few mm before meeting an electron and decaying to a pair of 0.511 Mev gamma rays. Two heavier, highly unstable, so-called 2nd & 3rd generation versions of the electron also exist: muon (x 207 heavier) and the tau lepton (x 3,477 heavier). Muons exist in the natural world because they are continually created in the upper atmosphere when air molecules are hit by high energy cosmic rays (high energy protons from space).
How big?
One
day I stumbled across the curious fact that the electron has different
sizes, and they are ratioed by the 'mysterious' (Feynman's word) constant
known as the 'fine structure constant'. I was sucked in and wanted
to know more. I am now reading Feynman's book for the second time and have
worked to integrate the random facts picked up on Wikipedia and HyperPhysics
sites.
The electron is an interesting beast. It can be approached classically (as it was historically) and by quantum physics. It can be floating free in space, bound to a single atom, or bound to a group of atoms. Virtually all light/heat photons go into, and come out of, electrons (QED teaches that any charged particle interacts with photons, so photons go into and come out of protons too), so the electron/photon theory QED (Quantum ElectroDynamics) explains how light works and how the world looks. Properties of electrons determine how they arrange themselves in atoms, so understanding this means an understanding of atoms and chemistry.
An electron 'fuzzes out' in space. How much fuzzing depends inversely on how accurately the momentum is known. My understanding is that velocity (momentum) of an electron in an atom is set equal to the uncertainty in velocity, which is calculated from the Heisenberg uncertainty principle given the dimensions of the atom. Since the (DeBroglie) wavelength of the electron (& all other particles) is planck's constant over momentum (h/p), it turns out that the fuzzed out (radial) width of an electron in atoms is a just a fraction (1/pi) of the electron DeBroglie wavelength.
Many properties of the electron are intertwined with a fundamental, dimensionless constant known as the 'fine structure constant'. It shows up in sizes of the electron, in the quantum mechanical 'amplitude' that an electron will emit or absorb a photon, in the charge of the electron, and the speed of electrons in atomic orbits..
History
As best as
I can piece it together, the history of the fine structure constant is
this. Bohr's electron orbits in atoms were circular (at specific integer
related radii). They were not (really) 'like planets' as is often said,
because all planetary orbits are (to some degree) elliptical.
Sommerfeld (in Germany) in 1916 extended Bohr's model by allowing the electron to move in added ways, such as elliptical orbits, and included in his analysis the relativistic dependence of mass on velocity. The equations derived by Sommerfeld (apparently) included a dimensionless term {alpha = e^2/2 e0 hc}. Sommerfeld found that the speed of the electrons on the inner circular Bohr orbit equaled (alpha x c), a little less than 1% the speed of light. And (apparently) this is the first time this term was seen in physics. This term together with ??? was able to explain the fine splitting of spectral lines (known before Sommerfield?), providing strong evidence that Sommerfield's orbital variations were probably real. It also, of course, explains the name given the term: 'fine structure constant'.
In 1928 Dirac's came up with a new relativistic theory of the electron replacing Bohr's. The fine structure constant showed up in Dirac's theory too.
Size of an electron
The size of
an electron is interesting. There are several possible way to figure the
electron's size, and they all give substantially different values. Curiously
many of the electron sizes are ratioed by a dimensionless fundamental physical
constant called the 'fine structure constant', which (in some sense) characterizes
the strength of the electromagnetic interaction. High energy tests on the
electron do not see any inside structure so physicist consider it a point
particle, therefore one of its possible radii is zero. (Of course, zero
might 'really' be planck length (1.6 x 10^-35 m), since many particle physicists
suspect this is the smallest possible length.)
In the table
below I scale up the 'classical electron radius' by the inverse of the
'fine structure constant' (x137) to get a series of radii, each bigger
by about two orders of magnitude.
|
|
ference |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
electron radius or (equiv) energy required to move in a test charge to classical electron radius = rest mass energy of electron (0.511 Mev) vel = 0.99995 c
|
|
|
|
|
|
rest mass energy (0.511 Mev) NIST calls this 'Compton wavelength
vel = 0.707 c
|
|
|
|
10^-12 m |
|
|
|
Compton wavelength (hc/wavelength) = rest mass energy of electron (0.511 Mev) relative uncertainty = 1.4 x 10^-9 |
|
|
|
|
|
|
hydrogen atom (peak of the radiual probability distribution) vel = alpha x c
|
|
|
|
|
sqrt{hbar/eB} |
a strong magnetic field (12.5 tesla) |
|
|
|
|
|
|
|
|
Looking at the options the Compton radius seems to make the most sense for 3.86 x 10^-12 m and Compton wavelength for its associated circumference 2.42 x 10^-12 m.
Radius comparison
Any one of
these three lengths can be written in terms of any other using the (dimensionless)
fine structure constant (alpha = e^2/(2 e0 hc) = 1/137.036.
* Bohr radius
is built from (m,e,h) ---- classical + quantum
The (actual) volume of space occupied by an electron in a hydrogen atom
(or any one
electron atom). It is the classical concepts of electrostatic attraction
and energy (PE and KE)
combined with quantum uncertainty of velocity resulting in quantized orbits.
* Compton radius
(wavelength) is built from (m,c,h) -- relativity + quantum
An (idealized) radius derived from the compton wavelength. This is
the electron's
de Broglie wavelength as it transitions to relativistic speed (v = 0.707
c) and is
important in electron - photon interactions. It is a triple energy point
where photons
with this wavelength have energy equal to both the electron's energy
assymptote (cp)
and rest mass energy.
* Classical
electron radius is built from (m,e,c) --- classical + relativity
An (idealized) radius that ignores quantum considerations. It is the size
of a charge sphere
where the energy stored in the (external) electric field becomes equal
to the electron's
rest mass energy.
Link below has info on four length scales: Bohr radius, compton length, classical electron radius (all separated by 137, the (inverse of) fine scale constant)
http://math.ucr.edu/home/baez/lengths.html
Derivation
of Bohr radius
In college
notes (102 slides from Univ of Arkansas) I found the derivation below of
the Bohr orbit. It gives the correct answer, but initially I didn't understand
why it worked. Later as I explored this approach I found it is quite powerful.
By simply combining heisenberg uncertainty with the classical concepts
of KE and PE you can not only calculate the Bohr orbit, but also get an
answer (based on heisenberg) to the classic question: Why doesn't the
electron fall into the proton?.
Falling into a coulomb energy well potential energy (PE), taken as zero outside, goes down as (1/r). The PE at a given 'r' can be found by calculating the work (force x distance) done on a test charge falling in, i.e. by integrating coulomb F dr = e^2 dr/(4 pi e0 r^2 from r to infinity. Kinetic energy (KE) as a function of r is found by using Heisenberg uncertainty setting the p = delta p and treating delta r as r. (The college notes use (delta p x delta r = hbar), which seems like a fudge of 4 because the usual formula is hbar/2 and the uncertainty in position is 2r, but maybe this is due to SD definitions).
PE = - e^2/(4 pi e0 r)
KE = p^2/2m
= (hbar/r)^2/2m
E = KE + PE
= (hbar/r)^2/2m - e^2/(4 pi e0 r)
= hbar^2/2mr^2 - e^2/(4 pi e0 r)
At large r energy is zero. Coming in from infinity |PE| increases first so energy goes negative, then KE kicks in and energy goes positive. Surprisingly the college notes find the Bohr radius by finding the radius where the slope of E (vs r) is zero. It turns out that this is x2 the radius where |PE| = KE (where E =0).
dE/dr = 0 = - 2hbar^2/(2mr^3) + e^2/(4 pi e0 r^2)
2hbar^2/(2mr^3) = e^2/(4 pi e0 r^2)
hbar^2/(mr) = e^2/(4 pi e0)
r = (4 pi e0)hbar^2/me^2
Bohr radius
= 12.56 x 8.85 x 10^-12 x (1.054 x 10^-34)^2/9.1 x 10^-31 x (1.6 x 10^-19)^2
= 5.30 x 10^-80/10^-69
= 5.30 x 10^-11 m
(5.292 x 10^-11 m ) OK
Why does this work?
Elsewhere
I calculate that an electron falling into a proton well ends up converting
half the lost PE to KE with the atom dissipating the other half of lost
PE as heat. (Chemistry in action --- when electrons move in closer to the
nucleus, heat is released.) So how at the Bohr radius does KE compare to
PE?
KE/PE = [hbar^2/2mr^2]/]e^2/(4 pi e0 r)]
= [hbar^2/2mr]/]e^2/(4 pi e0)]
= (1/r) x [hbar^2(4 pi e0)/2me^2]
= [me^2/(4 pi e0)hbar^2] x [hbar^2(4 pi e0)/2me^2]
= (1/2)
OK
So (apparently) the Bohr radius calculation can be done by finding r where KE = (1/2)|PE|. Let's check
KE = (1/2)|PE|
(hbar/r)^2/2m = (1/2)e^2/(4 pi e0 r)
(hbar^2/r)/m = e^2/(4 pi e0)
r = (4 pi e0) hbar^2/me^2
yup, Bohr radius
= (4 pi e0) hbar^2/mZe^2
(one electron Z atom)
But wait a minute -- In the second hydrogen orbit (n =2) velocity is half, so KE is down by 4, and the radius is up by 4, meaning PE is also down by 4, so the formula [KE = (1/2)|PE|] is still satisfied, but heisenberg is not satisfied! The reason the above formula yields the Bohr radius (that it works) is that the velocity plugged into the KE formula is derived from heisenberg uncertainty. This is the key.
Energy released as proton captures electron to form Bohr atom is (1/2) |PE|.
energy release = (1/2) |PE|
= (1/2) e^2/(4 pi e0) x [1/r]
= (1/2) e^2/(4 pi e0) x [me^2 /(4 pi e0) hbar^2]
= (1/2) me^4/(4 pi e0 hbar)^2
= (1/2) m(Z^2)e^4/(4 pi e0 hbar)^2
(one electron Z atom)
= (1/2) 9.11 x 10^-31 x (1.6 x 10^-19)^4/(4 pi 8.85 x 10^-12 1.055 x 10^-34)^2
= (29.85 x 10^-107/(117.3 x 10^-46)^2
= (29.85 x 10^-107/(1.376 x 10^-88) x [1 ev/1.6 x 10^-19]
= 21.7 x 10^-19/1.6 x 10^-19
= 13.6 ev
So why don't electrons in atoms radiate?
Bohr simply
postulated that electrons in atoms don't radiate (even though the electrons
are (apparently) in some way orbiting the nucleus, which means they are
being accelerated.) So what is the quantum explanation for this? (Also
consider that 'free' electrons that are accelerated or vibrating do radiate.)
I suppose the following argument could be made:
For an electron in a minimum orbit to radiate it would need to give up some of its kinetic energy and /or sink lower to make available potential energy, but orbits smaller than minimum aren't possible because heisenberg requires velocities go up as (1/r), meaning KE goes up as (1/r^2), but PE is only released as (1/r).Clear criteria for Bohr radius calculationIn other words below minimum orbits heisenberg requires more KE than is available from PE. This means that electrons in a (ground state) atom are in a minimum energy state, and therefore unable to radiate!. Do references ever say this? Check this out.
Find r where the release of PE by sinking (slightly) lower is just enough to provide the energy needed for the higher KE required by heisenberg. In other words an electron being captured by bare proton to make a hydrogen atom cannot fall below the radius where a further (incremental) fall will not release enough PE to provide the (incremental) increase in KE that heisenberg requires. Above the Bohr radius more inward fall is possible because more than enough PE is made available to provide for added KE with the excess energy being radiated away or (somehow) released by the atom as heat.Mathematically Bohr radius criteria is below. Note this is equivalent to the college note derivation above where E = KE + PE was differentiated and nulled.
- d PE/dr = d KE/dr
Minimum energy
radius
Below I plot
up the (heisenberg) KE, (classical) PE and total E vs radius for hydrogen
atom. It shows that the criteria above for the Bohr orbit is equivalent
to finding the radius where the 'total energy is minimum'. (Note this is
not
the radius where the magnitude of potential energy = kinetic energy.) That
explains why in the college notes they solved for zero slope of energy,
they were finding the energy minimum.

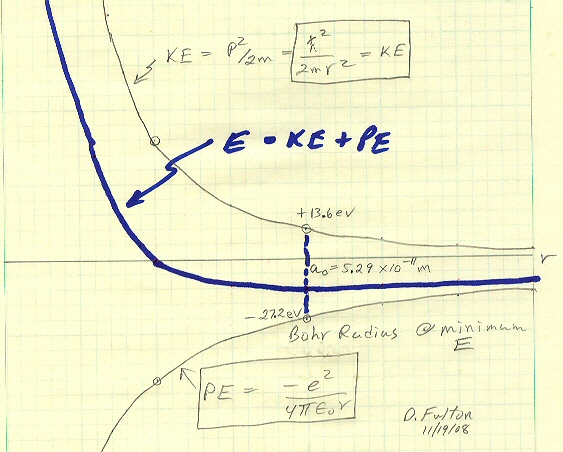
My plot of electron potential energy & kinetic
energy vs orbital radius
The potential energy an electron has is found by integrating the attractive force between the electron or proton (nucleus), which since the force varies a k/r^2, then the potential energy varies as -k/r. The potential energy 'captured' by the electron as it falls into the proton is negative, because the zero reference is at infinite distance.
The electron kinetic energy rising as 1/r^2 can be figured using Heisenberg uncertainty (delta p) x (delta r) > hbar (or hbar/2). Notice Heisenberg tells us that if we specify ('know') that the electron is within a specific radial fuzz zone, then ['v' x 'r' = constant], so 'v' must vary as 1/r, which means electron's kinetic energy must vary as 1/r^2. Therefore at large 'r' the total energy follows the potential energy and heads negative, but at some point the kinetic energy increases begin to dominate. The Bohr orbit corresponds to the electron settling out at the minimum energy of the system, where the total energy curve flattens out, where the incremental kinetic energy increases equal the incremental decreases in potential energy.
Note --- In all orbits in force varies as 1/r^2 systems (atoms and planets) the potential energy is always -2x kinetic energy, i.e. PE = -2KE (general rule).
E = KE + PE = KE - 2KE = -KE
This explains why electrons in higher orbital have higher energy. It is true that electrons in higher orbitals move slower and so have lower kinetic energy, but in higher orbitals the x2 larger potential energy is less negative with the result that total energy rises as the orbitals get larger.
Astronomical data -- 91.2 nm radiation
I searched
for info on the 'how' excess PE of 13.6 ev (when a proton captures an electron)
is radiated. I found in a book on stellar evolution that some types of
compact stars release copious quantities of radiation at 91.2 nm (13.6ev)
"created by the recombination of hydrogen ions". When 13.6 ev radiation
line is redshifted down to 10.2 ev (3/4 x 13.6ev) at 0.26c, these photons
are absorbed by (neutral) hydrogen atoms in jets adding energy to the jets
in a mechanism called 'line locking mechanism'.
Wikipedia (Nuclear Systematics) points out that the 13.6 ev released when a hydrogen atom forms is about 1 part in 10^8 of the inertia mass/energy of the system (about 1,000 Mev). And they add that in the typical chemical reaction the energy release per 'molecular process' is about 1 ev.
Source of the cosmic background radiation
Even more
interesting (from Wikipedia) is that the photons emitted about 240,000
years after the big bang by electrons combining with protons to form hydrogen
and helium, in a process called 'recombination', are the photons we detect
today (redshifted) as cosmic background radiation.
Where does [E = h x freq] come from?
I never
knew the history of where the photon energy relation [E = h x freq] come
from, so here is a review from Wikipedia (Plank's constant). [E = h x freq]
was figured out by Plank and is called the Plank relation. Working on black
body radiation (around 1900) modeled as coming from model (large number)
of harmonic oscillators he tried the formal assumption that their energy
had to be a multiple of some very small quantity (later called Plank's
const 6.6 x 10^-34 joule-sec).
Matching his model to know formulas for black body radiation (Stefan Boltzmann law and Wien's displacement law) it fell out that [E = h x freq] energy in light increases linearly with frequency with 'h' being the proportionality constant. Not only that but matching his quantized (linear with frequency) energy formula to known black body data he was able to get a value for Planks constant with about 2% of the current value.
Summarizing, about 1900 Plank finds with the assumptions that:
a) light energy is quantized and
b) light energy rises linearly with frequency [E = h x freq],
where
h = 6.6 x 10^-34 joule-sec
then he can model black body radiation.
Ultraviolet catastrophe
Unlike what
I have been thinking it wasn't until a little later (1905) that Reyleigh
and Einstein proved that classical electromagnetism could never
explain the spectrum of black body radiation. It was these proofs that
were called the 'ultraviolet catastrophe', and along with the photoelectric
effect it helped convince people that Plank's quantized formalism
probably reflected some sort of underlying reality. So it's still true
to say that Plank solved the 'ultraviolet catastrophe', even if the time
order is reversed!
Wikipedia points out there was other relevant data besides the black body data. Maxwell's equations predicted the energy in light should be a function only of its amplitude and independent of its frequency. But not only did the photoelectric effect (popping electrons off metals with light) show that frequency had a sharp threshold effect, but there were certain chemical reactions that worked the same way. The light required to trigger the reactions had to be above a threshold frequency.
Helium ionization
The Bohr equivalent
(one electron) helium atom (He+) has Z=2. This doubles the attractive force
and halves the radius, so all the energy numbers are x4, making the energy
release of an electron falling in to create He+ [13.6 ev x 4 = 54.4 ev]
equivalent to a wavelength [91.2 nm/4 = 22.8 nm]. These number are confirmed
in a textbook found on Amazon ('Physics and Chemistry of the Solar System').
The '2nd ionization' level of helium, which is the energy to remove a 2nd
electron from singly ionized atom, is also 54.4 ev.
The '1st ionization' level, which is removing one electron from a neutral atom, of helium is 24.59 ev. This value can be explained this way: if one electron was (close to) 100% effective at screening the nucleus, then a 2nd electron coming in (or going out) would see an effective Z of one, but the radius of the orbit is still half the size of hydrogen, so the ionization level would be expected to be about double hydrogen [13.6 ev (hydrogen) x 2 (half size radius) = 27.2 ev]. ( In a quantum physics textbook I found there is no closed quantum solution for helium ionization, all calculations are approximate.)
Ionization and orbits
The
1st ionization level of most elements is between 4 ev and 13 ev with noble
gases generally a little higher. Helium's 1st ionization level of 24.59
ev is the highest of any element. (Helium's 2nd ionization level of 54.4
ev is the highest too.) What does this tell us about outer orbits? A lot.
The 1st ionization
levels of most elements falls in narrow range that is little less than
hydrogen, so that must mean
a) Effective charge seen by the last incoming (or outgoing) electron
must be (about) Z-1. The electron cloud must be pretty effective screening
the nucleus such that the last electron 'sees' a charge that about the
same as hydrogen.
b) Outer orbits of most elements are probably just a little larger than
hydrogen's ground state orbit, because their ionization energies are just
a little lower than hydrogen's 13.6 ev. This is consistent with the fact
that the 'size' of most element atoms falls in a narrow range with hydrogen
the smallest.
I see references that say the ionization of noble gases is high because the 'shell is full', but if you look at atom size, you see than noble gases sizes are a little smaller (than nearby elements) with helium the smallest. I think it very likely that the real reason the ionization levels of full shell atoms is a little higher is that their outer orbits are a little closer to the nucleus while the effective charge seen by the last electron remains at Z-1.
ev <=> kj/mole
Chemists are
fond of specifying bond energies in terms of measurable quantities. For
electrons this is kj for a mole of electrons. Ionization energy of hydrogen
(13.6 ev) is 1,312.0 kj/mole, which is about x100 the ionization energy
in ev. The exact conversion factor is:
[kj/mole]/ev = 96.48
Check
A mole of
carbon is 12 grams, because the atomic weight of carbon is 12. The number
of atoms in a mole of any element is Avagardo's number (6.022 x 10^23),
so (I suppose) by extension a mole of electrons is also Avagardo's number.
Let's check
6.02 x 10^23 x 13.6 ev x [1.602 x 10^-19/1 ev] = 131.2 x 10^4 joule
= 1,312 k joule
checks
-----------------------------------------------------
Comparing the electron radii
Bohr radius (5.3 x 10^-11 m)
The Bohr radius
is the actual volume of space occupied by an electron in a hydrogen
atom (or any one electron atom). Its basis is the classical concepts of
electrostatic attraction and energy (PE and KE) combined with quantum uncertainty
of velocity resulting in quantized orbits.
Since the Bohr radius really is the peak of the electron charge density in the hydrogen atom as calculated by the Schrodinger wave equation and measured in experiments, isn't this really the size of the electron, or at least the size of an electron in a atom? Answer: No, it isn't this simple.
It's true that the Bohr radius (5.3 x 10^-11 m) is the (radial) size of an electron in hydrogen (in ground state), where, of course, the nucleus has one proton. But if you take a uranium atom, which has 92 protons in the nucleus, and strip away all the electrons but one, this 'high Z one electron atom' is also solvable using the Schrodinger wave equation, and the result is that the remaining electron orbits 92 times closer to the nucleus! Since the electron's (radial) location is more tightly determined, Heisenberg uncertainty forces the momentum (& energy) upward, thus reducing its DeBroglie wavelength (wavelength = h/p).
In other words an electron orbiting a uranium (Z=92) nucleus orbits x92 times closer than in hydrogen. With its linear dimension shrunken by x92 an electron bound to uranium occupies a volume of space almost a million times smaller than it does in hydrogen! In fact the electron radius in this one electron uranium atom is so shrunken that it's pretty close to the Compton radius (3.9 x 10^-13 m), and with x92 times higher momentum than in hydrogen (most of which increases the velocity) the velocity is pushing close to 0.707 c. With its x92 higher momentum its DeBroglie wavelength (h/p) is x92 smaller, so (in the ground state) one wavelength still fits around the nucleus.
The radius of the inner (two) electrons in normal uranium atom cannot be calculated exactly, but they are probably not too different from the 'one electron' version, which can be calculated. In other words as one goes up the periodic chart the 'size' of the electrons in the inner orbit (1s orbit) shrink, or if you will they are less spread out, by approx 1/(atomic number).
The kinetic energy of the single electron in hydrogen (in the ground state) at the Bohr radius is 13.6 ev (calculated below). In the 'single electron uranium atom' the velocity is (approx) x92 higher, so the electron kinetic energy is 13.6 ev x (92)^2 = 115,000 ev, which is closing in on the 511,000 ev where the kinetic energy equals the rest mass (m c^2) of the electron.
Compton radius (3.86 x 10^-13 m)
An (idealized)
radius derived from the compton wavelength. The compton wavelength is the
electron's de Broglie wavelength as it transitions to relativistic speed
(v = 0.707 c) and is important in electron - photon interactions. It is
a triple energy point where photons with this wavelength have energy equal
to both the electron's energy assymptote (cp) and rest mass
energy.
photon
E = h x freq = h x (c/wavelength)
wavelength = hc/E
electron
@ v = 0.707 c cp = cMv
= mc^2
wavelength = h/p = hc/cp = hc/mc^2 = hc/E rest mass
= h/mc
compton
radius = wavelength/2 pi = hbar/mc
= 1.055 x 10^-34 joule sec/(9.11 x 10^-31 x 3 x 10^8)
= 3.86 x 10^-13 m
The de Broglie wavelength of a particle [wavelength = h/p = h/Mv], where M is relativistic mass. A particle's wavelength can go down without limit as momentum (& energy) go higher and higher. (Does this mean in some sense an electron gets smaller as it moves faster?) At speeds below 0.7070c momentum can be considered classical, but above 0.707c momentum becomes relativistic with speed leveling out (close to) c and momentum increases due to relativistic mass (M) increases.
Thus the compton wavelength of an electron is its de Broglie wavelength as the particle approaches c and starts to become relativistic. This is at a speed of 0.707c, where momentum transitions from classical to relativistic.
compton wavelength = de Broglie wavelength @ v = 0.707c
= h/Mv
= h/1.41m x 0.707c
= h/mc
From another point of view the compton radius is just an idealized radius where Heisenberg uncertainty pushes the electron's kinetic energy up to equal its rest mass energy (mc^2 = 511,000 ev). As shown in my hand drawn sketch below, the kinetic energy equals the rest mass energy when the electron velocity is 0.707 c. Electrons in the inner orbit of uranium (element #92) move nearly this fast. Scaling from the Bohr analysis the average speed of uranium inner electrons is about 2/3rd the speed of light [92 x (1/137 c = 0.67 c].
Wikipedia makes the point that the compton wavelength sets (sort of) a fundamental limit (order of magnitude) on determination of position. To determine the position of a particle to a limit you need a photon with a wavelength of comparable (or shorter) wavelength. But when the photon energy becomes equal to (or greater) than the particle rest mass energy, there is the possibility that in the process of 'observing' the particle (hitting it with a photon) that a new particle will be created. One reference puts it this way:
"Trying to localize an electron to within less than its Compton wavelength makes its momentum so uncertain that it can have an energy large enough to make an extra electron-positron pair! This is the length scale at which quantum field theory, which describes particle creation, becomes REALLY important for describing electrons. The Compton wavelength of the electron is the characteristic length scale of QED (quantum electrodynamics)."Seems to me there are two different perspectives here. One is just on the photon. There is a natural break point where the energy (wavelength) of a photon equals (oris it double?) the rest mass energy of a particle. For the electron this is
The second focus is just on the electron and its energy due to heisenberg uncertainty. Compare the de Broglie wavelength formula with the heisenberg uncertainty formula:
wavelength = h/p
de Broglie
delta x > (hbar/2)/delta p
heisenberg
Interesting. We know for a moving particle the de Broglie wavelength @ v = 0.707c is the compton wavelength and the kinetic energy asymptote = mc^2. From the heisenberg formula we can that a non-moving particle if confined to a space where the velocity uncertainty is pushed up to 70.7% c , then that size uncertainty must be (order of magnitude) the compton wavelength (or compton radius) and the particle kinetic energy must equal its rest mass energy.
So if it's two different effects, how come the wavelengths come out to be the same? It's because the energies are the same (see above):
photon
wavelength = hc/E
electron
wavelength = hc/pc = hc/Ekinetic asymptote
The photon and electron formula in terms of the 'right' energy are similar, and this is what makes the wavelengths the same. And importantly from a photon-electron interaction view point at the compton wavelength both energies are equal to to the electron rest mass energy (mc^2), which means at these energies (& higher) only QED analysis really works.
Note the compton length scale where QED cuts in is about two orders of magnitude (alpha = 1/137) smaller than the size of the hydrogen atom (& all atoms), so that's why Bohr analysis (classical concepts mixed with heisenberg uncertainty) give generally correct answers.
Classical electron radius (2.8 x 10^-15 m)
This is an
even more idealized electron radius. This calculation totally ignores the
momentum and energy increases caused by Heisenberg uncertainty, which are
huge, and just classically calculates the radius of the electron where
the energy stored in the electric field equals the electron rest mass energy.
As the radius of a shell of charge decreases, the energy in the electric
field goes up. This is because as the radius drops the electrical energy
density rises faster (1/r^4) than the volume decreases (r^3) resulting
in the total energy stored in the field rising as 1/radius.
When quantum mechanical considerations are considered (meaning Heisenberg uncertainty), decreasing the radius x137 (1/alpha) from the Compton radius pushes up momentum x137. Since the electron velocity at the Compton radius is already at 70.7% speed of light, most of the x137 momentum increase [p= Mv = mv/sqrt{1-(v/c)^2}] results in an increase in (relativistic) mass. (Velocity will increase about 1.41 to extremely close to c, so that means mass increases x137/1.41 = 97.) The total energy at the classical radius can then be figured as 0.511 Mev (rest mass energy) x 97 (mass increase) = 50 Mev.
In other words the total energy at the tiny classical electron radius (2.8 x 10^-15 m), which is close to the size of a proton, is actually about one hundred times (50 Mev/0.511 Mev) the energy stored in the E field!
Compton radius
Notice in the table above the Compton radius has the simplest formula,
and interestingly it contains only Planck's constant, speed of light, and
the electron mass. The electron charge (e) is missing. The other two radii
(Bohr and classical) can be considered as the Compton radius just scaled
up and down by the dimensionless alpha (fine structure constant). Alpha
(e^2/(4 pi e0 hbar c) introduces the electron charge (squared) into both
these radii, in the numerator of the classical radius and the denominator
of Bohr's radius. It also causes Planck's constant (hbar) to disappear
from the classical radius and the speed of light (c) to disappear from
Bohr's radius.
Check
Compton radius = hbar/mc
= 1.055 x 10^-34/9.109 x 10^-31 x 3.00 x 10^8
= 3.86 x 10^-13 m
Units
hbar -- energy - sec
mc = (m c^2)/c -- energy/(distance/sec) = (energy - sec)/distance
hbar/mc -- distance
alpha (fine structure constant = 1/137) -- dimensionless
The formula for the Compton radius couldn't be much simpler (hbar/mc). Notice it has the form of the DeBroglie wavelength equation (divided by 2 pi)
wavelength = h/p
where p= Mv (DeBroglie
wavelength formula)
wavelength/2 pi = hbar/p
Compton radius = wavelength/2 pi = hbar/p
where
p = mc
Momentum term 'mc' (sort of) looks like the non-relativistic electron mass x speed of light, but, of course, that can't be right. What really happens to momentum as speed approaches its asymptotic limit (c) is that mass begins to increase. At 70.7% speed of light (c/sqrt{2}) mass has increased by 1.41 or (sqrt{2}) (see far below my handrawn sketch of energy vs speed), so the momentum term in the Compton formula becomes
p = Mv = m sqrt{2} x c/sqrt{2}
= mc
My handrawn sketch also shows that at v=0.707c the kinetic energy (cp = cMv) of the electron is exactly equal to the rest mass energy (mc^2). From DeBroglie's wavelength formula and Heisenberg's uncertainty (position x momentum) relationship both the momentum uncertainty (Mv) and energy (cMv) of the electron rise inversely as the specified radius of the electron. Hence the Compton radius can be interpreted as follows:
An electron localized to a region of space with the Compton radius has its kinetic energy pushed up (by the Heisenberg uncertainty principle) to equal the rest mass energy of the electron (0.511 Mev). The Compton radius is also the De Broglie wavelength (divided by 2 pi) of an electron moving (or with a velocity uncertainty?) of 0.707 the speed of light (equivalent to the non-relativistic mass of the electron moving exactly at the speed of light).At distance bigger than the Compton radius (like the Bohr radius) speeds are slower so relativistic effects are small and can be neglected. At distances shorter than the Compton radius speeds are close enough to the speed of light that relativistic effects dominate and quantum effects become really important. One ref puts it this way:
"Trying to localize an electron to within less than its Compton wavelength makes its momentum so uncertain that it can have an energy large enough to make an extra electron-positron pair!" (This is true when kinetic energy is > 2 x 0.511 Mev.)Fine structure constant
Hints --- If a formula has h in it, then quantum mechanics is involved. If a formula has c in it, then relativity is involved. If a formula has e in it, then electromagnetism is involved. Notice alpha has e, h and c.In plank units (used by Feynman in QED)
In normal units
QED electron/photon coupling (probability) amplitude = sqrt{alpha)
QED electron/photon coupling probability <==> alpha
electromagnetic force constant
coulomb force = alpha hbar c/r^2
(NIST)
As described below, the fine structure constant is associated with photon/electron coupling (probability) amplitude, a key number used in the quantum electro dynamics probability calculations, and the measured charge of the electron. Fine structure constant is one of the twenty-odd 'free' or 'external' parameters of the Standard Model of particle physics. Its value is not predicted by QED theory, its value is inserted based on experimental results.
NIST (standards site) says "We now consider alpha the coupling constant for the electromagnetic force, similar to those for other known fundamental forces like the gravitational force (G m1m2/r^2)."
Coulomb force = [1/(4 pi e0)] e^2/r^2
but
alpha = e^2/(4 pi e0 hbar c)
e^2/(4 pi e0) =alpha hbar c
so
Coulomb force = alpha hbar c/r^2
The 'fine structure constant' is nearly always given as 1/137 rather than 7.297 x 10^-3, because it's easy to remember, within 0.03% of the measured value, and historically there were quasi-theoretical speculations by Eddington it 'should be' (exactly) 1/136, whoops 1/137. A more exact value is 1/137.036. The name 'fine structure constant' is rather strange. My guess is that it's an historic name related to a splitting of spectroscopic lines. Spectroscopic lines are often described as having a 'fine structure', meaning under some conditions at high resolution they split into multiple lines.
Yup, the fine-structure constant was originally introduced into physics (by Arnold Sommerfeld in 1916). Sommerfeld changed Bohr's circular orbits to ellipses and including relativity in his analysis and with these changes he was able to calculate the fine splitting of some hydrogen spectral lines. Sommerfeld's analysis showed that the ratio of the (av) velocity of the electron in the first circular orbit (orbit 1s) to c is equal to the fine-structure constant (alpha), i.e. average electron velocity = (1/137.036) x c.
Feynman introduces the fine structure constant in QED this way:
"There is a most profound and beautiful question associated with the observed 'coupling constant', e, the (probability) amplitude for a real electron to emit or absorb a real photon. It is a simple number (e) that has been experimentally been determined to be close to 0.08542455 = 1/11.706 = 1/sqrt{137.036}. It has been a mystery ever since it was discovered more than fifty years ago, and all good theoretical physicists put this number up on their wall and worry about it." (QED p129)Planck charge is one of the base planck natural units; it is equal to e divided by sqrt{fine structure constant} coming out to be a charge about 11.7 times larger than e. Or from another point of view (e/planck charge)^2 is equal to the fine structure constant.Werner Heisenberg told his friends that the problems of quantum theory would disappear only when 137 was explained, and spent years trying to explain it (and failed). Pauli spent endless research time trying to multiply pi by other numbers to get 137.
* Elsewhere in the QED book e is identified as the observed 'charge' of the electron.
* Probability amplitudes are squared to find probability, so the fine structure constant (proportional to e^2) is associated with the probability of a quantum event occurring, the sqrt disappearing.
* In most of the book j is used for the theoretical coupling constant. Its value is given only as approx (0.1). The observed coupling constant, e (electron charge) is the theoretical j with some real world correction terms, coming out experimentally to be about 0.08542455, the sqrt{fine structure constant}.
Here's another take on the fine structure constant 'mystery'. For me this nicely explains what bothers physicists and clarifies Feynman's somewhat cryptic comments. I found it on something called the Feynman webring.
"Alpha [(e^2/hbar c) in cgs] is nothing more, nothing less than the square of the charge of the electron divided by the speed of light times Planck’s constant. Thus this one little number contains in itself the guts of electromagnetism (the electron charge), relativity (the speed of light), and quantum mechanics (Planck’s constant). All in one number! ... Physicists would like to believe that these phenomena fit together tidily in accordance with one big plan. They would like the ratio of electromagnetism, relativity, and quantum mechanics to be a number like one, or maybe two times pi. They do not like its being 137 — a prime number, for heaven's sake (well, not really)!" (Feynman webring)Fine structure equationWhat happened to e0? It disappears in the cgs system, where 1/(4 pi e0)=1. Wikipedia commenting on this says: "In SI units with alpha =(e^2/4 pi e0 hbar c) it may be clearer that it is in fact the complicated quantum structure of the vacuum that gives rise to a non-trivial vacuum permittivity."
"Sommerfeld's Fine-Structure Constant may be viewed as the only free parameter in QED (what about mass?), the relativistic quantum theory of the interactions between electrons and photons (for which Feynman, Schwinger and Tomonaga shared the 1965 Nobel Prize). In QED, the coupling constant's effective limit is simply the square root of alpha, and Feynman was understandably annoyed that QED was thus based on an unexplained numerical value. He expressed the wish that a deeper understanding of Nature would eventually explain that value and/or allow it to be expressed in terms of known constants, like p or e." (Feynman webring)
Fine structure constant is usually designated by 'alpha'.
alpha = e^2/(2 e0 hc) (fine structure constant)
but c = 1/sqrt{e0u0} = e0^-1/2 x u0 ^-1/2
alpha = e^2/(2h sqrt{e0/u0})
= e^2 sqrt{u0/e0}/2h
but sqrt{u0/e0} = Z the characteristic impedance
of free space = 377 ohms (376.730313461)
alpha = e^2 Z/2h
= (1.6 x 10^-19)^2 x 377/(2 x 6.63 x 10^-34)
= 1/137 (approx)
units check -- Z is in ohms = volt/amp = volt/(coulomb/sec)
= volt sec/coulomb
e is in coulombs
h can be put in units ev-sec = e volt sec
so
alpha = e^2 Z/2h = (e volt sec)/(e volt sec) (dimensionless)
This is an interesting and unusal way of writing the alpha. I virtually never see it written this way, but this way makes clear its intimate relation to the von Klitzing constant (see below). Z (along with e0, u0 and c) are defined constants in the SI system of units. It highlights that alpha is the electron charge squared divided by planck's constant scaled by an exact constant that is a function of the properties of the vacuum.
Another derivationWhat I learned in school as the rationalized MKS system of units (meter, kilogram, second, ampere) is now called the 'modern metric system' or SI (International System of Units) with NIST (National Institute of Technology and Standards) as the standard reference. The link below is a NIST search window to get the latest value and uncertainty of any constant.
Here's another, cleaner, way to see that alpha is dimensionless. Obviously the numerator of alpha (e^2/2e0 hc) has units of charge squared, so we need to show the denominator of alpha also has units of charge squared We use two equations (below) relating properties of the vacuum (e0 and u0) and speed of light (c) and the simple electrical relationships (V/Z = i), which is basically ohms law, and the definition of current (i = charge/sec):c = sqrt{1/e0 u0} speed of light
Z = sqrt{u0/e0} impedance of free spacealpha (donominator units) = e0 hc
= h x sqrt{e0/u0}
= joule-sec x 1/Z
= V charge-sec x 1/Z
= (V/Z) charge sec
= (i) charge sec
= (charge/sec) charge sec
= charge ^2 QED
http://physics.nist.gov/cuu/Constants/index.html
check
e^2 Z = e x e 377 volt/amp
= 377 ev x e/amp
= 377 ev x e/(1 coulomb/sec)
= 377 ev 1.602 x 10^-19 coulomb/(1 coulomb/sec)
= 6.04 x 10^-17 ev sec
alpha = e^2 Z/2h
= 6.04 x 10^-17 ev sec/2 x 4.14 x 10^-15 ev sec
= 0.729 x 10^-2
= 1/137.09
Related constants
There are
a couple of other constants that are similar to alpha in the sense they
are a mixture of e and h..
alpha e^2/(4 pi e0 hbar c) 1/137.036 dimensionless
von Klitzing
constant
h/e^2
25,813
ohms
hall effect
conductance quantum e^2/h
(inverse of von Klitzing constant)
inverse of
conductance quantum h/2e^2
12,906
ohms
(1/2 von Klitzing constant)
Josephson constant
2e/h
4.83 x 10^14 hz/v
magnetic flux
quantum
h/2e
2.07 x 10^-15 weber
(inverse of Josephson constant)
The von Klitzing constant is just the inverse of the fine structure constant scaled by a vacuum constant (2 e0 c) that is exact. Hence measuring the von Kitzing constant, which has been done to great accuracy, directly yields a value for alpha.
The von Klitzing constant arises from steps seen in the hall voltage. hall voltage is a 'cross' voltage seen in semiconductors that is proportional to an external magnetic field. (A common way of measuring currents in wires in power electrons is to use a 'hall sensor' to measure the magnetic field around the wire because it is proportional to the current in the wire.) The voltage steps seen in the hall voltage are found to be interger related to the fabulous accuracy of a part in a billion. Since the hall voltage is proportional to the bias current in the semiconductor, the units of the hall voltage step quantization come out to volt/amp, which is ohms.
The Josephson constant (basically the magnetic flux quantum) is the inverse of the von Klitzing constant divided by half the electron charge.
Interpretation
One refererence
puts the modern interpretation of the fine structure constant this way:
Since alpha is proportional to e2, it is viewed as the square of an effective charge "screened by vacuum polarization and seen from an infinite distance."Variation with energy
At shorter distances corresponding to higher energy probes (large momentum transfers), the screen is partially penetrated and the strength of the electromagnetic interaction increases since the effective charge increases. Thus depends upon the energy at which it is measured, increasing with increasing energy. At an energy corresponding to the mass of the W boson (approximately 81 GeV, equivalent to a distance of approximately 2 x 10^-18 m), alpha is approximately 1/128 compared with its zero-energy value of approximately 1/137. Thus the famous number 1/137 is not unique or especially fundamental.
Uncertainity
Interestingly
the attached link shows Z (impedance of free space) is exact, a
consequence of definitions. (c, u0, e0 are all exact too)
http://physics.nist.gov/cgi-bin/cuu/Value?z0
Wow, that makes alpha = (e^2 Z/2h) experimentally dependent only on measured electron charge e and planck's constant h (or vice versa). A fact that nowhere to date have I seen pointed out!
NIST reference site says this about relative uncertainties
alpha
6.8 x 10^-10 (also the uncertainty of Bohr radius, why?)
h
5 x 10^-8 (also uncertainty of electron mass, why?)
e
2.5 x 10^-8
I think these numbers imply that the value of e is (most accurately) known from measurments of alpha and h . (Or maybe h is known from measurements of alpha and e?) Alpha is the most accurately known, so half the uncertainty of h (due to e^2) becomes the dominant uncertainty in e (or vice versa). (e/h is shown as 2.5 x 10^-8)
Why is fine structure constant related to radius?
"According to the theory of renormalization group, the strength of the electromagnetic interaction (value of the fine-structure constant) depends on the energy scale. In fact, it grows logarithmically as the energy is increased. The measured value of the fine structure constant is associated with the energy scale of the electron mass (0.511 Mev). There is no energy scale below the electron (and positron), because they are the lightest charged objects, so we can say that 1/137.036 is the value of the fine-structure constant at zero energy." (Wikipedia, edited)While the Wikipedia description above is not very clear, here is my attempt to combine it with QED into simple terms:
Measurable "e" (charge of electron) is the ideal coupling constant "j" in QED that is modified by correction terms one of which includes "n" related to the mass of the electron. That makes the value of "e" dependent to some extent on the mass of the electron, lowest mass particle. And via relativity (E=m c^2) that makes the value of "e" dependent to some extent the energy of the electron (0.511 Mev).
Measuring fine structure constant
The fine structure
constant is a measured parameter. In fact is one of the twenty or fundamental
'free components' of QED, a cornerstone of fundamental physics.
Question --- Is the value of "e" actually derived from measurement of the fine structure constant? It could be, because the fine structure constant is known to many, many digits. From its equation the fine structure constant is the electron charge combined with the properties of free space (u0, e0) and planck's constant.
The fine structure constant is measured in two ways:
electron magnetic moment anomaly -- most accurate
quantum hall effect --- coorborates magnetic moment value
others
muonium hyperfine
splitting
fine structure
splitting
gyromagnetic
ratio of protons
h/mx ??
Derivation of the classical electron radius
In simple
terms, the classical electron radius is roughly the size the electron would
need to have for its mass to be completely due to its electrostatic potential
energy - 'not taking quantum mechanics into account'. It is a radius of
spherical shell of total charge whose electrostatic energy equals the rest
mass energy of the electron (0.511 Mev). Or put another way -- The total
energy stored in the electrical field outside the spherical shell of charge,
e, from radius r0 to infinity, is set equal to the electron rest mass energy
(m x c^2).
'Not taking quantum mechanics into account' is quite an understatement
To get the energy stored in the E field up to 511,000 ev (electron rest mass energy) the electron radius has to be reduced about four orders of magnitude from its size in the hydrogen atom (5 x 10^-11 m) down to (3 x 10^-15 m) in the range of a proton. When Heisenberg's uncertainty principle is applied to this tiny size, the momentum is so high that the speed is virtually at c (0.9999 c), which increases the relativistic mass about two orders of magnitude.
This means quantum effects cause the total electron energy at this tiny size (about 70 Mev) to be about two orders of magnitude higher than the electron rest mass energy. Hence the classical electron radius doesn't have any real meaning, at least in any sense that I can see, except perhaps as a pre-quantum mechanics relic.The formula for the classical radius is found by equating the potential energy (ke^2/radius) to the rest mass energy (mc^2) and solving for the radius.
classical radius = k e^2/mc^2
where
k = (1/4 pi e0)
A simple way to calculate the classical radius of an electron is find the energy required to move in a (point) 'test' charge from infinity to distance r0 from a (point) electron. The test charge is also a (point) electron, so it feels a repulsive (outward) Coulomb force that increases inversely as the square of the distance between the electrons, so we have to do work on the test charge to bring it in. (I read that this calculated work energy is the same value as the energy stored in the E field, but I have not verified this.) The classical electron radius (r0) is defined as the distance of the (point) test charge from the (point) electron where the total energy required to bring in the test charge (from infinity) becomes equal to the Einsteinian rest mass energy of the electron (0.511 Mev = E = m x c^2).
check
E = m x c^2
= 9.109 x 10^-31 kg x (3 x 10^8 m/sec)^2
= 8.20 x 10^-14 joule
= 8.20 x 10^-14 joule x (Mev/1.602 x 10^-13 joule)
= 0.512 Mev
Energy is (force x distance), and since the force varies with distance (radius), we integrate {force x dr} from infinity to r0 to find the energy.
Coulomb's repulsion force F = k x q^2/r^2 and E = F/q = k x q/r^2
where
F in newtons, q in coulombs
k = 1/(4 pi e0) = 8.99 x 10^9
e0 = 8.85 x 10^-12 (permittivity of free space)
Energy = integral {F dr}
= integral {k e^2 r^-2 dr}
= -k e^2 (- r^-1) from r0 to infinity
= k e^2/r0
= (1/4 pi e0)e^2/r0
= 8.99 x 10^9 (1.60 x 10^-19)^2/r0
Energy stored in E field
If an electron is
modeled as a thin spherical shell of charge (radius r0), then the energy
stored in the electron's E field can be easily calculated (and it comes
out finite!). I know from college physics that there is no gravity inside
a thin shell of mass, and since the equations of electrical coulomb force
are almost the same as gravity (both k/r^2), then there is probably no
force on a test charge inside the shell, and hence no E field since (Force
= q E). The energy stored in the E field outside is found by integrating
(summing) the energy stored in thin spherical shells from r0 to infinity.
Electrical energy density = (1/2) e0 E^2, and the volume of a thin sphrerical
shell is 4 pi r^2 dr, so
Energy = integral {(1/2 e0 E^2) x (4 pi r^2 dr)}
= integral {2 pi e0 E^2 r^2 dr}
= integral {2 pi e0 [1/(4 pi e0)^2 x e^2/r^4] r^2 dr}
= integral {(1/2) 4 pi e0 [1/(4 pi e0)^2 x e^2/r^2] dr}
= integral {(1/2) [1/(4 pi e0) x e^2/r^2] dr}
= integral {(1/8 pi e0) x e^2/r^2 dr}
= (1/8 pi e0) e^2 (-r^-1) from r0 to infinity
= (1/8 pi e0) e^2/r0
For some reason the field energy calculated by integrating the energy density (above) comes out to be half the value previously calulated by doing work on moving a test charge from infinity. Don't know where the error is. But the test charge method is probably right, since when the energy is equated with 0.511 Mev (electron rest mass) it gives the correct value (Wikipedia value) for the classical electron radius. (Generally 1/2 comes from integration, so it seems like using the 1/2 in the energy density formula is like a double integration??)
How does the energy in the E field energy vary?
So how is
the field energy distributed? The equation says the total E field
energy goes up inversely with the radius of the charge shell. Make the
shell radius x10 smaller, and there is x10 total energy stored in the E
field. (The energy stored in the electron E field goes toward infinity
as the radius of the electron goes toward zero, which, of course, is the
classic problem that for years drove QED researchers nuts.)
What's really going on, of course, is as you get closer to the 'point' electron field volume is going down as r^3, but energy density (1/2 e0 E^2), since (E =ke^2/r^2), is going up as 1/r^4, resulting in total energy in the field going up as 1/r.
Finding the classical electron radius
We set the
energy equal to the rest mass energy of the electron (0.511 Mev = 8.20
x 10^-14 joule), and solve for r0. Below uses the equation from moving
the test charge.
r0 = 8.99 x 10^9 (1.60 x 10^-19)^2/8.20 x 10^-14 joule
= 2.81 x 10^-15 meter
Wikipedia gives the classical electron radius = 2.82 x 10^-15 meter (checks)
In effect the classical electron radius is the size of a 'charge ball, such that energy in the electric field (outside the ball) represents all the energy of the electron. Wikipedia puts it this way:
"In simple terms, the classical electron radius is roughly the size the electron would need to have for its mass to be completely due to its electrostatic potential energy - not taking quantum mechanics into account. We now know that quantum mechanics, indeed quantum field theory, is needed to understand the behavior of electrons at such short distance scales, thus the classical electron radius is no longer regarded as the actual size of an electron." (title -- Classical electron radius)Another reference puts it this way:
"Renormalization is an aspect of field theory which deals with such issues as the fact that the electromagnetic field produced by an electron has energy and thus should be counted as part of the mass of the electron! The length scale at which these effects become really important is called the classical electron radius."Physical interpretations of the fine structure constant
alpha = e^2/(4 pi e0 hbar c) = 1/137
(fine structure constant)
= e^2/(2 e0 hc) = 1/137
= {e^2/(4 pi e0 r0)} x {(2 pi r0)/hc}
= {e^2/(4 pi e0 r0)} / {hc/(2 pi r0)}
(ratio of electron energy/photon energy)
The top alpha term {e^2/(4 pi e0 r0)} was derived in the section above. It is the energy required to bring in a test charge from infinity to r0 distance from an electron. And when r0= classical radius of this electron, this energy equals the rest mass energy of the electron (0.511 Mev).
For photons
c/wavelength = frequency
(2 pi r0) can be interpreted as a wavelength
energy of a photon = frequency x h
The bottom
alpha term is
hc/(2 pi r0) = h frequency
= energy of photon associated with radiation
whose wavelength = (2 pi r0)
Hence the fine structure constant can be interpreted as the ratio of the electron rest mass energy to the energy of a photon whose wavelength = (2 pi classical electron radius). As shown below, the energy of this photon is 137 times larger than the rest mass energy of the electron.
1
electron rest mass energy (0.511 Mev)
alpha = ----- = ---------------------------------------------------------------------------
137 energy of photon with
wavelength = (2 pi classical electron radius)
The classical electron radius (2.82 x 10^-15 m) is the smallest of the electron radii. There is another radius for the electron that is 137 times larger than the classical radius, so a photon associated with this radius (Compton radius) will have energy 137 times lower.
Hence a photon with a wavelength = (2 pi x Compton radius) = Compton wavelength, should have energy equal to the rest mass energy of the electron. Let's put in some numbers and check.
h x frequency = h x c/(2 pi Compton radius) = ? = 0.511 Mev
(6.626 x 10^-34
x 3 x 10^8)/(2 pi x 3.86 x 10^-13) = ? = 5.11 x 10^5 x 1.60 x 10^-19
8.20 x 10^-14 joule = 8.18 x 10^-14 joule
checks
Spectroscopic data
In 1885, long
before Rutherford and Bohr, Balmer found a simple formula that accurately
fit the visible spectroscopic lines of hydrogen. Using the formula he predicted
a hydrogen line should exist at a higher frequency, and it was found exactly
where Balmer predicted. There was no physics behind the formula, for unknown
reasons it just worked. Here is Balmer's formula (in modern form)
1/ wavelength = Rh (1/2^2 - 1/n^2)
where
n = 3,4,5 etc
Rh = 10,967,758 m^-1 (Rydberg constant)
Wavelength of first two Balmer lines:
(n=3)
1/ wavelength = 10,967,758 m^-1 x (1/4 - 1/9)
= 1.0968 x 10^7 x 0.139
= 0.1523 x 10^7 m^-1
wavelength = 6.565 x 10^-7 m
(or 656.5 nm)
(n=4)
1/ wavelength = 10,967,758 m^-1 x (1/4 - 1/16)
= 1.0968 x 10^7 x 0.1875
= 0.20565 x 10^7 m^-1
wavelength = 4.863 x 10^-7 m
(or 486.3 nm)

When looked at with high resolution spectrographs some hydrogen lines were found to be doublets. When hydrogen was placed in magnetic fields and electric fields, some of the lines moved or split. More line were found in the ultraviolet. All these line and their shifts and splits provided a wealth of information about how the one electron of hydrogen could move, if only it could be figured out. The emission spectrum of hydrogen was the key data used in early atomic models to quantify the possible orbits of the one electron about the hydrogen nucleus.
It was later found that Balmer's formula was a special case of the Rydberg formula, and that the constant in the Rydberg formula was a mixture of fundamental units.
Bohr's 1913 atomic model
Bohr's first
(1913) atomic model of hydrogen was simple with seemly arbitrary quantum
restrictions on electron orbits. It's real strength was that it (quantitatively)
explained the Balmer formula, and by providing a way to picture of how
electrons moved in orbits within atoms it (qualitatively) explained some
properties of the photoelectric effect too. It also was consistent with
(& predicted) some very high energy lines of hydrogen that can only
be observed astronomically where collisions of hydrogen atoms in the high
vacuum of space are rare.
The calculated energy difference in electron orbits turned out be proportional to the inverse of Balmer's wavelength formula with the constant of proportionality being the constant Planck had proposed a decade earlier to explain blackbody radiation. The picture provided by the model was that hydrogen emission lines were caused by electrons jumping between orbits with the frequency of the line corresponding to the energy difference between the orbits.
Soon after the Bohr model was introduced, however, it became clear it was not complete. Higher resolution spectral data on hydrogen showed some of the supposed single Bohr/Balmer lines were in fact two (or more) lines very close together. The Bohr model with its simple circular orbits had no explanation for this 'splitting' of lines.
Sommerfeld extended Bohr's model by assuming more complex electron orbits, like ellipses rather than circular, and he adding spins. Thus increasing the number of (so called) quantum numbers needed to describe the electron from 1 (Bohr) up to 4. While this elaborated model explained more lines of hydrogen, it was never successful with any element other than hydrogen.
Three key equations of quantum physics
About a 1/4
century of work (1900 to 1926) was needed to understand the frequency/wavelength
characteristics of quantum particles, and to understand how they are connected
to classical mechanical properties of particles like energy, velocity,
momentum and position. Three simple relationships were found that universally
applied, and amazingly all the relationships had only a single scaling
factor (Planck's constant) designated by the symbol, h. Planck's constant
has the units of energy x time (or momentum x distance) and in the MKS
system the value h = 6.63 x 10^-34 joule-sec or 4.14 x 10^-15 ev-sec.
Planck's constant can be found by measuring the energy in photons of different color light. Photons of high enough energy impinging on electrons in a metal (in a vacuum tube) will knock some electrons free (photoelectric effect). What happens is an electron 'recoils', acquires kinetic energy, as it absorbs a photon and its energy. The energy of the freed electrons in the vacuum of a vacuum tube is easily measured by varying the voltage on the electrodes and monitoring the current flow. A plot of energy of the freed electrons (which obtained their escape energy from incoming photons) vs frequency gives a straight line (see Essay 'Speed of Light'), thus confirming the linear relationship of energy to frequency. The slope of the line is planck's constant. Planck in his 1901 paper, using measured blackbody data, derived a value for h of 6.55 x 10^-34 J-sec, within 1% of the modern value. (hbar is h/2 pi = 1.04 x 10^-34 j-sec)
* Energy
is proportional to frequency
* Wavelength
is inversely proportional to momentum
where momentum is relativistic mass x velocity
(Note, this means momemtum continues to rise as kinetic energy rises,
since when speed tops out near c, mass starts to rise)
Wavelength is smaller for high momentum particles, which means fast moving
particles
and high mass particles. Also wavelength of a particles approaches infinity
as the
velocity of the particle approaches zero. (Not sure what this really means).
* Energy
uncertainty cannot be less than inverse of (measurement) time
or position uncertainty cannot be less than the inverse of momentum
uncertainty
where 'uncertainty' means standard deviation
Planck relationship E = h x freq
de Broglie wavelength
wavelength = h/p
where p= mv/sqrt{1-(v/c)^2}
Heisenberg uncertainty
delta p x delta pos > hbar/2 or delta pos > (hbar/2)/delta
p
delta E x delta t > hbar/2 or
delta E > (hbar/2)/delta t
where
delta means uncertainty (standard deviation)
Comparing Heisenberg's uncertainty and DeBroglie wavelength
Notice the interesting similarity between Heisenberg's position uncertainty
and DeBroglie's wavelength:
delta position > (hbar/2)/delta momentum
(Heisenberg's uncertainty)
wavelength = h/p
(DeBroglie formula )
Say the momentum is known to (about) 8%, in other words an 8% uncertainty, then delta p = 0.08 p
delta position > (hbar/2)/delta momentum
(Heisenberg's uncertainty)
> (hbar/2)/0.08 p
> (hbar/2)/ 0.08 (h/wavelength)
> (1/4 pi) x 12.5 x wavelength
> wavelength
Wow! We find that Heisenberg tells us that the position uncertainty of a particle (or its spread into space) at a given speed (momentum) is the just the wavelength of the particle when the speed (really momentum) uncertainty is about 8% [(1/ 4pi) = 0.0796]. So for a speed or momentum uncertainty of 0.8% the spread is 10 wavelengths, and for speed or momentum uncertainty of 80% its 0.1 wavelength.
Pulling together Heisenberg uncertainty, Bohr radius
and fine structure constant
Let's find
the velocity uncertainty for an electron if we take its position uncertainty
to be half the Bohr radius, i.e. half the radius of the inner electron
orbital of hydrogen. (Note slightly rigged --- I am using half Bohr
radius as the position uncertainty because the resulting vel uncertainty
comes out very interesting.)
delta p x delta pos > hbar/2
(Heisenberg's uncertainty)
delta p > (hbar/2)/delta pos
delta v > (hbar/2)/delta pos x m
(non-relativistic mass since v<<c)
> (hbar/2)/[(1/2) x Bohr radius] x m
> (hbar)/Bohr radius x m
> (1.0546 x 10^-34)/(5.2918 x 10^-11 m x 9.109 x 10^-31 kg)
> 0.02188 x 10^8 m/sec (or 0.74% c)
0.02188 x 10^8 m/sec <=?=> alpha x c = 1/137.036
x 2.9979 x 10^8 m/sec
= 0.02188 x 10^8 m/sec
Yes indeed, we find that if we try to locate an electron to within half of the Bohr radius the uncertainty principle tells us the electron velocity uncertainty comes out be exactly equal to (fine structure constant x speed of light). The Bohr radius is the peak of the probability distribution for the inner electron orbital of hydrogen (see orbital figure below).
In other words the uncertainty principle tells us the (standard deviation of) speed of electrons must equal (or exceed) (alpha x c) if we locate the electrons radially at the Bohr radius +/- (1/2 Bohr radius). Sommerfeld came up with the same value for the speed of the electrons in hydrogen in 1913, prior to Heisenberg formulating the uncertainty principal.
De Broglie wavelength for an electron in an atom
wavelength = h/p
where
p = momentum = m x v
(classical v<<c)
p = (m/sqrt{1-(v/c)^2}) x v (all energies)
check units --- plank's constant has
units of energy x time
h = m x (dist/time) x (dist/time) x time
= m x vel x dist
= p x wavelength OK
For an electron
v = 2.188 x 10^6 m/sec (typical velocity of an electron in a hydrogen
atom in the ground state, a little below 1% c)
This electron
speed can be derived by locating the electron with an uncertainty of +/-half
the Bohr radius and applying the Heisenberg uncertainty
(see above). It comes out to be the
speed of light scaled by the fine structure constant
(1/137.036).
Check: 3 x 10^8 m/sec/137.036 = 2.18817 x 10^6 m/sec
m = 9.11 x 10^-31 kg
h = 6.63 x 10^-34 joule-sec
So De Broglie
wavelength for an electron in lowest orbit of hydrogen atom is
wavelength = h/p = h/(m x v)
= 6.63 x 10^-34/(9.11 x 10^-31 x 2.188 x 10^6)
= 3.30 x 10^-10 m (0.33 nm)
3.30 x 10^-10 m <=?=> 2 pi x Bohr radius
= 6.28 x 5.29 x 10^-11 m
= 3.32 x 10^-10 m
Another interesting result --- We find the electron De Broglie wavelength for this electron speed (alpha x c) comes out to be equal to a circumference whose radius is the Bohr radius. In other words with the electron speed set at the minimum allowed by Heisenberg uncertainty one electron wavelength just fits in the inner hydrogen orbit!
Note also this distance is several thousand times shorter than the wavelength of hydrogen emission lines (400 to 700 nm is wavelength of visible light).
Bohr radius and hydrogen
The hydrogen
atom is the smallest atom about 5.3 x 10^-11 m (0.053 nm). This the ground
state radius. The hydrogen atom can be considered to 'swell up' as its
electron gets more energy and moves to higher orbits (there are six). 0.053
nm is the Bohr radius. A probability analysis using the Schroedinger equation
shows the probability of finding the electron (in ground state) is highest
at the Bohr radius. Multiplying the Bohr radius by 2 pi to get a circumference
we get 6.28 x 0.053 nm = 0.33 nm, which is the de Broglie wavelength (surprise).
Hence when de Broglie's proposed that particles had associated with them a wavelength that depended on plank's constant and momentum, a picture emerged that helped explain (sort of) why the allowable orbits in Bohr's atom had the values they did. One wavelength (of an electron in its lowest energy state) just fits in the smallest, lowest energy orbit. Higher orbits contain 2,3,4, etc wavelengths. Simple.
Re: question above --- It's all consistent (see table far below). Each quantum number n orbit has a radius n^2 larger than orbit with inner orbit (n=1). So for example for n=2 orbit the reason two wavelengths fit into an orbit with a circumference four times longer is that the electrons in the n=2 orbit move at half the speed of the inner electrons and have 1/4 the kinetic energy. Third orbit (N=3) electrons move at 1/3 speed and have 1/9 energy, etc. This explains nicely the Balmer/Rydberg formula for hydrogen lines, where photon energy represents jumps between orbits with energy proportional to {1/(n1)^2- 1/(n2)^2}. For emission n2 is the orbit jumped from and n1 the orbit jumped to, of course it's the reverse for absorption.The figure (below) is a 'visualization' of electron de Broglie waves in hydrogen. It shows that one cycle just fits into the lowest electron orbit, two cycles in the next orbit (four times the radius) with the electron moving at half speed, three cycles in the next orbit (nine times the radius) with the electron moving at one third speed, etc.
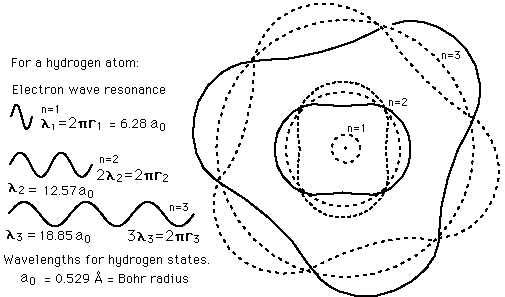
r1= a0, r2= 4a0 (from hyperphysics web
site)
Electron avoidance
QED (Quantum
Electro Dynamics) allows the calculation that two electrons (with the same
spin) will be at the same point in space and time, and as shown in Feynman's
book QED, the probability of this is exactly zero. Since electrons
are smeared out occupying an area in space of roughly their wavelength,
this sets the minimum space between them. Before QED was formulated in
the 1940's Pauli had discovered this property of electrons (in the same
state) to avoid each other and used it as a core building block of his
atomic/electron theory. Hence it's known as the Pauli Exclusion Principle.
Pauli Exclusion Principle ---- is a property not just of electrons, but all fermions. Protons and neutrons are fermions too, as opposed to bosons. Bosons 'enjoy' being in the same state and position and are particles like photons, think laser light.You could say electron avoidance is kind of important. It determines how electrons arrange themselves around the nucleus of atoms and molecules, so it determines all of chemistry and the property of molecules! Suskind in his book explains that Dirc guessed this feature of electrons from the way that atoms are arranged in the periodic table.
It also determines the character of white dwarf stars, which our sun will one day become. When stars of moderate mass run out of fuel and begin to cool down, their collapse is halted by the pressure arising from electron degeneracy, which is nothing more than the stellar application of the Pauli Exclusion Principle. Electron pressure is not temperature sensitive so even a very cold white dwarf star will not shrink much smaller than the size of the earth.
Electron orbitals -- basics
While
two electrons with the same spin can't be in the same orbit (same smeared
out point in space and time), two electrons with different spins can be.
The two electrons of a helium atom have different spins (one up, one down),
so they effectively occupy the same smeared out region of space
around the nucleus.
Chemists say helium's two electrons occupy the same orbit (more correctly called orbitals), which for historical/spectroscopic reasons is called the '1s' orbit. Spectroscopists letters for orbital states (s,p,d,f), which are roughly the shape of orbits (how many lobes), mean the following:
s sharp
s orbits are spherical
p principal
p orbits have two lobes
d diffuse
f fundamental
Here is a graphic showing sizes and shapes of some simple orbits. Notice 1s, 2s orbits are spherical with the 2s orbit x4 dia of the 1s orbit. The 2p orbits are in the same radial space as the 2s orbit, but have two lobes in three directions of space. Note if each 2 orbitals shown each holds two electrons (up & down spin), then all eight electrons in the 2 orbit are accounted for. Wikipedia lists the Electron Configuration of element #10 (neon) as 1s^2 2s^2 2p^6 (where ^ in this case indicates superscript), corresponding to the five pairs of orbits below.
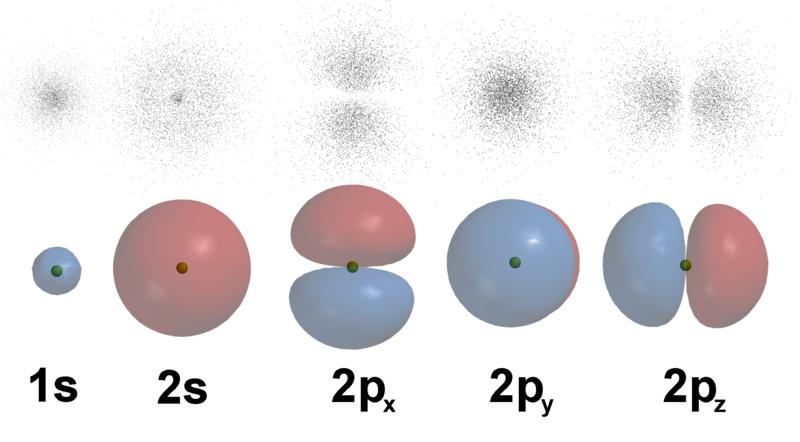
Orbitals of inner 10 electrons ('shell' of two and
eight)
Each orbital in figure holds 'two' electrons (with
opposite spins)
's' orbitals are spherical
'p' orbitals are double lobes in x, y, z
(from CreationWiki -- online encyopedia of Creation
Science (Yikes!), but it looks right)
The quantum numbers that make up electon orbit 'State' are given in the table below. The first designator is just the principal quantum n. This characterizes the orbital radius (up as n^2) and main energy level of the electron (down as 1/n^2) (explained in my table in the section below). The orbit designator after n, which are (s,p,d,f,g), characterizes the shape of the region in space (how many lobes) that the electron occupies. The three 2p orbit shapes in the figure above are due to the magnetic quantum number m (-1,0,+1), which indicates (sort of) the spacial orientation of the electron's angular momentum.
-- Sommerfeld introduced into Bohr's analysis the azimuthal quantum number (l), which relates the major and minor axes of the elliptical orbit to each other. He also found that a third quantum number (m) was needed to explain the splitting of spectral lines in the presence of an external magnetic field.
--The magnetic quantum number (m) determines the energy shift of an atomic orbital due to an external magnetic field, hence the name magnetic quantum number (Zeeman effect). (The analogous effect for splitting/shifting of lines due to an applied electric field is the Stark effect.) However, the actual magnetic dipole moment of an electron in an atomic orbital arrives not only from the electron angular momentum, but also from the electron spin, expressed in the spin quantum number. (Wikipedia)
-- The wavefunction of the Schrödinger wave equation reduces to the three equations that when solved lead to the first three quantum numbers. (Wikipedia)
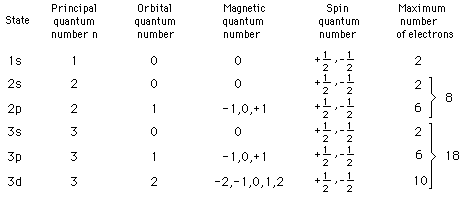
(from Hyperphysics site http://hyperphysics.phy-astr.gsu.edu/hbase/atpro.html#c2)
Electron orbitals -- helium & lithium
Atomic element
#3 lithium has three electrons. Since there are only two spin states (or
directions usually called up and down), all three electrons cannot occupy
the same orbit (1s orbits). One of the electrons is forced to occupy a
region of space further away from the nucleus (2s orbit). My first
guess was that the 3rd electron lives in a region of space that is twice
as far from the nucleus as the two inner electrons, but this is not right.
The (lithium) figure below from Hyperphysics shows that it lives
four
times further out, equivalent to the hydrogen n=2 orbit. If the outer electron
goes 1/2 the speed of the inner one, then it would have a wavelength twice
as long (since wavelength = h/p) as the inner electrons, and being four
times further out two of these wavelengths (n=2 orbit) would fit in the
circumference. Going at 1/2 speed it would have 1/4 the kinetic energy
of the inner electrons.
(n=2 orbits are x4 times radius of n=1 orbits. a0
is the Bohr radius. Fig from hyperphysics site)
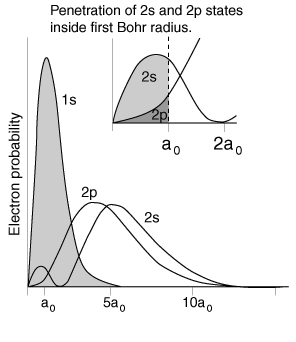
Why does the 2s (spherical) orbit have a little bump
near a0 (with a null at 2a0)?
An argument can be made that the outer lithium electron 'looking in' sees one 'net' positive charge, so it should have energy in the same ballpark as the single electron in hydrogen in n=2 orbit (why?). This turns out to true; lithium's outer electron is -5.1 ev vs -3.4 ev = (-13.6 ev/2^2) for hydrogen. The higher energy of lithium ascribed to the fact that its outer electron space somewhat overlaps the inner electrons space, so the positive charge shielding of the inner electrons is imperfect.
Lithium is a metal and like most metals it conducts electricity well, so that means that it takes very little energy to free its outer electron from the nucleus. In metals thermal energy is enough to pop a lot of electrons out of orbit so that the form a 'sea' of electrons moving between atoms. It then takes only a small externally applied voltage to get those electrons (on average) to move forming a current.
General rules of electron orbits
I think the
energy quoted for electrons in orbit is all kinetic energy, so outside
orbits with lower energy mean the electrons are traveling slower.
(update) "is all kinetic energy" --- Not really. It's correct that the speed of electrons, hence kinetic energy and momentum, is lower in outer orbits, but the potential energy is higher. When electrons absorb photons, they gain energy and jump to higher orbits, so electrons in higher orbits must have higher energy. What happens (see section below) is that potential energy goes up at twice the rate kinetic energy goes down. This explains why calculating with kinetic energy only gives the right answer numerically, but the wrong sign.If lithium's outer electron is going at half speed, then it would have 1/4 the kinetic energy of the inner electrons. This is certainly the case for the possible orbits of hydrogen for its single electron. When the x2 increase in potential energy is figured in, the total energy of orbits becomes less negative (i.e. energy increases) as orbits increase. Here are the (nominal) orbital energy values for hydrogen:
-13.6 ev/n^2 = -13.6 ev
n=1
-13.6/4 = -3.4 ev
n=2
-13.6/9 = -1.5 ev
n=3
check (n=1)
Speed of the hydrogen electron (lowest energy orbit) is a little less than
1% speed of light = (c x alpha), where alpha = 1/137.036, fine
structure constant.
1/2 m x v^2 = ? = 13.6 ev
1/2 m x {c^2 x alpha^2} = 1/2 alpha^2 x (mc^2)
where
mc^2 is electron rest mass energy (0.511 Mev)
= 1/2 alpha^2 x 0.511 ev
= 510,000 ev/(2 x (137.036)^2)
= 13.606 ev checks
Ionization energy
Below is a
cool graph from Wikipedia showing the 'ionization energy', or the energy
required to pull off one outer electron from an isolated atom of any element.
Note is doesn't vary much over the whole periodic table with most elements
between 5 and 10 ev. Hydrogen is at 13.6 ev and the prominent peaks are
those of noble gases with completed electron shells.
Ionization energy of single atoms of varying Z (source
Wikipedia)
Electron being captured by proton
When a ball
on earth is dropped and falls into the earth's (gravitational) energy well,
it trades potential energy for kinetic energy (as the ball speeds up).
Note with a falling ball (ignoring air friction) all of its lost
potential energy is converted into kinetic energy.
Consider what happens when a proton and electron 'slowly' meet, and the electron is captured by the proton forming a hydrogen atom. Let's assume the electron falls into the lowest possible orbit (n = 1). The electron falling into a proton's (coulomb) energy well is like the balling falling into a gravitation well, except there's a twist. Only half of the electron's potential energy (27.2 ev relative to the proton) is converted into kinetic energy (13.6 ev), and the other half is radiated away, by the electron emitting a 13.6 ev photon.
Necessary to explain the stability of atoms!
Why the difference between the ball and the electron? I don't know the technical answer, but it probably has something to do with the 'viral' theorem (with which I am not very familiar) that ratios potential to kinetic energy. But maybe it's as simple as this:
When the ball drops, it has the same energy. It's just trading potential energy for kinetic energy. But if the atom is to be stable, the electrons in lower orbits need to have lower energy. Hence the falling electron needs to get rid of some of its energy. But it can't radiate away all the lost potential energy, because it has to convert some of it into higher kinetic energy to satisfy the Heisenberg uncertainty requirement for fitting into a smaller (known) volume. (Well, from this point a view a 50/50 split, radiating half away and converting half, is pretty simple. Case closed?)Let's check the numbers. We already know the kinetic energy of the electron in the inner orbit of hydrogen (velocity alpha x c) is 13.6 ev. The spectroscopic lines emitted from hydrogen when electrons drop from higher orbits to the inner orbit is called the Lyman series. The highest energy Lyman line, which represents a fall from outside the nucleus to the lowest (n = 1) orbit, has a wavelength of 911.3 angstroms (911.3 x 10^-10 m).
A 13.6 ev photon has a frequency:
freq = E/h
= 13.6 ev x 1.6 x 10^-19 joule/6.62 x 10^-34 joule-sec
= 3.29 x 10^15 hz
(or cyles/sec)
In general (wavelength x freq = velocity), so for a photon (velocity = c) and we have
wavelength = c/freq
= 3 x 10^8 m/sec/(3.29 x 10^15 hz)
= 9.12 x 10^-8 m
(checks)
How does momentum divide?
The momentum
of the photon is
p = E/c = freq x h/c = h/wavelength
= 6.62 x 10^-34/9.12 x 10^-8
= 7.26 x 10^-27 kg-m/sec
From above we know an electron with 13.6 ev of kinetic has velocity of c x alpha (about 1% speed of light), so the (classical) momentum of a 13.6 ev electron is
p = m x v
= m x (c x alpha)
= 9.11 x 10^-31 kg x (3 x 10^8/137.036)
= 2.0 x 10^-24 kg-m/sec (or 2,000 x 10^-27
kg-m/sec)
Interestingly even though the photon carries off half the energy of the in-falling electron, we find it has only (7.26/2,000 = 1/275) or 0.36% of the momentum of the heavy electron. (This is generally consistent with classical mechanics. Kinetic energy goes as v^2 whereas p goes as v, so a light object with the same energy as a heavy object is going to have much less momentum.) Since the general quantum formula for wavelength = h/p, this means the wavelength of the 13.6 ev electron is 275 times smaller than the wavelength of the 13.6 ev photon.
wavelength = h/p
electron wavelength = (7.26/2,000) x photon wavelength
= (1/275) x 9.12 x 10^-8 m
= 3.31 x 10^-10 m
= 2 pi x 5.27 x 10^-11 m (or 2 pi x
Bohr radius)
This looks right. We find that that one wavelength of our 13.6 ev electron just wraps around the proton with an inner orbit radius equal to the Bohr radius (5.29 x 10^-11 m).
Bottom line --- The photon carries off half the energy of the in falling electron, but being light (zero rest mass, but relativistically it has mass) momentum divides very unevenly. The heavy electron ends up with x275 more momentum than the light photon. Since wavelength is inversely proportional to momentum, the electron wavelength, which (we find) defines the size of the Bohr atom, is 275 times smaller than the large wavelength of the emitted photon.
But why 275, where does this ratio come from? Clue: notice 2/alpha = 2 x 137 = 275 (very close).
We can equate the energy of the electron and photon and solve for the momentum ratio.
E photon = E electron
= 1/2 m x v^2
= 1/2 m x (c x alpha)^2
p photon = m x c = (E photon/c^2) x c = E photon/c
finding momemtum ratio
p electron/p photon = m x (c x alpha)/(E photon/c)
= m x (c x alpha)/{1/2 m x (c x alpha)^2)/c}
= 2/alpha
= 2 x 137.036
= 274
(13.6 ev photon relativistic) mass
= E/c^2
= 13.6 ev x 1.6 x 10^-19 joule/(3 x 10^8)^2
= 2.42 x 10^-35 kg
Another way to look at where 274 comes from is that the ratio of electron mass to (relativistic mass of a 13.6 ev photon) is 9.11 x 10^-31 kg/2.42 x 10^-35 kg = 37,600. But the photon is travelling x137 faster than the electron (c/c x alpha), so when combined the momemtum ratio is 37,600/137 = 274.
'Length' of a photon
A simple argument
from relativity and Heisenberg uncertainty (see Speed of Light essay) says
the 'length' of a photon, i.e how uncertain (fuzzed) it is in the direction
of travel, is just its wavelength, which is 9.12 x 10^-8 m for our
13.6 ev photon). It's hard to come up with a classic picture of how our
small atom can emit a photon whose wavelength (& quantum uncertainty
size) is x275 (or (pi to 2 pi) x275) longer than the emitting distance.
Electron 'shake' time as hydrogen atom forms (3/2011)
The classical
picture is a shaking electron emits radiation. So what is the period of
a 13.6 ev photon and how does that compare with the (nominal) orbit time
of a 13.6 ev electron in a Bohr orbit? A back of the envelope calculation
suggested the period of the photon was twice the orbit time of the electron
and that is confirmed below.
The frequency of a 13.6 ev photon isSo the the times are close with almost exactly a factor of two separating them. This is interesting
E = h x freq
freq = E/h
= (13.6 ev x 1.6 x 10^-19 joule/electron)/6.6 x 10^-34
= 3.3 x 10^15 hzso period of 13.6 ev photon is
period = 1/3.3 x 10^15 hz
= 3.0 x 10^-16 secNominal 'orbit' time for a 13.6 ev electron in a 53 pm Bohr orbit at speed of (1/137)c is
period = 2 pi x 53 x 10^-12 meter/(1/137) x 3 x 10^8 m/sec
= 3.33 x 10^-10 meter/2.18 x 10^6 m/sec
= 1.52 x 10^- 16 sec
As an electron drops into a proton to form a hydrogen atom the time required to emit one cycle of a 13.6 ev photon (the electron 'shake' time if you will) is almost exactly twice the time it takes the electron, once it has settled into its 53 pm Bohr orbit, to make an orbit. Not sure what to make of this, but it looks very suggestive. Does the electron shake for two orbits as the hydrogen atom forms?
How to think about electron orbits
It looks like
the way to think about electron orbits is shown in the table below. Note
all the orbit parameters, including radius and energy, are mostly set by
the principle quantum number 'n', which is the first number in the orbit
name. (The scaling of the outer orbits is relative to the inner '1s' orbit.)
The two key
facts to remember:
* Orbits radius grow as the square of the orbit number ('n')
* Electron in outer orbits move slower (speed goes as 1/n)
|
|
in orbit |
|
|
(= h/p) |
energy |
energy |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
In the late 1800's it was found empirically that emission lines of hydrogen had frequencies that fit Balmer and Rydberg formulas. Rydberg formula says the frequency varies as (1/2^2 - 1/n^2), where n=3,4,5, which is (1/4 -1/9) or (1/4 - 1/16), etc. The table above shows electron energy drops as 1/n^2, dropping in sequence 1, 1/4, 1/9, 1/16, etc as orbit radius increases. It appears that the photon energy corresponds to the difference in energy between orbits, for example (1/4 -1/9) or (1/4 - 1/16). This immediately explains the hydrogen spectroscopic data. The standard interpretation is that electrons are jumping orbits with photons bringing in or carrying off the orbital energy difference.
Odd --- Why is the first term in the eqation 1/4? Aren't there lines in the hydrogen spectrum where the electron drops from outer to the inner (n=1) orbit? Is this term missing in the Balmer equation because the frequency was too high to detect in the 19th century?Looking at the table above I realized we can say still more about electron orbits. Let's characterize the width (fuzz) of each orbit as roughly +/- wavelength/2pi (wavelength equivalent to an exponential time constant). With the orbit center radius increasing as n^2 and the orbit width increasing as n, then the following neat picture emerges --- All the orbits expand just enough to fill out the radial space (see table below). A very nice picture indeed.Yes, there are (a series of) hydrogen lines for electron jumps from outer to the inner (n=1) orbit. These lines are called Lyman lines and are in the ultaviolet. Balmer lines are visible lines. The first term in the Balmer equation is (1/2^2) because the electron jumps are from outer orbits to the n=2 orbit.
|
|
|
(radius) |
(wavelength/2pi) |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Note we can now understand why the radial '1' and '2' orbits in the figure above look as they do. Consistent with the table above the '2' orbit is about twice as wide as the '1' orbit and centered x4 higher. The height of the probability (density) curve of the '2' orbit in the figure is about half the '1' orbit. This is explained by the '2' lobes being about twice the width of the '1' lobe, and the area under each lobe must be probability 1 (an electron must be somewhere in its radial zone). We can predict that '3' orbits would be (roughly) x3 width of the '1' orbit, 1/3 the height, and centered at x9 of the '1' orbit. Here's a little sketch I made:
An electron in a higher hydrogen orbit moves slower
so it smears out more (wavelength = h/mv)
Are electron orbits like (some) star orbits?
In a globular
cluster or the Milky Way's spherical halo (population I stars) all the
stars loop through the center in individual highly elliptical orbits with
the result that the combination of all of these elliptical orbits randomly
aligned produces a spherical distribution. Are electron 's' orbits in atoms
like this? (It would be interesting to find a radial astronomical
distribution to compare with the schrodinger plots.)
How 'spread out' are electrons?
An electron
'fuzzes out' into space. How much an electron (or any quantum particle)
'spreads out' depends on the Heisenberg uncertainty principle. The relevant
form of the principle is
(momentum uncertainty) x (position uncertainty) > hbar/2
where
uncertainty (technically) = standard deviation
hbar = planck's constant (h) / 2 pi
Hence how much an electron 'spreads out', its position uncertainty, depends inversely on how accurately its momentum is known, which since the electron mass is known is equivalent to how accurately its speed is known. The uncertainty equation looks a lot like the DeBroglie wavelength equation (wavelength = h/p). So at first I thought that the 'spread out' was simply (a fraction of) the DeBroglie wavelength. Unfortunately, it's not that simple.
The DeBroglie
wavelength is a function of momentum, not momentum uncertainty.
The table below shows the calculated DeBroglie wavelength and (double)
the position standard deviation for an electron that is not moving and
moving at 1% c and 10% c, all with an uncertainty in velocity of 0.1% c.
|
|
(h/p) |
2 x (hbar/2)/(momentum uncertainty) |
|
|
|
|
|
|
|
|
|
|
|
|
The values in the wavelength column, which is calculated from DeBroglie's formula, show that as a free electron speeds up, its wavelength shrinks. An electron moving at 10% c has a wavelength about 1/10th the wavelength of an electron moving at 1% c. The last column, which is calculated from Heisenberg's uncertainty relationship, shows that the 'spread out' in space is the same in all three cases, since I assumed the uncertainty (+/- tol) in speed was the same in all three cases. In the case of the electron moving at 10% c +/- 0.1c, the DeBroglie wavelength is much shorter than the 'spread out' into space.
Notice that in the first case where the electron has no known (average) speed, the 'spread out' is in fact related to the wavelength, being the wavelength divided by 2 pi. It appears that this is how the speed and wavelength of the electron in the Bohr atom is derived.
Assume the 'spread out' of the electron is the Bohr radius. Then from table above 1/2 Bohr radius is used as the position uncertainty in the Heisenberg formula and minimum momentum uncertainty is calculated. With these assumptions we get the classic Bohr atom characteristics: one wavelength fits in an orbit with the Bohr radius and the electron speed is alpha x c.
momentum uncertainty = (hbar/2)/1/2 x Bohr radius
= (6.6 x 10^-34/ 4 pi)/1/2 x 5.29 x 10^-11
= 2.0 x 10^-24
Treat the momentum uncertainty as momentum
wavelength = h/p = 6.6 x 10^-34/2.0 x 10^-24
= 3.3 x 10^-10
= 2 pi x 0.529 x 10^-10
= 2 pi x Bohr radius
v = p/m
= 2.0 x 10^-24/9.1 x 10^-31
= 0.022 x 10^8
= (3 x 10^8)/137
= alpha x c
Derivation of the Bohr radius
The section
above is sort of a derivation of the Bohr radius. A classic way to derive
the Bohr radius is to equate electrostatic attraction (coulomb's law) with
centripital force.
coulomb force = centripital force
[1/(4 pi e0)] e^2/r^2 = m x v^2/r
or equivalently (?) some references say equate electron kinetic and potential energy. It's similar to above with one less r, but there's a factor of 2 problem (due to 1/2 in kinetic energy formula)
electron potential energy = electron kinetic energy
(1/4 pi e0) e^2/r = 1/2 m x v^2
solving for r (using coulomb force = centripital force)
r = [1/(4 pi e0)] e^2/ m x v^2
Of course, without a value for velocity we haven't learned much. In the section above we derived that Heisenberg uncertainty gives a momentum uncertainty, which treated as the minimum momentum leads to v = alpha c
alpha = e^2/(hbar c 4 pi e0)
v = alpha c = e^2/(hbar 4 pi e0)
r = [1/(4 pi e0)] e^2 [hbar 4 pi e0]^2/( m x e^4)
r = (4 pi e0) hbar^2/m e^2
This is the 'official' formula for the Bohr radius = 5.3 x 10^-11 m.
Electron shell radii vs atomic number
I initially
thought I could estimate the shrinkage of electron shells with atomic number
by just modifying the electrostatic attracting term (potential energy)
term in the Bohr radius formula. The attraction force on inner electrons
would increase as atomic number, analogous to the gravitation force on
the surface of a planet going up in proportion to the mass of the planet.
The inner electrons would respond with higher speed, thus decreasing their
wavelength and shrinking the orbit circumference and radius. I also initially
assumed that shell radius would grow as the square of shell number, the
same way the orbit radii increases in excited hydrogen. Here is the calculation:
coulomb force = centripital force
[1/(4 pi e0)] e^2/r^2 = m x v^2/r
[1/(4 pi e0)] e^2/r = m x v^2
[1/(4 pi e0)] e x [charge of nucleus]/r = m x v^2
[1/(4 pi e0)] e^2 x [atomic #]/r = m x v^2
For the inner orbit one wavelength fits in the orbit thus
the velocity is related to the radius by the relation
wavelength = 2 pi r = h/mv
v = h/(2 pi m r)
substituting
[1/(4 pi e0)] e^2 x [atomic #]/r = m x [h/(2 pi m r)]^2
[1/(4 pi e0)] e^2 x [atomic #] = m x [h/(2 pi m)]^2/r
so
r = m x [h/(2 pi m)]^2/[1/(4 pi e0)] e^2 x [atomic #]
= {m h^2 4 pi e0}/{4 pi^2 m^2 e^2 x [atomic #]}
= {e0 h^2}/{pi m e^2 x [atomic #]}
= {e0 4 pi^2 hbar^2}/{pi m e^2 x [atomic #]}
= (4 pi e0) hbar^2/ m e^2 x [atomic #]
but (4 pi e0) hbar^2/ m e^2 = (hydrogen) Bohr radius (see above), hence the inner shell radius is
r = (hydrogen) Bohr radius/[atomic #]
and radius of the outside shell, i.e the radius of the atom, is
atomic radius/hydrogen radius = [# of electron shells]^2 x 1/[atomic #]
This modified Bohr formula predicts the inner shell radius goes down as 1/[atomic #]. It also shows the radius going down as 1/mass of the orbiting charge. This turns out to be exactly right for special case of one 'electron' atoms. (See Muonic Atoms below) A reduction in inner shell radius with atomic # is very fast, and it leads to atoms shrinking as atomic number goes up, which is not quite right. For example, uranium (atomic # 92) has 7 electron shells, but 49 x 1/92 is a smaller outside radius than hydrogen, but measurements show uranium is larger than hydrogen with a radius x6 to x7 bigger. Since electron velocity in hydrogen is near 1% of the speed of light, if the uranium's inner orbit is x92 times smaller then hydrogen's, then electrons in uranium's inner orbit will be moving pretty close to the speed of light. (not something I have ever see written).
I can't find much on electron radial density of atoms. I'm beginning to suspect this is a difficult problem, not easily calculated (or measured?) Wikipedia says only hydrogen can be solved exactly. A Wikipedia element article typically shows two to four radii that often vary over a two to one range.
UpdateThere is an approx method (Hartree-Fock) that can be solved by computer. By 1976 it had been applied to the first 54 elements. I searched and searched, but the only multi-electron atom I could find was neon (atomic #10) (see below). It shows the inner orbit radius (center) to be about 10-15% of a0, where a0 (I presume) is the Bohr radius. Since neon is atomic # 10, the assumption that the inner electron radius goes down as (1/atomic #) may in fact not be too far off.
In a technical bookstore I found an advanced undergraduate atomic physics text book (The Physics of Atoms and Quanta book, by Haken, Wolf, Brewer). It states that the wave equation can be solved for a one electron atom of any atomic # (all but one electron stripped off) and that the modified Bohr formula (including atomic #) does in fact apply. Thus in this case the inner electron orbit radius does indeed go down as 1/[atomic #]!Googling 'hydrogen atom radius' up comes an 'elementary' physics site from Michigan State Univ. They give the solutions of Schroedinger wave equation for an electron in an attractive coulomb potential, where the radius is the average orbital radius in the ground state.
r (hydrogen) = 5.29 x 10^-11 (Bohr radius)
r (atomic # Z) = 5.29 x 10^-11/Z (Bohr radius/Z)
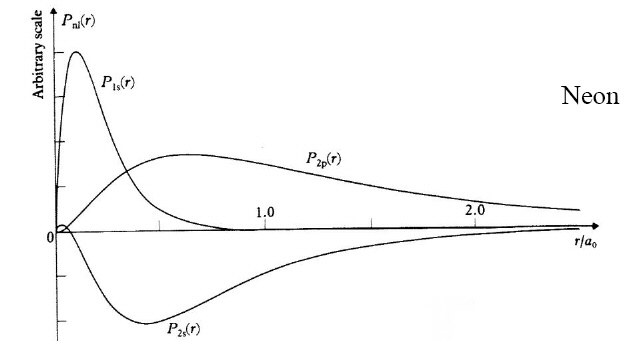
Neon electron shell proability densities,(a0 =Bohr
radius)
(calculated from Hartree-Fock equations, Notre Dame
physics)
Muonic atom
Below is an
set of really tight orbits I found in an atomic physics book. It
is (sort of) a 'one electron' atom, which means the Bohr equation applies
and can be used to calculate the orbits, and the wavefunction can be solved
exactly. The (modified) Bohr equation shows the radius of the inner orbit
is inversely proportional to the charge on the nucleus and inversely proportional
to the mass of the 'electron'. But here the electron is replaced by a muon,
which is basically a heavy electron with the same charge as an electron,
but is 207 times heavier. So in a muonic lead atom the inner radius
is smaller than the hydrogen Bohr radius (5.3 x 10^-11) by 82 (lead atomic
#) x 207 (ratio of muon/electron mass) = 16,974.
Bohr radius (muon & lead nucleus) = 5.3 x 10^-11 m/(82 x 207)
= 5.3 x 10^-11 m/1.7 x 10^4
= 3.1 x 10^-15 m
The amazing thing about the inner orbit (shown in the figure below) is that it shows there is a substantial probability of finding the muon within the nucleus (crosshatched area)! Note the scale is 10^-15 meter, which is the scale of the nucleus. (For some reason, maybe relativistic effects, the Bohr equation above come out to be half the peak of the figure inner orbit probability.) The other interesting thing shown in this figure is that the peak of the excited orbits follows the square rule seen in hydrogen, i.e. for (n = 2,3) the peaks are (pretty close to) x4 and x9 of the calculated 3.1 x 10^-15 inner orbit.
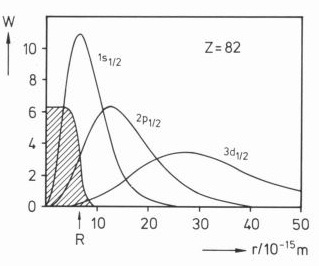
Muon radial (orbit) probability densities with a lead
nucleus (atomic # 82)
Crosshatched region is probability of protons/neutrons
(in the nucleus)
(The Physics of Atoms and Quanta book, by Haken, Wolf,
Brewer)
Bottom line -- Looking at the muon atomic orbits and the (approx) neon orbits, and considering that the fact that outside radius of atoms does not vary much, it must be that electron orbits in atoms shrink a lot as the charge on the nucleus rises, probably not shrinking quite as much as 1/[atomic #], but approaching this factor.
Orbit electrons potential vs kinetic energy
Mystery? ---
When I thought about it, the energy change between orbits didn't seem to
make any sense. One the one hand the DeBroglie wavelength indicated that
the kinetic energy varied (inversely) as radius squared consistent with
the Balmer formula for photon energy. The big problem being kinetic energy
went down as radius went up, when 'everyone knows' that electrons
jump to higher orbits when they absorb energy from photons.
One the other hand higher orbits do have higher potential energy, but I had no idea how the potential energy compared to the kinetic energy. Also the energy difference between orbits was dependent on the difference between a term going up and a term going down, which didn't seem right. So I did a calculation comparing the potential and kinetic energy change between orbits 1 and 2.
First, potential energy:
coulomb force = k x e^2/radius^2
where
k = 1/(4 pi e0) = 9.0 x 10^9
Integral of coulomb force (from infinity) to a distance
(from nucleus) is potential energy.
The radius of the 2nd orbit is four times the radius
of the inner orbit (r2 = 4 x r1).
potential energy @ r1 = k x e^2/r1
potential energy @ r2 = k x e^2/r2
delta potential energy = k x e^2 (1/r1 - 1/r2)
= k x e^2 (1/r1 - 1/4 x r1)
= k x e^2 (1-1/4)/r1
Setting r1 to the Bohr radius (r1 = 5.29 x 10^-11 m)
delta potential energy = k x e^2 x (3/4)/r1
= 9.0 x 10^9 x (1.6 x 10^-19)^2 x (3/4)/5.29 x 10^-11
= 3.27 x 10^-18 joule
(x2 kinetic energy change, see below)
= 3.27 x 10^-18 joule x [1 ev/1.6 x 10^-19 coulomb]
= 20.4 ev (3/4 x 27.2 ev)
Let's compare above to change in kinetic energy from orbit 1 to orbit 2. The electron in orbit 2 is moving half as fast as in orbit 1, so the energy difference is 3/4 of the kinetic energy of orbit 1. We'll take the velocity of orbit 1 to be the speed Sommerfeld found, which is alpha x c.
delta kinetic energy = - (3/4) x (1/2) m v^2
= - (3/8) x 9.1 x 10^-31 x (3 x 10^8/137.036)^2
= - 0.00164 x 10^-15
= - 1.64 x 10^-18
( x 1/2 potential energy change, see above)
The result is very interesting, and solves the mystery. The reduction in kinetic energy is exactly half the increase in potential energy. Potential and kinetic energy changes between orbits are clearly ratioed. In higher orbits (it appears) that exactly half the increase in potential energy is canceled by was a decrease in kinetic energy. Let's see if we find the relationship between potential and kinetic energy. The potential and kinetic energy differences from inner to nth orbit is (rn is radius of nth orbit)
delta kinetic energy = - (1- 1/n^2) x (1/2) m v^2
delta potential energy = (1-1/n^2) k x e^2 /r1
where
r1 = Bohr radius
v = vel @ Bohr radius = alpha x c
but alpha = e^2/(2h c e0), so e^2 = alpha x c2he0
Bohr radius = hbar/m c alpha
k = 1(4 pi e0)
delta potential energy = (1 - 1/n^2) k x alpha x c2he0 hbar/m c alpha
= (1 - 1/n^2) k x m (alpha x c)^2 x 2he0/hbar
= (1 - 1/n^2) k x m (alpha x c)^2 x (4 pi e0)
= (1 - 1/n^2) m x v^2
= 2 x (1 - 1/n^2) (1/2) m x v^2
delta potential energy = - 2 delta kinetic energy
Yup, the formula above shows that in general for the Bohr atom the net increase in electron energy vs orbit radius goes at exactly the magnitude of kinetic energy decreases. The reason being that the potential energy increase rate is exactly twice the kinetic energy decrease rate.
Satellite potential energy vs kinetic energy
After I did
the above analysis, I found there is a general relationship between kinetic
and potential energy for a collection of particles. It's called the virial
theorem. 'Virial' (Latin word) is the (vector) sum of the momentum
x position. For systems where the time average change of the virial is
zero the relationship between kinetic energy and potential energy is this:
potential energy = -2 kinetic energy
It turns out this relationship applies both to electrostatic systems and gravity systems. The relationship is easy to derive for an earth satellite. Think of pushing against a satellite as it falls in from infinity. The work the satellite does pushing against the opposing force is its potential energy, and since it's doing work it's losing energy (from zero), making its potential energy negative.
Potential energy = - integral {force dr}
= - integral {G m1m2/r^2 dr}
= + Gm1m2/r (from infinity to r0)
= - Gm1m2/r0
A satellite's orbital speed is such that its inward centripetal force, which has the same magnitude as outward centrifugal force, is provided by gravitation attraction between the earth (m1) and the satellite (m2).
centripetal force = gravitational force
m2 v^2/r0 = Gm1m2/r0^2
v^2 = Gm1/r0
Kinetic energy = (1/2) m2v^2
= (1/2) Gm1m2/r0
Note the satellite's potential energy (from infinity) comes out to be twice the kinetic energy with the opposite sign. It's true absolutely and true for deltas too, the 1/2 coming from the 1/2 in the kinetic energy formula.
Applying the
rule here's a table of energies for a satellites at radial distances r0
and 9r0 assuming its kinetic energy at r0 is Eo. The last line in the table
is the energy that must be added to the satellite to lift it from
an r0 orbit to a 9r0 orbit. Notice the result is basically the same as
'lifting' an electron in an atom from an inner to x9 larger outer orbit.
In both cases the energy needed is positive because the increase in potential
energy is larger, by exactly a factor of two, than the decrease in kinetic
energy.
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
-2Eo/9 - (-2Eo) = +16/9 Eo | |
In the two cases below, an object falling on earth surface (constant acceleration) and electron energy in hydrogen, all the potential energy (from infinity) is converted to kinetic energy. Conservation of energy.
Earth's surface
Drop an object (initially with zero speed) and measure its speed in one
second. Velocity is a triangle, so in one second speed is 9.8 m/sec. Distance
traveled is area under triangle (1/2) 9.8 m/sec x 1 sec = 4.9 m. Work required
to lift an object 4.9 m is force x distance, so this must be the gain in
potential energy.
Vel @ 1 sec
9.8 m/sec
Kinetic energy
(1/2) mass (9.8 m/sec)^2 = 48 mass m^2/sec^2
delta potential energy
force x distance
mass 9.8 m/sec^2 x 4.9 m = 48 mass m^2/sec^2
No factor of two! All the object's lost potential energy is converted to kinetic energy.
Hydrogen
Hydrogen's electron is well known to have an ionization energy of 13.6
ev, meaning you need to put in 13.6 ev of energy to remove the electron.
In the Bohr model the electron's velocity is c/137.
potential energy
-13.6 ev
speed
alpha c = c/137 = 2.19 x 10^6 m/sec
Kinetic energy
(1/2) m v^2
(1/2) 9.1 x 10^-31 (2.19 x 10^6)^2 x [1 ev/1.6 x 10^-19
coulomb]
13.6 ev
Again no factor of two
Solution
In Wikipedia 'Ionization
Energy' they argue as follows: When an electron falls into the potential
well of a nucleus, there is a loss of potential energy of 2E with half
(E) going into the kinetic energy of the electron and half (E) is considered
a loss of energy by the atom.
In other words I guess what they are saying is that in chemistry when an active atom like oxygen grabs an electron, half the loss of potential energy is released to the outside world (as heat) and half goes into speeding up the electron as it orbits the nucleus.What's so puzzling is that this point is almost never made (or never clearly made). It's really obscure. A search did turn up the following (half assed) reference:Thus an electron falling into orbit around a proton to make a hydrogen atom must lose 27.2 ev of potential energy, i.e. do 27.2 ev of work, resulting in it acquiring 13.6 ev of kinetic energy and (important part) the proton-electron system releasing 13.6 ev of heat!
Corollary
The corollary is very interesting. The ionization energy of hydrogen is 13.6 ev, meaning to remove the electron (at zero final speed) 13.6 ev of energy must be supplied. But it must take 27.2 ev to climb out of the potential hole the electron is in, because that was the amount of work the electron did as it fell in. Hence the remaining 13.6 ev needed to climb out of the well must be recovered from the kinetic energy of the electron!In other words (I suspect it must be a general rule) that when an atom is ionized half the energy needed to climb out of the potential well must come from the kinetic energy of the electron.
-- "13.6 eV (from a light flash) is just enough to tear the electron away (from hydrogen), so in the end the proton and electron are at rest far away from each other. The energy of the light was used up dragging the proton and electron apart—that is, it went into potential energy. It should be mentioned that the electron also loses kinetic energy in this process, 13.6 ev is the net energy required to break up the atom." (Virginia college physics notes)
I am not sure
why the object drop on the earth's surface does not show the factor of
2, but I suspect it's because I assumed that force is constant. It
may very well be that [potential energy = twice kinetic energy] only applies
in the case of inverse square force potential wells.
---------------------------
How big do atoms grow?
From the table
above it would appear that the size of an atom, if defined by the radius
of its outer electron orbits, should expand a lot if it has electrons in
higher orbits. For example, atoms with electrons in '4' orbits look like
they would be x16 dia of atoms with electrons in only '1' orbits. Is this
true? It seems inconsistent with the general rule that the atomic size
of most elements falls in a narrow range.
I need to research this, but I think the hydrogen atom can grow. When its electrons acquire energy and jump into higher orbits, the size of the hydrogen atms does indeed swell considerably as indicated in the table.
However, for higher elements (I think) there is an other factor in play. Higher positive charge in the nucleus tightens all the electron orbits. So while electron orbits in higher elements probably do follow the x4, x9, x16 etc ratio rule, this radial swelling is largely balanced out by radial shrinkage (in all orbits) due to higher positive charge in the nucleus.
Below is a
plot of atomic radii I found. (I don't what criteria they used for size.)
It shows several interesting things:
a) Hydrogen
radius (4 x 10^-11) is pretty close to the Bohr radius.
b) Helium
is nearly the same size as hydrogen, so the two electrons of helium are
pretty much in the same radial space.
c) There are
big jumps in radius (3:1 to 2:1) when the first electron goes into the
next higher quantum n orbit. These occur at lithium, sodium, potassium,
rubidium and cesium, which in the periodic table are all listed on the
left side under hydrogen. There is a 7th orbit that starts at element francium,
but it is element 87 which is above the range of this figure.
d) Higher
elements (after a radius jump) with more electrons in the same orbit then
shrink the size (factor of 1.5 to 2) as more positive charge in the nucleus
pulls all the orbits inward.
e) Elements
high in the periodic chart with electrons six orbits out from hydrogen
are about x4 times larger than hydrogen, not x36 times larger. This shows
that large positive charge in the nucleus of elements high in the periodic
chart has pulled in all the electron orbit radii by about a factor of 36/4
= 9.
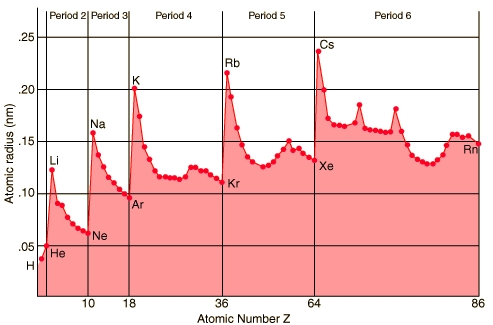
Electron probability 'smear'
Feynman says
in the book QED that the vagueness in location of electrons in atoms is
an example of the uncertainty principle, but there is no need to
use the uncertainty principle in this case because QED explains it directly.
In example after example in QED the probability of finding a particle or
photon is high over a distance range of +/- 90 degrees (approx) of
the particle frequency (technically the phase of the probability amplitude),
which is a distance range of (about) 1/2 wavelength.
The lowest orbital hydrogen electron has a speed (about 1% speed of light) and radius such that one wavelenght fits into its circumference. So a QED probability calculation that is high over (about) 1/2 wavelength range means that an electron in an atom can basically be anywhere in its orbital, hence is generally referred to as 'smeared out'.
Feynman's QED lectures
Feynman's famous
1985 book QED is based closely on a series of four lectures he gave for
the general public to explain the modern, probabilistic theory of light
and electrons that he (& others) had developed in the late 40"s and
which won him (& others) the Nobel prize. These four lectures, a 'warm
up' if you will, were first given in New Zealand in 1979 and were captured
on video and can be watched (streamed) from this link. Each lecture (with
questions) is about 90 minutes.
Most references for these lectures link to Vega.org (below), which steams the video from New Zealand. They have all four lectures. In several attempts I have found this New Zealand video stream to be barely watchable because it repeatedly hangs, and the resolution is not very good.
http://vega.org.uk/video/programme/46
Google video
I recently
discovered another source for the video stream (Google video), and it plays
cleanly, but unfortunately Google video appears to have only one of these
four lectures, lecture #1. Google video does, however, have other Feynman
lectures..
http://video.google.com/videoplay?docid=-8459058620572291798
Leonard Susskind on quantum mechanics
From reading
two of his books I have discovered Standford physicist Leonard Susskind
is a great writer and explainer of difficult physics. So I was pleased
to discover that there is available on video six of his lectures on quantum
mechanics. This lecture series is on the CosmoLearning site (whoever they
are), which has of lectures in a wide variety of science fields.
http://www.cosmolearning.com/physics/courses/Modern_Physics:_Quantum_Mechanics/
http://www.cosmolearning.com/
QED basics
QED figures
the probability amplitude of all possible way an interaction (between
particles) can take place. Each amplitude is a vector with a length and
an angle. Feynman in his book uses the image of a rapidly rotating stopwatch
to visualize the accumulated phase of a photon (see below). How much phase
is accumulated depends on the travel time (from the source to detector)
and the frequency of the light. The probability amplitude vectors are added
for all the possible paths producing one final probability amplitude
vector. The probability of the event is found by squaring the length
of the final vector.
{Later in QED Feynman says that his stopwatch turning (increasing phase) does not happen during photon travel time as he first indicated. It actually comes from linearly increasing phase of his monochromatic light source, which is a source with a dominant single frequency. Evidently it emits photons with a phase that follows its dominant sinewave frequency. Hence the phase of the amplitude arrows used to calculate the probability depend on the emission time from the source. The result (I think) is exactly the same as if you think about the stopwatch turning during photon travel time, which is very likely why he used the image initially.}The only paths that contribute (significantly) to the final amplitude vector are those where the phases (i.e. travel times) are nearly the same (within about +/- 1/4 wavelength). Adding paths with significantly more phase just causes the 'tips' of the final amplitude vector to curl about in tiny circles, thus having relatively little effect on the probability.
In QED the probability amplitude of all photon/electron interactions are calculated using two numbers that Feynman calls j and n. j is the probability amplitude that a photon and electron will exchange (either emit or absorb) a photon. Feynman generally characterizes j as just a number with a value of (about) 0.1 (well it's really -0.1 because there is also a 180 phase change). Adding a one photon exchange possibility to a zero photon exchange possibility increases the probability no more than (about) 20%, since {(0.3 + 0.03)^2/0.3^2 = 1.21}. Similarly adding a two photon exchange increases probability no more than (about) 2%, since (0.3 +0.003)^2/0.3^2 = 1.020}.
j and n, while
nominally representing charge and mass, are idealized. Real electrons and
photons have some probability of doing odd things. For example, an electron
can emit and reabsorb a photon, and a photon can change into a positron/electron
pair which then recombine. Any experimentally measured value of electron
charge or mass will include these 'correction factors', hence n in included
to some extent in measured e (charge), and j to some extent in measured
m (mass).
|
|
|
|
|
|
|
|
|
|
|
|
|
|
or coupling constant |
|
|
-sqrt{1/137.036} =
|
In QED Feynman says, "This number (j), the amplitude to emit or absorb a photon, is sometimes called the charge of a particle." (QED footnote, p91). Unfortunately in the book he does not make clear what units he is using. Planck units are a set of natural units and planck charge is a base planck unit. It turns out that e and planck charge are related by the sqrt of fine structure constant, e = sqrt{1/137.036} x planck charge, where sqrt{1/137.036} = 0.08542. So it looks like Feynman is probably using a unit system where planck charge (& all planck units?) is defined as unity, then his j as charge will have the value -0.08542.
Something I
don't understand (or is very surprising) is that the amplitude of an electron
absorbing or emitting a photon seems to be independent of the energy of
the photon or electron. An energized electron must be more likely to emit
a photon, so how does QED handle this. Also I researched gamma ray photon
energies and they go way up there (GEV and TEV), much much higher than
the rest mass of an electron (0.511 Mev). An electron absorbing one of
these photon is going to recoil at the speed of light.
===================================================================
Atomic magnetic effects -- big picture
Man, what
a job it is to unscramble and understand magnetic effects within atoms!
It's remarkably complicated and no reference seems to address the big picture,
it's all details. To wit:
#1 --- At the particle level what is the source of the permanent magnetic field (PM) that we know from the PM on our refrigerator? Are there little PM's within atoms? (Answer: Yes)
There are three different types of PM's within atoms: Each electron has an intrinsic magnetic moment (essentially a little magnet), the motion of each electron around the nucleus is a 'current', so this creates an orbital magnetic moment, finally each proton and neutron in the nucleus has its own intrinsic magnetic moment. (Going a level deeper the magnetic moments of the protons and neutrons come from the intrinsic dipole moments and motion of the 'up' and 'down' quarks that make up protons and neutrons, but thankfully we can just look at this at the proton and neutron level ignoring the quarks within.) And the magnitude of these three magnetic moments are roughly comparable.
Pairing
An important
consideration is that both electrons and nucleons (protons or neutrons)
will (if possible) pair with a like particle such that their magnetic
fields are opposed. The cause of this pairing (almost for sure) is that
it's a lower energy state because the magnetic field lines locally circulate
rather than projecting out (see figure below) so the energy stored in the
magnetic field is reduced. Pairing explains why electrons in filled
shells and atomic nucleuses with an even number of protons and neutrons
have zero net magnetic moment.
source -- 'Evolution of Pulsar Magnetic Fields', by
Flowers and Ruderman, Astrophysical Journal July 1977
Two electrons in the same orbital space always pair spin up and spin down, this is the Pauli exclusion principal. However, the fact that electrons in inner shells, which are all paired, contribute zero magnetic moment to the atom must mean that their orbital magnetic moments also cancel (though I have yet to see any reference say this explicitly). For two paired electrons to have no net angular orbital momentum they must (veiwed classically) be counter rotating, i.e. they going around the nucleus in the opposite directions.
I have see no details as to how nucleons arrange themselves so that for even numbers their intrinsic magnetic moments fully cancel. Can they can just 'flip over' so the magnetic fields cancel fields and energy is lowered? Also I don't know if they really pair, as electrons clearly do, or whether they just arrange half 'up' and half 'down'.
Bottom line --- Wikipedia says that the measurable magnetic field of (ferromagnetic) PM's is due only to the intrinsic magnetic moment of unpaired electrons.
"A permanent magnet, such as a bar magnet, owes its magnetism to the intrinsic magnetic dipole moment of the electron." (Wikipedia 'Dipole')The implication is that the magnetic field of the the nucleus (if odd) contributes nothing, and the orbital angular momentum of unpaired electrons contributes nothing. Why is this?
Of course, if a material is to have a measurable external magnetic field there must be some mechanism (probably lower energy) operating at the atomic level for the electrons or nuclei to align. Maybe this mechanism is only strong enough to produce alignment in the case of the electron intrinsic magnetic moment. I have yet to see any discussion of why some magnetic moments align and some don't.
#2 --- How come so few elements (mainly those labeled 'ferromagnetic') at the macro level have a measurable magnetic field? What's so different about the few ferromagnetic elements?
#3 --- How do atoms, electrons, or nucleons respond to external magnetic fields? How do they respond to DC fields, AC fields diverging magnetic fields? How to they respond when moving, when stationary? Obviously, this is a huge field. Probing atoms and particles with many kinds of magnetic fields have revealed a lot about their nature and led to several practical devices.
Tests with external magnetic fields have revealed that the angular momentum of all particles is quantized with a proportionality of hbar or (1/2) hbar. This is key data underlying quantum theory. Tests include changes in the motion of atoms (& particles) due to external magnetic fields and changes (line splitting) in the spectral emissions of atoms as electrons jump orbits. In the former case a diverging magnetic field directly applies torque to the internal magnetic moments of moving atoms changing their course. In the latter case an external magnetic field changes the potential energy landscape within atoms. This (slightly) changing the energy of electrons within the atoms and consequently shifts (slightly) the frequency of spectral lines due to jumps into or out of the orbits affected. Historically both techniques have been critical to understanding of the interior of atoms and the nature of fundamental particles.
Manipulation of nucleus magnetic moments with high frequency magnetic fields is the key mechanism in MRI (magnetic resonance imaging), which produces detail pictures of soft tissue widely used in medicine. Atomic clocks are another device based on manipulation of atoms with external magnetic fields.
Electron magnetic (dipole) moment
Each individual
electron 'outputs' a magnetic field. The proof of this is likely on your
refrigerator. The root source of magnetic field of a PM (permanent magnet)
is the magnetic fields of the electrons it contains. Wikipedia puts it
this way:
"In addition to current loops, the electron, among other fundamental particles, is said to have a magnetic dipole moment. This is because it generates a magnetic field which is identical to that generated by a very small current loop. However, to the best of our knowledge, the electron's magnetic moment is not due to a current loop, but is instead an intrinsic property of the electron (meaning its 'spin').Current flowing in a loop creates a magnetic field that flows up through the loop. The magnetic field lines circle back around the outside of the current loop and come back again into the bottom forming compete paths. The magnetic field of a current loop (& electron) is the same as a tiny bar magnetic, and like small bar magnets can be lined up (N to S) to form larger magnets.A permanent magnet, such as a bar magnet, owes its magnetism to the intrinsic magnetic dipole moment of the electron." (Wikipedia 'Dipole')
"The spin of charged particles is associated with a magnetic dipole moment in a way (g-factor different from 1) that is incompatible with classical physics." (Wikipedia 'Spin')
Magnetic moment
Mathematically
the magnetic moment of a current loop is
u = I x area of loop
amp x m^2
(or equivalently joule/tesla).
Unit check
joule/tesla = (i v time)/tesla
= (i dflux/dt time)/tesla
= i BA/B
= i A
checks
This equation looks strange to me, what is the magnetic moment really? A clue is that all the magnetic moments (in a cubic meter) add to M (magnetization), and M has the units of H, because for materials
B = u0 (H +M)
Maxwell's equations tell us the line integral of H is equal to the current enclosed, so (as shown below) the unit of H come out to be amp/meter. Below is the integral for a circular H path around a current (in a wire).
Integ{H ds} = I
H = I/(2 pi r)
amp/meter
Magnetic moment => incremental M
Magnetic moment
has units of amp x meter^2. How is that related to H?. The key is that
M of a material is found by multiplying magnetic moment (amp x meter^2)
of an atom times (number of atoms/meter^3), which results in amp/m,
the same as H.
Here is the calculation of M for iron (assumes magnetic field of all atoms are lined up). The measured dipole moment of iron is 2.22 ub (2.22 Bohr magnetron). Magnetization (M) is defined as the quantity of magnetic moment per unit volume.
M = # of iron atoms/m^3 x 2.22 x ub
= 8.5 x 10^28 atoms/m^3 x 2.22 x 9.25 x 10^-24 Am^2
= 17.4 x 10^5 A/m
Magnetic moment of hydrogen
-- "The magnetic
moment of a hydrogen atom (in ground state) is zero. (http://en.wikipedia.org/wiki/Stern–Gerlach_experiment))
Angular momentum => magnetic moment
There is a close relationship between magnetic moment and orbital angular
momentum. Classically the vector equations for torque (T) and angular momentum
(L) are similar:
T = r cross force
L = r cross p
where
p = mv (linear momentum)
And for an orbiting electron there is a close relationship between its orbital angular momentum and magnetic moment (u orbital)
u orbital = -(e/2m) L Hyperphysics
check (for a circular Bohr orbit)
L = rp = rmv
i = -e/orbit time
= -e/(2 pi r/v)
= -ev/(2 pi r)
u orbital = i x area
= -ev/(2 pi r) x (pi/4) 4 r^2
= -evr/2
substitute for v
u orbital = (-er/2) x v
= (-er/2) x (L/rm)
= -(e/2m) L
checks
This (I think) is a very general relationship. It says little incremental M's (magnetic moments) are really just electron orbital angular momentum(s) scaled by charge and mass.
Momentum is quantized
L is quantized
because only integer wavelengths (wavelength = h/p) are allowed in atomic
orbits (n = 1,2,3..). Radius goes up as n^2 and velocity goes down as (1/n),
so (scaling from inner Bohr orbit, r=a0)
L = rmv
= (n^2 a0) m (c alpha)/n
= n a0 m c alpha
but a0 = (hbar/mc)/alpha = hbar/(m c alpha), so for spherical orbits
= n [hbar/(m c alpha)] m c alpha
= n hbar
where n = 1,2,3...
yup, (References say this what Bohr postulated
in defining his model in 1913, but this may
not be the modern equation)
Sommerfeld elliptical orbits
Sommerfeld
added a second quantum number (l ) by thinking in terms of electron orbits
being ellipses. In this model each Bohr spherical orbit is replaced
by a set of elliptical orbits with the spherical orbit being a special
case. In a sense Sommerfeld elliptical orbits 'split' the Bohr orbits in
the same way that spectral lines were split.
"In elliptical orbits, electrons pass closer to the nucleus than in circular orbits. Accordingly, electrons would travel faster as they approached the nucleus. By applying special relativity to the set of allowed elliptical orbits, Sommerfeld obtained the same fine structure of energy levels observed in hydrogen." (http://www.omniinstruments.com/old.html)Don't know the proof of this, but reference say the most circular of the elliptical orbits (l = n-1) has the highest angular momentum.
Quantum numbers
At its most basic understanding the four quantum quantum numbers that describe
electron orbits is not really hard. The first three fall out of solutions
to schrodinger's wave equation in polar coordinates, the principle quantum
number (n) out of the radial equation. The last quantum number was added
ad hoc because spectroscopiclally two electrons were found in each orbit,
and it was later found to describe the direction of the electron's intrinsic
magnetic moment.
(A really annoying detail, though, is that even though the quantum concept has been around for nearly a decade, physicists have not standardized on either the notation, or even the names(!), of the four quantum numbers. For example, the 2nd quantum number has at least three names, and most references have it ranging from 0 to n-1, but my new quantum textbook has it ranging from 1 to n and calls it n-theta!!)
* 1st (n)
Principal quantum number (n = 1,2,3...)
Specifies how close (for a given Z) the electron is to the nucleus.
Thus it specifies
the velocity and most importantly the (kinetic and potential) energy of
(a set
of) orbits.
Orbits with the same 'n' will typically have different shapes, but the
same
energy.
* 2nd (l)
Orbital quantum number (l = 1,2,3 ... n-1)
(same as azimuthal quantum number & angular quantum number)
In classical terms specifies the how elliptical the orbit is, which
is equivalent to
specifying orbital angular momentum (L = hbar sqrt{l(l+1)}) of the
orbit
and the magnetic moment.
Somewhat counter intuitively spherical ('s') distribution, which have l
= 0,
have the most elliptical electron orbits, and zero angular
momentum (L).
At the n = 2 level l = 1 yields three double-lobed (probability) distributions.
The orbital distributions get more and more complicated as l rises.
Conventional orbit letters (s,p,d,f, g, h) correspond to l values as follows:
s (l = 0), p (l =1), d (l = 2), f (l = 3, g (l = 4), h (l =5)
* 3rd (ml)
Magnetic quantum number (ml = -l, -l+1, .. 0...l-1, l)
Specifies the orientation in space of the elliptical orbits. At
the n = 2 level it is
the 'ml' quantum number that specifies x, y, or z orientation of double
lobed
probability distribution.
The z component of the angular momentum is proportional to 'ml', which
makes z magnetic moment also proportional to ml.
Lz = ml hbar
uz = - ml ub where ub is Bohr magnetron and
z axis is the orientation of an external measurement
magnetic field
* 4th (ms)
Spin quantum number (ms = +1/2, -1/2)
Specifies the direction of the intrinsic angular momentum (and magnetic
moment) of the electron.
Has only two values of spin up (ms = +1/2) or down
(ms = -1/2).
Electrons like to pair up with down (in same orbital space) because this
has
lowest energy, typically each orbit two electrons with opposite spins.
Slides below are from Univ of Wisconsin physic (undergraduate physics)
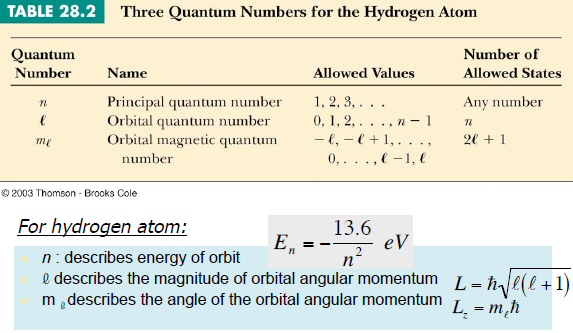
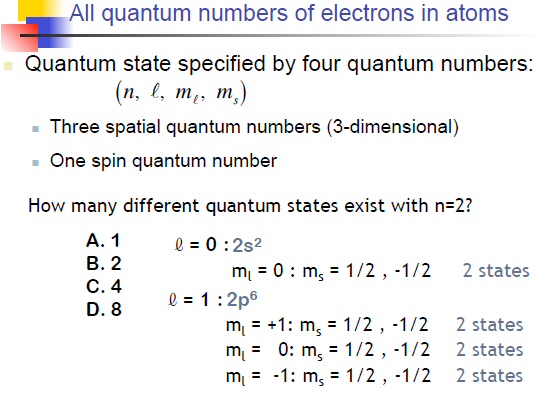

Below is a scan from my new quantum textbook, n-theta is labeled as the 'azimouthal' quantum number and it confirms that lowest values are most elliptical, and the maximum gives a Bohr circular orbit. All the orbits with same n (principal quantum number) have the same total energy with (in classical terms) the energy shifting between KE and PE as the electrons orbits similar to a comet.
Further confusion
In my new
quantum physics textbook (Eisberg) I find the amazing two statements about
the azimuthal quantum number
* n-theta is "called the azimuthal quantum number" (p115)
where
n-theta (see below) = 1,2,3 to n
* "l is sometimes called the azimuthal quantum number" (p 240)
where
l = 0,1,2,3 to n-1
Do you see a problem here? On p115 the 'azimuthal quantum number' starts at 1 and extends to n, and p240 it starts at 0 and extends to n-1, which is the usual range of l. Good grief! (It's not a misprint, because I saw a similar analysis in a 1985 book by Gamow).
What it means I think is that the 'azimuthal quantum number' has at some point been redefined since Sommerfeld. The 2nd quantum number 'l' falls out of a solution of the schrodinger's equation in three dimensions. There appears to be some subtle difference between the two usages. I am amazed that Eisberg in a 2nd edition book doesn't explain this. So is Sommerfeld's n-theta azimuthal quantum number just equal to l +1??
Sommerfeld elliptical orbits
Bohr showed
that the radius of spherical orbits go as n^2, so the ratio of n = 3 to
n = 2 orbits would be 3^2/2^2 = 9/4 = 2.25. The elliptical Sommerfeld orbits
below are drawn to scale. Notice that it shows the elliptical orbits
also scale in size as n^2, the same as (Bohr) spherical orbits. Another
interesting thing this figure shows, which is important (I think) to molecular
bonding is that the most elliptical orbits (l = 0, n-theta = 1) extend
about twice the distance from the nucleus as do the spherical (s)
orbits (for a given n).
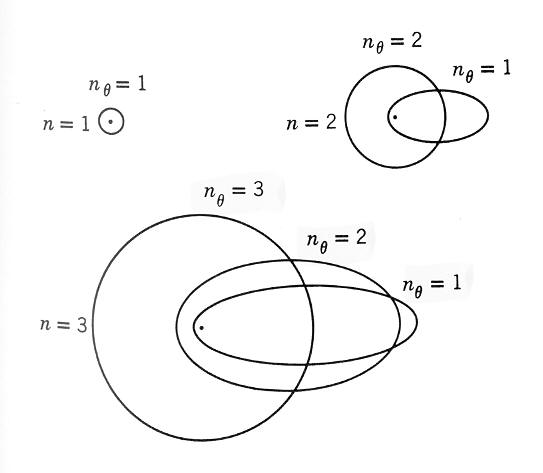
Bohr-Sommerfeld hydrogen elliptical orbits (to scale)
n-theta is supposedly 'azimuth' quantum number (equal
to l + 1 ?)
(scan from my quantum physics textbook Eisberg)
Sommerfeld finds alpha
Sommerfeld assumed
elliptical orbits and quantized both the radial and angular momentum with
two integers: n-theta and n-r. He calculated the formulas for major (a)
and minor (b) axis of the ellipse to be:
a = 4 pi e0 hbar^2 n^2/(u Z e^2)
where
n = principal quantum number
u = reduced mass of electron
b = a (n-theta/n)
where
n-theta = 1,2,3...n
E = - u Z^2 e^4/((4 pi e0)^2 x 2 hbar^2 n^2)
In other words the major axis of the ellipse varies only as n^2 (as in Bohr circular orbits) with n-theta scaling the minor axis to be various fractions of the major axis. For example, if n =3, then minor axis can be 1/3rd, 2/3rd and 3/3rd of the major axis (see figure below), the latter being Bohr's circular orbit . The energy (E) of all the orbits shown above come out to depend only on n.
However, this did not explain the fine structure splitting of the spectral lines, about 1 part in 10^4, which was the purpose of extending the orbit from circular to elliptical. Bohr had found the velocity of the electron (inner orbit) was about 1% c. When sommerfeld included the small relativistic mass increase of the electron he found E included a (v/c)^2 correction term (10^-4 term) as a function of n-theta, which explained the measured the fine splitting of the spectral lines. His new E formula was the E above scaled:
E = - [u Z^2 e^4/((4 pi e0)^2 x 2 hbar^2 n^2)] x [1 + (alpha^2 Z^2/n) (1/n-theta
- 3/4n)]
where
alpha = [1/(4 pi e0)] e^2/(hbar c) = 1/137 approx
And for the
first time the fine structure constant (alpha) appeared in physics.
--------------------------------
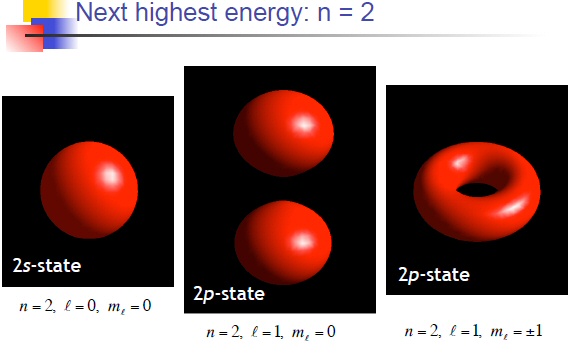
Notice the surprising result here. Spherical orbits (s orbits) have l = 0 and references say (see above) that l = 0 orbits are the most elliptical (least angular momentum). While at first glance a spherical probability distribution (left) looks like it should mean circular type orbits, apparently the opposite the case. Perhaps a good classical analogy is the highly elliptical orbits of stars that make up a spherical globular cluster of stars. It is the lobed 'p' orbits (center) that have more circular orbits with higher angular momentum.
Amazingly almost every orbital applet and drawing I see looks different. I finally figured out one reason some probability distributions look funny, like the torroidal distribution above right. Is is actually two distributions, a double lobed (m = +1) and double lobed (m = -1) drawn combined (very confusing). Above is also poor because an inherently fuzzy distribution have been idealized with a hard surface that doesn't exist. Below are the same states as above, but from the much more accurate Indiana University Southeast applet (right below does not include m = - 1).
My 2nd quantum physics textbook (Eisberg) says, "If all the probability densities for a given n and l are combined, the result is spherically symmetrical." In other words the three double lobed distributions below center ( m = 0) plus right (m = 1) plus (not shown m = -1) when combined are spherically symmetrical.
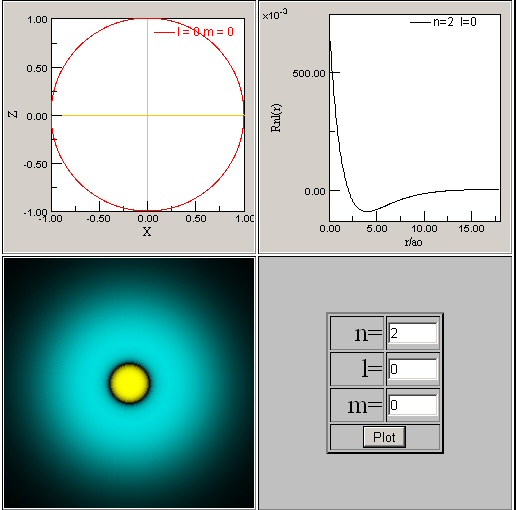 .
.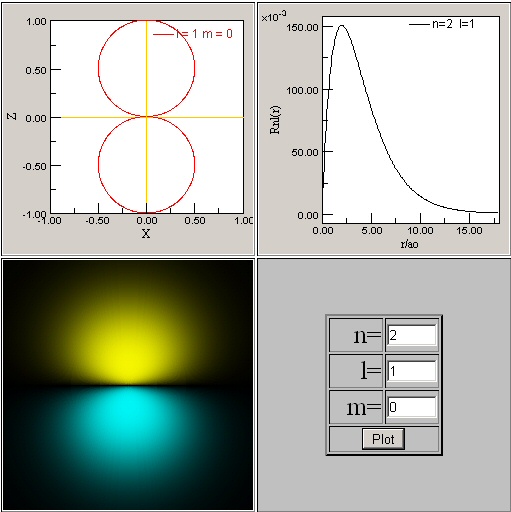 .
.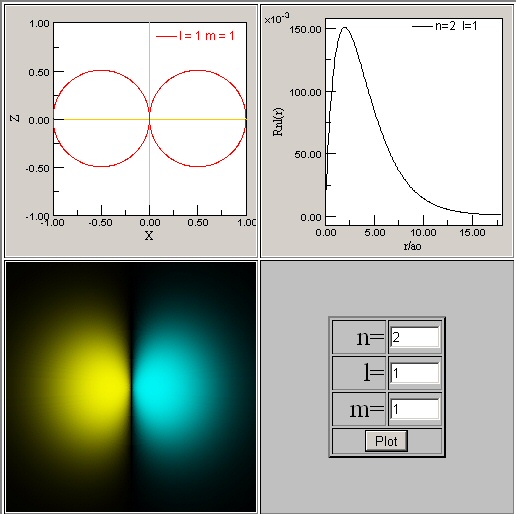
n = 2, l = 0, m = 0
n = 2, l = 1, m =0
n = 2, l = 1, m = 1
One electron orbital applet
Much better
than above is the one electron atom orbital applet online from Indiana
University Southeast physics (below). It shows wavefunctions on top and
probability distribution bottom. While n only goes up to 7 (or 8) in atoms
Schrodinger's equation has no such limit and the applet will work for n
up to 50. Playing around with it is interesting and I noticed some general
patterns.
 .
. 
One electron orbital applet from Indiana University
Southeast physics
top = wavefunctions (angular left, radial right)
bot = probability density (x-z plane)
http://physics.ius.edu/~kyle/physlets/quantum/hydrogen.html
High n means high energy, which means a high frequency and lots of standing waves. When l = (n - 1), which is highest angular momentum, there are lots of standing waves angularly, but only one standing wave radially (see left). As l is lowered, the radial waveform starts to look like a damped spacial sinusoid (see right). Now there are standing waves radially as well as angularly, and as l drops the number of angular nulls decreases.
I find the pattern is the number of radial probability density lobes is always equal to (n - l). Note left has one radial lobe, where n =7 and l = 6, and five radial lobes on right where n = 7 and l = 2. (The same pattern exists for n = 20, one radial lobe for l = 19 and five lobes for l = 15.)
When l = (n-1), which is maximum, the radial distribution is always has a single lobe that peak (close to) n^2 x a0. The angular distribution has (about) (2 x n) lobes around a circle for low m, and which get fewer as m increases. In all cases down to two lobes when m = l, four lobes at m = (l - 1), six lobes at m = (l - 3), etc..

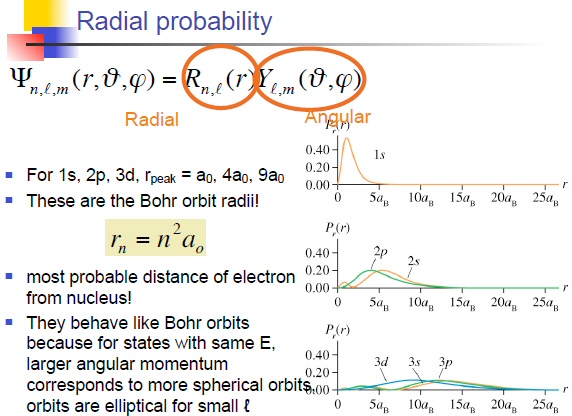
Last electron orbit of first 36 elements
Element 21 scandium (Sc) 21st electron drops down
into n = 3 level (3 d1)
Element 30 zinc (last 'transition metal') fills n
= 3 level (3d10) for a total of 18
Periodic table of elements showing electron configuration
for each element
Orbit notation inside a box is a deviation from the
normal sequence,
For example, element #23 => #24 removes a 4s electron
and adds two 3d electrons
element #23 vanadium V
Argon 4s2 3d3
element #24 cromium Cr
Argon 4s1 3d5
element #25 manganese
Mn Argon 4s2 3d5
(scan from atomic quantum textbook)
Orbits of elements fill in this order (Wikipedia --
Klechkowski_rule)
Below is a scan from my atomic quantum textbook showing the order in which atomic shells fill. It is basically the same information as above, but more understandable. It shows all the 'p' orbits have slightly more (relative) energy when outside compared to inside, the adjustment in the 'd' orbits is larger, leading to crossovers in how orbits fill, and the adjustment in 'f' orbits is larger still. So the 3d shell when outside having higher energy than the 4s shell causes the 4s shell to fill first.
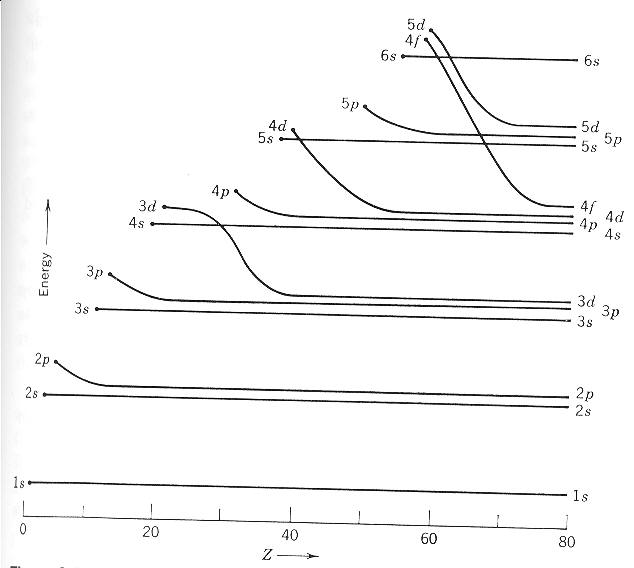
Relative energy of atomic shells (up to mercury #80)
Shows the relative increase in energy when shells
are outside vs inside
Above figure is misleading. The vertical axis is labeled 'energy', but is really relative energy. (There was a little footnote under this figure saying 'energy varies with Z and the energy scale is non-linear'.) As Z goes up the inner orbits shrink and the electrons move faster, so if the vertical axis was energy, rather than relative energy, all the curves would show a strong upward tilt.
Total angular momentum
After a lot
of work, think I have finally gotten a handle on angular momentum
for an electron in an atom. It's explained quite well on the hyperphysics
site, not very well on Wikipedia. Here's a good Hyperphysics entry link:
http://hyperphysics.phy-astr.gsu.edu/hbase/quantum/vecmod.html#c2
As the above link makes clear, the two angular momentums of the electron, orbital and intrinsic, are added vectorially to get a total angular momentum vector. The picture presented (see below) is of vectors that precess about an externally applied magnetic field. There is also a frequency (measurable?) associated with the precession rate. The external magnetic field is important because it defines the orientation of the z axis, which is repeatedly and confusingly talked about. And why this is relevant (I think) because is because it is the component of total momentum along the external magnetic field direction, so called z-axis, that is measurable.
Notice below it looks like the z-axis components are simply added. Yup, Hyperphysics shows that the z component of total angular momentum (Jz) ranges from hbar x (-l +/- 1/2 to +l +/- 1/2), where the +/- 1/2 is the spin angular momentum z component.
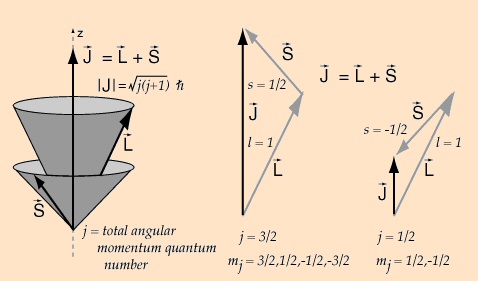
S = electron spin (intrinsic) angular momentum
L = electron orbital angular momentum
(http://hyperphysics.phy-astr.gsu.edu/hbase/quantum/vecmod.html#c2)
Angular momentum of inner orbits
References
say the magnetic moment (net angular momentum) of atoms is due only
to
outer electrons. But I have yet to find any clear explanation as to why
the net angular momentum of inner orbits is (exactly) zero. All inner electrons
are paired in up/down spin pairs, so it's very reasonable that the net
spin angular momentum (S) of inner electrons is zero. But what about the
orbital angular momentum of inner electrons? Why does this sum to zero?
(My speculation is that quantum analysis of paired up/down elections occupy
the same space forces a null orbital angular momentum)
Historic angular momentum/spin test --- Stern Gerlach
experiment
This is a
classic experiment that demonstrates that electrons have (intrinsic) angular
momentum and that it is quantized with proportionality of hbar. It
was first done in 1922 (by Stern & Gerlach), so it provided a key piece
of data underlying the theory of quantum mechanics, which was developed
a few years later. It apparently can be done with atoms or free electrons
(a voltage used to cancel the magnetic bending).
This experiment is a deflection of a moving particle (with intrinsic angular momentum) up or down by a specific amount in the direction of an applied (in-homogeneous) magnetic field. Normally about 50% of the particles deflect 50% up and 50% down. This is interpreted as due to angular momentum (spin) of the electrons (or electrons in atoms), which somehow, basically miraculously, seem to align themselves with the direction of the external magnetic field, such that about half of electrons are seen to be 'spin up' and half 'spin down'.
The atoms with some velocity travel a long path through an 'in-homogeneous' (see figure) PM field. Originally silver atoms, partly because they could be easily detected using photographic film. The reason that the PM magnetic field is made in-homogeneous is explained thusly by Wikipedia:
If the particle travels in a homogeneous magnetic field, the forces exerted on opposite ends of the dipole cancel each other out and the trajectory of the particle is unaffected. If the particle travels through an in-homogeneous magnetic field, then the force on one end of the dipole will be slightly greater than the opposing force on the other end of the dipole. (Causing the path of particle to be diverted along one axis in an amount that depends on the angular momentum).
Surprisingly (to me) the figure above shows the in-homogeneity of the PM field is side to side, perpendicular to the direction of travel!
Why intrinsic angular momentum?A classical result of the experiment would be that the (vertical) deflection would vary smoothly up or down, because the orientation of the atom angular momentum vector (relative to the PM field) should be random with the maximum deflection providing a measure of the angular momentum. But what is found is that the deflection is quantized. There are two lines on the film, meaning (about) half the atoms deflect up and half down by the same magnitude. So not only is the angular momentum quantized, but miraculously the angular momentum vector of the unpaired outer electron in the silver atom seems to align with the external magnetic field!
The claim is made that the Stern Gerlach experiment measures (only?) the intrinsic angular momentum of (one?) electron in an atom being tested. But doesn't this depend crucially on the atom chosen? And it appears to mean that the measured angular momentum can be assigned to only one electron, and the orbital angular momentum of all the electrons is null. (Or is the explanation that the intrinsic angular momentum is much larger than the orbital angular momentum?)The atom used by Stern and Gerlach was silver, which is element #47. According to Wikipedia silver has an electron shell structure: 2, 8, 18, 18, 1, so it has only one electron not in a filled shell. The net angular momentum of all the filled shells is null, but how can it be that the orbital angular momentum of the unpaired outer electron does not contribute to the measured angular momentum?
Triple Stern Gerlach structure --- electron spin filter
As shown in
the images below a spin electron filter can be built by a triple Stern
Gerlach structure (NSN PM). A sequence of NSN magnets can be designed such
that
all the electrons deflect in the center, either up or down
depending on spin, and then reverse direction coming out on the same axis
they went in. As shown, when the electrons physically separate (by
spin), one of the streams can be blocked. The result is a 'electron spin
filter'. Put in random electrons and about half come out all with the same
spin, which is miraculously aligned with the direction of the magnetic
field in the filter.
 .
. 
Triple Stern Gerlach PM structure is used to separate
the electron beam into spin up and down components
and then recombine it. Here the spin down component
is blocked to make a 'spin up filter'
(Univ of Toronto Physic Dept)
http://www.upscale.utoronto.ca/GeneralInterest/Harrison/SternGerlach/SternGerlach.html
Electron spin filters in cascade
The quantum
nature of electrons is further revealed when spin filters are put in cascade.
In the first figure below half the electrons come out of filter #1 all
with spin up, but these are all blocked by filter #2, which only passes
spin down electrons. "So, evidently once the first filter defines 'up'
that definition is the second filter's definition of 'down.'"
In the second figure the second filter is oriented 90 degrees. Now 1/2 of the so-called 'spin up' electrons coming out of the first filter make it through the second 'side' filter. The second filter appears to have redefined 'up'. When a third 'down' filter is added, the same thing happens again, 1/2 of the 'side' electrons from filter two make it though the third filter, so 1/8th of the electrons pass though all three filters. Notice what has happened. Adding a 'side' filter oriented 90 degrees to the first filter between the 'up' filter and 'down' filter has, mirable dictu, changed the number of electrons passing from zero to 1/8th!
 .
. .
. 
Output: 1/2 x 0 =0
Output: 1/2 x 1/2 = 1/4
Output: 1/2 x 1/2 x 1/2 = 1/8
The standard quantum Copenhagen interpretation is that the second filter in 'observing' the electrons changes the experiment and the outcome. As the Univ of Toronto says
"If we slowly rotate the orientation of the second filter with respect to the first one from zero degrees to 180 degrees, the fraction of the electrons from the first filter that passes through the second filter goes continuously from 100% to 0%." (following the curve cos^2(theta/2), where theta is the relative angle between the first and second filters. So at 90 degrees, cos^2(90/2) = (0.707)^2= 1/2.)Photon angular momentum effects
Put random light into a polaroad filter and about half the light is transmitted and half absorbed. In classical electromagnetic terms the spacial orientation of the E field of the light transmitted by a polaroid filter is determined by the spacial orientation of the long crystals making up the polarizing film. But classical electromagnetics cannot explain how (right, below) inserting a 45 degree filter between quadrture filters increases transmission from zero to 1/8th. It's a quantum effect with the polaroid sheet somehow acting as a "measurement" for each photon and reorienting its axis.
 .
.  .
.  .
. 
source ---Univ of Toronto Physics Dept (David Harrison)
http://www.upscale.utoronto.ca/GeneralInterest/Harrison/SternGerlach/SternGerlach.htm
Interestingly
the transmission characteristics of photons though poloroid filters in
pretty much the same as electrons through electron filters, except all
the angles for photons are half those of electrons. Zero transmission with
electrons is filter 180 degrees apart (up and down), bu t with photons
its filters 90 degrees apart (in quadrature). Similarly the 1/8th transmission
though a triple cascade depends on the 2nd filter (relative to first) being
90 degrees with electrons and 45 degress with photons.
Photon spin
(I don't yet understand
this, but) Reference say that at the photon level polarization is closely
related to (or same as?) the angular momentum (spin) of photons. Photons
apparently have real angular momentum, because when absorbed then deliver
not only energy (& pressure) but also a quantum of angular momentum.
Furthmore because they are relativistic, traveling at c, the only orientatons
allowed for the angular momentum vector (spin vector) is along the direction
of travel, either forward or backward. (Does this mean that a photon being
absorbed by an electron gives it a clockwise or counterclockwise 'twist'?
Just asking.)
Is the electron a spinning ball of charge?Magnetic moments of nucleii
A non-main stream book was published in the 1990's arguing that the electron is in fact a spinning ball of charge, which would explain its intrinsic angular momentum and magnetic moment. But the conventional view is that this 'can't be right', because the calculated spin rate is too high. Wikipedia puts it, "(It would spin) impossibly fast. The speed of rotation would be in excess of the speed of light and is thus impossible." Another reference says an electron shows no structure down to 10^-18 m, so the calculated rotation rate is 10^32.
For some reason (which I have not seen explained) it appears that the nucleus intrinsic angular momentum plays little, or no, role in measurable atomic (DC) magnetic effects. The DC magnetic field effects of atoms appear to all come from the outside shell electrons, from their angular momentum (intrinsic and orbital). Nuclear magnetic moments can be forced with RF magnetic fields, and measure of these RF absorption frequencies, which are related to atomic resonances (precession frequencies), provides a valuable probe of atoms and molecules.Atoms with odd # of nucleons (like hydrogen) placed in a strong DC magnetic field with an AC component will absorbed RF energy at some frequencies that depend on the DC magnetic field. This is the basis for MRI (Magnetic Resonance Imaging) machine now widely used in medicine.
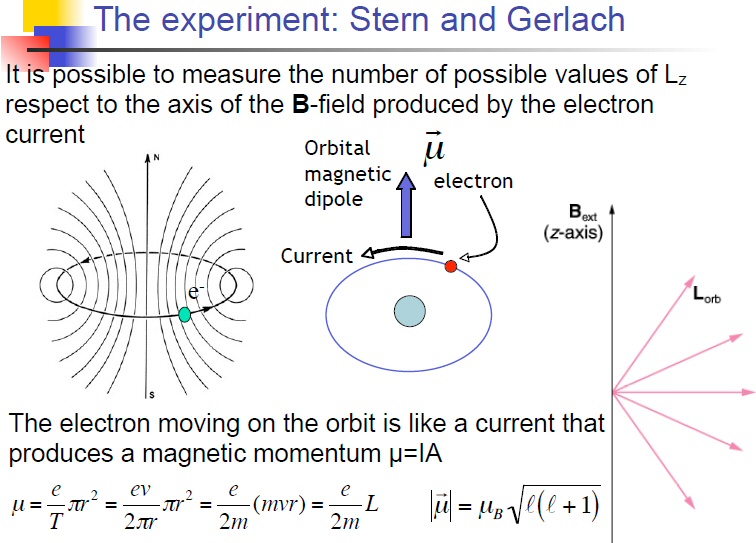
----------------------
A current
loop in an external magnetic field (B) feels a torque (torque = u cross
B), where u is a vector (pointing in the direction of the loop magnetic
field) called the magnetic moment (u) of the loop. (This torque
will cause current loops whose magnetic fields don't align with the external
B to precess).
----------------------------------------
text book
intrinsic dipole moment
ub approx one Bohr magnetron
orbital magnetic moment
intrinsic spin angular momentum s hbar/2
ratio of intrinsic magnetic moment to intrinsic spin is twice as large as the ratio of the orbital magnetic moment to orbital angular momentum
intrinsic dipole moments of paramagnetic materials (like hydgrogen) are due to the electronic orbits of the atoms.
magnetic dipole moment generated by the electron by virtue of its motion in orbit can be called the orbital dipole moment. Apart from this the electron has an intrinsic dipole moment of approx one Bohr magnetron.
*** looks like the intrinsic and orbital magnetic moments
(for hydrogen) are same! The ratio is different by factor of two because
the intrinsic angular momentum (hbar/2) is half the orbital angular momentum
(hbar)
Book derives
the magnetic dipole moment (u) for first orbit in hydrogen and they get
the same as I derive below:
u = hbar e/2m
Above is the "unit of magnetic dipole moment" in atomic physics and is called the Bohr magnetron (ub)
ub = 1 Bohr magnetron = hbar e/2m
= (1.054 x 10^-34 x 1.6 x 10^-19)/(2 x 9.11 x 10^-31)
= 9.25 x 10^-24 Am^2
check
(angular momentum of this first orbit = hbar)
Intrinsic spin angular momentum of the electron is
s = hbar/2
ratio of magnetic dipole moment of the orbit to its orbital angular momentum (for circular orbits) is
e/2m
check
u/angular momentum = [hbar e/2m]/hbar = e/2m OK
question --- Is measured dipole moment of (ferromagnetic) material due to a combination of intrinsic and orbital magnetic moments.
-- The origin of the magnetic moments responsible for magnetization can be either microscopic electric currents resulting from the motion of electrons in atoms, or the spin of the electrons or the nuclei
-- The spin of an electron, combined with its orbital angular momentum, results in a magnetic dipole moment and creates a magnetic field. (ferromagnetic materials))
** --- In many materials (specifically, those with a filled electron shell), however, the total dipole moment of all the electrons is zero (i.e., the spins are in up/down pairs)
** -- Only atoms with partially filled shells (i.e., unpaired spins) can experience a net magnetic moment in the absence of an external field (PM)
-- These permanent dipoles (often called simply "spins" even though they also generally include orbital angular momentum
-- (A related but much weaker effect is diamagnetism, due to the orbital motion induced by an external field, resulting in a dipole moment opposite to the applied field.)
-- Ferromagnetism involves an additional phenomenon, however: the dipoles tend to align spontaneously, without any applied field. This is a purely quantum-mechanical effect.
-- According
to classical electromagnetism, two nearby magnetic dipoles will tend to
align in opposite directions (which would create an antiferromagnetic material).
In a ferromagnet, however, they tend to align in the same direction because
of the Pauli principle
----------------------------------------
Zeeman effect
----------------------------------------
I read that
an electron has an intrinsic magnetic dipole moment of approximately
one Bohr magnetron. (My comment is that it is not at all intuitively obvious
why the intrinsic magnetic moment of an electron should be equal
to the current loop created by an electron orbiting a proton! One argument
says the magnetic moment of a loop is e/2m x L, where L is angular momentum,
so this formula was expected to apply to an electron too.)
The quantum formula has a multiplier of x2 (from Dirac) is scaled by electron spin (1/2) to yield 1 plus a small quantum correction term. The measured value is written (1/2) g ub, where g is called the gyromagnetic ratio and the electron spin g-factor has the value g = 2.00232. (A recent paper gives u = (g/2) x ub x S/hbar/2 with g/2 = approx 1 and I presume spin S must be hbar/2.) If the electron was a classical spinning sphere of charge then it would have angular momentum and a magnetic moment, but this classical picture cannot fit the size or quantized nature of the electron spin. (While not mainstream, there is a 1992 book that argues that an electron can indeed be modeled as a spinning sphere of charge.)
Bohr magnetron
My guess is
that the Bohr magnetron is just the magnetic moment (i A) calculated using
the parameters of the Bohr atom. (Yup) (Bohr radius is usually designated
by a0 and the velocity is alpha x c.)
i = charge/orbit time
= e/(2 pi r/velocity)
= e/(2 pi a0/alpha c)
= (e alpha c)/(2 pi a0)
area = (pi/4) x 4 a0^2 = pi a0^2, so
ub = iA = pi a0^2 x (e alpha c)/(2 pi a0)
= (1/2) a0 e alpha c
but a0 = (hbar/mc)/alpha = hbar/(mc alpha)
ub = (1/2) [hbar/(mc alpha)] e alpha c
= hbar e/2m
(Yup, agrees with references)
Angular momentum (L) is mvr, so for an electron in a Bohr
atom
L = mvr
= m (alpha c) (hbar/m c alpha)
= hbar
so a general way to write ub is
ub = (e/2m) hbar
= (e/2m) L
where L is angular momentum
values
ub = 1.055 x 10^-34 x 1.60 x 10^-19/2 x 9.11 x 10^-31
= 9.26 x10^-24 amp m^2 (or joule/tesla)
checks
Intrinsic magnetic moment
From references
the intrinsic magnetic moment 'u' of an elementary particle with charge
e, mass m, and spin s, is
u = g(e/2m)S
where
g is dimensionless 'g-factor'
S = angular momentum
Wikipedia comments about the intrinsic magnetic moment (u):
"One of the triumphs of the theory of quantum electrodynamics is its accurate prediction of the electron g-factor, which has been experimentally determined to have the value -2.0023193043622 +/- 15 (14 decimal places!). The value of 2 arises from the Dirac equation, a fundamental equation connecting the electron's spin with its electromagnetic properties, and the correction of 0.002319304… arises from the electron's interaction with the surrounding electromagnetic field, including its own field. (Wikipedia 'Spin')Quantum correction
g/2 = 1.001159652
in units of Bohr magnetrons
(or g = 2.0023192)
alpha = 1/137.035999
http://hussle.harvard.edu/~gabrielse/gabrielse/papers/2008/HarvardMagneticMoment2008.pdf
Cleartly g above is the same as g-factor (further above).
PM magnets
The magnetic
field of permanent magnets (ferromagnetism) arises from the magnetic action
of electrons in the atoms of the material. There are two magnetic electron
effects: the intrinsic electron magnetic moment (sort of like that of a
spinning charge ball) and the magnetic moment created by the electrons
orbiting the nucleus (sort of like a current loop). Surprisingly the reference
I have found don't ratio them, or even say which is dominant!
In materials with filled shelled all the electrons are paired up/down, so the intrinsic magnetic moments cancel. These materials have weak magnetism and ferromagnetic materials like iron (#26), cobalt (#27) and nickel (#28) all have a lot of unpaired electrons, both of which argue for the intrinsic magnetic moment of the electron is probably the dominant magnetic effect.
Noble gases
element #2 helium
2
1s2
element #10 neon
2, 8
[He] 2s2 2p6
element # 18 argon
2, 8 ,8
[Ne] 3s2 3p6
element #36 krypton
2, 8, 18, 8
[Ar] 3d10 4s2 4p6
element #54 xenon
2, 8, 18, 18, 8
[Kr] 4d10 5s2 5p6
element #86 radon
2, 8, 18, 32, 18, 8 [Xe] 4f14 5d10 6s2 6p6
 .
.  .
. 
#10 neon
# 18 argon
#36 krypton
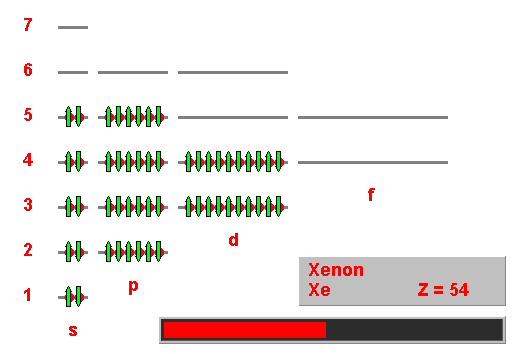 .
. 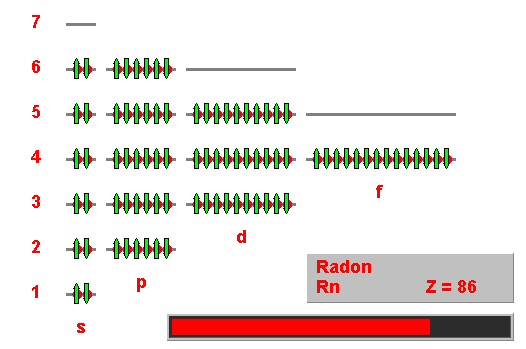
#54 xenon
# 86 radon
Ferromagnetic
There are
only three ferromagnetic elements at room temperature (iron, nickel, and
cobalt). Interestingly they are consecutive element in the periodic chart
with 6 (iron), 7 (cobalt), and 8 (nickel) electrons in the 3d orbitals,
which can hold a maxium of 10 electrons.
element #26 iron
2, 8, 14, 2
[Ar] 3d6 4s2
1,043 K
element #27 cobalt
2, 8, 15, 2
[Ar] 3d7 4s2
1,388 K
element #28 nickel
2, 8, 16, 2
[Ar] 3d8 4s2
627 K
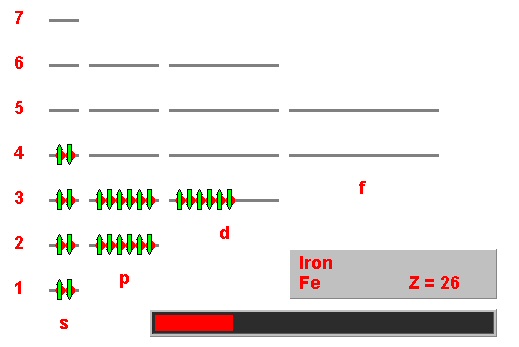
# 26 iron ferromagnetic
element #64 gadolinium
2, 8, 18, 25, 9, 2 [Xe]
4f7 5d1 6s2 292 K
element #66 dyprosium
2, 8, 18, 28, 8, 2 [Xe] 4f10
6s2
<77K
------------------------------------------
Electron Spin
From Wikepedia
'Spin'
Elementary particles, such as the photon, the electron, and the various quarks are particles that cannot be divided into smaller units (meaning they are not made up of rotating subcomponents). The spin that they carry is a truly intrinsic physical property, akin to a particle's electric charge and mass. In quantum mechanics, the angular momentum of any system is quantized: its magnitude can only take valuesBlackbody radiationS = hbar sqrt{s(s + 1)}
where
S = (total) angular momentum
s = spin quantum number
s = 1/2 for electron (1 for photon)
= hbar sqrt{(1/2)(1 + 1/2)}
= hbar sqrt{3/4}
= hbar sqrt{3}/2
Blackbody curve = (2 pi h c^2/wavelength^5)/{e^(hc/wavelength k T) -1}
As a function of freq and temperature
= (2 pi h freq^5/c^3/{e^(h freq/k T) -1}
where
h = planck's constant (6.63 x 10^-34 J-sec)
c = speed of light (3 x 10^8 m/sec)
k = Boltsman's constant (1.38 x 10^-23 J/K)
T = absolute temperature in kelvin (K) (centigrade = T + 273)
From the frequency formula you can see that at low frequencies (where h freq < k T) , (h freq/k T) can be nglected compared to the denominator changes very slowly with frequency so radiation rises as frequency to the fifth power. Near where (h freq > 5 x k T) the exponential growth of the denominator begins to dominate over the numerator, so the output begins to fall. So the peak radiation frequency is approx
h freq = 5 k T
freq = 5 k T/h
Note the (peak) frequency looks like it increases linearly with temperature. This turns out to be exactly true, known as Wein's Displacement Law. At room temperature (300K) we calculate
freq = 5 x 1.38 x 10^-23 J/K x 3 x 10^2/6.63 x 10^-34 J-sec
= 3.1 x 10^13 hz
Here's a plot of blackbody radiation vs frequency for various temperatures. It looks like the 300K curve peaks near 5 to 7 x 10^13 hz, so our simple approx is within about a factor of two of the peak.

Area under the blackbody curve
Area under the black body curve is total power radiated (vs temp). Doubling
temperature increased the peak radiated power by 8 (approx?) and doubles
the width (approx?), so the total radiated power over the whole spectrum
goes up by 16. Radiated power goes as the 4th power of (absolute) temperature
(Stefan's law of radiation)
Radiated power (total) = sigma x T^4
where
sigma = 5.67 x 10^-8 watt/m2/K4
Where does the exponential relationship come
from?
In 1880 chemists
found that the rate of chemical reactions was accurately described by the
formula (Arrenius formula)
rate constant
(of chemical reactions) = C e^Ea/RT
where
Ea = activation energy
R = gas constant
T = absolute temperature
"Arrhenius argued that in order for reactants to be transformed into products, they first needed to acquire a minimum amount of energy, called the activation energy Ea. At an absolute temperature T, the fraction of molecules that have a kinetic energy greater than Ea can be calculated from the Maxwell-Boltzmann distribution of statistical mechanics, and turns out to be proportional to e^-Ea/RT. The concept of activation energy explains the exponential nature of the relationship, and in one way or another, it is present in all kinetic theories." (Wikipedia, Arrhenius equation)When E is in joules per molecule instead of joules per mol, the Boltzmann constant (k) is used instead of the gas constant (R).
rate constant (of chemical reactions) = C e^E/kT
--------------------------------
A convenient
form for the DeBroglie wavelength expression.
wavelength = h/p = hc/pc = 1,240 ev nm/pc = 1,240 ev nm/energy (in ev)
where
hc = 6.63 x 10^-34 joule-sec x 3 x 10^8 m/sec x {1 ev/1.60 x 10^-19 coulomb}
= 12.43 x 10^-7 ev m
= 1,240 ev nm (nanometers)
pc = energy in ev {pc = momentum (mv) x c}
visible light photons have energy in the (1.6 to 3 ev) range, so the wavelength of light is in the range of 400 to 800 nm.
electron energies levels in hydrogen
E = -13.6 ev/ n^2
where
n = 1,2,3 etc (1 is the lowest orbit)
wavelength = 1,240 ev nm/pc = 1,240 ev nm x {n^2/13.6 ev}
= 91 nm x n^2
where n = 1,2,3,4, etc
radius of inner (n=1) hydrogen orbit = .0529 nm
(n=2) radius = .212 nm x4 inner
(n=3) radius = 0.477 nm x9 inner
since mass x velocity x wavelength = const (h), then
in outer orbits the electrons move slower, n=2 at 1/2 speed of inner electron
and n=3 at 1/3 speed of inner electron. (assumes n wavelengths fit in each
orbit)
so circumference is 2 pi x .0529 = 0.33 nm (supposed de broglie wavelength)
equivalent energy (n=1, hydrogen) = 1,240 ev nm/0.33 nm =3,758 ev
check
v = 2.2 x 10^6 m/sec (typical velocity of an electron in an
atom equal
to alpha x c, little less than 1% speed of light)
'orbit' time = 2 pi r/speed
(There is no real orbit time)
= 2 x 3.14 x 0.0529 x 10^-9 m/2.2 x 10^6 m/sec
= 1.51 x 10^-16 sec
equivalent current = q/orbit time
= 1.6 x 10^-19 coulomb/1.51 x 10^-16 sec
= 1.05 x 10^-3 amp
= 1.05 ma (for one electron in lowest hydrogen orbit)
m = 9.1 x 10^-31 kg
h = 6.6 x 10^-34 joule-sec
1 eV = 1.6 x 10^19 joule
wavelength = h/p = h/(m x v)
= 6.6 x 10^-34/(9.1 x 10^-31 x 2.2 x 10^6)
= 0.33 x 10^-9 m (0.33 nm)
So if the electron energy is all kinetic energy
(nope, but it does give the right answer because potential energy is twice
kinetic energy) and we just put the energy at about 1% the speed
of light, then the de broglie wavelength comes out to be the circumference
of the lowest orbit in a hydrogen atom. So we can draw 1 wavelength
around the orbit.
----------------------------------------------------
Deriving De Broglie wavelength of an electron from
its energy
(note this is from an oddball
paper)
"Another way
to think of this quantity is to consider the quantum uncertainty of the
electromagnetic radius of an electron from its mass energy and the speed
of light. "
E x time = h
m x c^2 x time =h
(m x c) x (c x time) = h
let's assign c x time = 'some' radius
m x c x radius =h
compton wavelength = radius = h/(m x c)
(same as above with v =c)
= 6.6 x 10^-34/(9.1 x 10^-31 x 3 x 10^8)
= 2.4 x 10^-12 m
Reflection of light
Feynman
points out that reflection of light from matter is just an electron in
an atom of a material absorbing an incoming photon and emitting another
photon (that's all he says). Our thinking about this needs to
take into account two facts:
1) Outgoing photon has the same color/frequency as the incoming photon
2) There is apparently no delay, or specific phase shifts like 90 degrees
or 180 degrees of the photon frequency. Classically a mirror is modeled
with zero delay. The exact QED just assumes a specific phase change, which
I think includes zero.
Seems to me that a reasonable model for how this happens must similar to the electron shaking/oscillation model of Thomson scattering (below). The phase of the of the emission then could easily have some phase shift relative to the exciting photon. Of course, for reflection the electrons absorbing and emitting are electrons bound to atoms. (check on this)
My working electron/photon absorption model --Circa 1924 de Broglie speculated in his thesis that for frequencies with wavelengths below atomic dimensions (10^-10 m, which is in the X-ray region of the spectrum) all the electrons in an atoms are vibrated by an incoming photon and the emitted photon is in phase with the incoming photon. For higher frequencies only individual electrons in an atom are shaken and he talks about a dipole model applying.
Photons have an embedded (E field) sinewave that deposits its energy into the electron by shaking the electron at the photon frequency. This oscillation of the electron then causes the emission of a new photon (in a different direction?) that then damps the electron ringing by taking away the energy."The effect of anomalous scattering can most simply be described by treating an electron as a classical dipole oscillator driven by a force effected by the incident X-ray and with damping corresponding to the bound state in which the electron exists." (misc ref) Also they derive the outgoing photon E field as the the incoming ? E field passed through a 2nd order high pass filter.
Electrons probably have other ways of absorbing energy. It could be that low frequency photons just add kinetic energy (to free electrons only?). My guess is this the energy limit for this might be somewhere around the rest mass energy of the electron (0.511 Mev) where the recoil speed would to 70.7% of c. Electrons in atoms can absorb energy be jumping orbits, but these energies are very low (few ev).
Data on electron-photon interactions
There are
two simple experiments that show that the energy exchanged when a electron
either absorbs or emits a photon is a simple, linear function of the frequency
of the light, and the proportionality constant between energy and frequency
is both cases is found to be planck constant ( h = 6.63 x 10^-34
joule-sec).
Taken together they constitute strong proof that photon energy [E = h x
freq]. When I first came across this formula in college physics, I was
amazed. It was both so strange and so simple.
The first experiment is the photoelectric experiment where electrons in metals absorb incoming photons of (visible to ultraviolet) light. The frequency of the light is varied and the recoil kinetic energy of the electrons is measured and found to depend linearly on the frequency of the light.
The second is sometimes called the 'reverse photoelectric effect'. In this experiment electrons are accelerated, and then rapidly slowed down by entering metals. As the electrons decelerate, they emit x-ray photons in a continuous spectrum with a sharp high cut off frequency. The voltage used to accelerate the electrons is varied, and the frequency of the emitted photons at the top of the spectrum (at cut off) is measured and is found to depend linearly on the kinetic energy of the electrons, which is just the [e x (accelerating voltage)].
Photoelectric experiment
The classic
photoelectric experiment is shown in the figure below. This experiment
was first done by Millikan and published in 1916. The maximum (kinetic)
energy of electrons emitted from a metal plate illuminated with light is
found to depend only on the frequency of the light. The electron
energy varies linearly with the light's frequency with a constant
of proportionality equal to planck's constant.
An evaluated glass tube has two metal electrodes, one of which is illuminated with visible to ultraviolet light. It generates an open circuit voltage (Vstop) of about 0.5V to 3V depending on the metal. A current flows when the terminals are shorted, the brighter the light the higher the current (Ia and Ib). Basically the tube is a (weak) photocell.
The open circuit voltage (Vstop) is found to be a linear function (with an offset) of the frequency (color) of the light and to be independent of the light's intensity. The slope of the (open circuit) voltage vs freq comes out to be planck's constant. If the light flashes, the current follow the light with a delay of no more than (?) 1 nsec).
This experiment is interpreted as follows: an electron loosely bound to a metal atom absorbs a single photon allowing it to escape its atom into the vacuum of the tube, i.e. climb out of the atomic energy well, with the excess energy carried by the electron as kinetic energy. The applied voltage required to kill the current (Vstop) is a direct measure (in ev) of the kinetic energy carried by the ejected electrons. The offset in the curve is the 'work function' of the metal, basically the ionization energy needed to escape from the nucleus, which is why it varies depending on the type of atom. (Note the energy comes out to be a few ev, which is the range of energies carried by visible and ultraviolet light.)
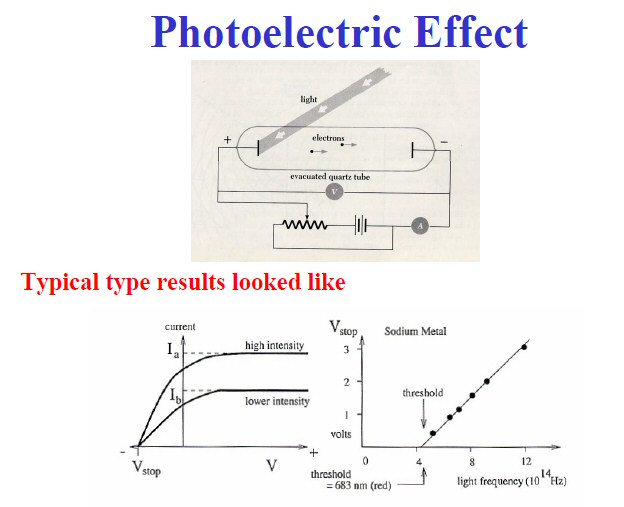
(physics lecture notes, Univ in UK)
I eyeball the curve (above) to show an increase in stopping voltage from one volt to two volts when the frequency increases from (about) 6.4 x 10^14 to 8.8 x 10^14 hz.
h = E/freq
= (delta) 1ev/(delta) 2.4 x 10^14 hz x [1.6 x 10^-19
joule/1ev]
= 6.66 x 10^-34 joule-sec
Planck's constant
In the real world this experiment is quite difficult to do. Surfaces get contaminated, and the current for a variety of reasons does not cleanly 'cut off' at a given voltage, rather the current has a tendency to approach zero asymptotically with a long tail. Thus a certain amount of idealizing, or fiddling with the data, is required to determine the cut off voltage, which is the important data. These problems are discussed in the paper below by a guy from York Univ (in UK) who built his own photoelectric tubes for demos in class. When he took a careful look at Millikan's data, he found that Millikan too had had a lot of problems with tails, and convinced himself it was legitimate to simply cut them off, and this is how Millikan published his data.
Reverse photoelectric effect
The data below
is from a detailed write up on how a SEM (scanning electron microscope)
works. It shows how continuum bremsstrahlung (German for 'braking radiation')
x-ray radiation from an SEM sample. Ib is beam current, Z is atomic # of
target, and E0 is accelerating voltage of electrons. The figure left shows
that as the beam current increases the intensity of x-rays increases, but
the frequency spectrum stays the same.
The figure right shows the reverse photoelectric effect. As the acceleration voltage increases from 10 KV to 50KV, increases the electron energy from 10 kev to 50 kev, the (sharp) upper frequency edge of the x-ray spectrum increases substantially. This is shown in figure by the left edge of the spectrum shifting to shorter and shorter wavelengths as the voltage rises. (Unfortunately the hor axis is not labeled in this figure, but French has similar data that shows the upper end of the bremsstrahlung x-ray spectrum, sometimes called the 'cut off' frequency, shifts in a manner consistent with photon energy = h x freq.)
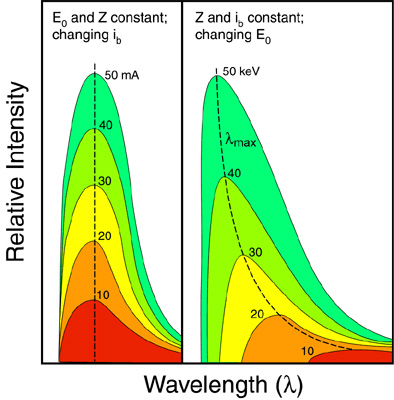
Northern Arizona Univ (geology dept) SEM --- http://www4.nau.edu/microanalysis/Microprobe-SEM/Signals.html
Electron recoil
An electron
absorbing a high frequency/energy photon must (I assume) be knocked for
a loop as it absorbs the photon's energy. Above I calculated that a photon
with Compton wavelength has the same energy as the rest mass energy of
the electron (0.511 Mev). I would expect an electron absorbing a photon
of this energy would be accelerated to about c/sqrt{2} (see my hand sketch
of energy vs velocity elsewhere in this essay). Gamma ray energies (from
outer space) go far higher than 0.511 Mev, to Gev and even Tev levels.
I found a brief forum note indicating that photon energy does go into kinetic energy of the electron, but when energies are high such that the electron speed would approach c (how close?) if fully absorbed apparently other things can happen. Scattering seems to indicate that the photon is absorbed and (immediately?) emitted again or it (in some sense) bounces off the electron??
free electron
Compton scattering -- elastic scattering of X-rays from free
electrons
free electron
Thompson scattering
bound electron
Rayleigh scattering
bound electron
knocked free photoelectric effect
Thomson scattering
Thomson scattering
is the scattering of electromagnetic radiation by free electrons.
This is a strong effect with a probability that is independent of light's
frequency. Plasmas are opaque because thompson scattering of free electrons
in the plasma readily via absorb (and remit) photons. In fact thompson
radiation from plasma is used measure plasma temperature and other plasma
parameters.
The hydorgen in the sun is mostly ionized, so it is thompson scattering of the sun's free electrons that causes a photon to take thousands of years to travel from the core to the surface. It is also thompson scattering that makes the early universe opaque, ending when molecular hydrogen forms causing the universe to become transparent and releasing what we now see as the cosmic background radiation.
It is the E field of the incident wave that causes the free electron to oscillate (at the E field freq). A simple model is just the Lorentz force from the E field {F = qE = ma} acting on the mass of the electron. As the electron accelerates, it in turn emits radiation, and in this way the incident wave is scattered. The frequency of the outgoing radiation is the same as the incoming radiation (only the directions are changed). The moving electron radiates most strongly in a direction perpendicular to its motion, meaning emitted radiation will be polarized along the direction of the electron motion.
Thompson scattering reference:
http://farside.ph.utexas.edu/teaching/em/lectures/node96.html#e9.46
Thomson scatter calculations
Thomson scattering
is a classical field analysis of a plane wave impinging on (many)
free electron. The equation of motion is solved for the E field of the
incident plane wave acting on the mass (& charge) of the electron.
This causes the electron to oscillate at the frequency of the incoming
wave making it (in effect) a little piece of current, technically an electric
dipole. The radiated field from the electron (modeling it as an electric
dipole) is then calculated and found to be fairly weak, proportional to
the classical electron radius squared.
The usual thomson scattering eferences never work any numbers for individual electrons, motion is not calculated, nor is electron energy. There is no requirement that an individual electron have more energy than a light photon. In fact there are no quantum considerations at all. This is totally a classical analysis. It's a low power approximation and applies when electron speeds are not relativistic. It must be reasonably accurate for some plasmas because thomson scattered light is used as probe to measure temperature and other properties of the plasma.
The above reference derives an electron cross sectional area for thompson scattering, meaning an area target to the incident wave or the 'spatial extent of the electron as far as its interaction with electromagnetic radiation is concerned'. Interestingly it turns out to be on the order of the (cross sectional) area of the classical electron [(x 8 pi/3) x (classical electron radius = 2.82 x 10^-15 m)^2], and this cross sectional area is independent of frequency of the (incident) radiation. The radiation pattern of the electron is that of a dipole causing it to have a sin^2 (angle) shape.
Photons are scattered by free protons too, but because the proton mass is so much higher than an electron, they are not accelerated very much. The formula for thompson scattering cross sectional area goes as 1/m^2, so a free proton is more than a million times less likely to scatter a photon than a free electron. Hence in a neutron plasma virtually all thompson photon scattering is done by the (free) electrons.
How far can a photon travel in the sun
The density of the sun is about the same as water (1,000 kg/m^3). The (av) density of electrons is, of course, the same as protons, so the (rough) electron density is [(10^3 kg/m^3/10^-27 kg (mass of proton) = 10^30/m^3]. The thompson electron cross sectional area is (rough) [(8 pi/3) x (2.82 x 10^-15 m)^2 = 10^-28 m^2]. Think of electrons as forming a cubic matrix with 10^10 electrons per dimension. The photon is modeled as just a point. So how far can a photon travel into the electron matrix before interacting with an electron, meaning the point hits a cross sectional area of an electron?
Well, the odds of the photon hitting an electron in the first 'row' of electrons is the matrix is the electron thompson cross sectional area divided by the cross sectional area in the matrix face occupied by a single electron.10^8 'rows' x 10^-10 m spacing = 10^-2 m (1 cm)10^-28 m^2/(10^-10 m)^2 = 10^-8 (first row odds)
Hence the photon can travel through [(1/10^-8) = 10^8] 'row's of electrons before hitting one. That makes the photon (av) travel distance in the sun's interior:
Thomson scattering --- working some numbers
I find Thomson
scattering of light is described in classical terms. The E field of incident
light does work on a (free electron) causing it to oscillate non-relativistically
at the frequency of the incoming light. The oscillating electron then radiates
away (some or all) of this energy. But I rarely see any numbers.
Let's calculate for visible light. Typical visible light is 6 x 10^14 hz with a photon energy (h x freq) of 2.5 ev or 4 x 10^-19 joule. So what do we know? It's probably a single electron (it is a free electron) and it's vibrating at the light frequency. E from E field force (F = qE) x distance is stored as kinetic energy. The speed is non-relativistic (assume < 10%c). The kinetic energy must be (at least) 2.5 ev of kinetic energy if it is to radiate at the same frequency.
So how much is the electron moving? The slope of position vs time of a sinewave can be approximated as 2delta x (displacement) divided by 1/3rd period.
Let's start by assuming speed is 10%c
delta t = (1/3) x 1/(6 x 10^14 hz)
= 5.5 x 10^-16 sec
speed = 2delta x/delta t
delta x = (1/2) speed x delta t
= (1/2) 3 x 10^7 m/sec x 5.5 x 10^-16 sec
= 8.5 x 10^-9 m
Let's check the kinetic energy
E = (1/2) m v^2
= (1/2) 9.1 x 10^-31 kg x (3 x 10^7 m/sec)^2
= 4.1 x 10^-16 joule x (1 ev/1.6 x 10^-19 joule]
= 2,600 ev
Looks much too high, its over 1,000 time the energy in
one photon of visible light. Lets reduce the speed so the kinetic energy
comes out to be the energy in one incoming photon (2.5 ev). Just
a guess, I have no idea if this is reasonable for Thomson scattering.
speed = sqrt{2.5 ev/2,600 ev} x 10% c
= (1/32) x 3 x 10^7 m/sec
= 9.3 x 10^5 m/sec
(0.31%c)
Displacement is reduced by the same amount as speed
delta x = (1/32) x 8.5 x 10^-9 m
= 2.6 x 10^-10 m
We can calculate the E field, because energy = force x displacement
energy = force x distance
= eE x delta x
E = energy/(e x delta x)
= 4 x 10^-19 joule/(1.6 x 10^-19 x 2.6 x 10^-10 m)
= 10^10 volt/m
Awfully big number!
check -- What is the E field energy in a cylinder with the radius of displacement and a length of delta t (1/3rd of the light period). Energy density is (1/2 e0 E^2).
E field energy = (pi/4) 4 (delta x)^2 x (delta t)c x (1/2 e0 E^2)
= 0.79 x 4 (2.6 x 10^-10)^2 x 5.5 x 10^-16 sec x 3 x 10^8 m/sec
x (1/2) 8.8 x 10^-12 x (10^10)^2
= 1.5 x 10^3 x 10^-20 x 10^-8 x 10^-12 x 10^20
= 1.5 x 10^-17 [ 1 ev/1.6 x 10^-19]
= 94 ev
(38 x 2.5 ev)
not too bad, within two orders of magnitude
What does really mean?
-- It is convenient
to define the scattering cross section as the equivalent area of the incident
wavefront which delivers the same power as that re-radiated by the particle.
-- The classical scattering cross section (5.73) is modified by quantum effects when the energy of the incident photons, h x freq, becomes comparable with the rest mass of the scattering particle, m c^2 .
-- Thomson scattering cross section is (8/3) (classical electron radius)^2 = 6.6 x 10^-20 m^2
-- Since this radius is extremely small, it is clear that scattering of radiation by a single electron (or any other charged particle) is a very weak process.
Sunlight intensity & photons
As a check
on E fields let's look at sunlight. The power of bright sunlight at the
earth's surface is about 1,000 watt/m^2. From an electromagnetic viewpoint
the energy in light is carried in the E and H fields, so we can use the
value of 1,000 watt/m^2 to figure the average E field density of sunlight.
Let's look at the solar energy in one cubic meter, say 1 meter above a 1 m^2 solar cell. Light travels the last 1 meter to the solar cell in about 3.3 nsec since light travels about 1 ft/nsec. 1,000 watts is 1,000 joules/sec so the energy in the one cubic meter is
energy/m^3 = 1,000 joules/sec/m^2 x 3.3 x 10^-9 sec/m
= 3.3 x 10^-6 joule/m^3
Half the energy is in the E field and half in the H field, so the average E field density is
(1/2) e0 E^2 = (1/2) 3.3 x 10^-6 joule/m^3
E = sqrt{3.3 x 10^-6/8.8 x 10^-12}
= sqrt{0.375 x 10^6}
= 600 volt/m
Accurate average (spacial & time) value
Let's find the approx number of visible photons in a cubic meter of sunlight. For above a photon near the middle of the visible spectrum has 2.5 ev or 4 x 10^-19 joule per photon.
# of photons/m^3 = energy per m^3/energy per photon
= 3.3 x 10^-6 joule/m^3/(4 x 10^-19 joule)
= 8 x 10^12 photon/m^3
If photons are real discrete things (physicists think so, but I think all anyone really knows is that energy transfers are quantized), then the photon number above means that photons in sunlight are spaced about 1/20th of a mm apart (0.5 x 10^-4 m).
Check on photon energy. E and H field energy in volume (0.5 x 10^-4 m) at an average 600 v/m
photon energy = 2 (1/2) e0 E^2 x volume
= (8.8 x 10^-12) x (6 x 10^2)^2 x (0.5 x 10^-4 m)^3
= 4 x 10^-19 joule
(2.5 ev photon energy)
Nobody knows how big a photon is. It's not really clear if a photon can be said to have an E (and H) field. Our calculations that solar photons are spaced (on average) 50 microns apart (0.5 x 10^-4 m) and with (artificial) assumption that E is uniform, then E = 600 v/m to give the photon the correct energy. If photons are assumed to be smaller (with E=0 gaps between), then a decrease in photon spacing by n must mean that the photon E field increases as n ^(3/2) to keep the photon energy the same.
So if the E field of our 2.5 ev visible photon is assumed to be occupy a cube with a dimension of the wavelength (600 nm), then E field must increase to
E field = (50 x 10^-6m/6 x 10^-7 m)^(3/2) x 600 v/m
= 761 x 600 v/m
= 4.5 x 10^5 v/m
(wavelength size photon)
For work done on an electron the dimension was 2 x (2.6 x 10^-10m) (from above)
E field = (50 x 10^-6m/5.2 x 10^-10 m)^(3/2) x 600 v/m
= (9.6 x 10^4)^(3/2) x 600 v/m
= 30 x 10^6 x 6 x 10^2 v/m
=1.80 x 10^10 volt/m
(vibrating electron with 2.5 ev kinetic)
Good, very close (within factor of 2) of the E field calculated
above (10^10 volt/m) for the work done on a vibrating electron by E field
to impart 2.5 ev of kinetic energy. Here is a summary of visible photon
E field (if it has one!) vs various possible photon sizes.
|
(assumed, cubic) |
|
|
|
|
|
|
|
|
|
|
|
|
|
(thomson scattering) |
Rayleigh scattering
Rayleigh scattering
is the scattering of light by neutral atoms, i.e. basically by electrons
in orbit around the nucleus of atoms. Is generally considered a much weaker
effect than thompson scattering (scattering by free electrons). Unlike
free electrons electrons in orbit can only (?) oscillate to the extent
that they can jump orbits. This leads to a (crude) model of the outer electrons
in orbit as simple harmonic oscillators (as the physicist like to
call them!). When the equations are written out (see reference below),
the ability of the electrons to oscillate (and thus absorb and emit photons)
looks like a 4th order underdamped high pass filter. The response rises
as freq^4, peaking at resonance, and then flattening with a gain of one
above resonance, meaning above resonance electrons in orbit scatter light
pretty much like free electrons.
The most common example of rayleigh scattering is the scattering of visible radiation from the sun by nitrogen and oxygen in the atmosphere. The scattering of visible light by nitrogen and oxygen is quite weak because the (lowest) resonance (emission) frequencies of these atoms are above the visible in the ultra-violet. It is rayleigh scattering that causes the daytime sky to look blue (not black), the sun overhead to look yellow (not white?), and the sun at sunset to look dimmer and redder. Water and dust in the atmosphere also contribute to rayleigh scattering.
Rayleigh scattering reference:
http://farside.ph.utexas.edu/teaching/em/lectures/node97.html
Compton scattering
A few years
after Millikan's 1916 photoelectric experiments Compton did x-ray experiments
that strongly indicated ("dramatic confirmation" in the words of my atomic
quantum textbook) that quanta of light act like particles. He fired monochromatic
x-rays at materials and measured the frequency of the scattered radiation.
He found that some of scattered (reflected) x-rays were at a slightly lower
frequency that his source. This was unexplainable classically, because
classically electrons are set into oscillation by the E-field of the incoming
radiation and so would radiated at exactly the same frequency. For this
work he won the 1927 nobel prize for physics.
Compton was able to calculate not only the frequency difference (between his source and reflected x-rays), but also how it varied with angle. His analysis assumed the photon and electron (in effect) bounced off each other like two (relativistic) particles, like two billiard balls. Colliding billiard balls is a classic mechanics experiment taught to all freshman physics. You are shown if a collision is elastic, meaning no energy is lost when particles collide, you can calculate the velocity and angle of the particles after the collision from conditions before using just the principles of the conservation of energy and conservation of momentum. Compton was able to do his analysis pretty much the same, except energy and momentum are equated relativistically, since photons traveling at c are relativistic particles and the electron recoil (with x-rays) can approach c.
Compton's analysis
The initial
conditions couldn't be more simple. An incoming photon hits a stationary
free
electron (in the material). The use of a free electron rather than an electron
bound in an atom of the material was first suggested by the fact that the
results of the experiment were the same for different materials.
The incoming photon comes in from the left along the x-axis carrying energy and momentum (E0, p0). The electron is sitting stationary at the origin with zero momentum, zero kinetic energy, and (m0c^2) rest mass energy. After the collision, it's assumed the electron and (scattered) photon fly out rightward, the photon with energy, momentum (E1, p1) upward at angle (theta), and the electron downward with kinetic energy, momentum (KE, p) at angle (phi). [This analysis is pretty much cribbed directly from Eisberg, p36]
conservation of momentum
The momentum
vectors are resolved into x and y components. The incoming photon along
the x-axis has no y momentum, so the outgoing photon and electron y components
have
to cancel, and of course, the x components have to add to the incoming
photon momentum.
y axis
y photon momentum = y electron momentum
p1 sin(theta) = p sin(phi)
squared
[p1 sin(theta)]^2 = [p sin(phi)]^2
x axis
incoming photon momentum = x photon momentum + y electron momentum
p0 = p1 cos(theta) + p cos(phi)
squared
[p0 - p1 cos(theta)]^2 = [p cos(phi)]^2
add the squares using identity [ sin^2 + cos^2 = 1]
[p1 sin(theta)]^2+ [p0 - p1 cos(theta)]^2 = p^2
[p1 sin(theta)]^2+ [p0^2 - 2 p1 p0 cos(theta) + (p1 cos(theta))^2] = p^2
p1^2 + p0^2 - 2 p1 p0 cos(theta) = p^2
relativistic conservation of energy
in photon
E + electron rest mass E = out photon E + electron kinetic energy +
electron rest mass E
in photon E = out photon E + electron kinetic energy
E0 = E1 + KE
E0 - E1 = KE
for photons p = E/c or pc = E
c(p0 - p1) = KE
in general the relativistic equation for total energy of any particle is (see my hand drawn sketch below)
E = sqrt{(cp)^2 + (m0c^2)^2}
squared
E^2 = [(cp)^2 + (m0c^2)^2]
but total energy for outgoing electron is also E = [KE + m0c^2], so
[KE + m0c^2]^2 = [(cp)^2 + (m0c^2)^2]
KE^2 + 2 KE m0c^2 + (m0c^2)^2 = (cp)^2 + (m0c^2)^2
KE^2 + 2 KE m0c^2 = (cp)^2
(KE/c)^2 + 2 KE m0 = p^2
substituting KE from above and p^2 from above
(KE/c)^2 + 2 KE m0 = p^2
(p0 - p1)^2 + 2m0c(p0 - p1) = p^2
(p0 - p1)^2 + 2m0c(p0 - p1) = p1^2 + p0^2 - 2 p1 p0 cos(theta)
p0^2 - 2 p0p1 + p1^2 + 2m0c(p0 - p1) = p1^2
+ p0^2 - 2 p1 p0 cos(theta)
- 2 p0p1 + 2m0c(p0 - p1) = - 2 p1 p0 cos(theta)
2m0c(p0 - p1) = 2 p0p1 - 2 p1 p0 cos(theta)
2m0c(p0 - p1) = 2 p0p1[1- cos(theta)]
m0c[(p0 - p1)/p0p1] = [1- cos(theta)]
m0c(1/p1 - 1/p0) = [1- cos(theta)]
for both particles and photons (wavelength = h/p), so
substituting
(m0c/h)(wavelength1 -
wavelength0) = [1- cos(theta)]
Compton wavelength shift
(wavelength1 - wavelength0) = (h/m0c)[1- cos(theta)]
where
(h/m0c) = compton wavelength
wavelength1 = wavelength of outgoing (scattered) photon
wavelength 0 = wavelength of incoming (source) photon
theta = angle of scattered photon from axis of incoming photon
This simple elastic collision model predicts a difference in wavelengths between scattered radiation and source radiation that is independent of the source frequency, varies with angle as [1 - cos(theta)], and has a value at (theta = 90 degree) of (h/m0c), known ever since as the compton wavelength. And for a given angle the wavelength difference is surprisingly a constant.
The compton wavelength (h/m0c) incorporates quantum theory (h), relativity (c) and the rest mass of the electron (m0). The value of compton wavelength is
h/m0c = 6.626 x 10^-34/(9.109 x 10^-31x 3 x 10^8)
= 2.425 x 10^-12 m
yup, Compton wavelength
What energy photon has a compton wavelength and what's
its momentum?
E = h x freq
= h x (c/wavelength)
= hc/(h/m0c)
= m0c^2
electron rest mass energy 0.511 mev
p = E/c
= m0c^2/c
= m0c
classically this is electron mass x speed of light
but in real world is 1.41 mo x 0.707c
So how much photon energy is lost in the 'collision'?
How to think of fixed wavelength difference --- Suppose the incoming photon
energy is 51,000 ev, which is 1/10 the electron rest mass energy. Then
the incoming photon wavelength is x10 the compton wavelength, so scattered
photons have x11 compton wavelength and would have lost (about) 10% of
their energy in the electron collision. If the incoming photon has 1/100
th of the electron rest mass energy (5,100 ev), it would have a wavelength
x100 compton, and the scattered photons x101 compton for 1% lose of energy
in the collision.
From a frequency point of view photon energy lose goes up linearly with frequency (as 's') with a pole at the compton wavelength. It's only as photon energies approach the rest mass energy of the electron that they begin to loose a significant fraction of their energy in the collision. Or put another way only high energy photons are able to transfer a signifcant fraction of their energy to the electron in a collision. And as the transfer of energy to the electron approaches 0.511 mev the electron will begin to 'recoil' at relativistic speeds.
calculated @ 135 degree: 2.425
x (1 - (-.707)) = 4.14 x 10^-12 m
data @ 135 degree: (0.0749 - 0.0709) = 0.004
x 10^-9 or 4 x 10^-12m
source: Hyperphysics (similar data from my quatum
textbook)
'Real' (photon) size of the electron
(seems to me) Compton's
simple collision model can be thought of as providing hard data on the
real
size of the electron, at least the electron size as seen by a photon
The model is a single photon hitting a single, free, stationary electron.
Can't get a much simpler, cleaner test than that.
As frequency goes up, photon size decreases and can be considered (in some sense) to be about a wavelength in size. It is only when a photon size gets down to the compton wavelength (2.4 x 10^-12 m) that a significant energy transfer takes place, so it can be argued that this is the size range of the electron from a photon's (relativistic?) point of view.
Compare this to a 'Bohr radius' electron size. In an atom the potential well created by the proton it really what sets the sets the size spread of the electron. The electron in a hydrogen atom spreads out roughly x100 times the compton size because it is at this size that electrostatic potential energy (from attraction to a single proton) and the electron's kinetic energy balance. Yet even in the Bohr atom the electron's location, while unknown because of heisenberg, is still considered to be at any given time in some (tiny) part of the electron's orbital volume.
'Real' (particle collision) size of the electron
A smaller
electron size when electrons interact with other particles. Phyics forum
points out that high energy particle experiments with electrons indicate
they look like point particles to something like 10^-16 m, a limit set
by exerimental energy. These experiments crash electrons into other electrons
or positrons.
'Real' (ion trap) size of the electron
The smallest
electron size comes from experiments of single electrons held in 'ion-traps'.
These are magnetic and electrostatic traps at cryogenic temperatures. The
physics forum gave as a reference Dehmelt, who won the Nobel prize for
physics in 1989. The forum guys remembered him putting the electron size
between 10^-19 and 10^-22 m. . Here is his Nobel lecture.
http://nobelprize.org/nobel_prizes/physics/laureates/1989/dehmelt-lecture.pdf
--------------
Classically the E field of incoming radiation should
force electrons to oscillate at that that frequency, so the frequency of
the scattered radiation should be the same as the incoming radiation.
Experiments in 1923
by Compton firing x-rays at materials provided further evidence, in the
words of my atomic quantum textbook , that radiation of the particle like
nature of radiation. Compton scattering is from a 1922 experiment where
an X-ray bean was fired at a carbon target and the frequency vs angle of
the outgoing radiation was measured. It was found at angles near 90 degrees
that there was 2nd peak in emitted radiation at a somewhat lower frequency
than the incident radiation. The interpretation is that the outgoing photon
energy (frequency) is lower because some of the incoming photon ennergy
goes into accelerating (recoiling) the nucleus
of the atom with
the balance being carried off by the outgoing photon. There is a nice simple
treatment of compton scattering in my new atomic quantum physics textbook.
Compton interaction is inelestic vs eleastic from Thomson scattering.
-------------
Millikan measures the charge of an electron
Millikan in 1909
first measured the charge of an electron using charged oil drops. He injected
(tiny) atomized oil drops into the top of a chamber filled with air. Using
a microscope he measured their terminal velocity (steady state rate of
fall in air). He had a formula which allowed him to figure the oil drop
mass from its terminal speed. Millikan's claimed his result was accurate
to 0.1%, but later investigators found he was low by about 0.6% because
he had an incorrect value for the viscosity of air.
He used X-rays to strip electrons off air molecules in the chamber some of which then attached themselves to the oil drops. By this means he was able to create oil drops of varying mass (that he could calculate) and with varying amounts of electrical charge. He then applied a voltage between plates at the top and bottom of the chamber. By making the top plate positive (relative to the bottom plate) oil drops with negative electron charges were attracted upward. The upward force being proportional to the charge on the oil drop and the applied voltage.
A particular oil drop could be made to go up or down depending on the applied voltage. Millikan continually tweaked his voltage working to get oil drops to briefly hover. He recorded the voltage that would get an oil drop to hover, and its terminal speed when the voltage was turned off. The calculation of the charge on a particular oil drop is found by equating the upward electric force with the downward gravity force.
force up = force down
(charge on oil drop) x E = (mass of oil drop) x g
q x E = m x g
q = m x g/E
where
q = charge on a particular oil drop (in coulombs)
m = mass of particular oil drop (in Kg) (found from terminal speed)
E = volts/(separation of plates in meters)
g = 9.8 m/sec^2 (gravitational acceleration on earth)
For fun, and as a reasonableness check, let's calculate about how much voltage Millikan needed. The link says a typical oil drop was 10^-15 kg. I am going to guess the space between his plates was pretty small to keep the voltage down, let's say 2 cm. The highest voltage would be needed for a drop with only one electron.
E = volts/meter = m x g/q
volts = m x g x plate separation/q
= 10^-15 kg x 9.8 m sec^2 x .02m/1.6 x 10^-19 coulomb
= 0.122 x 10^4
= 1,220 volt (OK, he used a >1,000 volt supply)
He repeated this experiment many time times getting a lot of different charge values. Arranged in ascending order the data would look something like a staircase (maybe with some steps missing). This revealed two key features of the electron.
a) electron charge was quantized (because it's a staircase not a line)
b) charge of one electron = height of a single step = 1.6 x 10^-19
coulomb
http://www68.pair.com/willisb/millikan/experiment.html
The above link is to a page on an incredible web site put up by Worsley elementary school in Northern Alberta, Canada! They have hundreds of pages on science and math, 2,500 pages total, and get 170,000 visits/month.
Electron wavelength
de
Broglie in his Phd thesis in 1924 proposed that all elementary particles
might have wave like properties. This was known at the time to be true
only for photons. de Broglie's formula was the same as for photons, i.e.
the wavelength equal to plank's constant divided by the particle momentum
(its mass times its velocity).
wavelength = h/p
where
h = plank's constant
p = momentum (mass x velocity)
The wavelike character of electrons was demonstrated by Davisson of Bell Labs in 1926 when an electron beam fired at a nickel crystal was found to reflect with a diffraction pattern. Here is Davisson's data from his 1937 Nobel lecture showing the wavelength (figured from the diffraction pattern) plotted against the inverse of the square root of the acceleration voltage in his tube. The electron kinetic energy is 1/2 m x v^2 = e x voltage, so the square root of the voltage is proportional to the momentum (& velocity) of the electrons. The data not only fell on a nice straight line, but just the line predicted by de Broglie's equation.
Note in the graph higher energy electrons are at bottom/left, so faster electrons have a smaller wavelengths. QED tells us that as electrons gain energy their smaller wavelengths means they spread out less.
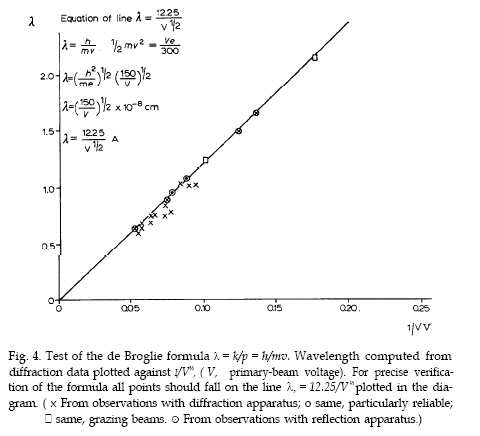
From the equation
on the graph we see that
electron wavelength @ 150 ev = 10^-10 m
check
An electron
accelerated by 150 V acquires a speed (from E = 1/2 m v^2, non-relativistic)
of
v = sqrt{2E/m}
and
wavelength = h/p = h/mv = h/(m sqrt{2E/m}) = h/sqrt{2mE)
= 6.63 x 10^-34/sqrt{2 x 9.11 x 10^-31 x 150 x 1.6 x 10^-19}
= 6.63 x 10^-34/sqrt{4,373 x 10^-50}
= 6.63 x 10^-34/66.1 x 10^-25
= 1.00 x 10^-10 m checks
For fun let's compare above to a photon with the same 150 ev energy. The wavelength of a photon = c/freq and photon energy = h x freq, so
photon wavelength @ 150 ev = c/freq = c/(E/h) = hc/E
= 6.63 x 10^-34 x 3 x 10^8/150 ev x 1.6 x 10^-19 j/ev
= 8.33 x 10^-9 m
The wavelength of a 150 ev photon is 83.3 times longer than an electron with 150 ev. Is this a universal ratio? No, it varies with energy as shown below.
photon wavelength = c/freq = c/(E/h) = hc/E
electron wavelength = h/p = h/sqrt{2mE}
The wavelength of a photon goes down faster with energy than with an electron, so at what frequency are the wavelengths the same?
photon wavelength = electron wavelength
hc/E = h/sqrt{2mE}
(c/E)^2 = 1/2mE
c^2 = E/2m
E = 2mc^2 (approx)
Above is approx because the E used to find the electron de Broglie wavelength was (1/2 m v^2) and the E above at twice the rest mass energy indicates this assumption is wrong. So above is probably telling us that the wavelength of a particle equals the wavelength of a photon with the same energy when the velocity of a particle is approaching c, with a good guess being 0.707c where the particle kinetic energy is equal to its rest mass energy.
de Broglie's Thesis
I came across
a link to a copy of de Broglie's thesis (in english). I read that some
aspects of his theory have been abandoned. Apparently (I have not read
it) he used a working assumption that the mass of a photon, while very
small, might not be exactly zero, but there is no evidence for this, so
standard thinking is that photons travel exactly at c and have exactly
zero rest mass energy.
http://www.ensmp.fr/aflb/LDB-oeuvres/De_Broglie_Kracklauer.pdf
Notes from reading de Broglie's thesis
Interestingly
in 1924 de Broglie gives the value of planck's constant to four decimal
places as 6.545, but this is 1.2% below the modern value (h = 6.6261 j-sec)
starts by
hypothesing that
h freq = m c^2
where m is rest mass
phase wave
is a substantial part of the electron
In a Bohr
atom with circular orbits the 'action' is n hbar or
m0c^2 radius^2 = n hbar
where m0 is rest mass
--------------------
looks funny, lets do a numerical check
radius = sqrt{hbar/m0c^2}
= sqrt{1.05 x 10^-34/9.1 x 10^-31 9 x 10^16}
= sqrt{12.8 x 10^-22}
= 3.58 x 10^-11 m
close, but correct inner Bohr hydrogen radius is 5.29 x 10^-11
--------------------
(he later gives) exact Bohr formula
radius = hbar/m0 velocity
must be coherence with phase wave passing by at small distances
(which leads him to say the radius of an electron must
be about 10^-15 m)
can be regarded as a phase wave resonance condition for an electron in
orbit about a proton
"proper mass" (m0) of a light quanta = E/c^2, where E is its energy
light quanta has associated with it a periodic phenemoa with
freq = (1/h) m0 c^2
classical wave of a photon is a time average of a real distribution of
phase
waves accompanying the light quanta
we may assume that light quanta move with a velocity very close to, but
still slightly less than c
**
we are trying to establish a correspondence between light waves and phase
waves
photographic effects (detection of light by photograhic plates) occur in
proportion to the
electric field intensity
**
(in italics) A phase wave (of light) passing through material bodies induces
them to emit
additional photons whose phase wave is identical to that of the stimulus
(he proposes) that a light emission (by an atom) always comproses just
one
photon
** (interesting discussion of scattering of photons
by atoms)
When wavelength is long compared to 10^-10 m atomic size all the atom electrons
together in-phase, but things are different when wavelengths shrink
to atomic distances.
(this is)
freq = c/wavelength
= 3 x 10^8/10^-10
= 3 x 10^18 hz
Wikipedia says X-ray wavelength is factor of 1,000 (10 to .01 nm)
which is 10^-11 m to 10^-8 m
**
for atoms
h freq = m0c^2 + kinetic energy
he talks about coherence of photon waves, saying this makes them not independent
**
near the end of the thesis he (finally) writes the equation for which he
is famous
(but only for the non-relativistic case, which is surprising because his
whole thesis
s full of relativistic equations)
wavelength = h/p = h/mv where m = m0 (particle rest mass)
=======================================================
Relation between speed of light and electron charge
There is a
fundamental relationship speed of light (c) and the charge of the electron
(e) known by the non-descript name Fine Structure Constant (alpha).
The value of the fine structure was first determined by spectroscopists
from the fine splitting of hydrogen emission lines before its full significance
as the fundamental electromagnetic interaction coefficient was discovered.,
so the name 'fine structure constant' doesn't really do it justice.
The fine structure constant is considered a fundamental constant because it is dimensionless, and it is one of the two dozen or so 'external constants' of the standard model of particle physics. It has been determined experimentally to over 12 digits and has the value (approximately) of 1/137.
Alpha is the ratio of the charge of the electron squared over the speed of light. The scaling constant K contains Plank's constant (h) and the permittivity of free space (e0). e0 is a constant related to the electric field. It scales E^2 to energy stored in the electric field (energy density = 1/2 x e0 x E^2), and e0 with u0 determine the speed of light (c = 1/sq rt {e0 x u0}).
alpha = K x e^2/c or c = K x e^2/alpha
where
K = 1/(2 x eo x h)
where
e0 = permittivity of free space
h = plank's constant
alpha = e^2/(2 x eo x h x c) = (e^2/2h) x sq rt (u0/e0) = 1/137
In the theory of quantum electrodynamics, the fine structure constant plays the role of a coupling constant, representing the strength of the interaction between electrons and photons. Historically, the first physical interpretation of the fine-structure constant was the ratio of the velocity of the electron in the first circular orbit of the Bohr atom to the speed of light in vacuum. The formulation of alpha on the right that excludes c and has the term sq rt (u0/e0) shows that alpha depends on the ratio of magnetic to electric energy in light.
Richard Feynman first found that the Fine Structure Constant (alpha) was involved in the probability a real electron would emit or absorb a real photon. He referred to alpha as "one of the greatest damn mysteries of physics: a magic number that comes to us with no understanding by man." and "It has been a mystery ever since it was discovered more than fifty years ago, and all good theoretical physicists put this number up on their wall and worry about it".
Wikipedia says, "The fine-structure constant is probably the best known dimensionless fundamental physical constant. Nobody knows why it takes on the value that it does (currently measured at about (137.035999)-1). Many attempts have been made to derive this value from theory, but so far none have succeeded".
Whenever gas absorbs light, electrons within the atoms jump from a low energy state to a higher one. These energy levels are determined by how tightly the atomic nucleus holds the electrons, which depends on the strength of the electromagnetic force between them--and therefore on the fine-structure constant. The way in which the wavelengths change depends critically on the orbital configuration of the electrons. For a given change in alpha some wavelengths shrink, whereas others increase. The complex pattern of effects is hard to mimic by data calibration errors, which makes the test astonishingly powerful.
The speed of light also shows up in several equations of constants related to the atom. One of these constants is the Rydberg constant. This constant allows the frequencies of light emitted from hydrogen and other atoms to be calculated. It has been measured very accurately (11 digits).
Rydberg constant = me x e^4/8 x e0^2 x h^3 x c = alpha^2 x me x c/2h
where
me = (rest) mass of electron
e = charge of electron
e0 = permittivity of free space
h = plank's constant
c = speed of light in a vacuum
Dirac
I read that
Dirac in 1931 came up with the definitive, elecgant, theory describing
a free electron. At this point I have zero understanding of it. It appears
to be complex mathematically, and I am going to make no effort to learn
it. A huge achievment of the equation was it properly described electron
spin, which had been recognized since the late 1920's. What amazed everyone,
was when relativity was put in, spin just fell out. Cropper's says Dirac's
starting point was the special relativity energy formula below (more in
'Total Particle Energy' below). It makes the resulting equation fully consistent
with special relativity, whereas Scroedinger's equation, which it replaced,
was not.
E^2 = (cp)^2 +(mc^2)^2
E = +/-sqrt {(cp)^2 +(mc^2)^2}
A second big achievment of the Dirac equation was that it led to the prediction of a new particle (later found), the first anti-matter particle. The left side of the relativistic energy equation is E^2, so taking the square root to get E, yields two solutions, one with positive energy and one with negative energy. One solution was taken to be the electron while the other term was posulated to be an anti-electron, same mass as the electron, but a positive charge (later to be called the positron). Dirac and Schroader shared the Nobel prize for their work.
Cloud chamber shows anti-electrons
In 1932 Carl
Anderson was using the cloud chamber to study the shower of particles made
by cosmic rays, which are mostly high speed protons that come streaming
in from deep space (other galaxies?). A magnetic field pointed across the
chamber makes the visible path of charged particles in the chamber curve.
According to Blackett (Nobel prize winner in 1948 for cloud chamber work)
Anderson found about half of his curving light weight particles
curved the 'wrong way' indicating they had positive charge. They were positive
electrons, Anderson named them positrons. The fact that electrons
& positrons appeared in equal numbers in cosmic ray showers indicated
that they were likely being created (from energy) as matter/anti-matter
pairs.
Anderson found positrons soon after Dirac had predicted they might exist, but he wasn't looking for them, they just popped up in the chamber. Anderson and his grad student Seth Neddermeyer found cosmic rays were making another new particle too, the muon. Anderson got the Nobel prize for finding these particles.
Electron and positron tracks (right and left) in Anderson's
Wilson cloud chamber. The right image is a mirror image of the left.

Cosmic ray shower (with electrons and positrons) recordered
by Anderson on Pike's Peak
Who says the rest mass energy of an electron (&
positron) is 511 kev?
The data below
shows the spectrum of gamma rays emitted by decaying sodium 22. Notice
it has a large sharp spike at 511 kev. Sodium 22 beta decays by emitting
a positron (and neutrino). When the positron comes to rest in the crystal
by 'running into' an electron, a pair of 511 kev photons are created coming
out in opposite directions. (Data taken by MIT undergraduates in x-ray
physics student lab, posted on MIT site.)
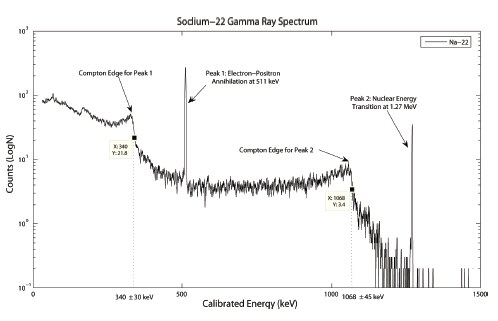
Total particle energy
In electrical
engineering it is very common for a parameter to be the 'root-sum-square'
of two (or more) parameters, meaning parameters are squared, then summed,
then the square root is taken.
Why root mean square?It turns out that formula for total (relativistic) energy of a particle has this form. Energy total is the root-sum-square of kinetic energy (cp) plus rest mass energy (E = mc^2). Note mass in the rest mass formula is (lower case m) rest mass, whereas the mass in momentum (p) is (upper case M) relativistic mass. (For speed of light particles like photons this formula needs to be modified. See the next section.)
Why are rest mass energy and kinetic energy combined in a 'root-sum-square' manner to get total particle energy? Is rest mass energy somehow in quadrature with kinetic energy? I am not sure I know the answer. But I think these two considerations are relevant. One, as my sketch shows 'root-sum-square' combining produces a sharp crossover between the two energy asymptotes similar to the sharp crossover between crossing frequency asymptotes on a Bode diagram. And, two, the 'root-sum-square' energy combination can be rewritten into a very simple form (see below) that is just rest mass energy scaled up by a Lorenz transformation, indicating that it probably falls out of special relativity.
E = sqrt {(cp)^2 +(mc^2)^2}
where
E = total energy
m = rest mass
c = speed of light
p = Mv
(momentum)
M = m/sqrt{1-(v/c)^2} (relativistic
mass)
v = velocity
As an electrical engineer I know that a very useful and powerful way to work with, and better understand, a root-sum-square function is to find and plot its assumptotes.
For example, the impedance (Z) of a series resistor (R) and inductor (L) as a function of frequency (w) is Z = sqrt {R^2 + (Lw)^2}. At low frequencies where Lw < R, we simplify by dropping Lw, then the function simplifies (greatly) to Z=R. At high frequency where Lw>R, we simplify by dropping R, then the function again simplifies (greatly) to Z=Lw. These two simple functions are the assymptotes that the full function approaches at low and high frequencies.The idea is in the summation just retain the largest term (in each variable range) and drop the other terms. What you get is a series of assumptotes that the full function approaches in the variable range where that term is dominate. The intersection and character of the assymptotes throw light on the nature of the full function, which is closely approximated by a 'smoothed' line connecting the assumptotes (see my figure below).
I found assumptotes for the energy formula above (E = mc^2 and E=cp=cMv), and below I plot the assumptotes and total energy vs velocity. I learned a lot doing this. This is a 'step beyond' the textbooks. I have never come across this plot before. I found from this work that kinetic energy of a particle becomes equals its rest mass energy (point at which the two assumptotes cross) at 0.707 c or {c/sqrt(2)}. At speed lower than 0.707 c the total energy of a particle is (pretty much) constant at its rest mass energy. At speeds higher than 0.707 c the kinetic energy dominates over rest mass energy, and the energy of the particle can increase without limit as v approaches c due to the relativistic increase in mass.
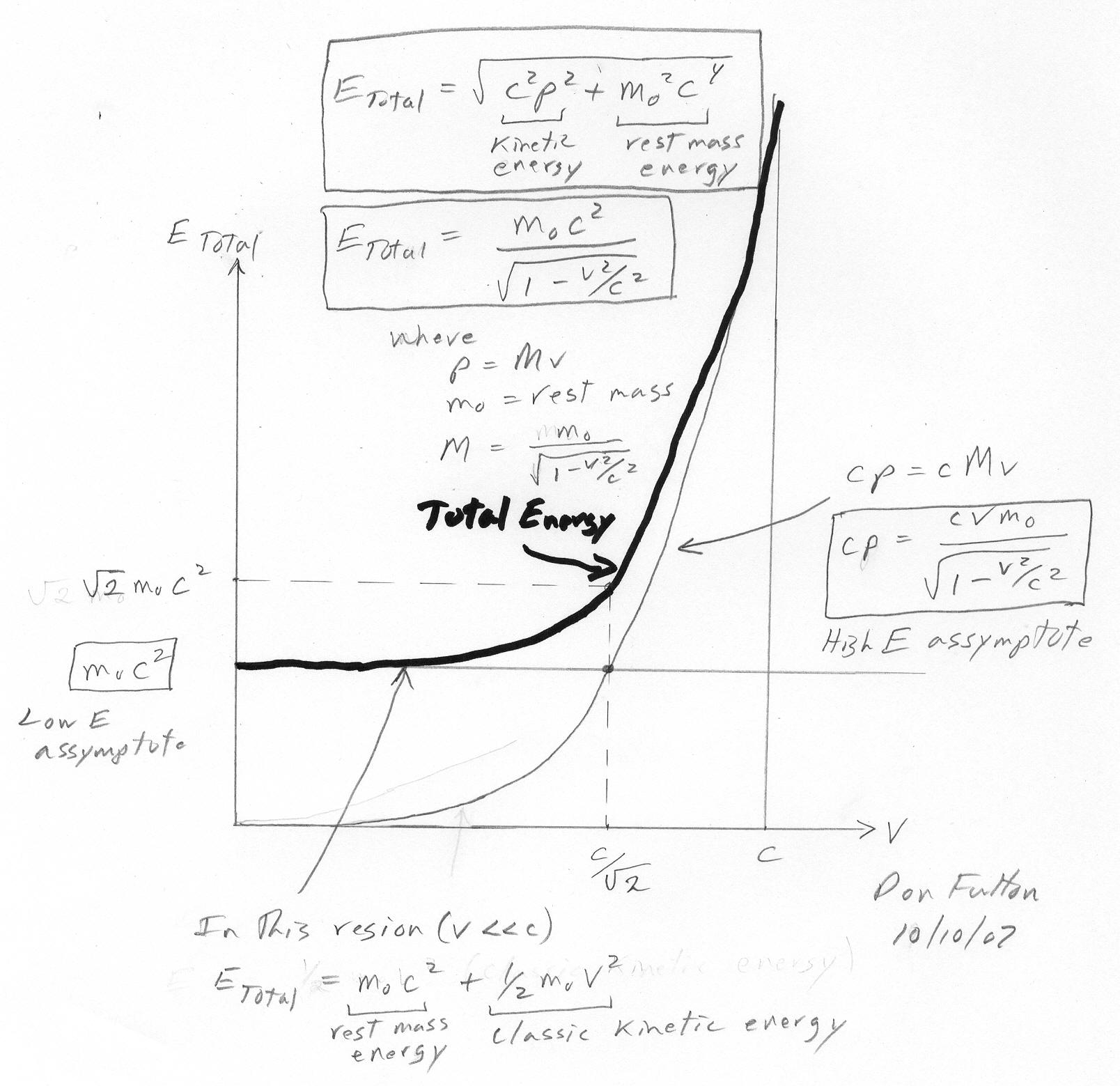
For particles --- Rest mass energy dominates at lower
speed (< 0.707 c) and kinetic energy dominates at higher speed (> 0.707
c)
Interestingly the equations below show the total energy (E) can be written in a simpler manner. The 'root-sum-square' of rest mass energy and kinetic energy is just equal to the rest mass energy scaled up by a Lorenz transformation, or equivalently the relativistic mass x c^2.
E = sqrt {(cp)^2 +(mc^2)^2} = ?= mc^2/sqrt{1-(v/c)^2}
sqrt {(cp)^2 +(mc^2)^2} = sqrt {(cMv)^2 + (mc^2)^2}
= sqrt{[cmv/sqrt(1-(v/c)^2)]^2 + (mc^2)^2}
= sqrt{(cmv)^2/(1-(v/c)^2)] + (m^2c^4}
= sqrt{(m^2c^4)[(v/c)^2/(1-(v/c)^2)] + 1}
= sqrt{(m^2c^4)[(v/c)^2/(1-(v/c)^2)] + (1-(v/c)^2)/(1-(v/c)^2)}
= sqrt{(m^2c^4)[1/(1-(v/c)^2)]}
= mc^2/sqrt{1-(v/c)^2}
checks
Note, however, the we are most often concerned with how much energy of a particle changes. The 2nd term in the total energy formula is a true constant (assuming mass doesn't change), so energy as a function of speed depends only on the first term.
E total = sqrt {(cp)^2 +(mc^2)^2}
E (funct of speed) = cp
= Mvc
= mvc/sqrt{1-(v/c)^2}
Is this consistent with above? Well, it checks at v=c/sqrt{2}
E (funct of speed @ v = c/sqrt{2}) = mvc/sqrt{1-(v/c)^2}
= (mcc/sqrt{2}) x sqrt{2}
= mc^2 checks
E (total @ v = c/sqrt{2}) = mc^2/sqrt{1-(v/c)^2}
= mc^2 x sqrt{2} checks
Since relativistic mass (M) equals m/sqrt{1-(v/c)^2}, the total energy formula just becomes Einstein's famous E = mass x speed of light square with mass being relativistic mass.
E = mc^2/sqrt{1-(v/c)^2}
= M c^2
where
M = m/sqrt{1-(v/c)^2}
E = total energy
In the region where (v<<c) it's easy to show that the total energy formula becomes rest mass energy (mc^2) plus classical kinetic energy (1/2 mv^2).
E = mc^2/sqrt{1-(v/c)^2}
E = mc^2 x {1-(v/c)^2}^-1/2
for v<c we can approximate
E = mc^2 x {1 + 1/2 (v/c)^2}
E total = mc^2 + 1/2 mv^2 checks
Total photon energy
The relativistic
energy-momentum equation in the section above applies directly to non-speed
of light particles, however, it needs to modified for speed of light particles,
like photons. Photons have zero rest mass (m=0) and a speed of c (v=c),
but a direct substitution into the formula for relativistic mass (M = m/sqrt{1-(v/c)^2})
doesn't work (is undefined) because it becomes zero divided by infinity.
Photon energy can be figured as follows:
p = h/wavelength
wavelength x frequency = c
p = h/(c/frequency) = h x frequency/c = E/c
E = cp = h x frequency
In other words for photons the 2nd term in the relativistic-momentum equation is zero, photons have no rest mass., so E = cp = h x frequency. In a sense the energy of a photon is all due to its kinetic energy, which is equal to its momentum times the speed of light. The momentum of photons is real and can be measured. In fact pressure from solar photons on solar sails is used for orientation control in some satellites.
You can also define a relativistic (or inertial) mass of a photon using Einstein's formula as shown below:
photon mass = E/c^2 = h x frequency/c^2 = cp/c^2 = p/c.
Feynman et al
The next step
forward (in the 40's) was a theory of how the electron and photon interact.
This is QED (quantum electrodynamics). Invented by Feynman & a few
others. All the inventor worked the math, but only Feynman provided
those cute interaction (Feynman) diagrams, which helped make the math a
lot more tractable. For a long time QED theory was plagued with infinities,
which were removed in a suspicious way (subtraction), but nevertheless
the theory matched reality very well, able to make many predictions confirmed
to many decimal places.
Muons
The muon is
(essentially) a heavy electron. It has (exactly) the same charge and spin
as an electron (and it's a lepton too), but it's about 200 times heavier
than an electron (about 1/10th the mass of the proton).
Feynman in QED introduces muons this way: "(The muon) is in every way exactly the same as the electron, except that its mass is much higher -- 105.8 Mev, compared to 0.511 Mev for the electron, or about 206 times heavier. It's just as if God wanted to try out a different number for the mass! All the properties of the muon are completely describable by the theory of electrodynamics -- coupling constant j (charge) is the same, (probability formula is same), you just put in a different value for n (mass)." (Feynman's QED book, p143)Unlike an electron the muon is unstable (2.2usec lifetime). However, it exists on earth in large numbers because it is being continually being created by cosmic rays in the upper atmosphere. The very high-energy protons (cosmic rays) that begin the process are thought to originate from acceleration by electromagnetic fields over long distances between stars or galaxies, in a manner somewhat analogous to the mechanism of proton acceleration used in laboratory particle accelerators. An estimated 150 muons per square meter per second come screaming in from the atmosphere at (almost) the speed of light and penetrate hundreds of meters into the surface. Indeed muons form a major part of the natural background ionizing radiation to which we are all exposed.
Muons decay mostly into electrons or positrons (anti-matter electrons) and neutrinos. If they decay is in the atmosphere, the decay products do not reach the surface as the electrons and positrons have little penetrating power and are absorbed quickly by air molecules.
Cosmic ray generated muons have a range of energies, but there is a definite peak in the muon energy spectrum between 2 and 3 GeV (says SLAC) with the mean energy of cosmic ray muons at sea level at about 2 GeV. Since the muon rest mass is (about) 100 MeV/c^2, the relativistic mass increase is about 2 Gev/100 Mev = 20, whereas the usual value obtained from muon time dilation measurements is about six. (They should match, and I have not seen an explanation of this difference.)
The muon was the first elementary particle discovered that does not exist in ordinary atoms. They were identified from tracks in cloud chambers by Anderson in 1936. Muons had a distinctive curvature in a magnetic field different from electrons and protons. They curved less than electrons, but more than protons. The fact that they curved in the same direction as electrons indicated they were negatively charged, and the degree of curvature provided information about their mass and speed.
Standford Linear Accelerator (SLAC) has a real time online muon counter (link below). You can see the number of cosmic ray generated muons detected each minute for the last hour. The SLAC muon detector is pair of panels covered with aluminum foil and dark plastic each about 5 in x 8 in spaced about 20 inches apart. A muon causes a tiny light flash in the panel (scintillation) as it passes through, 20,000 photons per muon says SLAC. The photons are directed into a photomultiplier tube by a plastic light guide. A count is registered only if a scintillation occurs in both panels at (about) the same time. Scintillation is the same method used by Rutherford over a hundred years ago to detect alpha particles (except Rutherford's guys looked directly at the light flashes in a dark room with microscopes). The link below calls this counter a 'cosmic ray detector', but it's a muon detector as SLAC details elsewhere on the site.http://www2.slac.stanford.edu/vvc/cosmicrays/crdatacenter.html
Tau (too)
At higher
energies a still heavier 'electron' was found in the 1970's. It's called
tau and is 16.8 times heavier than the muon. Its mass/energy is 1,777 Mev,
nearly the mass of two protons. In spite of 20 years of looking no electron
type particle heavier than tau has been found. It has (exactly) the
same charge and spin as an electron (and it's a lepton too), and (presumably)
all the QED rules for electrons apply with just a change in n, the mass
term.
There is this difference between tau and the other electron particles (muon and electron). The tau is the only lepton that can decay into hadrons (particles made of quarks); the other leptons do not have the necessary mass. Like the other decay modes of the tau lepton, the hadronic decay is through the weak interaction.
Muon catalyzed fusion
While researching
muons, I came upon an interesting topic that I had never heard of --- 'Muon-catalyzed
fusion'. This is a type of cold fusion using muons, deuterium, and tritium.
What happens if muons are injected into a solution of deuterium and tritium
is this.
Muons being negatively charged can go into 'orbit' around a positively charged deuterium or tritium nucleus forming (sort of) a muon hydrogen atom. But muons weigh about 200 times (x207) more than an electron, which means, due to their 200 times higher energy, they have a (de Broglie) wavelength 200 times smaller than an electron. This causes them to 'orbit' about 200 times closer to the nucleus than an electron. Hence a tritium nucleus that picks up a muon can get about 200 times closer to a deuterium nucleus than normal since the tritium positive charge is so well shielded by the muon. Deuterium and tritium nuclei can get so close that low temperature thermal vibrations are enough (via tunneling) to cause the two nuclei to merge releasing the muon and 17.6 Mev of energy in the form of a fast neutron (14.1 Mev) and an alpha particle (helium nucleus) of 3.5 Mev. Wikipedia puts it this way:
"Due to the strong nuclear force, whenever the triton and the deuteron in the muonic molecular ion happen to get even closer to each other during their periodic vibrational motions, the probability is very greatly enhanced that the positively charged triton and the positively charged deuteron would undergo quantum tunneling through the repulsive coulomb barrier that acts to keep them apart. Indeed, the quantum mechanical tunneling probability depends roughly exponentially on the average separation between the triton and the deuteron, allowing a single muon to catalyze the d-t nuclear fusion in less than about half a picosecond." (Wikipedia - Muon-catalyzed fusion)Note (e^200 = 7 x 10^86), so a muon by allowing the nuclei to come 200 times closer together improves the chance that they will fuse by an astounding 87 orders of magnitude! Also the muon is released in the process so it is available to be reused, which is why this process is called muon catalyzed fusion.
Muon catalyzed fusion has been studied theoretically and experimentally for 50 years (and by some big guns in physics too like Sakharov and Alvarez). In practice fusion does occur (at low temperature), but the efficiency of the process is too low. In principle each muon should be able to catalyze as many as 4 million fusing (2.2usec lifetime/0.5 picosecond fuse time), but in practice the number of fusing per muon is more like 100. The problem is that the alpha decay product has about a 1% chance of capturing the muon. And of course energy must be expended to manufacture both muons and tritium (12 yr lifetime). Muons catalyze deuterium-deuterium fusion too, but for various reasons this process puts out only 2% of the energy of deuterium-tritium fusion. Wikipedia sums up the current status of muon catalyzed fusion this way:
"It remains an active area of research worldwide among those who continue to be fascinated and intrigued (and frustrated) by this tantalizing approach to controllable nuclear fusion that almost works. Clearly, as Jackson observed in his 1957 paper, muon-catalyzed fusion is "unlikely" to provide useful power production… unless an energetically cheaper way of producing mesons (muons) can be found."Electrons from photons
In Feynan's book on QED for the lay reader (QED) many of the Feynman diagrams show photons splitting into electron/positron pairs that rapidly recombine into a photon again. In the diagrams the wavy line (photon) changes to a circle (electron/positron) and back to a wavy line again. Feynman is not clear if these are real or virtually particles, probably because as far as QED probability calculations go it does not matter.
Aside --- Feynman in QED says electrons in atoms 'orbiting' the positive nucleus are constantly exchanging photons with the nucleus, but says later that these are virtual photons.So how much energy does the photon need to do this? Cropper in his book says that counterintuitively the energy need not be all that high, because for a time specified by the uncertainty principle the energy needed for an electron/positron pair can be borrowed from the vacuum and then quickly paid back when the electron and positron recombine. The electron/positron created this way are virtual particles, meaning they are not directly detectable.
I find on (what appears to be) a high quality, comprehensive, physics web site (hyperphysics) that the photon energy required to generate a real electron/positon pair must exceed 1.022 Mev. Both the electron and positron have 0.511 Mev of rest mass energy, so this is equivalent to saying the energy of a photon must exceed the total energy of the real particles it creates.
We can estimate the minimum (photon) frequency needed to create real electrons by remembering that the energy of visible light photons is only a few ev. Since photon energy increases linearly with frequency, for a photon to generate a real electron/positron pair it must have a frequency almost a million times the frequency of visible light, approximately 10^6 x 4 x 10^14 = 4 x 10^20 hz.
References
Charge of
an electron = 1.6 x 10^-19 coulomb
Mass of an
electron = 9.1 x 10^-31 kg
angstrom
= 10^–10 meter
-------------------------------------------------------------------------
Electron field
An isolated,
stationary electron, or any charged particle, can be viewed as radiating
an electric field in all directions. Electrical engineers visualize this
electric field, or 'E' field, as a bunch of (straight) arrows coming out
in all directions and heading toward infinity. The length of the arrows,
which represent the local strength of the electric field, shorten inversely
as the square of the distance from the electron. The convention is that
the arrows point outward if the charge is positive and for a negative particle
like the electron the arrows point inward.
The arrows are useful because indicate what force anywhere in space that a 'test charge' will feel. If the test charge is another electron, then its charge is negative (q = -e) so the force is the opposite direction from the inward arrows, meaning it is outward. A complicated way of saying two electrons repel. The general equation for the electric force on charged particle is below.
force = q x E
where
force and E are vectors
q is charge of test charge
Here's a common sense way of seeing why the E field falls off as the square of the distance. As a thought experiment, assume the field of an electron 'turns on' at time t=0. When it 'turns on', it begins to radiate out an E field in every direction that grow with the speed of light. If at time t=t0 the E field sphere around the electron has radius r=r0, then at twice the time (t=2t0) the radius has doubled (r=2r0).
Energy for sphere of 1/2 radius
distance dn 2
E up 2^2 = 4
energy density up E^2 = 16
vol dn 2^3=8
energy in inner sphere = 16 x 1/8 = 2
E and B fields
There are
two types of electromagnetic fields: E (electric) fields and B (magnetic)
fields. E fields arise from an unbalance in charge and B fields arise from
the motion of charges. Both types of fields store energy within
the field itself, and in the real world this property is exploited all
the time. Almost every electronic circuit board contains components that
are optimized to store energy in the electric field (capacitors) and in
the magnetic field (inductors).
What about gravity?
Harvard physicist
Lisa Randall says in her book 'Warped Passages' (page 250) that the repelling
electromagnetic force between two electrons is "about a hundred, million,
trillion, trillion, trillion" (or 10^2 x 10^6 x 10^12 x 10^12 x 10^12 =
10^44) times larger than the attractive gravity force they feel. Let's
check the numbers, since both forces fall off as distance squared, the
ratio is unaffected by the distance between the two electrons.
Fcoulomb/Fgravity = [(1/4 pi e0) e^2/r^2]/[G m^2/r^2]
= (1/4 pi e0) e^2/G m^2
= (1/12.6 x 8.8 x 10^-12) (1.6 x 10^-19 coulomb)^2
/6.67 x 10^-11(9.1 x 10^-31 kg)^2
= 8.89 x 10^9 (2.6 x 10^-38 coulomb)^2
/6.67 x 10^-11(83 x 10^-62 kg)^2
= 23 x 10^-29
/554 x 10^-73)
= 0.04 x 10^44
= 4 x 10^42
Randall is only off by a factor of 100! I found
a confirming reference (42 orders of magnitude)
==============================================================
How do electrons/atoms absorb photons?
How can a
tiny atom 0.3 nm dia, or electrons in an atom, absorb light which has wavelength
thousands of times longer? Can this be explained without resorting
to quantum mechanics? The section below attempts a classical explanation.
It is in two parts: first, an argument is made on how small driven antennas
can capture much more power from a field. It makes sense to me, but seems
to be based on some odd ball papers. (check this out) The second
part is pure speculation that atoms might do the same thing. No data or
references here, just speculation. Still it's interesting.
Do atoms have resonance and act like small driven
antennas?
Here is an interesting (speculative) argument using classical EM field
theory that atoms may be like small driven antennas. An LC resonator
(by driving the antenna) can "funnel" incoming electromagnetic waves towards
tiny (much smaller than 1/3 wavelength) antennas. Technically the EA (effective
aperture) of a small antenna is increased. Below is the poynting vectors
due to superposition of the local field of the driven antenna combined
with the incoming field. Supposedly AM radio ( & crystal radio) antennas
are coupled directly to a high Q LC circuit and work this way.
http://amasci.com/freenrg/sukdynam.html
http://amasci.com/tesla/nearfld1.html

EM energy flow around a small antenna (Poynting vector
field)
If an atom resonates electromagnetically at the same frequency as the incident light waves, then, from a Classical standpoint, that atom's internal resonator will store EM energy accumulated from the incoming waves. It will then behave as an oscillator, becoming surrounded by an increasingly strong AC electromagnetic field as time goes by. If this alternating field is locked into the correct phase with the incoming light wave, then the atom's fields can interact with the light waves' fields and cancel out quite a bit of the light energy present in the nearfield region around the atom. The energy doesn't vanish, instead it ends up INSIDE the atom. Half of the energy goes into kicking an electron to a higher level, and the other half is re-emitted as "scattered" waves. (This antenna theory from two obscure 1980's papers, one in a Soviet journal)
The speculation
continues that when the fields build up to intense levels that something
changes ('breaks') and the energy contained within the oscillating
fields is suddenly dumped into some other energy-storage mechanism, and
the resonance of the "circuit" is ruined. The energy has gone into lifting
the electron to a higher orbital, and orbital which has a different resonant
frequency. The analogy is that in an LC oscillator you can capture
& hold the oscillating energy by opening a series switch when all the
energy is in the capacitor.
--------------------------------------------------------
Oddball paper claiming electrons are black holes,
but notes in last half have a good discussion of electron size issues
www.jayel.net/wmm/bhe2.doc
-------------------------------------------------------
According
to QED, the forces between electric charges are carried by virtual photons
which travel back and forth between them, and the space around each charge
is filled with a cloud of virtual charges which are created in pairs and
disappear before they can be detected. The virtual charges form dipoles
which group in such a way that the point-like charge of an electron is
smeared out over a greater volume to such an extent that the electron itself
even looses its identity.
--------------------------------------------------
** reader feedback to a (really) oddball paper
Well, it impresses me.
I imagine that if I discovered "THE SINGLE EQUATION EXPLAINING THE ENTIRE UNIVERSE" then I wouldn't bother with peer-review by comptent scientists or writing it up in full mathematical form with predictions for science journals.Instead I'd immediately post it on for the instant gratification of my true peers. Obviously geniuses of this magnitude don't need to sweat spelling the title of the post correctly. Misspellings are part of the burden of being a supergenius who starts his own branch of science.
Has anyone
called the Nobel Committee?
------------------------------------------
posting & reply (interesting) --- electrons do
not really 'orbit' ?
>Do you know, without doubt, that *all* motion
in that atom were to
>cease that there would still be an emission?
There is no ``motion''
going on. That's why an electron in a particular energy level is called
a ``stationary state''. If you disagree with that, then I suggest you try
to explain an atomic s-state, which has an angular momentum of zero and
is spherically symmetric. If the electron actually orbited the atom, the
orbit would define an orientation and the atom could not be spherically
symmetric. You also can't use the
argument that the direction it defines averages to zero.
It's possible to tell the difference.
--------------------------------------------------------
Physics forums
There are Google groups on phyics (above is from one) --- check it out (here is a link)
another one -- Physics Banter
http://www.physicsbanter.com/particle-physics/46451-qm-predictions-4.html
--------------------------------------
fine structure constant & electron charge
fine structure constant = e^2/(h xc) = 1/137
oddball paper (but reference for fine structure constant
is Purdue Univ KEK collider, Koltick (scientist)
tests with
high energy particle fired at electrons to penetrate the electron cloud
measure
fine structure constant = 1/128.6
supposedly
the calculated value (unshielded) is 1/128.5
---------
** oddball zero point energy paper (these papers have
a lot on vacuum)
This paper has three excellent references 42 -45 and
says this
This has been explored theoretically for the case of the reduced energy density of the vacuum between two Casimir plates. Scharnhorst [42] and Barton [40] linked this reduced energy density with a proportionally reduced permittivity and permeability of the vacuum and a higher light-speed that would result. This is explained in the QED model by the fact that a decrease in the energy density of the ZPE would also result in a decrease in the number of virtual particles per unit distance. Light photons are absorbed by these virtual particles and then re-emitted. As Barnett has pointed out, “this process makes a contribution to the permittivity of the vacuum (e ) and therefore to the speed of light” [43]. Consequently, if there is any decrease in the ZPE and the number of virtual particles per unit distance, then the speed of light will inevitably increase. Conversely, with any increase in the ZPE, and thus the number of virtual particles per unit distance, the speed of light will inevitably decrease.
In summarising these results, Barnett commented that “The vacuum is certainly a most mysterious and elusive object … The suggestion that [the] value of the speed of light is determined by its structure is worthy of serious investigation by theoretical physicists” [43]. Latorre ... concluded that if a vacuum had a lower energy density (fewer virtural particles) than the standard vacuum, then light-speed would be proportionally greater than the standard speed.
(to explain u0 the argument is given that H to E is ratioed by impedance of free space (377 ohms?) and that is fixed. Also energy of H^2 equals E^2, so its only necessary to expain changes in e0)
Seven possible sizes of electron
Of immediate interest
here is the size of the electron. MacGregor has pointed out that there
are seven possible values for this quantity [67]. They range from the Compton
radius of 3.86151 ´ 10–11 cm to those measured by high-energy scattering
experiments that come out to something less than 10–16 cm. The classical
electron radius of 2.81785 ´ 10–13 cm was obtained from calculations
based on the mass being entirely electro-magnetic in origin [F. 66]. Interestingly,
from the SED approach, Haisch, Rueda, & Puthoff point out that “one
defensible interpretation is that the electron really is a point-like entity,
smeared out to its quantum dimensions by the ZPF fluctuations” [10]. MacGregor
initially emphasised that this “smearing out” of the electronic charge
by the ZPF involves vacuum polarisation and the Zitterbewegung [67]. When
Haisch, Rueda & Puthoff did the calculations for SED using these phenomena,
the Compton radius for the electron was indeed obtained.
10. B. Haisch, A. Rueda and H. E. Puthoff, Spec. in Sci.
and Tech. 20 (1997), 99.
42. A. Scharnhorst, Phys. Lett. B 236:3 (1990), 354.
43. S. M. Barnett, Nature 344 (1990), 289.
44. J. I. Latorre, P. Pascual and R. Tarrach, Nuc. Phys.
B 437 (1995), 60.
67. M. MacGregor, “The Enigmatic Electron,” p. 5-6, Dordrecht:
Kluwer, 1992.
----------------------------------------------------------------------------
Electron and neutrino symmetry (check)
--- Lee Smolin says electron and neutrino
are (in some sense) the same. The electron and neutron are treated symmetrically
in the electroweak theory. The electron gets its mass from the (postulated)
electron Higgs particle and the neutron from the (postulated) neutrino
Higgs particle, but due to broken symmetry the world is filled only with
electron Higgs particles. This explains (to a particle physict) why the
electron has all this mass relative to the neutrino. It's because it has
to drag around a bunch of Higgs particles, whereas the neutrino does not.
(Smolin did not explain how the charge difference between the neutrino
and electron comes about..)
-- In the Stern-Gerlach
experiment an atomic beam of spin 1/2 was split into 2 beams by an inhomogeneous
magnetic field. From this observation it was concluded that the magnetic
moment can only have 2 orientations in space and that therefore only 2
orientations of the particle's spin are possible. (oddball)
========================================================
oddball papers, but interesting, paper explaining electrons
classically
http://www.ag-physics.org/electron/
http://www.memetaworks.com/e_as_Quantum/
First derive a radius of 3.86 x 10^-13 m !!
2nd derives compton wavelength. If this is the compton
wavelength is circumferance of of an orbit the 3.86 x 10^-13 m becomes
a radius
=========================
Wow (very intesting Univ of Ill site)
http://online.physics.uiuc.edu/courses/phys435/fall07/Lecture_Notes/P435_Lect_01_QA.pdf
I independently
figure out that this explains the speed of light, nicely confirmed here!!
What is the physics origin of the 1/e0 dependence of
Coulomb’s
force law?
At the microscopic level, virtual photons exchanged between two electrically
charged particles propagate through the vacuum – seemingly empty space.
However, at the microscopic level, the vacuum is not empty – it is a very
busy/frenetic environment – seething with virtual particleantiparticle
pairs that flit in and out of existence – many of these virtual particle-antiparticle
pairs are electrically charged, such as virtual e+ e- , and muon+ muon-
,tau+ tau- pairs{heavier cousins of the electron}, 6 types of quark-antiquark
pairs qq and also W W+ ? pairs (the electrically-charged W bosons are one
of two mediators of the weak interaction), as allowed by the Heisenberg
uncertainty principle (delta E delta t) > hbar. The macroscopic, time-averaged
electric permittivity of free space 8.85 10^-12 F/m is a direct consequence
of the existence of these virtual particle antiparticle pairs at the microscopic
level.
4.) What is
the physics origin of the 1/4 pi factor in Coulomb’s force law?
At the microscopic level, electrically charged particles
emit virtual photons into 4pi steradian.
A quasi-atom called the positronium can be made with the positron (anti-electron) replacing the proton (lifetimes 0.1 to 1 usec).
positronium radius = 2.65 x 10^-11m (exactly?? (see below) Bohr 1/2
radius = 1/2 a0)
Interesting
Formula for
electron energy (in an atom) has a mass in it called 'reduced mass'.
This turns out to be the parallel equivalent of the positive center particle
(proton or positron) and electron. In hydrogen this is (about) 2,000/2,001
x electron mass, whereas for the positronium it is exactly 1/2 electron
mass. (Wikipedia)
energy = - (reduced mass) q^4/8 h^2 e0^2 x 1/n^2
= - 6.8 ev/n^2 (positronium) {-13.6 ev/n^2 for
hydrogen}
where n = 1,2,3
fine structure
constant = dimensionless strength of EM interactions (at low energies)
(I think it has been shown to vary at high energies)
Bohr radius derived by equating the classical potential energy to the rest mass energy.
=========================
Physics of Hard Drives Wins Nobel Prize
By DENNIS OVERBYE
NYT 10/10/07
(Info on giant
magnetoresistance effect -- quantum electron spin effect that (in effect)
amplifies small magnetic fields)
Two physicists who discovered how to manipulate the magnetic and electrical properties of thin layers of atoms to store vast amounts of data on tiny disks, making iPods and other wonders of modern life possible, were chosen as winners of the Nobel Prize in Physics yesterday. Albert Fert, of the Université Paris-Sud in Orsay, France, and Peter Grünberg, of the Institute of Solid State Research at the Jülich Research Center in Germany, will share the $1.5 million prize awarded by the Royal Swedish Academy of Sciences.
Dr. Fert, 69, and Dr. Grünberg, 68, each working independently in 1988, discovered an effect known as giant magnetoresistance, in which tiny changes in a magnetic field can produce huge changes in electrical resistance.
The effect is at the heart of modern gadgets that record data, music or snippets of video as a dense magnetic patchwork of zeros and ones, which is then scanned by a small head and converted to electrical signals. Experts said the discovery was one of the first triumphs of the new field of nanotechnology, the science of building and manipulating assemblies of atoms only a nanometer (a billionth of a meter) in size.
The scanning heads in today’s gizmos consist of alternating layers only a few atoms thick of a magnetic metal, like iron, and a nonmagnetic metal, like chromium. At that small size, the strange rules of quantum mechanics come into play and novel properties emerge. The Nobel citation said Dr. Fert and Dr. Grünberg’s work also heralded the advent of a new, even smaller and denser type of memory storage called spintronics, in which information is stored and processed by manipulating the spins of electrons.
It has long been known that magnetic fields can affect the electrical resistance of magnetic materials like iron. Current flows more easily along field lines than across them. The effect was useful for sensing magnetic fields, and in heads that read magnetic disks. But it amounted to only a small change in resistance, and physicists did not think there were many prospects for improvement. So it was a surprise in 1988 when groups led by Dr. Fert at the Laboratoire de Physique des Solides and by Dr. Grünberg found that super-slim sandwiches of iron and chromium showed enhanced sensitivity to magnetic fields — “giant magnetoresistance,” as Dr. Fert called it. The name stuck.
The reason for the effect has to do with what physicists call the spin of electrons. When the magnetic layers of the sandwich have their fields pointing in the same direction, electrons whose spin points along that direction can migrate freely through the sandwich, but electrons that point in another direction get scattered. If, however, one of the magnetic layers is perturbed, by, say, reading a small signal, it can flip its direction so that its field runs opposite to the other one. In that case, no matter which way an electron points, it will be scattered and hindered from moving through the layers, greatly increasing the electrical resistance of the sandwich.
As Phillip Schewe, of the American Institute of Physics, explained, “You’ve leveraged a weak bit of magnetism into a robust bit of electricity.” Subsequently, Stuart Parkin, now of I.B.M., came up with an easier way to produce the sandwiches on an industrial scale. The first commercial devices using giant magnetoresistance effect were produced in 1997.
Dr. Grünberg
was born in Pilsen in what is now the Czech Republic and obtained his Ph.D.
from the Darmstadt University of Technology in Germany in 1969. He has
been asked many times over the years when he was going to win the big prize,
and so was not surprised to win the Nobel, according to The A.P. Dr. Fert
was born in Carcassonne, France, and received his Ph.D. at the Université
Paris-Sud in 1970. He told The A.P. that it was impossible to predict where
modern physics is going to go.
----------------------------------------------
-- If the
incident X-ray energy is much greater than the binding energies of electronic
states within the atom then the electrons may be considered to be free
and Thompson scattering holds.
-- The X-ray wavelengths used for crystallographic structure determination are typically of similar size to the dimensions of the atoms being investigated (of the order of 1 Å, or 10^-10 m)
-- Detectors can measure with precision many aspects of photons. These include energy, direction, time of arrival, and polarization.
-- Scattering off of free electrons. At low energy, UV, this process is elastic (the photon energy after scattering equals the photon energy before scattering), and is called Thomson scattering.
-- Free-free absorption. A photon can be absorbed by a free electron (i.e., one not in an atom) moving past a more massive charge (such as a proton or other nucleus). The inverse process, in which a photon is emitted by an accelerating charge, is called bremsstrahlung.
-- Atomic absorption.
The two main types are bound-free (in which an electron is kicked completely
out of an atom by a photon) and bound-bound (in which an electron goes
from one bound state to another). Free-free and bound-free absorption cross
sections tend to decrease with frequency like ? (in the bound-free case
this of course applies only above the ionization threshold). Bound-bound
absorption is peaked strongly
around the energy difference between the two bound states.
-- Molecular absorption. The extra degree of freedom associated with multiple atoms in a molecule allows for vibrational and rotational transitions. For relatively simple reasons, there tends to be a strong ordering of energies: atomic >>vibrational >> rotational
** -- For our purposes “high energy” photons means that the photon energy is comparable to or larger than the rest mass-energy of an electron (0.511 Mev) (This is wavelength equal to Compton wavelength.)
-- At these energies, the photon momentum is significant. As a result, electron recoil must be included in electron scattering.
** --- To understand this, consider a photon scattering off an electron. In a classical sense, what is happening is that the oscillating electric field of the photon accelerates the electron up and down. Accelerated charges radiate, thus the electron sends out a photon in some direction. The net result is that the photon hits the electron and bounces off in some other direction.
-- How would
this change if it occurred in a very strong magnetic field? If the electron
moves parallel to the field, there is no difference because there is no
resisting force. Therefore, for photons polarized along the field, the
scattering cross section is basically the same as it was before (roughly
Thomson). However, for photons polarized across the field it’s different.
The electron has great difficulty moving in that direction, so it is tough
to radiate and thus the cross section is decreased a lot.
----------------------------------------------------------------
(paper below discusses photon energy in relation to human
radiation, so it focues on photons with energies of MEV and higher)
Interactions of Photons with Matter
Photons are
electromagnetic radiation with zero mass, zero charge, and a velocity that
is always c, the speed of light. Because they are electrically neutral,
they do not steadily lose energy via coulombic interactions with atomic
electrons, as do charged particles. Photons travel some considerable distance
before undergoing a more “catastrophic” interaction leading to partial
or total transfer of the photon energy to electron energy. These electrons
will ultimately deposit their energy in the medium. Photons are far more
penetrating than charged particles of similar energy.
Energy Loss Mechanisms
* photoelectric effect
* Compton scattering
* pair production
Photoelectric Effect
In the photoelectric
absorption process, a photon undergoes an interaction with an absorber
atom in which the photon completely disappears. In its place, an energetic
photoelectron is ejected from one of the bound shells of the atom. For
gamma rays of sufficient energy, the most probable origin of the photoelectron
is the most tightly bound or K shell of the atom.

The photoelectron appears with an energy given by Ee- = hv – Eb (Eb represents the binding energy of the photoelectron in its original shell) Thus for gamma-ray energies of more than a few hundred keV, the photoelectron carries off the majority of the original photon energy. Filling of the inner shell vacancy can produce fluorescence radiation, or x ray photon(s).
The photoelectric
process is the predominant mode of photon interaction at
* relatively low photon energies
* high atomic number Z
The photoelectric interaction is most likely to occur if the energy of the incident photon is just greater than the binding energy of the electron with which it interacts. This severe dependence of the photoelectric absorption probability on the atomic number of the absorber is a primary reason for the preponderance of high-Z materials (such as lead) in gamma-ray shields.
Compton Scattering
Compton scattering
takes place between the incident gamma-ray photon and an electron in the
absorbing material. It is most often the predominant interaction mechanism
for gamma-ray energies typical of radioisotope sources. It is the most
dominant interaction mechanism in tissue.
In Compton scattering, the incoming gamma-ray photon is deflected through an angle with respect to its original direction. The photon transfers a portion of its energy to the electron (assumed to be initially at rest), which is then known as a recoil electron, or a Compton electron.
All angles
of scattering are possible. The energy transferred to the electron can
vary from zero to a large fraction of the gamma-ray energy. The Compton
process is most important for energy absorption for soft
tissues in the range from 100 keV to 10MeV.
The Compton
scattering probability is is symbolized ? (sigma):
* almost independent of atomic number Z;
* decreases as the photon energy increases;
* directly proportional to the number of electrons per gram, which only
varies by 20% from the lightest to the heaviest elements (except for hydrogen).
Maximum energy transfer to recoil electron:
* angle of
electron recoil is forward at 0°, ? = 0°,
* scattered
photon will be scattered straight back, ? = 180°
The Table below illustrates how the amount of energy transferred to the electron varies with photon energy. Energy transfer is not large until the incident photon is in excess of approximately 100 keV. For low-energy photons, when the scattering interaction takes place, little energy is transferred, regardless of the probability of such an interaction. As the energy increases, the fractional transfer increases, approaching 1.0 for photons at energies above 10 to 20 MeV.
Pair Production
If a photon
enters matter with an energy in excess of 1.022 MeV, it may interact by
a process called pair production. The photon, passing near the nucleus
of an atom, is subjected to strong field effects from the nucleus and may
disappear as a photon and reappear as a positive and negative electron
pair. The two electrons produced, e- and e+, are not scattered orbital
electrons, but are created, de novo, in the energy/mass conversion of the
disappearing photon.
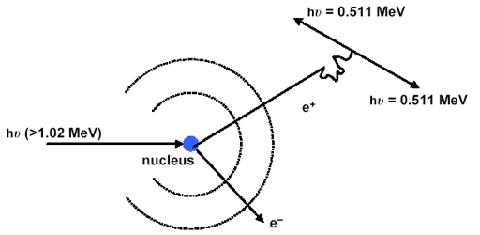
The kinetic energy of the electrons produced will be the difference between the energy of the incoming photon and the energy equivalent of two electron masses (2 x 0.511, or 1.022 MeV).
Ee+ + Ee- = hv - 1.022 (MeV)
Pair production probability, symbolized
? (kappa),
* Increases
with increasing photon energy
* Increases
with atomic number approximately as Z2
Photoelectric effect: produces a scattered
photon and an electron, varies as ~Z4/E3
Compton effect: produces an electron,
varies as ~ Z
Pair production: produces an electron
and a positron, varies as ~Z2
Energy transferred “locally” i.e., to electrons.
photoelectric
effect -- is the average energy emitted as fluorescence
Compton scattering
-- Eavg/h? is the average photon energy converted into electron energy
pair production
-- (h?-mc2)/h? is the fraction of energy converted to photons by
e+, e- annihilation.
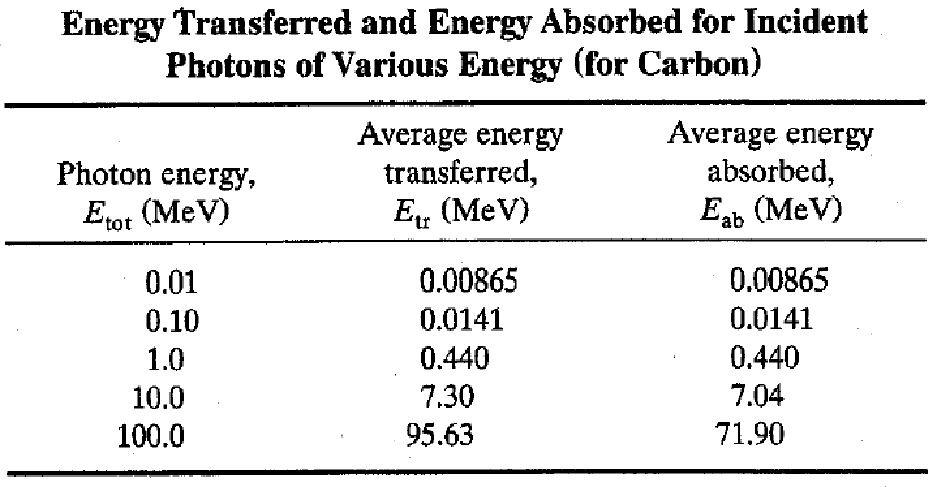
-----------------------------------------------------------------
Mamet's say Bohr radius changes explain relativity
I found an
interesting set of (oddball) papers (books too!) by a (real) physics professor
in Canada (Paul Marmet). It's interesting because he pushes Bohr and classical
physics argments hard to explain effects of special and general relativity.
On a quick look it appears he argues (due to his principle of conservation
of mass-energy) Bohr radius (a0 = 4 pi e0 hbar^2/m e^2) is a key parameter
1) Bohr radius increases in a gravitation field --- Electron mass goes down in a gravitational field, since things in gravitation potential wells have given up energy. Lower mass causes the Bohr radius to go up, which results in the DeBroglie wavelength fitting into the orbit to be longer. Hence emitted energy due to electrons jumping orbits is less. For this reason atomic clocks runs slower in gravitational fields (no need for general relativity!).
2) Bohr radius increases with velocity --- From argument 1) you would think that (rapidly) moving hydrogen atoms would have smaller Bohr orbits. While this might explain 'length contraction' it would be inconsistent with 'time dilation'. But Mamet says no, moving hydrogen atoms have larger Bohr radii. True he says the electron mass is higher, but Planck constant also changes (why? well maybe it's this argument: E = h x frequency, and as frequency goes down (due to time dilation) and E stays constant, h must go up) and it's squared in the equation, hence the Bohr radius goes up. This explains special relativity time dilation! (What about length contraction? see 3)
He calculates clasically that the Bohr radius increases when an atom moves at relativistical speeds, and since n wavelengths still fit in each orbit, the wavelength goes up (hence frequency down) as the atom is seen to go faster. At 0.866c he has Bohr radius doubled. The claim is that this is the 'true' cause of moving atomic clocks running slow, not time dilation. Why, because Planck's constant changes! --" One must then realize that when the velocity increases, the electron mass becomes larger but the decrease of the Planck parameter corresponds to a decrease of the force between the electron and the proton."
3) Length contraction does not happen! --- -- "This means that the size of all material matter increases with velocity. The fact that we are led from our reasoning to length dilation instead of length contraction does not represent a problem since the assumed phenomenon of length contraction has never been observed experimentally in special relativity. On the contrary, we need length dilation to be compatible with the slowing down of clocks, which is also required by quantum mechanics and has been observed experimentally."
Author of more than 100 papers in the field of Electron Spectroscopy. Professor, Physics, Laval University, Québec, Canada: 1962-83, Senior Research Officer, National Research Council of Canada: 1983-90, Visiting, Adjunct, Professor, University of Ottawa, 1990-99. Laval is a major univeristy with 37k students where teaching is in French. According to an Marmet's obituary (by his son), he was a reputable experimentalist with grants (even president for one year of the Canadian physicts association), but had his research grants dryed up and eventually got fired because of his work on fundamental physics (claiming it was all wrong!)
http://www.newtonphysics.on.ca/EINSTEIN/index.html
===============================================================
Quantum mechanics
created (9/08)
Good intro lecture (Arkansas State)
http://www.clt.astate.edu/sreeve/Dawn%20of%20Quantum%20Theory.pdf
Decoding Feynman's book QED
If you know
a little bit of physics (like I do) then Feynman's QED book, while very
interesting, is also frustrating. For example, what really are the 'phases'
of those 'arrows' he sums? What really is 'j' the coupling constant?
How was it determined? In a footnote (p91) it says 'j' is "sometimes" called
the charge, but later we find out it's not really exactly the (measured)
electron charge, which of course is 'e'! On page 90 he introduces the electron
parameter 'n' without telling you that it's the electron mass (m),
well not really, but it's sort of the electron mass. What units
is he using? Feynman does not make it clear in his examples where the photons
are real, and where they are just mathematical constructs (virtual
photons) to allow calculations. Or does Feynman think all his photons are
real? He drops hints here and there, but they are scattered over 100 pages
in odd comments and in footnotes.
There is a little more information in the video lectures (recorded in New Zealand in 1979), but watching the (free) streaming video from New Zealand is really tedious, because in my experience watching one it repeatedly froze and had to be restarted again and again. Oh how I wish a few page appendix had been added to the book to take it up a level or two for those interested. The new (2006) edition of QED with its new forward adds little.
Feynman first applies his stopwatch model with the phase summation to photons. The stopwatch rotates while the photon travels from source to target. Quite a few pages later (p 34, p102) he explains the rate of turning of the stopwatch hand depends on the color of the light, saying the "the amplitude for a (monochromatic) blue source turns nearly twice as fast as for a red source." Then on page 103 we get (paraphasing), you know I told you the stopwatch was turning as the photon traveled, well 'forget that shit'. He now says "the angle of the amplitude for a given path depends on the time (emphasis in original) the photon is emitted from the monochromatic source (adding in the video that his source is a constant energy source). "Once the photon has been emitted there is no further turning of the arrow" as the photon moves from point to point.
Photon phasede Broglie wavelength of a photon
Feynman's light source is a pure (narrow freq color) sinusoid. The sources amplitude vector has constant amplitude, constant frequency (constant energy) and a linearly increasing phase. At the detector light arriving via a longer path (or through some transparent material) has a different phase only because it had to have been emitted earlier from the sinusoidal source.But at this time I am confused about photon 'phase'. Is there such a thing? He tells us light is not a wave it is photons, Feynman spends nearly an hour in the first video lecture hammering this home. And the photons each have a 'frequency', well sort of, maybe its best thought of as an energy, yet the 'phase' of the photons, which determines how they interfere, is determined by (only?) by the phase of the source at the time they are emitted? (A study of short laser pulses and how slows speed of light in materials affects interference would be helpful. Or is this an example of the classic problem of not being able to relate (in any common sense way) quantum physics probability amplitudes to pictures in the real world!) I read that the conventional quantum mechanical view is that there is no wave function for the photon.
de Broglie wavelength = h/p
The general formula for momentum is (relativistic mass x velocity). The speed of a photon is c, and we get the relativistic mass of a photon from its energy (E = M c^2).
p = M x v
= (E/c^2) x c
= E/c
The energy of photon is just its frequency scaled by planck's constant (E = h x freq), and the general formula relating frequency, wavelength, and velocity is (frequency x wavelength = velocity). So
p = h x frequency/c
= h x (c/'color' wavelength)/c
= h/'color' wavelength
or
'color' wavelength = h/p
Yup, same as de Broglie wavelength
Photon conclusion --- Normal 'color' wavelength of a photon is its de Broglie wavelength!
Confirmation --- Feynman's offhandedly comments in lecture one that the "rate of turning" of the photon arrow is about 10^15 times/sec (visible light frequency is 4 to 7 x 10^14 hz)
de Broglie wavelength of an electron
The book is
not very clear about whether Feynman applying his stopwatch model to electrons,
but in the lecture he makes it clear he is. He shows an electron moving
between two points on a time-space diagram. He says the rate of turning
of the electron 'amplitude' (stopwatch) depends on the 'rest mass' of the
electron (no mention of velocity!), giving several contradictory values
(about) 10^20, 130 million million. Is this the de Broglie wavelength?
wavelength = h/p
= h/Mv
but
wavelength x freq = v
v/freq = h/Mv
freq = Mv^2/h
From above it's clear that both the electron momentum and its freq (rate of turning) depend on how fast the electron is moving. The frequency varies as Mv^2, which is its energy of motion (kinetic energy?). A stationary electron has zero frequency and an infinitely long wavelength. Let's see what the de Broglie frequency would be if an electron has energy of motion equal to its rest mass energy (0.511 Mev). My plot (far below) shows that at v = 0.707c relativistic mass (M = 1.41 m) so
Mv^2 = 1.41 m x (0.707 c)^2
= 0.707 m c^2
freq = Mv^2/h
= 0.707 m c^/h
= 0.707 x 9.1 x 10^-31 x 9 x 10^16/6.6 x 10^-34
= 8.77 x 10^19 hz
So Feynman in his lecture probably misspoke (he does this several times). He probably meant that an electron moving 'point to point' at a speed where its energy of motion equals its rest mass energy has a 'rate of turning' (about) 10^20 hz (agreeing with above).
Electron conclusion --- QED amplitude analysis uses the de Broglie wavelength of the electron (wavelength = h/Mv), and its 'rate of turning' depends on its kinetic energy (Mv^2).
What ? --- [Wikipedia -- de Broglie hypothesis] is not very clear but it does say "The second de Broglie equation relates the frequency of the wave associated to a particle to the total energy of the particle". (Well this is true at high speeds.) An (oddball) 1992 book on the electrons by H. Mac Gregor says de Broglie derived the equation for a stationary electron as freq = m c^2/h, which of course means E = rest mass energy.
Charge & mass
The
'ideal' electron (straight line in Feynman diagrams) takes a simple direct
path from a point to another in the space-time, and it is this ideal electron
that has parameters 'n' (mass) and 'j' (charge & coupling constant
to the photon). Late in book Feynman says while 'n' is the theoretical
electron mass, 'm' is the measured electron mass, likewise while 'j' is
the theoretical charge, 'e' is the observed (measured) charge.
In most of the book j is used for the theoretical coupling constant (emission or absorption) between photon and electron. Its value (j) is given only as approx -0.1, which is 1/10th vector length with a 180 degree 'arrow turn'. The observed coupling constant, e (electron charge) is the theoretical j with some real world correction terms, coming out experimentally to be about -0.08542455, the sqrt{1/137.035}, where (1/137.035) is the fine structure constant.
The loose end that keeps troubling me is how different are the theoretical values j and n from observable values e and m. In other words how big are these correction factors from ideal to real world. All that Feynman says in QED is that j is about -0.1 and e is measured to be -0.08542455. This probably means that the correction factor is no more than 15%, but it might be a lot less, because Feynman might just have been rounding -0.085 to -0.1 for simplicity. I searched and searched to find more on this and found only that the electron's "renormalized charge" (observable) is the "bare charge" (ideal) x sqrt{Z3}, and the lowest order Z3 = 1. (Ref: graduate level quantum physics book p245)
A justification for renormalization in QED is that it began to be underestood that the electrons' charge and mass in the equations did not actually correspond to the physical constants as measured in the laboratory, rather they were bare quantities that did not take into account the contribution of virtual-particle loop effects that come into play when the quantities are measured.
The picture drawn to explain the correction between (n, j) and (m, e) is that the electron is surrounded by a cloud of virtual charge particles that partially screens the electron. It appears that the vacuum correction factors include the real electron exchanging virtual photons with itself and that such photons may occasionally turn into electron/positron pairs, meaning the observed mass (m) depends on ideal charge (j), and observed charge (e) depends on ideal mass (n).
Decode --- As best as I can figure out, an approx perturbation calculation is done (using of course Feynman diagrams) relating ideal (n, j) to measured (m, e), and in this way (n, j) in the theory are adjusted based on measured values of (m, e). Apparently this 'calculation' between (n, j) to measured (m, e) involves the subtraction of infinities, which is renormalization. It was renormalization that allowed QED theory to calculate accurate probabilities.Misc QED
http://homepage.mac.com/stevepur/physics/matter/matter.3.html
-- According to Freeman Dyson, "Feynman regards the graph (Feynman diagram) as a picture of an actual process which is occurring physically in spacetime". Whereas Dyson considered them to be merely an aid to calculation, as "help in visualizing the formula which we derive rigorously from field theory". (from book, Drawing Theories Apart: The Dispersion of Feynman Diagrams in Postwar Physics by David Kaiser).
-- 'kernel' used in calculating an electron goes from point to point is a Green's function solution of the electron's equation of motion. The kernel for a free electron was obtained from the (relativistic) Dirac wave equation.
-- Renormalization --- Infinities disappeared if the observed finite electron mass and charge were associated not with the parameters m and e appearing in the Lagranian, but with electron m and e calculated from m and e taking into account that the electron and photon are always surrounded by a cloud of virtual photons and electron-positron pairs. (Weinburg Nobel lecture, 1979)
--Feynman diagrams consists of three subdiagrams: photon propagator, electron propagator, and the three pronged electron-photon vertex.
-- Calculations are possible with Feynman diagrams without even know anything about Green functions. The comment is 'anyone can do it' following the mechanical rules.
-- Feynman diagrams with loops photon self-eneergy diagrams, or vacuum polarization diagrams.
-- Probability amplitudes are squared to find probability.
-- An interaction between two electrons involves the exchange of (at least) one (virtual, not real) photon, so the fine structure constant (proportional to e^2) is associated with the probability of a quantum event occurring, the sqrt disappearing.
Brief history of wavefunction
* de Broglie
proposes (all) particles have a wave property (wavelength = h/p).
* Schrodinger treats de Broglie waves as sinewaves in the complex plane each having an amplitude and phase. The general form for his 'wavefunction' is psi = A e^i(kx -wt), where k is wavenumber = 2 pi/wavelength. By rewriting the classical equation for particle energy [E = T (kinetic) + V (potential)] using the de Broglie equation (wavelength = h/p) and Einstein's relationship (E = h frequency), he is able to write a differential equation for psi. A solution to the Schrodinger equation is called a wavefunction.
* A wavefunction is described in Schrodinger's equation by three properties (later Wolfgang Pauli added a fourth: spin). The three properties were (1) an "orbital" designation, indicating whether the particle wave is one that is closer to the nucleus with less energy or one that is farther from the nucleus with more energy, (2) the shape of the orbital, i.e., an indication that orbitals were not just spherical but other shapes, and (3) the inclination of the orbital, which determines the magnetic moment of the orbital around the z-axis. These three properties were called collectively the wavefunction of the electron and are said to describe the quantum state of the electron.
* Schrodinger has produced his equation mathematically and he doesn't really understand what his wavefunction (psi) represents. He thinks it might be a description of charge density, but this turns out to be wrong.
* Max Born proposes that Schrodinger wavefunction is to be interpreted as a probability amplitude, which statistically describes where within the volume occupied by the wave the particle is located. The square of the wavefunction (probability amplitude) when scaled by an incremental volume give the probability density of finding the particle in this volume. The function is normalized to make the total area under the probability density curve equal to one.
Schrodinger wave equation
There are
three (known) ways to mathematically describe a quantum mechanical system:
matrix mechanics, invented first (1925) by Werner Heisenberg; wave equation,
invented second (1925) Erwin Schrodinger; and path integral method, invented
last (1948) by Richard Feynman. The methodologies are all very different
and a problem easily solved by one method can be nearly intractable with
another method. All three methods have been shown to be mathematically
equivalent.
Matrix mechanics (as best as I can tell) is the physics equivalent of modern control theory. An opaque, black box procedure using matrixes that provides zero understanding of what is going on. You just crank the matrix multiplications and out pops the answer. There is no picture.
Solutions to Schrodinger's wave equation (sometimes called wave mechanics) detail where de Broglie 'matter' wave is and how it is spread out. It gives a 'fuzzy' picture that contains probability information as to where within the region of space occupied by the wave the particle will be detected. I suspect the Schrodinger wave method is the easiest to apply to a wide variety of problems, and thus is most widely used. The statistical wave uncertainty is (or includes) the Heisenberg uncertainty criteria.
Feynman's path integral method is (I assume) essentially the perturbation method that he details in his quasi-technical book QED. In Feynman's view this method provides a (sort of) physical picture of more likely (and less likely) electron and photon paths. (On the other hand Feynman's associate Freeman Dyson considered the path integral method to be purely a mathematical technique with no pictures attached.) I don't know what the currently accepted view is now. As Feynman makes clear in QED, there is no need to separately add in heisenberg uncertainty, it just falls out of the path integral analysis.
Simple, heuristic, derivation of Schrodinger's equation
A simple (heuristic)
derivation of the Schrodinger wave equation is found in the Wikipedia Schrodinger
article. It gives good insight as to how Schrodinger came up with the equation
and what it means. Here is the Schrodinger equation:
i hbar d/dt(psi) = - (1/2m) hbar^2 (d/dx)^2 (psi) + V psi
(Schrodinger eq)
where
(d/dx)^2
(psi) is 2nd derivative (with respect to x) of psi
or [(d/dx)^2 + (d/dy)^2 + (d/dz)^2] in three dimensions
The above equation is really nothing more than the classical (non-relativistic) equation for particle energy [E = T (kinetic) + V (potential)] rewritten substituting Einstein's and de Broglie's equations for energy and momentum. It's just Einstein's relationship (E = h frequency) and de Broglie equation (wavelength = h/p) applied to a sinewave in the complex plane (psi = Ae^i(kx -wt).
The energy of a particle (ignoring rest mass energy) is the sum of kinetic energy plus potential energy, but classically kinetic energy is just momentum squared divided by 2m, so energy is the sum of momentum squared (scaled by 1/2m) + potential energy.
E = T + V
where
E = total energy of a particle
T = kinetic energy
V = potential energy (varies with position (& maybe time))
psi = Ae^i(kx -wt)
sinewave as a complex number
d/dt(psi) = -iw psi
d/dx(psi) = ik psi
(d/dx)^2(psi) = -k^2 psi
2nd derivative (with respect to x)
E = h freq = hbar w
Einstein
E psi = hbar [w psi]
= hbar [i d/dt(psi)]
wavelength = h/p
de Broglie
p = h/wavelength = [h/(2 pi)]/[wavelength/(2 pi)]
p = hbar k
(k = wavenumber = (2 pi)/wavelength)
p^2 psi = (hbar k)^2 psi
= hrar^2 [k^2 psi]
= hbar^2 [-(d/dx)^2(psi)]
Scaling [E = T + V] by psi and plugging in (from above)
E psi = T psi + V psi
i hbar d/dt(psi)
= (p^2/2m) psi + V psi
i hbar d/dt(psi)
= - (1/2m) hbar^2 (d/dx)^2 (psi) + V psi
Above is the
time
dependent Schrodinger's equation. A time independent version
of the equation is created by not using Einstein's (E = h freq) and just
entering E (as a function of x), as shown below:
.
E psi = - (1/2m) hbar^2 (d/dx)^2 (psi) + V psi
Plugging plane wave (in cartesian form) into Schrodinger's
equation
Plugging in
the plane wave in cartesian form [psi = cos(kx - wt) + i sin(kx - wt)]
into Schrodinger's equation and separating real and imaginary parts we
get an interesting result.
real
i hbar (-i
w) cos( ) = - (1/2m) hbar^2 (-k^2) cos( ) + V cos( )
hbar w = (1/2m) hbar^2 (p^2/hbar^2) + V
hbar w = (1/2m) (p^2) +V
E = kinetic energy + potential energy
imaginary
i hbar (w) sin( ) = - (1/2m) hbar^2 i (-k^2) sin( ) + V i sin( )
hbar w = (1/2m) hbar^2 (p^2/hbar^2) + V
hbar w = (1/2m) (p^2) +V
E = kinetic energy + potential energy
The real and imaginary equations are both the same! And the equations are just saying quantum energy (E = h freq) equals the sum of the kinetic and potential energy.
Electron in an energy well
A lot of insight
into quantum systems and how particles 'fuzz' out can be gained by studying
a few simple idealize cases where the Schrodinger (time independent) wave
equation is fairly easily solved. A classic case is an electron trapped
in a one-dimensional energy well. Inside a one-dimensional (rectangular)
well V (potential energy) equals zero, whereas outside the well V = V0.
(I remember solving some of these quantum physics energy well problems
in school.)
E psi = - (1/2m) hbar^2 (d/dx)^2 (psi) + V(x) psi
(1/2m) hbar^2 (d/dx)^2 (psi) = [V(x) - E] psi
(d/dx)^2 (psi) = (2m/hbar^2) [V(x) - E] psi
where
(d/dx)^2 = 2nd derivative of x
(not 1st deriv of x squared)
V(x) = potential energy (as a function of x)
* E (energy) looks like an input, but it's really an output to be solved for. In general E = h x frequency, so solutions to the equation typically exist for quantized values of frequency and energy.
* The well has identical boundary conditions at both ends. The result is that the solutions to the equation for inside the well are sin and/or cos waves with integral number of cycles. The frequency comes out to be a function of the dimensions of the well and the energy, for example, cos w0, cos 2w0, cos 3w0, in other words the frequency is quantized.
* The boundary conditions for outside the well (V = V0) are (potentially) asymmetrical. At the well boundary the outside wave needs to be continuous (equal) to the inside wave, but outside the well the wave needs to die away, because of course the electron is (nominally) trapped inside the well. (The general rule is that for the wavefunction to represent a physical situation is that psi and the first derivative of psi must both be continuous across any barrier.) The result is that the solution to the equation is a simple exponential decay of the form e^-x/b, where 'b' is the spacial 'time constant'. It is, of course, the existence of exponential wave decays into the well potential barrier that explains how, when the barrier is narrow, an electron can occasionally escape the well by tunnelingthrough the barrier.
* The Schrodinger equation is linear. Mathematically this means superposition applies. If there is a series of solutions of the form cos w0, sinw0, cos 2w0, sin2w0 etc, then the complete solution is the sum of all of them. This is extremely important when the Schrodinger equation is applied to the atom. Here it is rewritten in spherical coordinates, broken apart into radial and two angular equations and solved for (V = k/r) and various energy levels. The sum of all these solutions gives us the well known electron orbits of chemistry (1s, 2px, 2py, 2pz, etc).
How much fuzz?
* So just
how is the exponential decay into the barrier (in essence the 'fuzz') related
to the wavelength (& frequency) of the electron inside the well? According
to my textbook the answer are (by 'time constant' I mean the [wavelength/2
pi]).
Cos/Sin wavelength
= (2 pi) hbar/sqrt{2mE}
= h/sqrt{2mE}
Barrier spacial 'time constant' =
hbar/sqrt{2m(V0 - E)}
where
hbar = h/2pi
De Broglie's equation for wavelength as the function of momentum (wavelength = h/p) can be rewritten in terms of energy since [E (kinetic) = (1/2) m v^2 = p^2/2m]:
Broglie wavelength = h/p
= h/sqrt{2mE}
So we end up
with the interesting relationships that the electron in the well has an
amplitude wavelength exactly equal to its de Broglie wavelength.
The decay distance ('fuzz') is closely related to the de Broglie wavelength
(in well), except it is (1/2 pi) smaller and varies with the potential
height of the barrier, the steeper the barrier the faster it goes to zero.
Note that if the barrier height is (V0 = 2 E) so that (in a sense) the
(kinetic) energy inside the barrier is the same as in the well, i.e. (V0
- E) = (2E - E) = E, then the spacial decay ('time constant') is just the
de Broglie wavelength /2 pi.
---------------------
Using the
uncertainty principle [delta pos > (hbar/2)/delta p] for a well of L length:
Lp = hbar/2
p = hbar/2L
wavelength = h/p
= h/(hbar/2L)
= 2hL/[h/2 pi]
= 4 pi L ????
I suspect there
are hidden standard deviation spectrums hidden in [delta pos > (hbar/2)/delta
p]. My textbook gives the relationship simply as [delta pos > (h)/delta
p]. Now everything works:
Lp = h
p = h/L
wavelength = h/p
= h/(h/L)
= L
(agrees with above)
----------------------
Standing wave
All time invariant
solutions to Schrodinger's equation, like for example an electron in a
well, can be considered (the probability amplitude of) a standing wave.
A standing wave is equivalent to the sum of two equal amplitude, traveling
waves (with the same frequency as the standing wave) that are traveling
in opposite directions. This is clearly shown in the neat gif image below
from Wikipedia (Standing Waves).
Note this means that in the case of an electron in a well, which appears to be time invariant, we can use de Broglie's formula for wavelength (wavelength = h/p). You can see in the figure that the traveling waves have the same wavelength as the standing wave. In the case of the infinite well the electron is moving at a constant speed, and as shown below this allows the energy to be easily calculated (agreeing with a direct solution of the wave equation as shown in French).
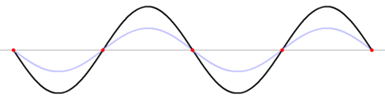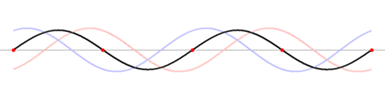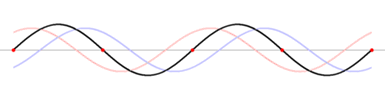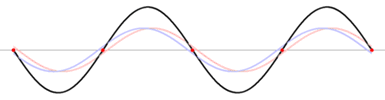
Standing Wave --black is sum of red and blue traveling
waves
(Wikipedia -Standing Wave)
Electron in a well
Well with infinite barrier height
An infinite
height barrier means zero probability of finding the electron outside the
well, so the wavefunction must be zero everywhere outside the well. Since
psi is a continuous function, this makes the boundary conditions inside
the well zero. The result is that the generic sinewave/exponential solutions
of the wave equation in this case come out to be an integer series of half
cycle sinewaves with periods (2L/n), where n = 1,2,3 etc (see fig below).
Lowest is 1/2 cycle, then 1 cycle, 1 1/2 cycle etc.
psi = sqrt{2/pi} sin (n pi/L)
where
n = 1,2,3

One full wavelength is twice the well length (2L), so de Broglie wavelength for the lowest energy is 2L. Higher frequencies (n = 2,3 etc below) have wavelengths = 2L/n, hence their momentum (wavelength = h/p) is
p = h/wavelength
= h/(2L/n)
= n h/2L = mv
With an expression for p we can find the kinetic energy for a particle in the nth quantum state in a well of length L with infinite barrier height.
E = 1/2 m v^2
= p^2/2m
= [n h/2L]^2/2m
= (nh)^2/(8mL^2)
agrees with French (p 116)
where
n = 1,2,3
Thus we see the velocity, momentum and energy are all quantized. Velocity (and momentum) increase linearly with 'quantum number n' and vary inversely with the size of the energy well. The de Broglie wavelength being twice the length of the well. With velocity going up as 'n' E (kinetic energy) goes up as n^2.
Well with finite barrier height
With a finite
barrier potential there is some penetration of the barrier, so the boundry
conditions sort of ride up on the sinewave (see fig below). The solutions
of the wave equation are now approximately an integrer series of half cycle
sinewaves (see fig below) but with a somewhat lower frequency than before.
This is analagous to the stretching out of the period of oscillation of
a resonant circuit as Q is lowered. There is now a series of standing waves
in the well with a wavelength (either exactly or approx) integrer related
and an exponential tail extending a little into the barrier.
Something that French notes about psi at the boundry is visible in the figure above. Psi has an inflection point at the boundry, i.e. it curves 'upward' in the barrier and curves 'downward' inside the well.
Barrier penetration
The wavefunction
inside the barrier has the form of a simple exponential. As a circuit designer
I find the best way to think about this is to analogize it to an electrical
time (tau), which is the time required for values in RC or LC circuits
to decay by 'one time constant', which is (1/e) = 37% of the initial value.
So I have written the psi inside the barrier in a slightly different form
than the physics use. Tau_x is the distance from the barrier where the
wavefunction exponential has decayed to (1/e) or 37% of its initial value.
psi (inside barrier) = A e^-x/tau_x
For the tau_x concept to be useful tau_x needs to be referenced to some distance, and the logical distance to reference it to is the de Broglie wavelength (wavelength = h/p) of solution at hand. In other words what we want is, "What fraction of a (de Broglie) wavelength is tau_x"? If this value is constant, then the solution is easily remembered.
For a finite barrier height the (great) Hyperphysics site gives the value of tau_x as
tau_x = hbar/sqrt{2m(U0 - E)}
French agrees
where
U0 = barrier height
E = energy
Note sqrt{2m x kinetic energy} = sqrt(2m x (1/2) mv^2} = squrt{p^2} = p, so tau_x has units of distance because it has units of h/p (same as de Broglie wavelength). By inspection the de Broglie wavelength of the lowest energy sinewave is twice the barrier length (2L)
check
wavelength = h/p
= h/sqrt{2mE}
= h/sqrt{2m (nh)^2/(8mL^2)}
where
n = 1
= 1/sqrt{1/4L^2}
= 2L
checks
If the barrier energy is twice the well energy (U0 = 2E), then the tau_x equation looks a lot like the equation for the de Broglie wavelength, except the numerator is hbar rather than h, so tau_x is (1/2 pi) x de Brolie wavelength (2L). For higher levels of U0 tau-x goes down approx as the square of U0, reducing by 1/3rd at U0 = 10E, and by about 100 for U0 = 10,000 E. If the barrier height is considerably larger than allowable electron energies, then tau_x, is independent of E and about the same for all the solutions. This is consistent with the Hyperphysics figure above which shows about the same absolute penetration into the barrier for n =3 as n = 1.
Bottom line --- tau_x (sort of) starts off at (1/2 pi) x de Broglie wavelength and then goes down as the square root of the barrier height.
Negative kinetic energy
I have noticed
an interesting way to interpret the equation for tau_x. The de Broglie
wavelength can be written as a function of kinetic energy.
wavelength = h/p = h/mv
= h/sqrt{2m(E_kinetic)}
where
E_kinetic = (1/2)mv^2
Inside a well the expression (E - V) represents the kinetic energy of the particle, where E represents the sum of kinetic energy and potential energy and is treated as a constant across the well dimension. In wells with a variable 'V' bottom the kinetic energy varies across the well. French points out (p 140) that the kinetic energy (E - V) is the key to sketching the wavefunction (psi) in the well. Where the kinetic energy is low (caused by high 'V'), the de Broglie wavelength is longer and the amplitude larger, larger because (in a sense) the particle is moving 'slower', and where the kinetic energy is high the de Broglie wavelength is shorter and the amplitude smaller. In other words a particle in a well with a variable potential energy (V) bottom acts much like a (classical) ball moving in a smooth well, as it moves its fixed energy continually shifts between kinetic and potential energy.
With above as background we can interpret our expression for tau_x.
tau_x = hbar/sqrt{2m (U0 - E)}
2 pi tau_x = h/sqrt{2m(U0 - E)}
Outside the well V = U0 and U0 > E, so outside the well and inside the barrier (E-V) is negative. Compare (2 pi tau_x) with the de Broglie wavelength formula written in terms of energy (above). Notice they are basically the same. So the distance the wave exponential 'tail' extends into the barrier can be consider to be (sort of) a de Broglie wavelength (divided by 2 pi) determined by the magnitude of the negative kinetic energy in this region.
Parabolic potential well ( V = k x^2) of a simple harmonic
oscillator
A well with
a potential (V) in it that rises from null as the square of distance is
the quantum equivalent of the the 2nd order resonant system like an LC
oscillator or spring/mass oscillator, what physicists call a 'harmonic
oscillator'. Its practical significance is that it is considered a good
model for vibration between atoms in molecules, especially two atom (diatomic)
molecules, and it applies too to the vibration of atoms in lattices and
the theory of heat capacity.
In a well where the restoring V (potential energy) is proportional to x^2 psi comes out to be a normal distribution (gaussian) centered in the well
psi = A e^-{x^2/2 c^2}
where
c = standard deviation
The lowest E (ground state energy) comes out to be
E = (1/2) h freq = (1/2) hbar w
where
V = (1/2) k x^2
freq = w/(2 pi ) = (1/2 pi) sqrt{k/m}
k = spring constant (equivalent) in a spring/mass oscillator
---------------------------------------------------------
Check that above is a solution to Schrodinger's equation
(1/2m) hbar^2 (d/dx)^2 (psi) = [V(x) - E] psi
(d/dx)^2 (psi) = (2m/hbar^2) [V(x) - E] psi
psi = A e^-{x^2/2 c^2}
(d/dx) (psi) = A(-2x/2 c^2)e^-{x^2/2 c^2}
(d/dx) (psi) = A(-x/c^2)e^-{x^2/2 c^2}
(d/dx)^2 (psi) = A(-x/c^2)^2e^-{x^2/2 c^2} - A(1/c^2)e^-{x^2/2 c^2}
(d/dx)^2 (psi) = (-x/c^2)^2 psi - (1/c^2) psi
[V(x) - E] psi = [(1/2) k x^2 - (1/2) h (1/2 pi) sqrt{k/m}] psi
equating
(-x/c^2)^2 - (1/c^2) = (2m/hbar^2) [(1/2) k x^2 - (1/2) h (1/2 pi)
sqrt{k/m}]
equating the square terms
x^2/c^4 = (2m/hbar^2) (1/2) k x^2
1/c^4 = (m/hbar^2) k
c^4 = hbar^2/mk
agrees with French
equating the linear terms
(1/c^2) = (2m/hbar^2) (1/2) h (1/2 pi) sqrt{k/m}
= (m/hbar^2) hbar sqrt{k/m}
= (m/hbar) sqrt{k/m}
= (1/hbar) sqrt{km}
c^2 = hbar/sqrt{km}
c^4 = hbar^2/km
checks
finding E
E = (1/2) h freq
= (1/2) h (1/2 pi) sqrt{k/m}
= (1/2) hbar w
agrees with French
where
w = sqrt{k/m}
-------------------------------------------
The hyperphysics
site emphasizes that the minimum energy is not zero. In other words in
this quantum system there is no damping and particles always oscillate,
saying it is sometimes called "zero point vibration". French comments that
zero point energy is "clearly a completely non-classical phenomenon." A
typical 'zero point' freq for diatomic molecules is up around 10^13 hz.
French describes how Mulliken in 1924 (before the quantum theory was developed)
had been able to figure out using spectroscopic data from diatomic gases
that a zero point energy of [(1/2) h freq] must exist. Quite remarkable,
he explains, because zero-point energy can never be radiated! Hyperphysics
adds (assuming the model applies!) that zero point energy must mean that
atoms in lattices and gases must vibrate even at absolute zero.
A classic example (says Hyperphysics) of spectroscopic data (for diatomic) molecules is below. It shows resonances due to molecule rotations and vibration. The double spike (at the top) is a slight frequency difference due to the fact that the chlorine in the HCl is a mixture of two isotopes (C35, C37). Data like this is used to figure bond lengths and the molecular spring constant for molecules. (Hydrogen and chlorine bond by covalent sharing of one electron. Since chlorine is x35 time heavier than hydrogen, in vibration mode the single proton of hydrogen does almost all of the moving!)
HCl diatomic gas spectrum (hyperphysics site)
missing line at 8.66 x 10^13 hz is vibration energy
many closely spaced line are quantized rotational
energy
In this type of well energy goes as [E = (1/2 + n) h freq], where n = 0,1,2,3, so energy rises (after the zero point) linearly with n, whereas in simple wells with V = 0 in the well energy rises as n^2. French comments that the 'equal spacing' of energy levels is a unique property of the quantum harmonic oscillator. (Certainly the energy levels in simple wells and in the hydrogen atom go as n^2.)
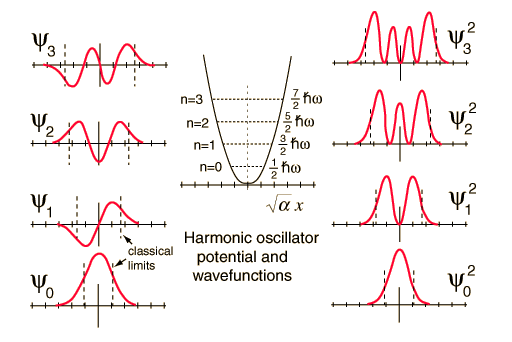
Wave solutions of x^2 quantum oscillator (hyperphysics
site)
[E = (1/2 + n) h freq]
[V = (1/2)wx^2]
An explanation (of sorts) for why the energy function has a '(1/2)' is revealed in the nicely drawn figure (above) from Hyperphysics. Note the ground state the probability density peaks at the bottom of the well, meaning (in a sense) that the atoms while vibrating move 'slowly' near null and 'rapidly' away from null. Whereas in all the higher energy modes, it's the opposite. The curves show oscillations like other wells, but the probability density peaks out near the 'edges' or up on the V curve, which is where the potential energy is high and the kinetic energy is low. This is more like a classical oscillator, for example a ball oscillating in a smooth well. The ball spends more time up on the sides, where it turns around, and less time in the center, where it is moving fast.
Derivation from Heisenberg uncertainty
Hyperphysics
shows that the zero point (vibration) energy of the quantum oscillaor can
be derived (exactly) from Heisenberg [(delta p) (delta x) > hbar/2].
E = kinetic E + potential V
= p^2/2m + (1/2) mw^2 x^2
substitute for p = delta p and x = delta x
E = (delta p)^2/2m + (1/2) mw^2 (delta x)^2
but from Heisenberg (minimum) delta p = (hbar/2)/delta x, so
E = [(hbar/2)^2/2m] (delta x)^-2 + (1/2) mw^2 (delta x)^2
find the minimum E by taking the derivative and equate to zero
0 = [(hbar/2)^2/2m](-2) (delta x)^-3 + mw^2 (delta x)
[hbar^2/4m] (delta x)^-3 = mw^2 (delta x)
hbar^2/4m = mw^2 (delta x)^4
hbar^2/4m^2w^2 = (delta x)^4
hbar/2mw = (delta x)^2
delta x = sqrt{hbar/2mw}
substitute (delta x) back into E
E = [(hbar/2)^2/2m] (delta x)^-2 + (1/2) mw^2 (delta x)^2
= [(hbar/2)^2/2m] /(hbar/2mw) + (1/2) mw^2 (hbar/2mw)
= hbar 2mw/8m + hbar mw (1/4m)
= hbar w/4 + hbar w/4
= (1/2) hbar w = (1/2) h freq
checks
-------------------------------
Electron wavefunction in hydrogen
The wavefunction,
which is the solution to the Schrodinger wave equation, basically just
details how the de Broglie wave fuzzes out. I was surprised to find that
the for an electron in orbit around a proton (hydrogen atom) the wavefunction
is extremely simple. It is just a simple decaying exponential, where 'a'
is the Bohr radius. (Note in circuit terms 'a' is the equivalent to a 'time
constant'.) (Technically this amplitude function is the simplest case (n=1,
l=0), which results in a spherically symmetric distribution.)
psi = A e^-(r/a)
where a = Bohr radius
(magnitude) psi^2 is probability density. Integration of probability density (psi^2) over a volume gives probability of finding the electron within this volume region of the 'matter' or de Broglie wave (per unit time).
Why r^2 x psi^2?Area of sphere = 4 pi r^2
One way to look at the volume integral is this. Psi^2 gives (sort of) an ideal radial probability density, which peaks in the center, but there's almost zero volume near the center. What we really want to know is at what radius are we likely to find the electron, so we need to scale psi^2 by r^2, because the volume of a thin spherical shell varies with r^2. Hence the hydrogen electron probability distribution we normally see is r^2 x psi^2.
Probability of finding the electron within a thin spherical shell (P(r), where (delta r) is the thickness of the shell, is
P(r) = (area of sphere) x (psi^2)
= 4 pi r^2 x A^2 e^-(2r/a)
= 4 pi A^2 [ r^2 x e^-(2r/a)]
Obviously integral {4 pi A^2 [ r^2 x e^-(2r/a)](delta r)} =1 with limits r = 0 to infinity.
taking the derivative to find the peak
d[ r^2 x e^-(2r/a)] = 2r x e^-(2r/a) + r^2 x (-2/a) e^-(2r/a) = 0
(2/a) r^2 x e^-(2r/a) = 2r x e^-(2r/a)
r = a
trying few values
r = a
a^2 x e^-2
r =2 a
4a^2 x e^-4 = 4e^-2 [a^2 x e^-2]
= 0.54 [a^2 x e^-2]
r = 3a
9a^2 x e^-6 = 9e^-4 [a^2 x e^-2]
= 0.165 [a^2 x e^-2]
r = a/2 0.25 a^2 x e^-1 = 0.25 e^+1
[a^2 x e^-2]
= 0.68 [a^2 x e^-2]
Yes, consistent with plot below, which is the classic radial density function of the (inner) Bohr orbit !
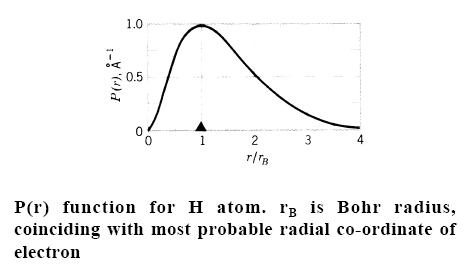
(source -- http://www.phys.unsw.edu.au/COURSES/FIRST_YEAR/pdf%20files/LecNotesWeek1.pdf
Using the CRC tables we can do the integration of a function [ r^2 x e^-(2r/a)] dr
Integral{r^2
e^br dr} = (r^2 e^br) /b - (2/b) Integral{r e^br dr}
= (r^2 e^br) /b - (2/b) ((e^br)/b^2)(br-1)
where
b = -(2/a)
@ r=infinity
= (r^2 e^-2r/a) /b - (2/b) [e^-(2r/a)/b^2](br-1)
= 0
@ r=0
= (0) - (2/(-2/a) [1/(-2/a)^2](0 - 1)
= - (a) [a^(2)/4]
= - (1/4) a^3
Complete integral (r=0 to infinity) = 1
= 4 pi A^2 [0 - (- (1/4) a^3)]
= pi A^2 a^3 = 1
so
A = sqrt{1/pi a^3}
making the wavefunction
psi = A e^-(r/a)
where
A = sqrt{1/pi a^3}
Yup, I checked two textbooks and
this is correct!
At the peak (r = a) the probability density is
4 pi A^2 [a^2 x e^-2]
4 pi (1/pi a^3) [a^2 x e^-2]
(4/a) e^-2
0.54 a
For a 'rectangular' probability pulse with this height the width would be (delta) r = 1.85a, or in absolute terms r = a +/- 0.92a (or r = .075a to 1.925a ), which is reasonable.
Interestingly a physic's guy who wrote online electron orbit applets found that the complete, complex, normalization factor (A) in three text books was not right, and he had to derive his own value.Check psi = A e^-(r/a) satisfies Schrodinger's equation
i hbar d/dt(psi) = - (1/2m) hbar^2 (d/dx)^2 (psi) + V psi Schrodinger's equation
psi = A e^-(r/a)
d/dt(psi) = 0
(d/dr)^2 (psi) = (1/a^2) A e^-(r/a)
0 = - (1/2m) hbar^2 (1/a^2) A e^-(r/a) - (K/r) A e^-(r/a) does'nt check
Whoops, I had guessed I could just plug in 'r' for 'x' in the divergence, but that's not right. The equation needs to rewritten in spherical coordinates and the radial terms separated out to solve for the radius.
I found it's just too hard to learn quantum mechanics (totally) from the web, so I bought a relatively inexpensive ($40 new) MIT quantum mechanics textbook: An Introduction to Quantum Physics, by French and Taylor (1978). It's probably cheap since it was first published 30 years ago, but its still an assigned book for some MIT physics course.My textbook gives the radial Schrodinger equation in a curious format (below). They show that by defining a new variable (r x psi), the radial (time invarient) Schrodinger equation can be put in the same form as the Schrodinger equation in x. The radial wavefunction is then the new variable divided by r.
E (r psi) = -(1/2m) hbar^2 (d/dr)^2 (r psi) + V(r) (r psi)
compare with (from above)
E psi = - (1/2m) hbar^2 (d/dx)^2 (psi) + V psi
----------------------------
(needs work)
Let's check if psi = A e^-(r/a) satisfies the radial Schrodinger equation with the potential energy similiar to the potential energy of a planet in orbit: V = - K/r
E (r psi) = -(1/2m) hbar^2 (d/dr)^2 (r psi) + V(r) (r psi)
r psi = r A e^-(r/a)
(d/dr) (r psi) = A e^-(r/a) +r (-1/a) A e^-(r/a)
if 2nd derivative
(d/dr)^2 (r psi) = (-
1/a) A e^-(r/a) + (-1/a) A e^-(r/a) + r (1/a)^2 A e^-(r/a)
= (-2/a) A e^-(r/a) + r (1/a)^2 A e^-(r/a) doesn't
look too promising
----------------------------
Wavefunction of a electron vs time
Wavepackets
I've long
been puzzled by wavepackets. I couldn't figure out what there were or where
they fit in. In other words, What the hell are they? I think I now
have a handle on the basics.
Suppose the location (at time t = 0) of a free electron (or any particle) is described by a rectangular probability distribution of width L. In other words the electron has equal proabibility of being anywhere within L. That means that psi, the wavefunction @ (t = t0), is rectangular too, because squared it gives a flat probability distribution. All psi are solutions of Schrodinger's equation, which basically means sinewaves and exponentials.
This is basically just a frequency analysis problem, the type of problem that electrical engineers study extensively. Psi is the equivalent of a time waveform, and its frequency components are the solutions of the wavefunction! It's really that simple.
The solution of the rectangular psi (eq time waveform) is a series of closely spaced frequencies within an envelope (sin x/x). The range of frequencies having a period clustering around the width of the rectangular pulse. As the rectangular pulse width narrows approaching an impulse the frequency ranges widens toward a flat frequency range. It's common to use a gausian for the pulse shape of psi because a gausian has the nice feature that its fourier transform, i.e. its frequency components, is also a a gausian.
Key concepts
To localize
an electron at a given time means the electron's wavefunction at
that time is a range of closely spaced frequencies. This is the
so-called 'wave packet'. Each of these frequencies travels at a slightly
different rate (haven't figure out the details of this yet), which means
over time the wavefunction spreads out spacially, it disperses.
This means that over time the position of the electron becomes more and
more uncertain! This spreading of the wavefunction with time is shown for
a gaussian pulse below.
Dispersion
(I think) is pretty easily explained from the Heisenberg uncertainty principle.
An electron known at t =0 to be in a spacial range (delta x) has a corresponding
uncertainty range of momentum (delta p = h/delta x). Hence over time
the uncertainty in velocity (delta v = delta p/m) translates into an a
wider range of positions.
----------------------------------------------
Following
are notes on gausian wave packets from public online physics courses
by Prof. Jess H. Brewer,
Dept. of Physics & Astronomy, Univ. of British Columbia,
Vancouver, B.C., Canada
http://musr.physics.ubc.ca/~jess/p200/gwp/gwp.html
gausian wave of a stationary 'particle' spreading
over time
(source prof Jess Brewer @ Univ of Britsh Columbia)
gausian envelope of a moving 'particle' wave spreading
over time showing de Broglie 'ripples' in real component
(not shown are quadrature 'ripples' in complex component)
(source prof Jess Brewer @ Univ of Britsh Columbia)
-- The center of a wave packet with a finite ko moves with time at the group velocity, as expected for the mean position of a particle. It simultaneously broadens just like the (on average) stationary particle; this must be so in order to preserve Galilean invariance, which is still applicable as long as the velocities are nonrelativistic.
-- What do the 'wiggles' represent? When the particle is (on average) at rest, its wave packet is just a 'bump'' (spacial probability amplitude shape) that spreads out with time; when it is moving, it acquires all these oscillations of phase with a wavelength satisfying de Broglie's formula (wavelength = h/p).
** -- Is it really 'there' at the peaks and 'not there' at the points where the function crosses the axis? No. Except for the overall 'envelope' it is just as ``there'' at one point as at another. This is a direct consequence of using the complex exponential form (rather than a cosine) for the traveling wave. Although the plots above show only the real part, there is an imaginary part that is a maximum when the real part is zero and vice versa so that the absolute magnitude is always (except for the overall 'envelope') the same.
-- In that case, what is the point of even having these 'wiggles?' Well, although no experiment can measure the absolute phase of a wavefunction, the relative phase of two probability amplitudes being added together is what causes interference, which is the key to all observable quantum mechanical phenomena.
-- By adding
together two traveling waves propagating in opposite directions it is possible
to make a standing wave, whose wavefunction really is a real oscillatory
function for which the particle is actually never found at positions where
the amplitude is zero.
-----------------------------------
Waves --- photons vs electrons
Photons and
electrons (particles) both have 'waves' whose vector amplitude squared
is a probability density, but there are lots of difference between the
waves of a photon and electron.
Frequency
The frequency
of electron's wave depends (entirely) on the electron's motion,
whereas the frequency of a photon's wave depends (entirely) on the frequency
of the photon's source, which typically is a vibrating electron.
The frequency of a photon, which is proportional to its energy since (E
= h freq) is exactly the same as the frequency of the source, whether
this is electrons in a radio antenna oscillating up and down, or the synchronized
laser emission as electrons in atoms change orbits.
In any traveling wave [frequency x wavelength = velocity]. All photons travel at the same speed, which in vacuum is speed of light (c), so the wavelength of a photon is the speed of light divided by the frequency of the source [wavelength = c/frequency].
The de Broglie wave of an electron moves with the electron, so if the electron is moving at velocity (v), then 'v' is the velocity of the wave. The electron's de Broglie wavelength is equal to planck's constant divided by momentum (wavelength = h/p). The electron's de Broglie wave frequency is the wave speed 'v' divided by wavelength. Substituting for wavelength we find that that the electron's wave frequency is proportional to the electron's kinetic energy.
frequency = v/wavelength
= v/(h/p)
= vp/h
= mv^2/h
= 2E_kinetic energy/h
Quadrature vs in-phase
Electrons
have de Broglie waves that oscillate in quadrature in the complex
plane in the form of a real cosine and a sine wave on the imaginary axis.
Ae^i(theta) = A cos(theta) +i A sin(theta)
The figure (above from Prof Brewer's site) that shows de Broglie 'ripples' within the moving electron's wave packet envelope. Brewer notes that in addition to the (real) ripples shown in the figure there are similar quadrature ripples on the complex 'i' axis (not shown). For a moving waveform (theta = w time) so one wave is cos(w time) and the other is sin (w time), so clearly the electron's de Broglie waves are in time quadrature.
By analogy with de Broglie waves for electrons it would be logical to think that photons also have two wave components and that they are in time quadrature. Wrong! The photon has two wave components, but they are not in time quadrature; they are, however, in spacial quadrataure.
The photon's probability amplitude wave is the electric field vector (E field), which is analogous to the (real) cos wave of the (moving) electron. All electromagnetic traveling waves have a 2nd wave component that travels with the electric field (E field) known as the magnetic field (H field). The H field is in quadrature with the E field spacially, but only spacially, not in time. An electromagnetic wave is a transverse wave, meaning the E and H vectors are perpendicular to the direction of travel, but they are also perpendicular to each other (hence spacial quadrature).
As viewed from the side, the wave appears to be a
fixed E,H pattern that moves through space
As viewed from a single location in space (on the
Z axis), E and H are seen to pulsate (in-time phase) as the wave moves
by
(The orientation of E determines the polarization
of the light (& photons))
I had always assumed that in a traveling plane wave the H field was in time quadrature with the E field, because electrical engineers know that a changing electric field makes a magnetic field and a changing magnetic field makes an electric field. (Even Feynman talks poetically about E and H chasing each other's tails as light propagates.) But in fact the E and H vectors oscillate up/down in time phase (though some nitwits on the web refuse to accept this). Feynman's Lectures on Physics have a nice simple proof of the in-phase character of E and H, which (in fig below) I apply to the propagation of a wavefront in a cable. (I have written up both of these aspects of light in some detail in my Speed of Light essay.)
I swapped several emails with a guy online who claims the "textbooks are wrong" about E and H being in time phase. He argues thay have to be in time-quadrature, because only then is the energy content of the wave constant in time (based on the trig identity sin^2 + cos^2 =1).My argument to him was that if an electromagnetic wave is hitting you, you do indeed sense an energy pulsation (through a cycle). The reason that the energy received pulsates is that the energy sent (from the source) pulsates. This is most easily seen at radio frequencies where the product of voltage and current fed to electrons oscillating up and down in a radio antenna can be seen to have pulsations. When E and H of the wave leaving the antenna go through null, at those instants no power is coming off the antenna, no power is being sent.
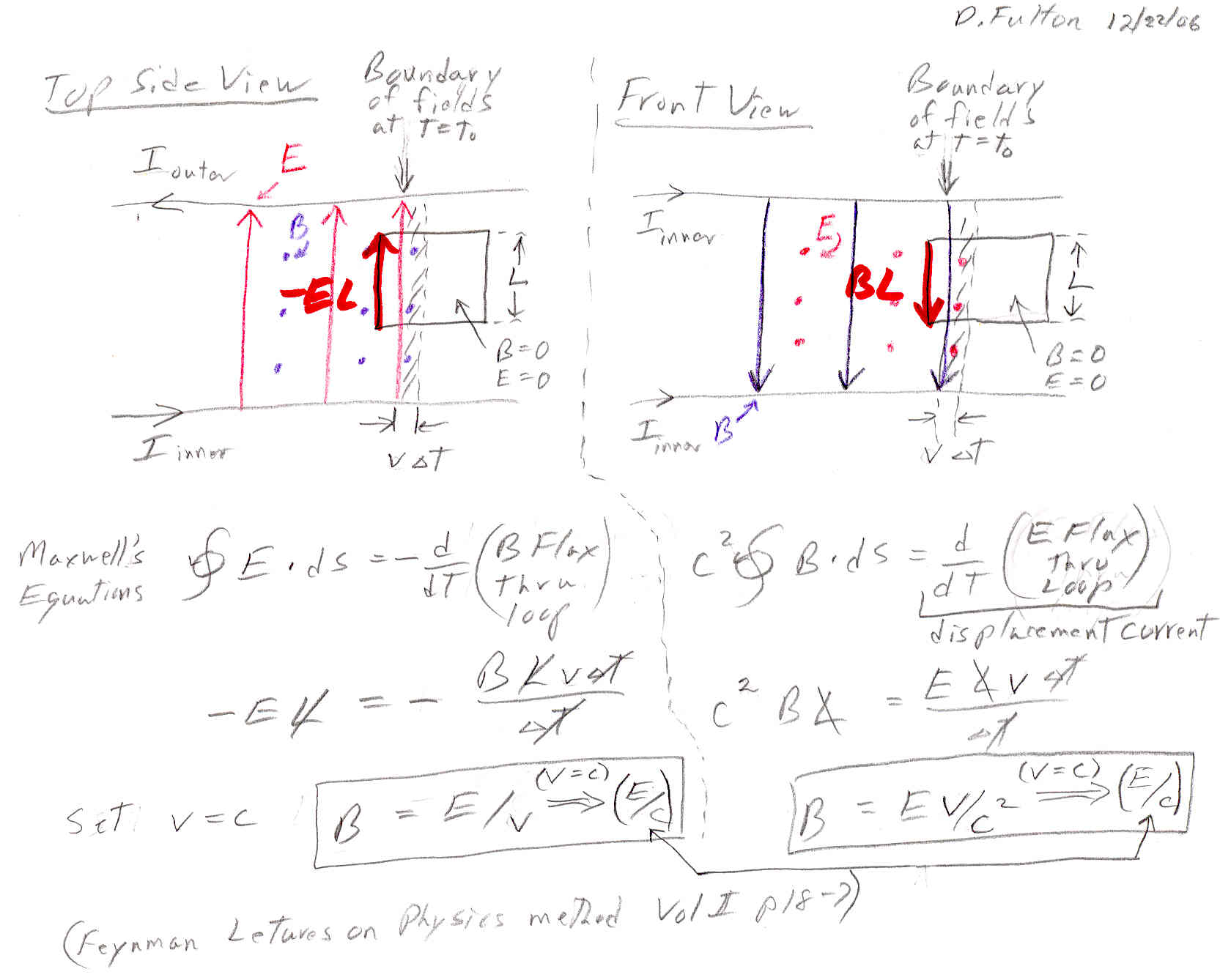
Quantum things
generally 'fuzz out' about one wavelength. This is true for light too as
can be seen by the fact that passing light through a slit narrowed to about
a wavelength (wide) causes a strong diffraction pattern to develop. The
implication is that the 'width' of the traveling photon must be the wavelength
of the source, and the 'logical' length (in direction of travel) for a
photon is the source wavelength. This means the E and H field energies
occupy a region of space about a wavelength cubed.
Model
E = qE distance. If we assume distance can't be more than 10-10 m atomic
dimensions, then we can find that E must be 10^10 volts/meter or so to
ionize a molecule (deliver a few ev). With the assumption that a photon
is the field energy of one wavelength (this could be wrong), then the photon
field energy must not be even over the wavelength cubed volume. Assuming
it is distributed evenly along the direction of travel, then the E field
value (to reach 10^10 volt/m) must be concentrated tightly (within 1% wavelength)
along line of travel.
Already a constradiction .... It must be spread out sideways by a wavelength or so to diffract, but it needs to be concentrated to classically force an electron. Do we get out of this by using quantum mechanics to figure the photon electron interaction?? Prehaps the picture is that the field energy is uncertain (what do we get with Heisenberg?) and statistically it sometimes falls into a narrow channel such that it can force an electron in atom. Is it clue that the e Feynman uses is about 1/10, which when squared give 1%??
Heisenberg for photon p x = (1/10) h
mc^2 = E, so p = E/c
x = 6.6 x 10^-35/(E/c)
= 6.6 x 10^-35 x 3 x 10^8/5 x 10^-19
= 4 x 10^-8
promising 1/10 wavelength. (I don't know how to figure Heisenberg for three
dimensiuons)
** The physicists guys say E = pc, so if light is really
monochromatic and pure (just a wave train) then p is so tightly known that
delta x (position of the photon) is very unknown >> a wavelength. So this
argument says statistically an electron is getting energy from many cycles,
Anothre contradiction, what then quantized energy!!!
----------------------------------------
Blue light (wavelength = 400 nm)
photon energy
= h freq
= h(c/wavelength)
= 6.6 x 10^-34 j-s x 3 x 10^8 m/sec/4 x 10^-7 m
= 5 x 10^-19 joule x [1 ev/1.6 x 10^-19 joule]
= 3.1 ev
So E,H field density (1/2 e0E^2 +1/2 u0 H^2) is energy/volume
= [h(c/wavelength)]/ wavelength^3
= hc/wavelength^4
= 6.6 x 10^-34 j-s x 3 x 10^8/(4 x 10^-7 m)^4
=7.7 joule/m^3 or 1,900 joule/m if eneregy in (wavelength/2 pi)^3
half the field energy is in the electric field and half in the magnetic field.
(1/2) [(1/2) e0 E^2] = hc/wavelength^4
= hc/[c^4/ frequency^4]
= (h/c^3) x frequency^4
if you double the frequency, the photon has double energy, but the wavelength 'box' has 1/8th volume, so the energy density must go up by 16, hence E varies as freq^2.
E^2 = [7.7 joule/m^3]4 e0
= 7.7/ [4 x 8.8 x 10^-12]
= 22 x 10^10
E = 4.7 x 10^5 volts/meter (av) or 1.16 x 10^8 volts/meter
time one wavelength takes to arrive (1/frequency)
time to arrive = wavelength/c
= 4 x 10^-7/3 x 10^8
1.33 x 10^-15 sec
if one photon grabs and shakes on electron in an atom, the work done on electron is
work = (force x vel) x time
= force x c x (wavelength/c)
= force x wavelength
= q E wavelength
= 1.6 x 10^-19 x 4.7 x 10^5 x 4 x 10^-7 m
= 0.30 x 10^-19
Lets work the E field for a 3.1 ev photon to deliver all its energy into an electron in an atom. I'sl asssume the atom can be moved an atomic dimension 10^-10 m
energy = force x distance
force = q E = energy/distance
E = energy/distance x q
E = 5 x 10^-19 j/ 10^-10 m x 1.6 x 10^-19 coulomb
E = 3 x 10^10 volts/meter
-------------------------------------------------
Working some #'s for a 1 Mhz AM radio wave
photon energy
h freq = 6.6x 10^-34 joule-sec x 10^6 hz
= 6.6 x 10^-28 joule
power received
1 uw = 10^-12 joule/10^-6 sec
energy received/cycle
10^-12 joule
photons/cycle
10^-12 joule per cycle/6.6 x 10^-28 joule/photon
= 1.5 x 10^15
wavelength
= c/frequency
= 3 x 10^8/10^6
= 300 m
how much enerergy is a visual photon at atomic size (10^-10
m) square
wavelength (400 blue to 700 nm red)
freq = 3 x 10^8 m/sec/7 x 10^-7 m
= 3 x 10^15 hz (red)
E in red photon = h freq
= 6.6 x 10^-34 j-sec x 3 x 10^15 hz
= 2 x 10^-18 joule
how long does it take one wavelength to pass by?
time = 1/3 x 10^15 hz
= 3 x 10^-16 sec
assume one cycle delivers one photon (10^-18 joules comes
in in 10^-16 sec)
how big is E if one photon is the energy contained in
a parallelogram the cross section of an atoms x wavelegth long.. Half energy
density is in E and half in H.
(1/2) e0 E^2 x (10^-10 m)^2 x 10^-7 m = 2 x 10^-18 joule
e0 E^2 x 10^-27 = 4 x 10^-18
8.8 x 10^-12 E^2 x 10^-27 =
E^2 = 4 x 10^-18/8.8 x 10-39
= 5 x 10^20
E = 10^10 volts/meter
there is simple rational for this. visible light is few
ev, so one volt needs to be delivered to 10^-10 meter requiring an E field
of 10^10.
force = qE
energy = force x distance
= q E 10^-10 m
= 1.6 x 10^-19 x 10^10 m x 10^-10
= 1.6 x 10^-19 joule vs 2 x 10^-18 joule
OK check order of mag
This view translates into E amplitude going up linearly with freq. Say at x10 freq energy per photon is up by 10, then E^2 density up x100 and volume is down by 10, so energy per cycle is up by 10 consistent with one photon per cycle.
Summary
One photon/cycle.
E amplitude varies linearly with freq
visual photons deliver about 1 voltage of work/energy to the electron in
a 10^-10 atoms size
so their E field is about 10^10 volt/meter
-------------------------------
(needs work)
Phase velocity
The velocity
of individual wave solutions to Schordinger's equation, called the phase
velocity, are easy to figure. The explanation given for the dispersion
of the wave packet is that the (slighly) different frequencies that make
it up have slighly differnent velocities.
Velocity in general is the product of frequency x wavelength and that is the case here.
v phase = freq x wavelength
but
E = h x freq
wavelength = h/p
so
v phase = E/h x h/p
= E/p
------------------------------------------
-- It is instructive
to estimate the rate of dispersion (how fast the wave packet spreads
out). First consider an electron that is initially confined to a region
(delta x = 10^-10 m) (roughly atomic dimensions) in a gaussian wave packet.
For simplicity we will let ko = 0; i.e. the electron is (on average) at
rest. If the electron is free, then its wave packet will expand to sqrt{2}
times its initial size in
(2m/hbar) x (delta x)^2 = 2 x 9.1 x 10^-31/1.05 x 10^-34 x (10^-10 m)^2
= 1.72 x 10^-16 sec (for 10^-10 m)
and
17 nsec from one micron (10^-6
m)
17 msec from 1 mm (10^-3 m).
----------------------------------------------
from an oddball paper that references Brewer
gaussian st dev (t) = sqrt{hbar t/m}
check against above
17 nsec
= sqrt{1.055 x 10^-34 x 17 x 10^-9/ 9.1 x 10^-31}
= sqrt{1.97 x 10^-12}
= 1.41 x 10^-6
17 msec
= sqrt{1.055 x 10^-34 x 17 x 10^-3/ 9.1 x 10^-31}
= sqrt{1.97 x 10^-6}
= 1.41 x 10^-3
------------------------------------------------
k = 2 pi/wavelength = 2 pi/[h/p] = p/hbar
for photons
E = cp
p = E/c
for electrons
E = (1/2) m v^2
(non-relativisti kinetic energy)
= (1/2m) (mv)^2
= (1/2m) p^2
p = sqrt{2mE}
-- The big
picture: for a free electron (i.e. in the presence of no external potential)
the wave function is given by the integral because it is a sum of different
momentum modes. (Alternatively you can Fourier transform and say it is
a sum of position modes.)
-----------------------------------------------------------
Basics of wavefunction
For the electron
there are two classic cases. One, a free electron, which has no force on
it represented by zero potential energy (V = 0). Two, an electron in an
atom attracted toward the nucleus by coulomb force represented by potential
energy that varies inversely with radius (V = k/r).
Free electron
In this case
there are no boundary conditions on the wave equation so the wavefunction
is time varying. If the electron is known to be in some region of
space at t = 0, then as time goes on the region where the electron may
be found expands as sqrt{t}. Draw a wavefunction at time zero [psi(x) at
t = 0], then as a function of time it expands. If the electron is stationary,
then the wavefunction just broadens in all directions.
Moving free electron
If the electron
is moving at t = 0, then the wavefunction has de Broglie frequency 'ripples'
enclosed in an envelope similar to a stationary electron. Over time the
waveform envelope broadens exactly the same as for a stationary electrron.
In other words the uncertainty range increases centered around an average
velocity.
Dispersion
Basically this 'dispersion'
of the wavefunction can be explained by the Heisenbery uncertainty principle.expansion.
The electron confined to a delta x range has uncertainty in its momentum
and velocity inversely proportional to the spacial range. It is (I think)
the uncertainty in the velocity of the electron at t = 0 that over time
causes the spacial range over which it may be found to expand.
Fourier transform
To an electrical
engineer a fourier transforms is a way to break down a waveform in timeinto
a bunch of sinewaves in frequency or (1/time). Any time waveform
can (in principle) be produced by adding a series of sinewave of the correct
frequency, amplitude and phase. Fourier transforms play a similar role
in quantum mechanics, but with space replacing time and wavenumber (1/space)
replacing frequency.
A fourier transform is a way to break down a probability amplitude waveform in space [psi (x)] into a bunch of sinewaves in wavenumber, which in units is the inverse of space. This is the basis of the wavepacket. If you define a region of space where a particle is expected to be found, say by drawing wavefunction shape on the x axis [psi(x)], then its fourier transform are sinewaves in wavenumber. These sinewave in wavenumber are solutions to the Schrodinger wave equation and are called a wave packet. For a free particle both the spacial psi wavefunction and its spacial fourier transformer change with time.
If psi(x) is an impulse, then its fourier transform
is flat.
Translation
--- If a particle location known, then its wavepacket is all wavenumber
(& all wavelenghts) from very low to very high. Wavenumber (k) is proportional
to momentum (k = 2 pi/wavelength = 2 pi/(h/p) = p/hbar), so it looks like
the wide range in momentum (& velocity) from the wave equation is just
what Heisenberg uncertainty requires. I haven't fully decoded phase
velocity yet, but my guess is that the (phase) velocity depends linearly
on wavenumber, which is basically momentum.
If psi(x) is flat, then its fourier transform is an
impulse
Translation
--- If a particle can be anywhere, then the solution to its wave equation
is just a single spacial sinewave.
If psi(x) is rectangular, then its fourier transform
has the shape sin (x)/x
Translation
--- If rectangular width x = b, then the fourier transform is sin(kb/2)/(k/2)
(French page335). (French shows the fourier transform plotted vs k, where
k is wavenumber = 2 pi/wavelength.) The width of the big fourier lobe is
found (he says) by setting kb/2 = pi, where k is to be interpreted as the
range of wavenumber (or range of momentum). k varies from zero to:
kb/2 = pi
so
k = 2 pi/b
but k = 2 pi/wavelength and b = delta x, so
2 pi/wavelength = 2pi/delta x
(longest) wavelength = delta x
Interesting result. The longest wavelength is (approx) the width of the psi wavefunction (in x), and the wavenumber varies from zero to approx 2 pi x (1/delta x). As French show this result can be compared to Heisenberg by substituting wavelength = h/p, where here p is really delta p, since wavelength is delta x and smaller values..
h/ delta p = delta x
delta x deltap = h
This exceeeds the Heisenberg limit by a little, so is
OK.
-------------------------------------
(from French)
dispersion
goes as
w = E/hbar
E turns out to be kinetic energy (1/2 m v^2)
= p^2/(2m hbar)
= hbar k^2/2m
OK
where k = 2 pi/wavelength and wavelength = h/p
so k = 2 pi p/h = p/hbar
so phase increases as k^2, or faster for high (momentum) frequencies
group vel = dw/dk = 2 hbar k/2m = hbar k/m = p/m = v
Note above
shows velocity is proportional to k (momentum). French says the 'group
velocity' is higher for higher (k) frequencies and lower for lower (k)
frequencies, which causes the dispersion of the wave packet. So from French
it is pretty clear that each 'k' frequency of the wavefunction is traveling
at a velocity that is proportional to its k, which is (in a sense) its
'momentum'.
--------------------------------
Particle dispersion time
To find how
a particle 'spreads out' with time its wave packet amplitude [psi(x)] is
often assumed to be gaussian. This makes its fourier transform (momentum
distribution), whose frequencies are the solutions to the wave equation,
also a gaussian, and the wave equation can be solved exactly. French starts
with a momentum distribution with center k0 and width (delta k) (p 341).
He gives no derivation, he just states the answer in the form of the probability
amplitude squared (probability density), which is also a gaussian. I have
put French's result into the standard gaussian form:
Gaussian ref:
A e^-{[x-b]^2/2 c^2}
where
b = center of distribution
c = standard deviation
2c = width of distribution
! psi (x,t) |^2 = A e^-{[(x -(hbar k0/m) time]^2}/{2 [alpha + (hbar time/2
m)^2/alpha]}
where
k0 = (av or peak) momentum
delta x0 = 1.41/delta k
alpha = (delta x0)^2/4
so 'b' gives us the x center of the distribution.
b = (hbar k0/m) time
(Note if k0 is zero, then b = 0, which means the particle is not moving in the sense that it has no average velocity).
and '2c' = delta x, which is the width of the distribution for t > 0
c = sqrt{alpha + (hbar time/2 m)^2/alpha}
= sqrt{alpha [1 + (hbar time/2 m alpha)^2]}
= sqrt{(delta x0)^2/4 [1 + (hbar time/2 m ((delta x0)^2/4))^2]}
= (1/2) delta x0 sqrt{1 + (2 hbar time/m (delta x0)^2)^2}
delta x = 2c
= delta x0 sqrt{1 + [2 hbar time/m (delta x0)^2]^2}
( Note the dispersion of the particle, meaning the widening of its probability distribution, depends only on time and the initial position range (delta x0). (Delta x) @ t = 0 = (delta x0). Dispersion is independent of whether the particle is moving or not.)
We can define a threshold time (tau0) which makes (delta x) easier to interpret (French doesn't do this)
delta x = (delta x0) sqrt{1 + (time/tau0)^2}
where
tau0 = (m/2 hbar) (delta x0)^2
An online ref (Brewer) gets basically the same formula, except for a x4 higher scale factor, but he may have been calculating probability amplitude vs time rather than probability density as French does.
(Note the threshold time (tau0) for the dispersion to really get going depends strongly on (delta x0), so tight distributions begin to disperse more quickly. (see table below).)
The dispersion of the probability distribution is a 'root sum square' function. For (time < tau0) the dispersion is slow. At (time = tau0) delta x has widened to 1.41 delta x0. For (time > tau0) delta x expands linearly with time (with a scale factor that depends inversely on delta x0).
For time > tau0
delta x = delta x0 (time/tau0)
= (delta x0/[(m/2 hbar) (delta x0)^2]) time
= [(2 hbar/m)/delta x0] time
Dispersion rate
d(delta x)/d(time) = (2 hbar/m)/delta x0
Dispersion time (delta x0 to delta x)
time = (m/2 hbar) (delta x) (delta x0)
Putting in some numbers (which physicists do far too rarely). How long for delta x to expand to 1 meter in size from atomic size (delta x0 =10^-10 m)?
time = [m/2 hbar] (delta x) (delta x0)
= [9.1 x 10^-31/2 x 1.055 x 10^-34] 1 m x 10^-10 m
= [4.3 x 10^3] x 10^-10
= 4.3 x 10^-7 sec
(0.43 usec)
In about 1/2 usec where a free electron may be located expands from atom size to 1 meter!! Yikes! The smaller the initial spacing the faster the electron range expands to a specific size, because heisenberg pushes up the velocity. Below is a table for the time it takes the range of a free electron (represented by a gaussian wave packet) to expand to 1 meter from an initial dimension (size). The formula shows the time increases linearly with the starting dimension (delta x0).
Electron dispersion time vs initial dimension
(calculated for gaussian wave packet)
|
(delta x0) |
(m/2 hbar) (delta x0)^2 |
(delta x) |
(m/2 hbar) (delta x) (delta x0) |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
The mathematians say the standard deviation (c) of the fourier transform of a gaussian function is the inverse of the original gaussian standard deviation (1/c) (see Wikipedia). French gives the width of the momentum gaussian as (delta k) and the relation between it and delta x0 as
delta x0 = 1.41/delta k0
Well it's almost the inverse, but (for some reason) a sqrt{2} is thrown in. (Maybe because it's the probability distribution not amplitude distribution?)
k is defined as 2 pi/wavelength, so define @ t = 0 [delta k0 = 2 pi/(delta wavelength 0)]
delta x0 = 1.41/delta k0
= 1.41/[(2 pi/(delta wavelength 0)]
= (1.41/2 pi) (delta wavelength 0)
So we find
a simple relationship between how tightly x is known and the de Broglie
wavelength. For gaussians (at t =0) the width of the frequency distribution,
as measured in terms of wavelengths, divided by 2 pi is the same
as width of the x spacial probability distribution (except for the mysterious
1.41).
------------------------------------------------
Classical wave equation
(dy/dt)^2 = vel^2 (dy/dx)^2
with solution
y (x,t) = x0 e^i(kx - wt)
where
w = 2 pi freq
k = 2 pi/wavelength
check
(dy/dt)^2 = -w^2 y (x,t)
(dy/dx)^2 = -k^2 y (x.t)
so
vel^2 = [(dy/dt)^2]/[(dy/dx)^2]
= - w^2/-k^2
vel = w/k
= ([2 pi freq]/[2 pi/wavelength] = freq x wavelength)
------------------------------------------------
Gaussian formula
The 'normal'
gaussian formula is loved by all students because it is so easy to manipulate.
The product of two gaussians is a gaussian, and the fourier transform of
a gaussian is also a gaussian. In the case of a fourier transform a narrow
gassian (with st dev = c) transforms into a wide gaussian (st dev = 1/c)
and vice versa.
f(x) = a e-[(x-b)^2/2 c^2]
where
a = max height
b = center (mean value)
c = standard deviation
Graphically 'c' is half the width of the pulse measured at (approx) 60% of the peak height, because for (x = b +/-c) the function {e- [(x-b)^2/2 c^2} reduces to e^-(1/2) = 0.6065
The integral of f(x) from - infinity to + infinity = ac sqrt{2 pi}, so setting
a = 1/c sqrt{2pi}
normalizes the integral to the value of one.
In quantum
physics the probablility amplitude 'wave packet' of a localized free particle
is often chosen to be gaussian. This makes the square (probability density)
and fourier tranform of the probability amplitude also gaussian, and more
importantly it allows the Schrodinger time dependent equation to be solved.
The result is a wavefunction that (rapidly) spreads out over time due to
different frequencies in the wave packet traveling at different speeds.
-------------------------------------------------------------------
--Quantum
harmonic oscillator --- One of the few quantum mechanical systems
for which a simple exact solution is known. (V is proportional to x^2)
-- A wave packet is a localized disturbance that results from the sum of many different wave forms. If the packet is strongly localized, more frequencies are needed to allow the constructive superposition in the region of localization and destructive superposition outside the region.
-- The group velocity of a wave is the velocity with which the variations in the shape of the wave's amplitude (known as the modulation or envelope of the wave) propagate through space.
-- Because waves at different frequencies propagate at differing phase velocities in dispersive media, for a large frequency range (a narrow envelope in space) the observed pulse would change shape while traveling, making group velocity an unclear or useless quantity.
-- The function w(k), which gives w as a function of k, is known as the dispersion relation. If w is directly proportional to k, then the group velocity is exactly equal to the phase velocity.
In quantum mechanics w is proportional to k^2 causing the phase velocity to be half the group (envelope) velocity, as shown below:
w = E/hbar
= p^2/(2m hbar)
= hbar k^2/2m
where k = 2 pi/wavelength and wavelength = h/p
so k = 2 pi p/h = p/hbar
phase velocity
w/k = hbar k^2/2m k = hbar k/2m = hbar (p/hbar)/2 m = mv/2m = v/2
group velocity
dw/dk = 2 hbar k/2m = 2 hbar (p/hbar)/2m = p/m = v
---------------------------------------------------
-- The concept
of "group velocity" has meaning only when w is a linear function of k,
or nearly so. Then the derivative dw/dk is a constant and it doesn't make
any difference what value of k you evaluate the derivative at.
-- If dw/dk isn't constant, the wave packet changes shape as time passes, and eventually 'falls apart'.
-- For a group velocity to be meaningful, the wave packet has to be composed of wavenumbers in a narrow range.
-- Wave function of a photon (apparently) doesn't conventionally exist. Schordinger's equation is not relativistic and photons travel at c. Also Schordinger's equation has m in denominator (of course m = E/c^2). I found a reference to a published paper in physics forum (below) that attemps a derivation of the wave function of a photon, claiming only that "it may play a useful pedagogical role, providing a missing link between classical Maxwell theory and quantum electrondynamics."
http://www.cft.edu.pl/~birula/publ/APPPwf.pd
-- In the paper above it says dirac talked a lot about the role of the wave function in the interference of photons, but he never discusses it mathematically! Also he says there are statements in many textbooks that a wave function for the photon cannot be found.
-- (good photon description) An electromagnetic wave at 500 THz is a pattern of variations in the electric and magnetic field that moves through space at the speed c (and has periodic occurences of concurrently zero field strength). In order to interact with this wave (i.e. detect it), only discrete chunks of energy = 2 eV can be extracted. These extractions are the photons.
-- If you think of the proton as the size of a grain of salt, then the electron cloud would have about a ten foot radius. If you probe, you'll probably find the electron somewhere in that region.
-- So the (electron) cloud (in a hydrogen atom) can lower its potential energy by squishing in closer to the nucleus, but when it squishes in too far its kinetic energy goes up (due to Heisenberg) more than its potential energy goes down. So it settles at a happy medium, with the lowest possible energy, and that gives the cloud and thus the atom its size.
-- What's more it's not just the energy that is quantized, other properties that an electron can posess are also split into distinct units with no in betweens. The angular momentum is quantised, the spin is quantised, the component of the angular moment in any direction that you care to choose is quantised.
-- Each different shell is subdivided into one or more orbitals, each of which has a different angular momentum.
-- A freely moving electron with velocity 'v' can be considered to be a wave packet, which travelis in the direction of the electron motion 'v' and has wavefronts perpendicular to 'v'. (In other words the wave pakcet is more line a pulse or step)
-- The concept of de Broglie's wave in superconductors is applied to pairs of electrons called 'Cooper pairs'. The wave equation solution is the same expcept m = 2e and the 'v' is the sum of the two veloicityes. It can easily be zero for the pair (one electron +v and the other electron -v). This make the debroglie wavelength infinite and the wave phase (kx +vt) = 0 (or a constant). In a superconductor the waves of all the electron Cooper pairs connect to form a single conherent wave.
-- Notice one remarkable feature of the time independent schrodinger equation—the i on the left means that psi cannot be a real function.
-- Schrödinger's
wave equation has the same predictions made by the uncertainty principle
because uncertainty of location is built into the definition of a widespread
disturbance like a wave.
============================================================
Euler's formula
The wavefunction
is related to one of the most remarkable formulas in mathematics:
e^(i pi) = -1
This formula is at first mind boggling. It combines in a simple way three seemingly unrelated terms: 'e', pi, and (complex number) 'i' and out pops -1. This formula is called Euler identity and is a special case of a formula I well remember from math class called Euler's formula:
e^(i x) = cos(x) + i sin(x)
The proof of this can be seen by doing a Taylor expansion of e^y
e^y = 1 + y + (1/2!)y^2 + (1/3!)y^3 + (1/4!)y^4 +(1/5!)y^5 ...
setting y = ix and separating terms
e^(ix) = [1 - (1/2!)x^2 + (1/4!)x^4 ...] + i[ x - (1/3!)x^3 +(1/5!)x^5 ...]
The first expansion in brackets is cos(x) and the second expansion is sin(x), which proves (to a non-mathematician) that e^(i x) = cos(x) + i sin(x).
Setting x = wt [e^(iwt)] we get a rotating (with time) unity magnitude vector with angle wt that in cartesian terms is sin & cos waveforms in quadrature. Setting x = kx +wt [e^(i(kx + wt))] gives sin & cos quadrature waveforms that vary both in time and space.
The solutions
to the Schrodinger equation are all of the basic form e^i(kx - wt). For
a moving particle the (av) 'w' is the de Broglie frequency and 'k' is 2
pi x (1/de Broglie wavelength).
==============================================================
The probability of a specific event happening, like the probability of photon fired from a source arriving at a particular location or of finding a particle at a specific location, is found by working out a complex probability 'amplitude'. The square of the magnitude of the complex probability amplitude (technically the probability amplitude times its complex conjugate) gives the probability density, which when summed up, or integrated over a range, gives the probability of the event occurring, results that can be verified with experiments run many times.
Feynman comments in QED that probability amplitudes may seem bizarre (or something eq), but points out that it is second nature to physicists, because this approach to understanding the quantum world was developed in the 1920's, roughly eighty years ago.There are three separate ways the probability amplitude may be computed: Heisenberg's matrix mechanics, which (very likely is a matrix method, Feynman's path integral, which I think is what he is describing in his 'add the vectors for all possible ways' method in his popular book QED, and Schrodinger's wave equation, which was my introduction to quantum mechanics in school.
For photons the probability amplitude is the electric field (E field) of light with the caveat that the E 'field' can only be defined (measured?) when there are lots of photons together. Light beams superimposed appear to naturally sum their E field vectors, because measurable light intensity is found to be equal to the resultant E field vector magnitude squared. (In QED Feynman shows that the interferrance between photons bouncing off the front and back surfaces of glass (sort of) generate a reflection pattern that varies sinusoidally with the thickness of the glass.)
For massless photons, which travel at the speed of light:
E = h freq = cp
wavelength = c/freq
De Broglie generalized the wavelength formula:
wavelength = c/freq = hc/(h freq) = hc/E = hc/cp
= h/p
With 'c' dropping out the association with photons is loosened and de Broglie suggested in his 1924 Phd thesis that it (might) apply to all particles. This would mean that an electron has associated with it a wave whose wavelength varies with inversely to the electron's momentum (& speed). A striking success of this proposal was that it provided (sort of) an explanation of why (& how) electron orbitals in atoms are quantized, because it was found that in hydrogen an integer # of wavelengths just fit into the circumference of each orbital with one wavelength fitting into the lowest orbit.
Who first figured out that interger electron wavelength help make sense of Bohr's quantized orbits I have not been able to determine. It may have been a gradual realization in the years 1924 to 1926, which is about a decade after Bohr formulated his orbits. I read de Broglie thesis and all he says is, '(electron waves) can be regarded as a phase wave resonance condition for an electron in orbit about a proton.' He may have not been able to do the calculation. Schrodinger in 1926 applied his wave equation for electrons to hydrogen and was able to get quantitative results that matched experiment when he calculated hydrogen's spectral lines.In general [frequency x wavelength = velocity], so the 'frequency' of the electron wave is proportional to its kinetic energy.
freq = velocity/wavelength
= velocity/(h/p)
= (1/h) mv^2
It was soon confirmed that electrons had wave properties when experiments in 1927 revealed that an electron beam reflecting off a crystal showed interference effects, and the angles where the reflections peaked could be calculated from de Brolie's wavelength formula. (Two years later de Broglie was awarded the Nobel prize for physics)
With (monochromatic) light it was pretty clear from Maxwell's formulas that its wavelength was just the distance between peaks of a traveling sinusoidal (transverse) E & H field that varied inversely with the frequency or color of the light. But what did the 'wavelength' of an electron (or other particle) mean? How was this to be interpreted? It was pretty clear from the defraction experiments that electrons seemed to be interfering, sometimes canceling sometimes adding, in much the same way classical waves like water waves did.
In classical electromagnetic theory interference (& polarization) of light is explained and calculated by adding (technically superposition) E fields of the various beams. The intensity of light, which is what is actually measured, is proportional to the final electric field amplitude squared (E^2).
Clearly superposition of E fields and then squaring to find intensity is directly analogous with how matter waves (each a solution to Schrodinger's equation) are superimposed and then squared to find the probability of a quantum event or configuration. When de Broglie introduced matter waves and Schrodinger showed they were solutions to a linear equation, it was very natural to apply superposition and square the result in the manner of electromagnetic fields. It took a little while, however, for the understanding to arise that this approach yielded a probability for a quantum experiment.Schrodinger in 1926? came up with an equation, basically an energy equation written in quantum terms, that applied to matter waves. Solutions to the Schrodinger equation, at least those in bound states, are quantum amplitudes. The Schrodinger equation can be solved for standing waves in the cases where the electron is confined. This provides probability about where the electron is likely to be found. For free electrons the equation can be solved to show how the wave spreads out with time. This tells us that the that the region where a free electron is likely to be found expands with time. The cool figure below from the hyperphysics site shows how useful the Schrodinger equation is.

Range of applications for Schrodinger wave equation
(hyperphysics site)
The solutions to Schrodinger equation are of the form A e^(i x) =A cos(x) + i Asin(x), which is just an A amplitude vector with an angle 'x' plotted on a real hoz axis and complex vertical axis. More generally the solutions to the Schrodinger equation are all of the basic form e^i(kx - wt). For a moving particle the (av) 'w' is the de Broglie frequency and 'k' is 2 pi x (1/de Broglie wavelength).
In introductory quantum mechanics text books the Schrodinger wave equation is generally solved for five classic cases. The first three are one dimension standing waves for idealized potential wells. The 4th is a standing wave for an electron trapped in a potential well around a proton. The 5th case shows how the (probability amplitude) wave for a free electron whose location is known at t0 spreads out with time.
1) infinite well
With barriers
infinitely high there is zero probability electron is not in well, so the
wave, which is the probability amplitude, must be zero at both boundaries.
The wave solutions are sinewaves of integer related frequency increasing
in the well by half cycles [n/2 cycle = 1/2 cycle, 1 cycle, 1.5 cycle,
etc]. The probability (density) vs distance for the electron, which of
course is the probability wave amplitude squared, has a sin^2 shape.
This simple case demonstrates that energy of the electron is also quantized, increasing as n^2. A standing wave is the sum of two counter propargating waves, so a doubling of the frequency (n = 2) means the electrons in the propagating waves are moving twice as fast, because they have half the wavelength (wavelength = h/p), so they have four times the kinetic energy. Sure enough French derives E for the solutions and it increases as n^2, and E also goes up as 1/(well dimension)^2..
I made up the explanation that speed of a traveling wave goes up with n. Seems reasonable. Another explanation is Heisenberg uncertainty. The electron appears to be located (somewhere) within each half cycle, so as the half cycles shrink in dimension by 1/n velocity has to increase as n.2) finite well
3) V = x^2 restoring force
This is well
that has square law restoring force (V = x^2) and is easily solved. Since
a perturbation analysis of atom held by electron bonds in molecules are
often approx square law, this case allows calculation of vibration modes
of atoms in molecules. The solution has this char:..............
4) V = k/r potential well
A (single)
electron in an atom, bound by 1/r^2 force attraction to the nucleus, is
modelled as existing in a V = k/r potential well, where k = eZ The
wave equation solved in only the radial dimension yields the classic 's'
(spherical) orbits of hydrogen (& other one electron atoms). Compared
to the inner orbit the 2nd orbit has x4 radius (n^2), 1/2 electron velocity
and 1/4th kinetic energy, but higher potential energy. (A much more complex
solution in three spherical coordinates yields the classic family of chemistry
electron orbits?)
5) free electron (V = 0)
The 5th case
is an electron with no forces on it. At t0 there is assumed to be a known
probability amplitude reflecting probability about where the electron is
initially located. It is usually solved for assuming a gaussian position
waveform at t0, because then the math is simple. The envelope of the sinewave
solutions also comes out to have gaussian waveshape (plotted in momentum).
The wave solution shows that over time the region where we think the electron
could be expands with time, slowly at first and then more rapidly. The
expansion is due to the range of frequencies making up the wave in time
all of which have slightly different speeds. This case also has within
the envelope the de Broglie frequency, whose wavelength varies inversely
with the electron velocity.
Are electron de Broglie waves real or is it just a mathematical device for calculating probabilities based on constructive and destructive interference? de Broglie in his thesis speculated that they were real in the sense that he figured that the measurable E field of light was just a statistical average of photon 'waves'. Feynman too thought that the sum of paths model indicated that was really how photons travel. He says this repeatedly in his book QED. On the other hand Freeman Dyson taught that matter waves were just a mathematical tool with no reality. French also emphasizes (he puts it in italics) that matter waves:
Particle waves, however, are not associated with any macroscopic property (unlike the E field associated with photons). Therefore the "only conclusion to be drawn is that particle waves are an expression of the probabilistic behavior of large numbers of (identically prepared) particles -- and nothing else!" (emphasis French)An important point, which I find not clearly made in references, is that Schrodinger's wave equation does not apply to photons, at least it doesn't apply directly. For one thing Schordinger's equation is non-relativistic and of course photons move at the speed of light. Also Schrodinger's wave equation contains the parameter 'mass'. Schrodinger's equation is used to calculate how electrons respond. (Apparently) the electromagnetic theory of E fields allows calculation of some photon probabilities, but I doubt that this is sufficient. From Feynman's book QED there clearly are rules in quantum electrodynamics for calculating how photons and electrons interact, apparently in all cases.
Discussion of this point in physics forums are very confusing. One poster says there is an equivalent equation to Schordinger's for the photon, but others apppear to say it's not that simple. One poster references recent papers by a polish phyicist who is attempting to develope a wave theory for the photon ('On the wave function of the photon', 1994), which starts, "It is believed that certain matrix elements of the electromagnetic field operators in quantum electrodynamics, in close analogy with nonrelativistic quantum theory of massive particles, may be treated as photon wave functions." and "The very concept of the photon wave function is not new, but strangely enough it has never been systematically explored." and "One takes the energy density as a relative measure of the probability density to find the photon. ... After all, one detects photons by absorbing their energy: the photon is where its energy is localized."Well perhaps relevant is that electrons are used in place of photons in very high resolution microscopes. Why?
Electron microscope------------------------------------
Let's compare the de Broglie wavelength of a reasonably fast moving electron with a visible photon. At this point I have no idea what the speed of an electron is in an electron microscope, but knowing that electrons are pretty easily accelerated I am going to guess [v = 0.707 c]. For light I will use blue light.photon wavelength 475 nm = 4.75 x 10^-7 m (blue)
electron wavelength h/p = 6.63 x 10^-34/(9.1 x 10^-31)(0.707 x 3 x 10^8)
= 3.4 x 10^-12 m (@ 0.707 c)The electron wavelength (@ 0.707 C) comes out to be more than a 100,000 times smaller than the wavelength of a blue photon. 3 x 10^-12 m is on the order of 1/100th the size of an atom. Only 100V will acclerate electrons to about 1% c. This gives a wavelength near atomic dimensions (10^-10 m) and more than 1,000 times smaller than blue light. (Researching I find that practical electron microscopes are said to 'amplify' 2 million time vs 2 thousand times for the best optical microscopes.)
* Associated waves only really exist for 'large' numbers of (identically prepared) photons or electrons. He says specifically an individual photon has no "color". I think this statement is quite bizarre.
Color (frequency) for photon is the same as (extractable) energy, since for photons E = h freq. The photoelectric effect demonstated that individual photons differ in their energy level, and that energy level is calculable from the color/frequency seen when the light intensity is increased.He is less clear about individual electrons. (I think a wave packet describes an individual free electron, so I don't understand French here.)
* Photon waves are associated with a (seemingly) concrete macroscopic property, an oscillating electric field. In visible light its spacial orientation is light's polarization, and in a radio antenna it shakes the electrons in the antenna at the electric field frequency creating a measurable current. (Yet Feynman in the book QED makes it clear that photon waves are to be treated in quantum mechanics as probability amplitudes, and that a squaring is needed to find the probability or probability density.)
* Particle waves, however, are not associated with any macroscopic property. Therefore the "only conclusion to be drawn is that particle waves are an expression of the probabilistic behavior of large numbers of (identically prepared) particles -- and nothing else!" (emphasis French)
* The behavior of photons in a beam is independent of the intensity of the beam; the photons behave -- most conveniently! -- in a manner independent of one another. We can carry out experiments with a bean of visible light, and we are assured that this experiment will yield results equivalent to those for a low intensity experiment (assuming the same photon count). (p243)
What this means, I think, is that a classical analysis of light interference (or polarization) done classically for a light bean with E fields reinforcing and canceling results in the same mathematics as a quantum probability analysis for photons.--------------------------------------
Mathematically the E field of the of light from the 1st polarizer can be 'resolved' along the axis of the 2nd polarizer, meaning it can be considered as the vector sum of two components, one component aligned with the molecules of the 2nd polarizer, which will be absorbed, and the other component in quadrature, which will be transmitted. From trigonometry the E field amplitude of the transmitted component of light varies as cos (theta), where 'theta' is the angle between the polarizer disks. Since the intensity of light, meaning the energy it carries, is known to depends on E^2, this provides an explanation of the experimental result that the fraction of light (intensity) passing through two polarizers varies as cos^2(theta).
At low intensity where individual quantums enter the 2nd polarizer from the 1st polarizer the same cos^2(theta) result is found. French comments that if you think about it the implications of this result are "quite startling", since all the photons leaving the 1st polarizer have the same polarization state, yet the experimental result is that some are stopped by the 2nd polarizer and some are passed. The conclusion drawn is that the probability of photon transmission through the 2nd polarizer varies as cos^2(theta)'
In general the energy carried in a beam of light is proportional to E^2 since, because energy density (of the beam) is proportional to E^2 (1/2 uo E^2). French makes clear the relation between high numbers of photons and low intensity individual photon measurements. He says, you need not take a long time doing experiments with individual photons, because a quick experiment with visible beams of light will yield the same result much more quickly, because photons at high density, i.e. beams of light, act the same way as (large numbers of) individual photons.
Seems to me this must mean that treating electromagnetic fields as de Broglie waves to find photon probability must yield the same mathematics as treating light beams as E fields to be superimposed and squared to find intensity.-------------------------------------------------------------------------------------------
new state of matter at very low temperatures (170 x 10^-9 K ) where atoms reveal their quantum properties and quantum properties domininate, and a quantum thing can actually be seen "by the naked eye". It does not exist in anywhere in nature. First theorized by Einstein in 1924 (extending the work of Bose) and first made at NIST and at MIT in 1990's winning the Nobel prize 2001. At Harvard a condensate has been used to slow down light to a few meter/sec..
Explanation
from PBS show (MIT physics professor)
-- at very low temperatures atoms can be considered each as a bunch of
"wave packets"
-- as temperature drops the wavelengths of the atoms gets longer
-- at some point the (de Broglie) wavelengths start to overlap and individual
atoms "lose their
identity", they are everywhere at once, they are at rest, they are just
one big quantum system.
-- MIT cooled the atoms using their magnetic poles
-- NIST team started using laser cooling
-------------------------------------------------------------------------------------------
Nice college lecture slides (Univ of Wisconsin, undergraduate physics) on Introductory quantum mechanics
One dimensional box
n is the number
of (half) wavelengths that fit in box. Since (wavelength = h/p), velocity
goes up n and energy goes up as n^2. (In other word with n =2 electron
moves twice as fast leading to a double frequency standing wave and double
humped probability.) General quantum rule is quantum number 'n' determines
energy.
Two & three dimensional box
Below is probability
in a two dimentsional box with different n's on each axis. The (2,1) and
(1,2) case have the same energy as each other, but higher energy than the
ground state (1,1).
(top) Ground state
(bot) Double standing waves on the axis with x2 velocity
and x4 (kinetic) energy
Here's the three dimension box equivalent:
(top) Ground state
(bot) Double standing waves on the axis with x2 velocity
and x4 (kinetic) energy

What? Where does the rt side 2p taurus apply?
Other refereces all show 2p states as three sets (x,y,z)
of double lobes (sort of like center)
Left OK (see below) More tauruses?
Orbits from Wikipedia.
(confirms above (left) 3d orbit)
-- The classical model of the atom doesn't fail because the electron is approximated as a point particle, it fails because the radial states are not quantized.(physics forum)
-- Using classical
electrostatics, the amount of energy required to assemble a sphere of constant
charge density, of radius r_e and charge e is: E = (3/5)e^2/(4pi e0
r). Ignoring the factor of 3/5, if this is equated to the relativistic
energy of the electron (E = mc^2) and solved for r, the electron classical
radius is obtained. (physics forum)
mc^2 = e^2/(4pi e0 r)
r = e^2/(4pi e0 m c^2)
= [e^2/(4pi e0 hbar c)] x [hbar/mc]
= alpha (hbar/mc)
classical electron radius
---------------------------------------------------------------------------------------------------------------------
Principle of 'least action' (3/2011)
I have long
known of the 'principle of least action', but never really understood it.
I knew for example from a biograph of Feynman that he heard about this
from his HS physics teacher, and it was important in his formulation of
quantum mechanics. But only in reading a new book on Feynman, 'Quantum
Man' by Lawrence Krauss, did I gain some understanding of the principle
of least action.
Krauss (p14) says the princple of least action states that the difference between the kinetic energy of (a freely moving) object at any instant and its potential energy at the same instant, when calculated for every point on the path and then added up along the path, will be smaller for the actual path the object takes than for any other trajectory. So what this means he points out is that, an object somehow adjusts its motion so that the kinetic energy and potential energy are as closely matched, on average, as is possible.
Potential energy trades for kinetic energy
Only in recent
years had I begun to understand that falling objects are converting potential
energy into kinetic energy. For an object falling in earth's gravity (ignoring
air losses) or an electron falling into a proton (to make a hydrogen atom)
its gain of kinetic energy is exactly balanced by its loss of potential
energy. It is (in effect) converting potential energy into kinetic energy.
From an 'action' viewpoint the action is zero all along the path
as potential energy converts to kinetic energy.
For an object falling in earth's gravity (with the usual simplifying assumption that gravity is constant) PE = mgh, so loses in potential energy translate to gains in kinetic energy and are both are proportional to distance fallen. Thus velocity can only increases as the square root of distance fallen, x2 speed means x4 distance. But since by F=ma, speed is proportional to time, so x2 time means x4 distance, i.e. distance goes up as time squared.Krauss does not do equations, so he does not explain 'action' mathematically. So I turned to Wikipedia to see the math. A glance at 'Principle of Least Action' in Wikipedia shows why I didn't understand it. The simple explaination of Krauss is not there. But by using three Wikipedia articles ('Principle of Least Action' => Action (physics) and 'Lagrangian') the basic math becomes clear.
From Lagrangian
Langrangian = kinetic energy - potential energy
L = T - V
From Action (physics)
-- The action
(S) is represented as an integral over time, taken along the path of the
system between the initial time and the final time of the development of
the system.
S = integral{L dt}
= integral{(T - V) dt}
So the the
'action' is not a distance integral, but a time integral (while moving
along a path) of the kinetic energy minus potential energy.